Wigner–Seitz Cell on:
[Wikipedia]
[Google]
[Amazon]
The Wigner–Seitz cell, named after  The unique property of a crystal is that its
The unique property of a crystal is that its
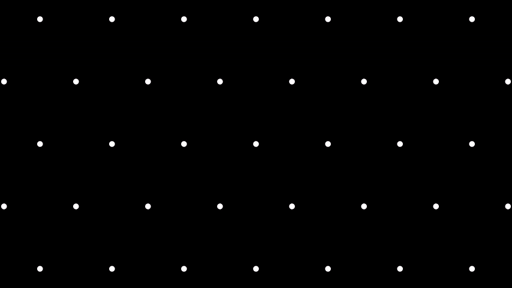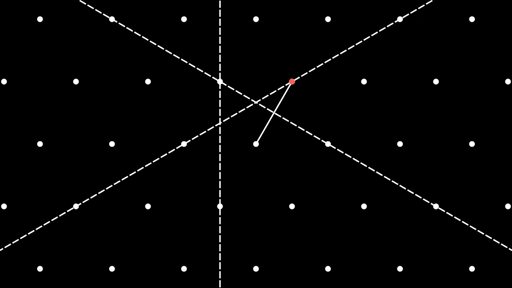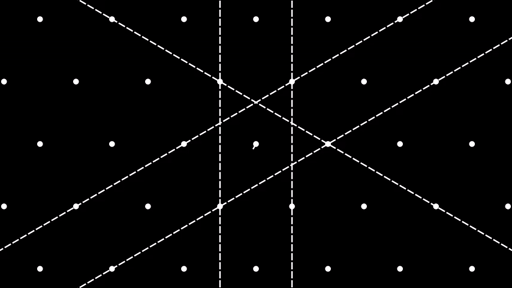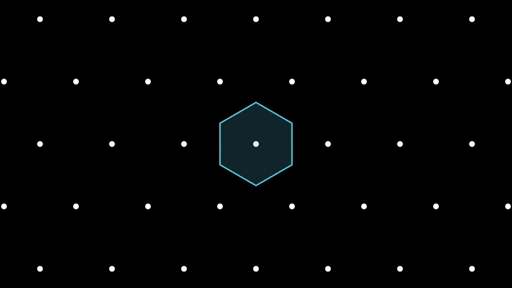 The cell may be chosen by first picking a lattice point. After a point is chosen, lines are drawn to all nearby lattice points. At the midpoint of each line, another line is drawn normal to each of the first set of lines. The smallest area enclosed in this way is called the Wigner–Seitz primitive cell.
For a 3-dimensional lattice, the steps are analogous, but in step 2 instead of drawing perpendicular lines, perpendicular planes are drawn at the midpoint of the lines between the lattice points.
As in the case of all primitive cells, all area or space within the lattice can be filled by Wigner–Seitz cells and there will be no gaps.
Nearby lattice points are continually examined until the area or volume enclosed is the correct area or volume for a
The cell may be chosen by first picking a lattice point. After a point is chosen, lines are drawn to all nearby lattice points. At the midpoint of each line, another line is drawn normal to each of the first set of lines. The smallest area enclosed in this way is called the Wigner–Seitz primitive cell.
For a 3-dimensional lattice, the steps are analogous, but in step 2 instead of drawing perpendicular lines, perpendicular planes are drawn at the midpoint of the lines between the lattice points.
As in the case of all primitive cells, all area or space within the lattice can be filled by Wigner–Seitz cells and there will be no gaps.
Nearby lattice points are continually examined until the area or volume enclosed is the correct area or volume for a
Eugene Wigner
Eugene Paul Wigner (, ; November 17, 1902 – January 1, 1995) was a Hungarian-American theoretical physicist who also contributed to mathematical physics. He received the Nobel Prize in Physics in 1963 "for his contributions to the theory of th ...
and Frederick Seitz, is a primitive cell
In geometry, biology, mineralogy and solid state physics, a unit cell is a repeating unit formed by the vectors spanning the points of a lattice. Despite its suggestive name, the unit cell (unlike a unit vector, for example) does not necessaril ...
which has been constructed by applying Voronoi decomposition to a crystal lattice
In crystallography, crystal structure is a description of ordered arrangement of atoms, ions, or molecules in a crystal, crystalline material. Ordered structures occur from intrinsic nature of constituent particles to form symmetric patterns that ...
. It is used in the study of crystal
A crystal or crystalline solid is a solid material whose constituents (such as atoms, molecules, or ions) are arranged in a highly ordered microscopic structure, forming a crystal lattice that extends in all directions. In addition, macros ...
line materials in crystallography
Crystallography is the branch of science devoted to the study of molecular and crystalline structure and properties. The word ''crystallography'' is derived from the Ancient Greek word (; "clear ice, rock-crystal"), and (; "to write"). In J ...
.
 The unique property of a crystal is that its
The unique property of a crystal is that its atom
Atoms are the basic particles of the chemical elements. An atom consists of a atomic nucleus, nucleus of protons and generally neutrons, surrounded by an electromagnetically bound swarm of electrons. The chemical elements are distinguished fr ...
s are arranged in a regular three-dimensional array called a lattice. All the properties attributed to crystalline materials stem from this highly ordered structure. Such a structure exhibits discrete
Discrete may refer to:
*Discrete particle or quantum in physics, for example in quantum theory
* Discrete device, an electronic component with just one circuit element, either passive or active, other than an integrated circuit
* Discrete group, ...
translational symmetry
In physics and mathematics, continuous translational symmetry is the invariance of a system of equations under any translation (without rotation). Discrete translational symmetry is invariant under discrete translation.
Analogously, an operato ...
. In order to model and study such a periodic system, one needs a mathematical "handle" to describe the symmetry and hence draw conclusions about the material properties consequent to this symmetry. The Wigner–Seitz cell is a means to achieve this.
A Wigner–Seitz cell is an example of a primitive cell
In geometry, biology, mineralogy and solid state physics, a unit cell is a repeating unit formed by the vectors spanning the points of a lattice. Despite its suggestive name, the unit cell (unlike a unit vector, for example) does not necessaril ...
, which is a unit cell
In geometry, biology, mineralogy and solid state physics, a unit cell is a repeating unit formed by the vectors spanning the points of a lattice. Despite its suggestive name, the unit cell (unlike a unit vector
In mathematics, a unit vector i ...
containing exactly one lattice point. For any given lattice, there are an infinite number of possible primitive cells. However there is only one Wigner–Seitz cell for any given lattice. It is the locus of points in space that are closer to that lattice point than to any of the other lattice points.
A Wigner–Seitz cell, like any primitive cell, is a fundamental domain
Given a topological space and a group acting on it, the images of a single point under the group action form an orbit of the action. A fundamental domain or fundamental region is a subset of the space which contains exactly one point from each ...
for the discrete translation symmetry of the lattice. The primitive cell of the reciprocal lattice
Reciprocal lattice is a concept associated with solids with translational symmetry which plays a major role in many areas such as X-ray and electron diffraction as well as the energies of electrons in a solid. It emerges from the Fourier tran ...
in momentum space is called the Brillouin zone
In mathematics and solid state physics, the first Brillouin zone (named after Léon Brillouin) is a uniquely defined primitive cell in reciprocal space
Reciprocal lattice is a concept associated with solids with translational symmetry whic ...
.
Overview
Background
The concept of Voronoi decomposition was investigated byPeter Gustav Lejeune Dirichlet
Johann Peter Gustav Lejeune Dirichlet (; ; 13 February 1805 – 5 May 1859) was a German mathematician. In number theory, he proved special cases of Fermat's last theorem and created analytic number theory. In analysis, he advanced the theory o ...
, leading to the name ''Dirichlet domain''. Further contributions were made from Evgraf Fedorov
Evgraf Stepanovich Fedorov (, – 21 May 1919) was a Russian mathematician, crystallographer and mineralogist.
Fedorov was born in the Russian city of Orenburg. His father was a topographical engineer. The family later moved to Saint Petersb ...
, (''Fedorov parallelohedron''), Georgy Voronoy
Georgy Feodosevich Voronyi (; ; 28 April 1868 – 20 November 1908) was an Imperial Russian mathematician of Ukrainians, Ukrainian descent noted for defining the Voronoi diagram.
Biography
Voronyi was born in the village of Zhuravka, Pyriatyn, in ...
(''Voronoi polyhedron''), and Paul Niggli (''Wirkungsbereich'').
The application to condensed matter physics
Condensed matter physics is the field of physics that deals with the macroscopic and microscopic physical properties of matter, especially the solid and liquid State of matter, phases, that arise from electromagnetic forces between atoms and elec ...
was first proposed by Eugene Wigner
Eugene Paul Wigner (, ; November 17, 1902 – January 1, 1995) was a Hungarian-American theoretical physicist who also contributed to mathematical physics. He received the Nobel Prize in Physics in 1963 "for his contributions to the theory of th ...
and Frederick Seitz in a 1933 paper, where it was used to solve the Schrödinger equation
The Schrödinger equation is a partial differential equation that governs the wave function of a non-relativistic quantum-mechanical system. Its discovery was a significant landmark in the development of quantum mechanics. It is named after E ...
for free electrons in elemental sodium
Sodium is a chemical element; it has Symbol (chemistry), symbol Na (from Neo-Latin ) and atomic number 11. It is a soft, silvery-white, highly reactive metal. Sodium is an alkali metal, being in group 1 element, group 1 of the peri ...
. They approximated the shape of the Wigner–Seitz cell in sodium, which is a truncated octahedron, as a sphere of equal volume, and solved the Schrödinger equation exactly using periodic boundary conditions
Periodic boundary conditions (PBCs) are a set of boundary conditions which are often chosen for approximating a large (infinite) system by using a small part called a ''unit cell''. PBCs are often used in computer simulations and mathematical mod ...
, which require at the surface of the sphere. A similar calculation which also accounted for the non-spherical nature of the Wigner–Seitz cell was performed later by John C. Slater.
There are only five topologically distinct polyhedra which tile three-dimensional space
In geometry, a three-dimensional space (3D space, 3-space or, rarely, tri-dimensional space) is a mathematical space in which three values ('' coordinates'') are required to determine the position of a point. Most commonly, it is the three- ...
, . These are referred to as the parallelohedra
In geometry, a parallelohedron or Fedorov polyhedron is a convex polyhedron that can be translated without rotations to fill Euclidean space, producing a honeycomb in which all copies of the polyhedron meet face-to-face. Evgraf Fedorov identif ...
. They are the subject of mathematical interest, such as in higher dimensions. These five parallelohedra can be used to classify the three dimensional lattices using the concept of a projective plane, as suggested by John Horton Conway
John Horton Conway (26 December 1937 – 11 April 2020) was an English mathematician. He was active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory. He also made contributions to many b ...
and Neil Sloane
__NOTOC__
Neil James Alexander Sloane FLSW (born October 10, 1939) is a British-American mathematician. His major contributions are in the fields of combinatorics, error-correcting codes, and sphere packing. Sloane is best known for being the cre ...
. However, while a topological classification considers any affine transformation
In Euclidean geometry, an affine transformation or affinity (from the Latin, '' affinis'', "connected with") is a geometric transformation that preserves lines and parallelism, but not necessarily Euclidean distances and angles.
More general ...
to lead to an identical class, a more specific classification leads to 24 distinct classes of voronoi polyhedra with parallel edges which tile space. For example, the rectangular cuboid
A rectangular cuboid is a special case of a cuboid with rectangular faces in which all of its dihedral angles are right angles. This shape is also called rectangular parallelepiped or orthogonal parallelepiped.
Many writers just call these ...
, right square prism, and cube
A cube or regular hexahedron is a three-dimensional space, three-dimensional solid object in geometry, which is bounded by six congruent square (geometry), square faces, a type of polyhedron. It has twelve congruent edges and eight vertices. It i ...
belong to the same topological class, but are distinguished by different ratios of their sides. This classification of the 24 types of voronoi polyhedra for Bravais lattices was first laid out by Boris Delaunay
Boris Nikolayevich Delaunay or Delone (; 15 March 1890 – 17 July 1980) was a Soviet and Russian mathematician, mountain climber, and the father of physicist, Nikolai Borisovich Delone. He is best known for the Delaunay triangulation.
Biograph ...
.
Definition
The Wigner–Seitz cell around a lattice point is defined as the locus of points in space that are closer to that lattice point than to any of the other lattice points. It can be shown mathematically that a Wigner–Seitz cell is aprimitive cell
In geometry, biology, mineralogy and solid state physics, a unit cell is a repeating unit formed by the vectors spanning the points of a lattice. Despite its suggestive name, the unit cell (unlike a unit vector, for example) does not necessaril ...
. This implies that the cell spans the entire direct space without leaving any gaps or holes, a property known as tessellation
A tessellation or tiling is the covering of a surface, often a plane, using one or more geometric shapes, called ''tiles'', with no overlaps and no gaps. In mathematics, tessellation can be generalized to higher dimensions and a variety ...
.
Constructing the cell
The general mathematical concept embodied in a Wigner–Seitz cell is more commonly called aVoronoi cell
In mathematics, a Voronoi diagram is a partition of a plane into regions close to each of a given set of objects. It can be classified also as a tessellation. In the simplest case, these objects are just finitely many points in the plane (calle ...
, and the partition of the plane into these cells for a given set of point sites is known as a Voronoi diagram
In mathematics, a Voronoi diagram is a partition of a plane into regions close to each of a given set of objects. It can be classified also as a tessellation. In the simplest case, these objects are just finitely many points in the plane (calle ...
.
 The cell may be chosen by first picking a lattice point. After a point is chosen, lines are drawn to all nearby lattice points. At the midpoint of each line, another line is drawn normal to each of the first set of lines. The smallest area enclosed in this way is called the Wigner–Seitz primitive cell.
For a 3-dimensional lattice, the steps are analogous, but in step 2 instead of drawing perpendicular lines, perpendicular planes are drawn at the midpoint of the lines between the lattice points.
As in the case of all primitive cells, all area or space within the lattice can be filled by Wigner–Seitz cells and there will be no gaps.
Nearby lattice points are continually examined until the area or volume enclosed is the correct area or volume for a
The cell may be chosen by first picking a lattice point. After a point is chosen, lines are drawn to all nearby lattice points. At the midpoint of each line, another line is drawn normal to each of the first set of lines. The smallest area enclosed in this way is called the Wigner–Seitz primitive cell.
For a 3-dimensional lattice, the steps are analogous, but in step 2 instead of drawing perpendicular lines, perpendicular planes are drawn at the midpoint of the lines between the lattice points.
As in the case of all primitive cells, all area or space within the lattice can be filled by Wigner–Seitz cells and there will be no gaps.
Nearby lattice points are continually examined until the area or volume enclosed is the correct area or volume for a primitive cell
In geometry, biology, mineralogy and solid state physics, a unit cell is a repeating unit formed by the vectors spanning the points of a lattice. Despite its suggestive name, the unit cell (unlike a unit vector, for example) does not necessaril ...
. Alternatively, if the basis vectors of the lattice are reduced using lattice reduction
In mathematics, the goal of lattice basis reduction is to find a basis with short, nearly orthogonal vectors when given an integer lattice basis as input. This is realized using different algorithms, whose running time is usually at least expon ...
only a set number of lattice points need to be used. In two-dimensions only the lattice points that make up the 4 unit cells that share a vertex with the origin need to be used. In three-dimensions only the lattice points that make up the 8 unit cells that share a vertex with the origin need to be used.
Composite lattices
For composite lattices, (crystals which have more than one vector in their basis) each single lattice point represents multiple atoms. We can break apart each Wigner–Seitz cell into subcells by further Voronoi decomposition according to the closest atom, instead of the closest lattice point. For example, the diamond crystal structure contains a two atom basis. In diamond, carbon atoms have tetrahedral sp3 bonding, but since tetrahedra do not tile space, the voronoi decomposition of the diamond crystal structure is actually thetriakis truncated tetrahedral honeycomb
The triakis truncated tetrahedral honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space made up of triakis truncated tetrahedra. It was discovered in 1914.
Voronoi tessellation
It is the Voronoi tessellation of the ca ...
. Another example is applying Voronoi decomposition to the atoms in the A15 phases, which forms the polyhedral approximation of the Weaire–Phelan structure.
Symmetry
The Wigner–Seitz cell always has the same point symmetry as the underlyingBravais lattice
In geometry and crystallography, a Bravais lattice, named after , is an infinite array of discrete points generated by a set of discrete translation operations described in three dimensional space by
: \mathbf = n_1 \mathbf_1 + n_2 \mathbf_2 ...
. For example, the cube
A cube or regular hexahedron is a three-dimensional space, three-dimensional solid object in geometry, which is bounded by six congruent square (geometry), square faces, a type of polyhedron. It has twelve congruent edges and eight vertices. It i ...
, truncated octahedron
In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces (8 regular hexagon, hexagons and 6 Squa ...
, and rhombic dodecahedron
In geometry, the rhombic dodecahedron is a Polyhedron#Convex_polyhedra, convex polyhedron with 12 congruence (geometry), congruent rhombus, rhombic face (geometry), faces. It has 24 edge (geometry), edges, and 14 vertex (geometry), vertices of 2 ...
have point symmetry Oh, since the respective Bravais lattices used to generate them all belong to the cubic lattice system
In crystallography, a crystal system is a set of point groups (a group of geometric symmetries with at least one fixed point). A lattice system is a set of Bravais lattices (an infinite array of discrete points). Space groups (symmetry groups ...
, which has Oh point symmetry.
Brillouin zone
In practice, the Wigner–Seitz cell itself is actually rarely used as a description of direct space, where the conventionalunit cell
In geometry, biology, mineralogy and solid state physics, a unit cell is a repeating unit formed by the vectors spanning the points of a lattice. Despite its suggestive name, the unit cell (unlike a unit vector
In mathematics, a unit vector i ...
s are usually used instead. However, the same decomposition is extremely important when applied to reciprocal space
Reciprocal lattice is a concept associated with solids with translational symmetry which plays a major role in many areas such as X-ray diffraction, X-ray and Electron diffraction, electron diffraction as well as the Electronic band structure, e ...
. The Wigner–Seitz cell in the reciprocal space is called the Brillouin zone
In mathematics and solid state physics, the first Brillouin zone (named after Léon Brillouin) is a uniquely defined primitive cell in reciprocal space
Reciprocal lattice is a concept associated with solids with translational symmetry whic ...
, which is used in constructing band diagrams to determine whether a material will be a conductor, semiconductor
A semiconductor is a material with electrical conductivity between that of a conductor and an insulator. Its conductivity can be modified by adding impurities (" doping") to its crystal structure. When two regions with different doping level ...
or an insulator.
See also
*Delaunay triangulation
In computational geometry, a Delaunay triangulation or Delone triangulation of a set of points in the plane subdivides their convex hull into triangles whose circumcircles do not contain any of the points; that is, each circumcircle has its gen ...
* Coordination geometry
* Crystal field theory
* Wigner crystal
References
{{DEFAULTSORT:Wigner-Seitz Cell Crystallography Mineralogy