theorems in functional analysis

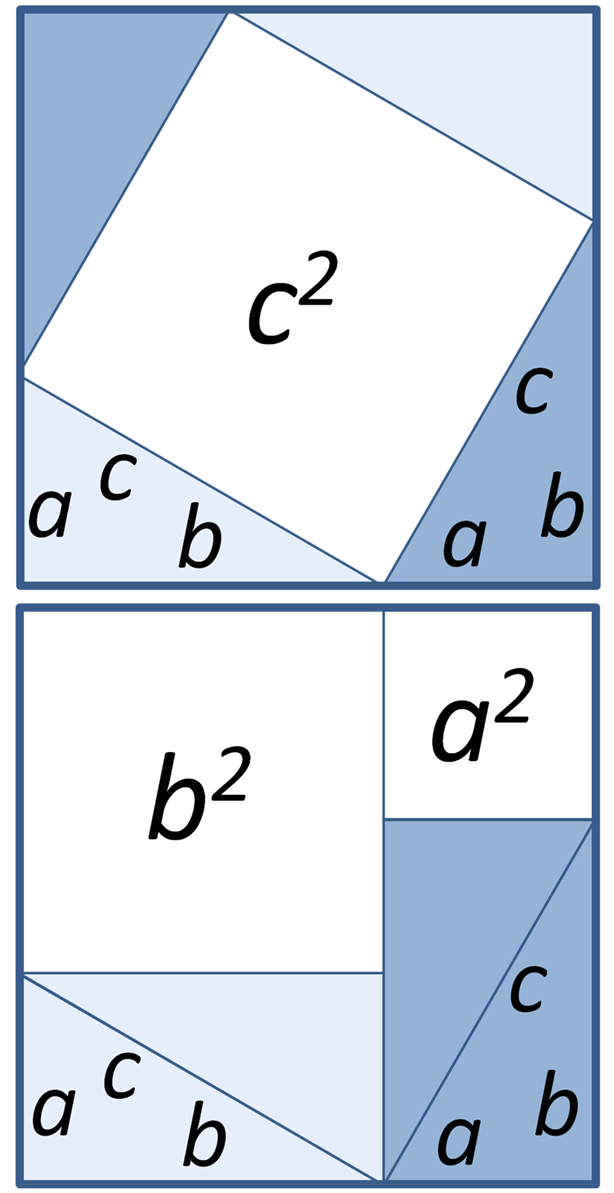mathematics Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...

, a theorem is a statement that has been proved, or can be proved. The ''proof'' of a theorem is a

logical argument An argument is a statement or group of statements called premises intended to determine the degree of truth or acceptability of another statement called conclusion. Arguments can be studied from three main perspectives: the logical, the dialectic ...

that uses the inference rules of a

deductive system A formal system is an abstract structure used for inferring theorems from axioms according to a set of rules. These rules, which are used for carrying out the inference of theorems from axioms, are the logical calculus of the formal system. A form ...

to establish that the theorem is a

logical consequence Logical consequence (also entailment) is a fundamental concept in logic, which describes the relationship between statements that hold true when one statement logically ''follows from'' one or more statements. A valid logical argument is on ...

of the

axiom An axiom, postulate, or assumption is a statement that is taken to be true, to serve as a premise or starting point for further reasoning and arguments. The word comes from the Ancient Greek word (), meaning 'that which is thought worthy or f ...

s and previously proved theorems. In the mainstream of mathematics, the axioms and the inference rules are commonly left implicit, and, in this case, they are almost always those of

Zermelo–Fraenkel set theory In set theory, Zermelo–Fraenkel set theory, named after mathematicians Ernst Zermelo and Abraham Fraenkel, is an axiomatic system that was proposed in the early twentieth century in order to formulate a theory of sets free of paradoxes such as ...

with the

axiom of choice In mathematics, the axiom of choice, or AC, is an axiom of set theory equivalent to the statement that ''a Ca