symmetry order on:
[Wikipedia]
[Google]
[Amazon]
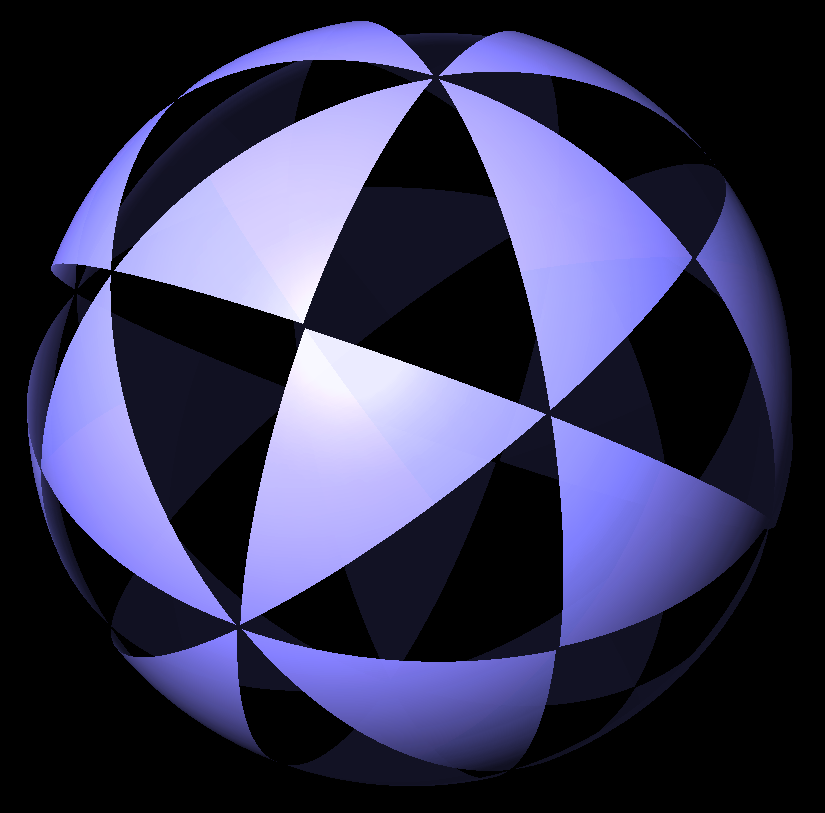The symmetry number or symmetry order of an object is the number of different but indistinguishable (or equivalent) arrangements (or views) of the object, that is, it is the order of its
The symmetry number or symmetry order of an object is the number of different but indistinguishable (or equivalent) arrangements (or views) of the object, that is, it is the order of its symmetry group
In group theory, the symmetry group of a geometric object is the group of all transformations under which the object is invariant, endowed with the group operation of composition. Such a transformation is an invertible mapping of the ambient ...
. The object can be a molecule, crystal lattice, lattice, tiling, or in general any kind of mathematical object that admits symmetries.
In statistical thermodynamics
In physics, statistical mechanics is a mathematical framework that applies statistical methods and probability theory to large assemblies of microscopic entities. It does not assume or postulate any natural laws, but explains the macroscopic b ...
, the symmetry number corrects for any overcounting of equivalent molecular conformations in the partition function. In this sense, the symmetry number depends upon how the partition function is formulated. For example, if one writes the partition function of ethane
Ethane ( , ) is an organic chemical compound with chemical formula . At standard temperature and pressure, ethane is a colorless, odorless gas. Like many hydrocarbons, ethane is isolated on an industrial scale from natural gas and as a petr ...
so that the integral includes full rotation of a methyl, then the 3-fold rotational symmetry of the methyl group contributes a factor of 3 to the symmetry number; but if one writes the partition function so that the integral includes only one rotational energy well of the methyl, then the methyl rotation does not contribute to the symmetry number.
Symmetry Numbers for Rigid, Flexible and Fluxional Molecules: Theory and Applications. M.K. Gilson and K. K. Irikura. J. Phys. Chem. B 114:16304-16317, 2010.
See also
*Group theory
In abstract algebra, group theory studies the algebraic structures known as group (mathematics), groups.
The concept of a group is central to abstract algebra: other well-known algebraic structures, such as ring (mathematics), rings, field ...
, a branch of mathematics which discusses symmetry, symmetry groups, symmetry spaces, symmetry operations
* Point groups in three dimensions
In geometry, a point group in three dimensions is an isometry group in three dimensions that leaves the origin fixed, or correspondingly, an isometry group of a sphere. It is a subgroup of the orthogonal group O(3), the group of all isometrie ...
* Space group
In mathematics, physics and chemistry, a space group is the symmetry group of an object in space, usually in three dimensions. The elements of a space group (its symmetry operations) are the rigid transformations of an object that leave it unchan ...
in 3 dimensions
* Molecular symmetry
Molecular symmetry in chemistry describes the symmetry present in molecules and the classification of these molecules according to their symmetry. Molecular symmetry is a fundamental concept in chemistry, as it can be used to predict or explain m ...
* List of the 230 crystallographic 3D space groups
In mathematics, physics and chemistry, a space group is the symmetry group of an object in space, usually in three dimensions. The elements of a space group (its symmetry operations) are the rigid transformations of an object that leave it unchan ...
* Fixed points of isometry groups in Euclidean space A fixed point of an isometry group is a point that is a fixed point for every isometry in the group. For any isometry group in Euclidean space the set of fixed points is either empty or an affine space.
For an object, any unique centre and, more g ...
* Symmetric group
In abstract algebra, the symmetric group defined over any set is the group whose elements are all the bijections from the set to itself, and whose group operation is the composition of functions. In particular, the finite symmetric group \m ...
, mathematics
* Symmetry group
In group theory, the symmetry group of a geometric object is the group of all transformations under which the object is invariant, endowed with the group operation of composition. Such a transformation is an invertible mapping of the ambient ...
, mathematics
References
{{reflist Symmetry