Square root of 3

The square root of 3 is the positive real number that, when multiplied by itself, gives the number 3. It is denoted mathematically as $\sqrt$ or $3^$. It is more precisely called the principal square root of 3 to distinguish it from the negative number with the same property. The square root of 3 is an irrational number. It is also known as Theodorus' constant, after Theodorus of Cyrene, who proved its irrationality.

, its numerical value in decimal notation had been computed to at least ten billion digits. Its decimal expansion, written here to 65 decimal places, is given by :
:

The fraction $\frac$ (...) can be used as a good approximation. Despite having a denominator of only 56, it differs from the correct value by less than $\frac$ (approximately $9.2\times 10^$, with a relative error of $5\times 10^$). The rounded value of is correct to within 0.01% of the actual value.

The fraction $\frac$ (...) is accurate to $1\times 10^$.

Archimedes reported a range for its value: $(\frac)^>3>(\frac)^$.

The lower limit $\frac$ is an accurate approximation for $\sqrt$ to $\frac$ (six decimal places, relative error $3 \times 10^$) and the upper limit $\frac$ to $\frac$ (four decimal places, relative error $1\times 10^$).

Expressions

It can be expressed as the continued fraction .

So it is true to say:
:$\begin1 & 2 \\1 & 3 \end^n = \begina_ & a_ \\a_ & a_ \end$
then when $n\to\infty$ :
:$\sqrt = 2 \cdot \frac -1$

It can also be expressed by generalized continued fractions such as
: ; -4, -4, -4, ...= 2 - \cfrac

which is evaluated at every second term.

Geometry and trigonometry

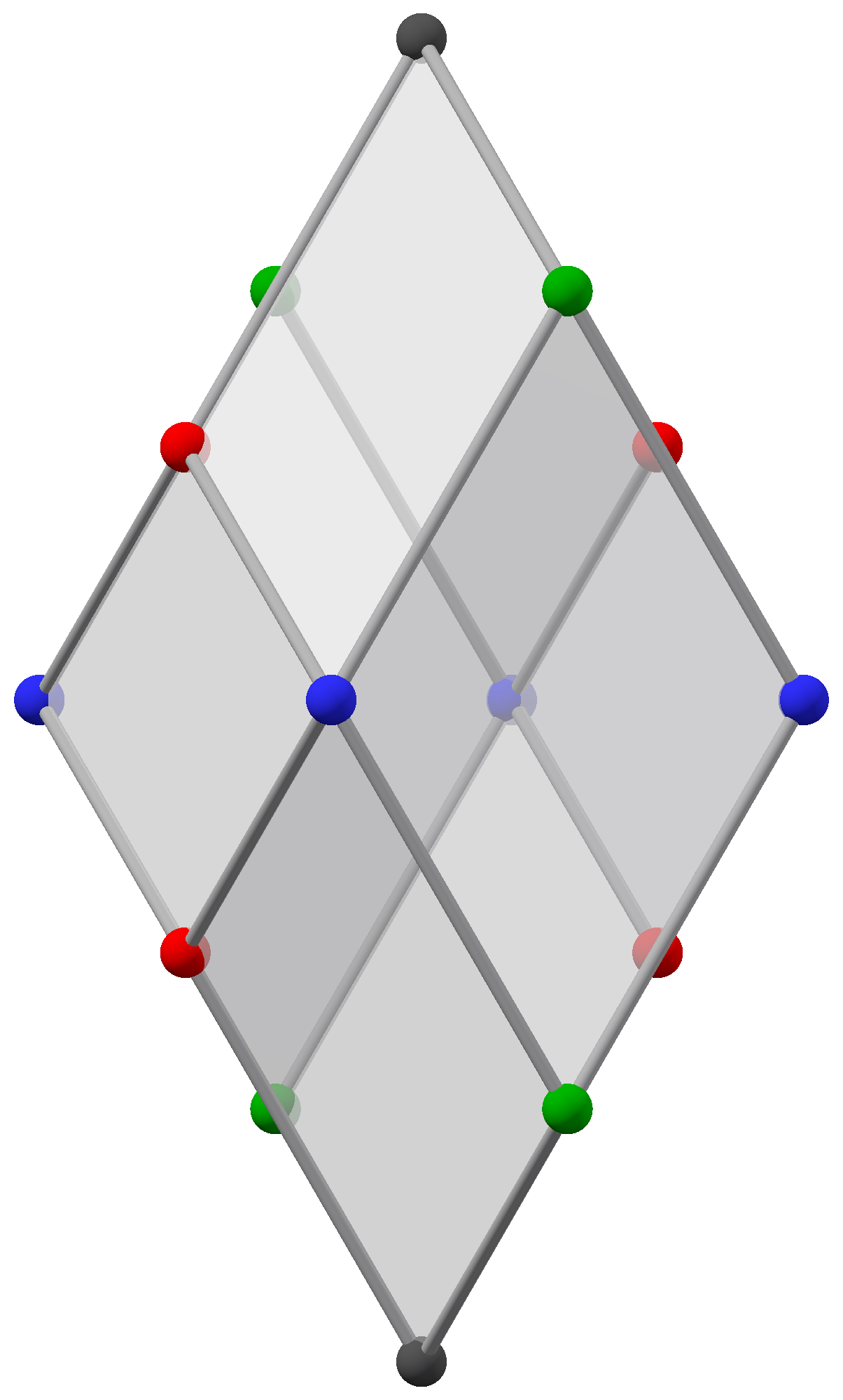
The square root of 3 can be found as the leg length of an equilateral triangle that encompasses a circle with a diameter of 1.

If an equilateral triangle with sides of length 1 is cut into two equal halves, by bisecting an internal angle across to make a right angle with one side, the right angle triangle's hypotenuse is length one, and the sides are of length $\frac$ and $\frac$. From this, $\tan=\sqrt$, $\sin=\frac$, and $\cos=\frac$.

The square root of 3 also appears in algebraic expressions for various other trigonometric constants, including the sines of 3°, 12°, 15°, 21°, 24°, 33°, 39°, 48°, 51°, 57°, 66°, 69°, 75°, 78°, 84°, and 87°.

It is the distance between parallel sides of a regular hexagon with sides of length 1.

It is the length of the space diagonal of a unit cube.

The vesica piscis has a major axis to minor axis ratio equal to $1:\sqrt$. This can be shown by constructing two equilateral triangles within it.

Other uses

Power engineering

In power engineering, the voltage between two phases in a three-phase system equals $\sqrt$ times the line to neutral voltage. This is because any two phases are 120° apart, and two points on a circle 120 degrees apart are separated by $\sqrt$ times the radius (see geometry examples above).

See also

* Square root of 2
* Square root of 5

Other references

*
*
*

References

External links

Theodorus' Constant
at MathWorld

Kevin Brown

E. B. Davis

{{DEFAULTSORT:Square root of three
Quadratic irrational numbers
Mathematical constants