Runcinated Pentachoron on:
[Wikipedia]
[Google]
[Amazon]
In four-dimensional

 The runcitruncated
The runcitruncated

 (4.6.6)
(4.6.6)
20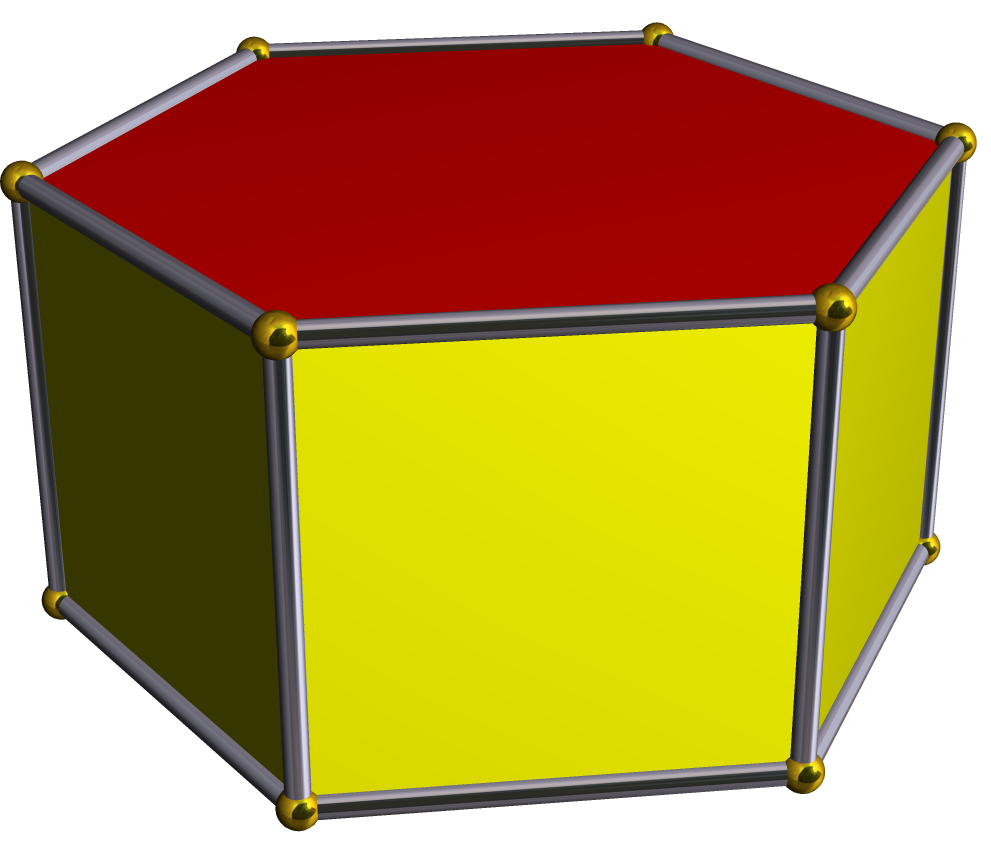 (4.4.6)
, -
, bgcolor=#e7dcc3, Faces
, 150
, 90{4}
(4.4.6)
, -
, bgcolor=#e7dcc3, Faces
, 150
, 90{4}
60{6} , - , bgcolor=#e7dcc3, Edges , colspan=2, 240 , - , bgcolor=#e7dcc3, Vertices , colspan=2, 120 , - , bgcolor=#e7dcc3,

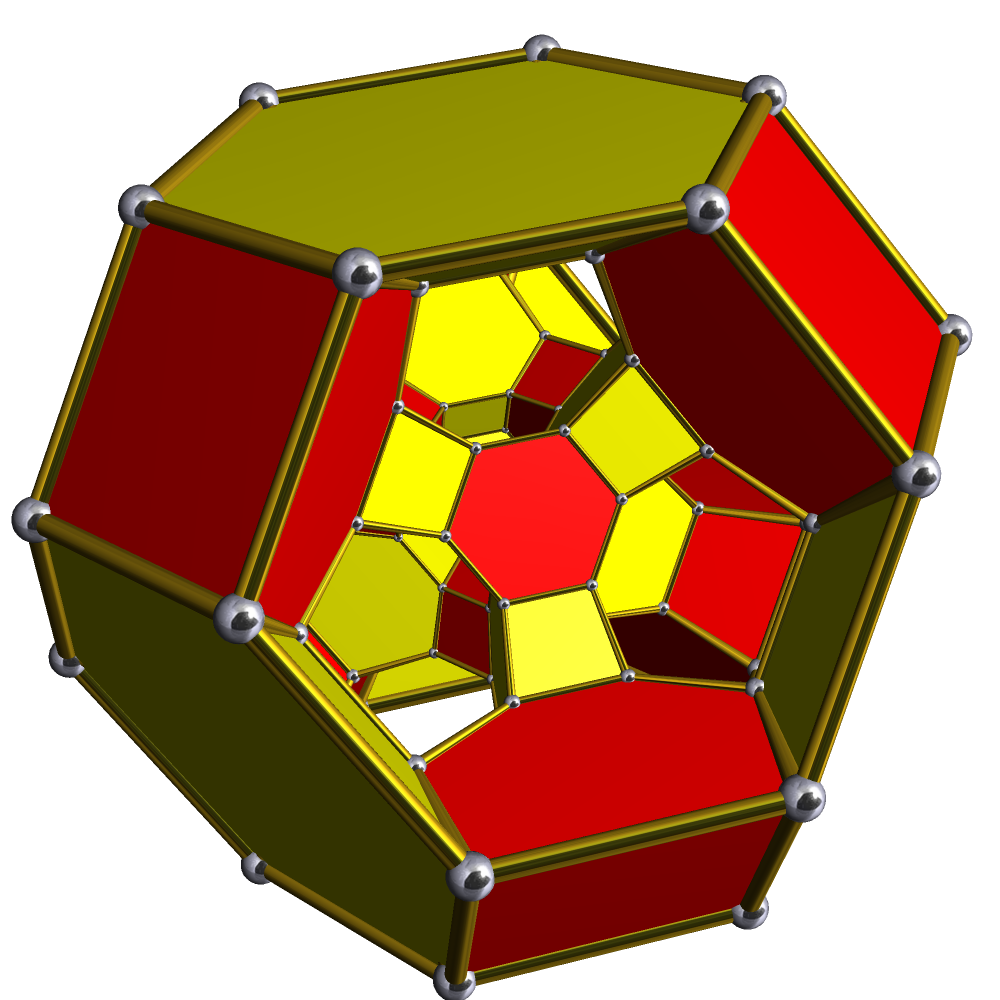
Omnitruncated 5-cell ,
Dual to omnitruncated 5-cell
Perspective
Centered on



 The full snub 5-cell or omnisnub 5-cell, defined as an Alternation (geometry), alternation of the omnitruncated 5-cell, cannot be made uniform, but it can be given Coxeter diagram , and symmetry +, order 120, and constructed from 90 cells: 10
The full snub 5-cell or omnisnub 5-cell, defined as an Alternation (geometry), alternation of the omnitruncated 5-cell, cannot be made uniform, but it can be given Coxeter diagram , and symmetry +, order 120, and constructed from 90 cells: 10
*** (Paper 22) H.S.M. Coxeter, ''Regular and Semi Regular Polytopes I'', ath. Zeit. 46 (1940) 380-407, MR 2,10*** (Paper 23) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes II'', ath. Zeit. 188 (1985) 559-591*** (Paper 24) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes III'', ath. Zeit. 200 (1988) 3-45* Norman Johnson ''Uniform Polytopes'', Manuscript (1991) ** N.W. Johnson: ''The Theory of Uniform Polytopes and Honeycombs'', Ph.D. * * o3x3x3o - spid, x3x3o3x - prip, x3x3x3x - gippid {{Polytopes 4-polytopes
geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, a runcinated 5-cell is a convex uniform 4-polytope
In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.
There are 47 non-prismatic convex uniform 4-polytopes. There ...
, being a runcination
In geometry, runcination is an operation that cuts a regular polytope (or honeycomb) simultaneously along the faces, edges, and vertices, creating new facets in place of the original face, edge, and vertex centers.
It is a higher order truncatio ...
(a 3rd order truncation, up to face-planing) of the regular 5-cell
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
.
There are 3 unique degrees of runcinations of the 5-cell, including with permutations, truncations, and cantellations.
Runcinated 5-cell
The runcinated 5-cell or small prismatodecachoron is constructed by expanding thecells
Cell most often refers to:
* Cell (biology), the functional basic unit of life
Cell may also refer to:
Locations
* Monastic cell, a small room, hut, or cave in which a religious recluse lives, alternatively the small precursor of a monastery w ...
of a 5-cell
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
radially and filling in the gaps with triangular prism
Prism usually refers to:
* Prism (optics), a transparent optical component with flat surfaces that refract light
* Prism (geometry), a kind of polyhedron
Prism may also refer to:
Science and mathematics
* Prism (geology), a type of sedimentary ...
s (which are the face prisms and edge figures) and tetrahedra
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the ...
(cells of the dual 5-cell). It consists of 10 tetrahedra and 20 triangular prisms. The 10 tetrahedra correspond with the cells of a 5-cell and its dual.
Topologically, under its highest symmetry, 3,3,3
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
E. L. Elte
Emanuel Lodewijk Elte (16 March 1881 in Amsterdam – 9 April 1943 in Sobibór) Emanuël Lodewijk Elte
...
identified it in 1912 as a semiregular polytope.
...
Alternative names
* Runcinated5-cell
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
( Norman Johnson)
* Runcinated pentachoron
* Runcinated 4-simplex
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
* Expanded 5-cell/4-simplex/pentachoron
* Small prismatodecachoron (Acronym: Spid) (Jonathan Bowers)
Structure
Two of the ten tetrahedral cells meet at each vertex. The triangular prisms lie between them, joined to them by their triangular faces and to each other by their square faces. Each triangular prism is joined to its neighbouring triangular prisms in ''anti'' orientation (i.e., if edges A and B in the shared square face are joined to the triangular faces of one prism, then it is the other two edges that are joined to the triangular faces of the other prism); thus each pair of adjacent prisms, if rotated into the samehyperplane
In geometry, a hyperplane is a subspace whose dimension is one less than that of its '' ambient space''. For example, if a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hype ...
, would form a gyrobifastigium
In geometry, the gyrobifastigium is the 26th Johnson solid (). It can be constructed by joining two face-regular triangular prisms along corresponding square faces, giving a quarter-turn to one prism. It is the only Johnson solid that can tile ...
.
Dissection
The ''runcinated 5-cell'' can be dissected by a centralcuboctahedron
A cuboctahedron is a polyhedron with 8 triangular faces and 6 square faces. A cuboctahedron has 12 identical vertices, with 2 triangles and 2 squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such, it ...
into two tetrahedral cupola. This dissection is analogous to the 3D cuboctahedron
A cuboctahedron is a polyhedron with 8 triangular faces and 6 square faces. A cuboctahedron has 12 identical vertices, with 2 triangles and 2 squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such, it ...
being dissected by a central hexagon into two triangular cupola
In geometry, the triangular cupola is one of the Johnson solids (). It can be seen as half a cuboctahedron.
Formulae
The following formulae for the volume (V), the surface area (A) and the height (H) can be used if all faces are regular, wi ...
.
: 
Images
Coordinates
TheCartesian coordinate
A Cartesian coordinate system (, ) in a plane is a coordinate system that specifies each point uniquely by a pair of numerical coordinates, which are the signed distances to the point from two fixed perpendicular oriented lines, measured i ...
s of the vertices of an origin-centered runcinated 5-cell with edge length 2 are:
An alternate simpler set of coordinates can be made in 5-space, as 20 permutations of:
: (0,1,1,1,2)
This construction exists as one of 32 orthant facets
A facet is a flat surface of a geometric shape, e.g., of a cut gemstone.
Facet may also refer to:
Arts, entertainment, and media
* ''Facets'' (album), an album by Jim Croce
* ''Facets'', a 1980 album by jazz pianist Monty Alexander and his tri ...
of the runcinated 5-orthoplex
In five-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order truncation (runcination) of the regular 5-orthoplex.
There are 8 runcinations of the 5-orthoplex with permutations of truncations, and cantellati ...
.
A second construction in 5-space, from the center of a rectified 5-orthoplex
In five-dimensional geometry, a rectified 5-orthoplex is a convex uniform 5-polytope, being a rectification of the regular 5-orthoplex.
There are 5 degrees of rectifications for any 5-polytope, the zeroth here being the 5-orthoplex itself, and t ...
is given by coordinate permutations of:
: (1,-1,0,0,0)
Root vectors
Its 20 vertices represent the root vectors of thesimple Lie group
In mathematics, a simple Lie group is a connected non-abelian Lie group ''G'' which does not have nontrivial connected normal subgroups. The list of simple Lie groups can be used to read off the list of simple Lie algebras and Riemannian symme ...
A4. It is also the vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
for the 5-cell honeycomb
In four-dimensional Euclidean geometry, the 4-simplex honeycomb, 5-cell honeycomb or pentachoric-dispentachoric honeycomb is a space-filling tessellation honeycomb. It is composed of 5-cells and rectified 5-cells facets in a ratio of 1:1. Struct ...
in 4-space.
Cross-sections
The maximal cross-section of the runcinated 5-cell with a 3-dimensionalhyperplane
In geometry, a hyperplane is a subspace whose dimension is one less than that of its '' ambient space''. For example, if a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hype ...
is a cuboctahedron
A cuboctahedron is a polyhedron with 8 triangular faces and 6 square faces. A cuboctahedron has 12 identical vertices, with 2 triangles and 2 squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such, it ...
. This cross-section divides the runcinated 5-cell into two tetrahedral hypercupolae consisting of 5 tetrahedra and 10 triangular prisms each.
Projections
The tetrahedron-firstorthographic projection
Orthographic projection (also orthogonal projection and analemma) is a means of representing three-dimensional objects in two dimensions. Orthographic projection is a form of parallel projection in which all the projection lines are orthogonal ...
of the runcinated 5-cell into 3-dimensional space has a cuboctahedral envelope. The structure of this projection is as follows:
* The cuboctahedral envelope is divided internally as follows:
:* Four flattened tetrahedra join 4 of the triangular faces of the cuboctahedron to a central tetrahedron. These are the images of 5 of the tetrahedral cells.
:* The 6 square faces of the cuboctahedron are joined to the edges of the central tetrahedron via distorted triangular prisms. These are the images of 6 of the triangular prism cells.
:* The other 4 triangular faces are joined to the central tetrahedron via 4 triangular prisms (distorted by projection). These are the images of another 4 of the triangular prism cells.
:* This accounts for half of the runcinated 5-cell (5 tetrahedra and 10 triangular prisms), which may be thought of as the 'northern hemisphere'.
* The other half, the 'southern hemisphere', corresponds to an isomorphic division of the cuboctahedron in dual orientation, in which the central tetrahedron is dual to the one in the first half. The triangular faces of the cuboctahedron join the triangular prisms in one hemisphere to the flattened tetrahedra in the other hemisphere, and vice versa. Thus, the southern hemisphere contains another 5 tetrahedra and another 10 triangular prisms, making the total of 10 tetrahedra and 20 triangular prisms.
Related skew polyhedron
Theregular skew polyhedron
In geometry, the regular skew polyhedra are generalizations to the set of regular polyhedra which include the possibility of nonplanar faces or vertex figures. Coxeter looked at skew vertex figures which created new 4-dimensional regular polyhe ...
, , exists in 4-space with 6 squares around each vertex, in a zig-zagging nonplanar vertex figure. These square faces can be seen on the runcinated 5-cell, using all 60 edges and 20 vertices. The 40 triangular faces of the runcinated 5-cell can be seen as removed. The dual regular skew polyhedron, , is similarly related to the hexagonal faces of the bitruncated 5-cell
In geometry, a truncated 5-cell is a uniform 4-polytope (4-dimensional uniform polytope) formed as the truncation of the regular 5-cell.
There are two degrees of truncations, including a bitruncation.
Truncated 5-cell
The truncated 5-cell, ...
.
Runcitruncated 5-cell
 The runcitruncated
The runcitruncated 5-cell
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
or prismatorhombated pentachoron is composed of 60 vertices, 150 edges, 120 faces, and 30 cells. The cells are: 5 truncated tetrahedra
In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 equilateral triangle faces, 12 vertices and 18 edges (of two types). It can be constructed by truncating all 4 vertices of a regular tetrahedron ...
, 10 hexagonal prism
In geometry, the hexagonal prism is a prism with hexagonal base. Prisms are polyhedrons; this polyhedron has 8 faces, 18 edges, and 12 vertices..
Since it has 8 faces, it is an octahedron. However, the term ''octahedron'' is primarily used t ...
s, 10 triangular prism
In geometry, a triangular prism is a three-sided prism; it is a polyhedron made of a triangular base, a translated copy, and 3 faces joining corresponding sides. A right triangular prism has rectangular sides, otherwise it is ''oblique''. ...
s, and 5 cuboctahedra. Each vertex is surrounded by five cells: one truncated tetrahedron, two hexagonal prisms, one triangular prism, and one cuboctahedron; the vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
is a rectangular pyramid.
Alternative names
* Runcitruncated pentachoron * Runcitruncated4-simplex
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
* Diprismatodispentachoron
* Prismatorhombated pentachoron (Acronym: prip) (Jonathan Bowers)
Images
Coordinates
TheCartesian coordinate
A Cartesian coordinate system (, ) in a plane is a coordinate system that specifies each point uniquely by a pair of numerical coordinates, which are the signed distances to the point from two fixed perpendicular oriented lines, measured i ...
s of an origin-centered runcitruncated 5-cell having edge length 2 are:
{, class="wikitable collapsible collapsed"
! colspan=2, Coordinates
, -
,
:
:
:
:
:
:
:
:
:
,
:
:
:
:
:
:
:
:
:
,
:
:
:
:
:
:
:
:
:
The vertices can be more simply constructed on a hyperplane
In geometry, a hyperplane is a subspace whose dimension is one less than that of its '' ambient space''. For example, if a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hype ...
in 5-space, as the permutation
In mathematics, a permutation of a set is, loosely speaking, an arrangement of its members into a sequence or linear order, or if the set is already ordered, a rearrangement of its elements. The word "permutation" also refers to the act or p ...
s of:
: (0,1,1,2,3)
This construction is from the positive orthant facet
Facets () are flat faces on geometric shapes. The organization of naturally occurring facets was key to early developments in crystallography, since they reflect the underlying symmetry of the crystal structure. Gemstones commonly have facets cu ...
of the runcitruncated 5-orthoplex
In five-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order truncation ( runcination) of the regular 5-orthoplex.
There are 8 runcinations of the 5-orthoplex with permutations of truncations, and cantella ...
.
Omnitruncated 5-cell
{, class="wikitable" align="right" style="margin-left:10px" width="250" , - , bgcolor=#e7dcc3 align=center colspan=3, Omnitruncated 5-cell , - , bgcolor=#ffffff align=center colspan=3,
Schlegel diagram
In geometry, a Schlegel diagram is a projection of a polytope from \mathbb^d into \mathbb^ through a point just outside one of its facets. The resulting entity is a polytopal subdivision of the facet in \mathbb^ that, together with the ori ...
with half of the truncated octahedral cells shown.
, -
, bgcolor=#e7dcc3, Type
, colspan=2, Uniform 4-polytope
In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.
There are 47 non-prismatic convex uniform 4-polytopes. There ...
, -
, bgcolor=#e7dcc3, Schläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to mor ...
, colspan=2, t0,1,2,3{3,3,3}
, -
, bgcolor=#e7dcc3, Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington t ...
, colspan=2,
, -
, bgcolor=#e7dcc3, Cells
, 30
, 10  (4.6.6)
(4.6.6)20
 (4.4.6)
, -
, bgcolor=#e7dcc3, Faces
, 150
, 90{4}
(4.4.6)
, -
, bgcolor=#e7dcc3, Faces
, 150
, 90{4}60{6} , - , bgcolor=#e7dcc3, Edges , colspan=2, 240 , - , bgcolor=#e7dcc3, Vertices , colspan=2, 120 , - , bgcolor=#e7dcc3,
Vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
, colspan=2, 

Phyllic disphenoid
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular Pyramid (geometry), pyramid, is a polyhedron composed of four triangular Face (geometry), faces, six straight Edge (geometry), edges, and four vertex ( ...
, -
, bgcolor=#e7dcc3, Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean ref ...
, colspan=2, Aut
AUT may refer to the following.
Locations
*Austria (ISO 3166-1 country code)
* Agongointo-Zoungoudo Underground Town, Benin
*Aktio–Preveza Undersea Tunnel, Greece
*Airstrip on Atauro Island, East Timor (IATA airport code)
Organizations
* Arr ...
(A4), , order 240
, -
, bgcolor=#e7dcc3, Properties
, colspan=2, convex
Convex or convexity may refer to:
Science and technology
* Convex lens, in optics
Mathematics
* Convex set, containing the whole line segment that joins points
** Convex polygon, a polygon which encloses a convex set of points
** Convex polytop ...
, isogonal, zonotope
In geometry, a zonohedron is a convex polyhedron that is centrally symmetric, every face of which is a polygon that is centrally symmetric (a zonogon). Any zonohedron may equivalently be described as the Minkowski sum of a set of line segments in ...
, -
, bgcolor=#e7dcc3, Uniform index
, colspan=2, '' 8'' 9 '' 10''
The omnitruncated 5-cell or great prismatodecachoron is composed of 120 vertices, 240 edges, 150 faces (90 squares
In Euclidean geometry, a square is a regular quadrilateral, which means that it has four equal sides and four equal angles (90-degree angles, π/2 radian angles, or right angles). It can also be defined as a rectangle with two equal-length ad ...
and 60 hexagon
In geometry, a hexagon (from Greek , , meaning "six", and , , meaning "corner, angle") is a six-sided polygon. The total of the internal angles of any simple (non-self-intersecting) hexagon is 720°.
Regular hexagon
A ''regular hexagon'' h ...
s), and 30 cells. The cells are: 10 truncated octahedra
In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces (8 regular hexagons and 6 squares), 36 ...
, and 20 hexagonal prism
In geometry, the hexagonal prism is a prism with hexagonal base. Prisms are polyhedrons; this polyhedron has 8 faces, 18 edges, and 12 vertices..
Since it has 8 faces, it is an octahedron. However, the term ''octahedron'' is primarily used t ...
s. Each vertex is surrounded by four cells: two truncated octahedra, and two hexagonal prisms, arranged in two phyllic disphenoidal vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
s.
Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
calls this Hinton's polytope after C. H. Hinton
Charles Howard Hinton (1853 – 30 April 1907) was a British mathematician and writer of science fiction works titled ''Scientific Romances''. He was interested in higher dimensions, particularly the fourth dimension. He is known for coining th ...
, who described it in his book ''The Fourth Dimension'' in 1906. It forms a uniform honeycomb
In geometry, a uniform honeycomb or uniform tessellation or infinite uniform polytope, is a vertex-transitive honeycomb made from uniform polytope facets. All of its vertices are identical and there is the same combination and arrangement of fac ...
which Coxeter calls Hinton's honeycomb.''The Beauty of Geometry: Twelve Essays'' (1999), Dover Publications, , (The classification of Zonohededra, page 73)
Alternative names
* Omnitruncated5-cell
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
* Omnitruncated pentachoron
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
* Omnitruncated 4-simplex
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
* Great prismatodecachoron (Acronym: gippid) (Jonathan Bowers)
* Hinton's polytope (Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
)
Images
{, class=wikitable , +Net
Net or net may refer to:
Mathematics and physics
* Net (mathematics), a filter-like topological generalization of a sequence
* Net, a linear system of divisors of dimension 2
* Net (polyhedron), an arrangement of polygons that can be folded u ...
, 
Omnitruncated 5-cell ,

Dual to omnitruncated 5-cell
Perspective projections
{, class=wikitable width=600 , + , - align=center ,
Perspective
Schlegel diagram
In geometry, a Schlegel diagram is a projection of a polytope from \mathbb^d into \mathbb^ through a point just outside one of its facets. The resulting entity is a polytopal subdivision of the facet in \mathbb^ that, together with the ori ...
Centered on
truncated octahedron
In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces (8 regular hexagons and 6 squares), 36 ...
, 
Stereographic projection
In mathematics, a stereographic projection is a perspective projection of the sphere, through a specific point on the sphere (the ''pole'' or ''center of projection''), onto a plane (the ''projection plane'') perpendicular to the diameter th ...
Permutohedron
Just as thetruncated octahedron
In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces (8 regular hexagons and 6 squares), 36 ...
is the permutohedron
In mathematics, the permutohedron of order ''n'' is an (''n'' − 1)-dimensional polytope embedded in an ''n''-dimensional space. Its vertex coordinates (labels) are the permutations of the first ''n'' natural numbers. The edges iden ...
of order 4, the omnitruncated 5-cell is the permutohedron of order 5.
The omnitruncated 5-cell is a zonotope
In geometry, a zonohedron is a convex polyhedron that is centrally symmetric, every face of which is a polygon that is centrally symmetric (a zonogon). Any zonohedron may equivalently be described as the Minkowski sum of a set of line segments in ...
, the Minkowski sum
In geometry, the Minkowski sum (also known as dilation) of two sets of position vectors ''A'' and ''B'' in Euclidean space is formed by adding each vector in ''A'' to each vector in ''B'', i.e., the set
: A + B = \.
Analogously, the Minkowsk ...
of five line segments parallel to the five lines through the origin and the five vertices of the 5-cell.
Tessellations
The omnitruncated 5-cell honeycomb can tessellate 4-dimensional space by translational copies of this cell, each with 3 hypercells around each face. This honeycomb'sCoxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington t ...
is . Unlike the analogous honeycomb in three dimensions, the bitruncated cubic honeycomb
The bitruncated cubic honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space made up of truncated octahedra (or, equivalently, bitruncated cubes). It has 4 truncated octahedra around each vertex. Being composed entirely of t ...
which has three different Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean ref ...
Wythoff construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
s, this honeycomb has only one such construction.
Symmetry
The omnitruncated 5-cell has extended pentachoric symmetry, , order 240. Thevertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
of the omnitruncated 5-cell represents the Goursat tetrahedron
In geometry, a Goursat tetrahedron is a tetrahedral fundamental domain of a Wythoff construction. Each tetrahedral face represents a reflection hyperplane on 3-dimensional surfaces: the 3-sphere, Euclidean 3-space, and hyperbolic 3-space. Coxeter n ...
of the ,3,3Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean ref ...
. The extended symmetry comes from a 2-fold rotation across the middle order-3 branch, and is represented more explicitly as +[3,3,3.
:
Coordinates
TheCartesian coordinate
A Cartesian coordinate system (, ) in a plane is a coordinate system that specifies each point uniquely by a pair of numerical coordinates, which are the signed distances to the point from two fixed perpendicular oriented lines, measured i ...
s of the vertices of an origin-centered omnitruncated 5-cell having edge length 2 are:
{, class=wikitable
,
:
:
:
:
:
:
:
:
:
,
:
:
:
:
:
:
:
,
:
:
:
:
:
:
:
These vertices can be more simply obtained in 5-space as the 120 permutation
In mathematics, a permutation of a set is, loosely speaking, an arrangement of its members into a sequence or linear order, or if the set is already ordered, a rearrangement of its elements. The word "permutation" also refers to the act or p ...
s of (0,1,2,3,4).
This construction is from the positive orthant facet
Facets () are flat faces on geometric shapes. The organization of naturally occurring facets was key to early developments in crystallography, since they reflect the underlying symmetry of the crystal structure. Gemstones commonly have facets cu ...
of the runcicantitruncated 5-orthoplex, t0,1,2,3{3,3,3,4}, .
Related polytopes
Nonuniform variants with ,3,3symmetry and two types of truncated octahedra can be doubled by placing the two types of truncated octahedra on each other to produce a nonuniform polychoron with 10truncated octahedra
In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces (8 regular hexagons and 6 squares), 36 ...
, two types of 40 hexagonal prism
In geometry, the hexagonal prism is a prism with hexagonal base. Prisms are polyhedrons; this polyhedron has 8 faces, 18 edges, and 12 vertices..
Since it has 8 faces, it is an octahedron. However, the term ''octahedron'' is primarily used t ...
s (20 ditrigonal prisms and 20 ditrigonal trapezoprisms), two kinds of 90 rectangular trapezoprisms (30 with ''D2d'' symmetry and 60 with ''C2v'' symmetry), and 240 vertices. Its vertex figure is an irregular triangular bipyramid
In geometry, the triangular bipyramid (or dipyramid) is a type of hexahedron, being the first in the infinite set of face-transitive bipyramids. It is the dual of the triangular prism with 6 isosceles triangle faces.
As the name suggests, i ...
.

Vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
This polychoron can then be alternated to produce another nonuniform polychoron with 10 icosahedra
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetri ...
, two types of 40 octahedra
In geometry, an octahedron (plural: octahedra, octahedrons) is a polyhedron with eight faces. The term is most commonly used to refer to the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at ...
(20 with ''S6'' symmetry and 20 with ''D3'' symmetry), three kinds of 210 tetrahedra
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the ...
(30 tetragonal disphenoids, 60 phyllic disphenoids, and 120 irregular tetrahedra), and 120 vertices. It has a symmetry of 3,3,3
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
sup>+], order 120.

Vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
Full snub 5-cell
 The full snub 5-cell or omnisnub 5-cell, defined as an Alternation (geometry), alternation of the omnitruncated 5-cell, cannot be made uniform, but it can be given Coxeter diagram , and symmetry +, order 120, and constructed from 90 cells: 10
The full snub 5-cell or omnisnub 5-cell, defined as an Alternation (geometry), alternation of the omnitruncated 5-cell, cannot be made uniform, but it can be given Coxeter diagram , and symmetry +, order 120, and constructed from 90 cells: 10 icosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetric ...
s, 20 octahedron
In geometry, an octahedron (plural: octahedra, octahedrons) is a polyhedron with eight faces. The term is most commonly used to refer to the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at e ...
s, and 60 tetrahedron
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the ...
s filling the gaps at the deleted vertices. It has 300 faces (triangles), 270 edges, and 60 vertices.
Topologically, under its highest symmetry, +, the 10 icosahedra have ''T'' (chiral tetrahedral) symmetry, while the 20 octahedra have ''D3'' symmetry and the 60 tetrahedra have ''C2'' symmetry.
Related polytopes
These polytopes are a part of a family of 9Uniform 4-polytope
In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.
There are 47 non-prismatic convex uniform 4-polytopes. There ...
constructed from the ,3,3Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean ref ...
.
Notes
References
*H.S.M. Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington t ...
:
** H.S.M. Coxeter, ''Regular Polytopes'', 3rd Edition, Dover New York, 1973
** Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995,*** (Paper 22) H.S.M. Coxeter, ''Regular and Semi Regular Polytopes I'', ath. Zeit. 46 (1940) 380-407, MR 2,10*** (Paper 23) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes II'', ath. Zeit. 188 (1985) 559-591*** (Paper 24) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes III'', ath. Zeit. 200 (1988) 3-45* Norman Johnson ''Uniform Polytopes'', Manuscript (1991) ** N.W. Johnson: ''The Theory of Uniform Polytopes and Honeycombs'', Ph.D. * * o3x3x3o - spid, x3x3o3x - prip, x3x3x3x - gippid {{Polytopes 4-polytopes