resonant circuit on:
[Wikipedia]
[Google]
[Amazon]
 An LC circuit, also called a resonant circuit, tank circuit, or tuned circuit, is an
An LC circuit, also called a resonant circuit, tank circuit, or tuned circuit, is an
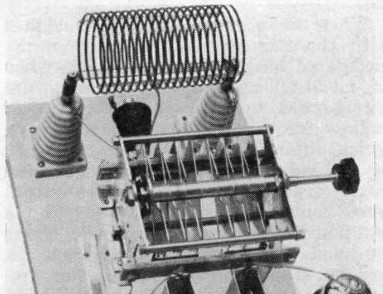
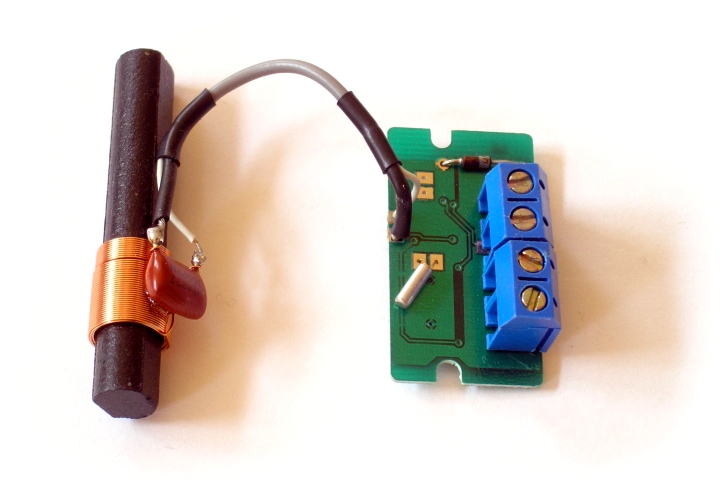 The resonance effect of the LC circuit has many important applications in signal processing and communications systems.
* The most common application of tank circuits is tuning radio transmitters and receivers. For example, when tuning a radio to a particular station, the LC circuits are set at resonance for that particular
The resonance effect of the LC circuit has many important applications in signal processing and communications systems.
* The most common application of tank circuits is tuning radio transmitters and receivers. For example, when tuning a radio to a particular station, the LC circuits are set at resonance for that particular
 In the series configuration of the LC circuit, the inductor (L) and capacitor (C) are connected in series, as shown here. The total voltage across the open terminals is simply the sum of the voltage across the inductor and the voltage across the capacitor. The current into the positive terminal of the circuit is equal to the current through both the capacitor and the inductor.
:
In the series configuration of the LC circuit, the inductor (L) and capacitor (C) are connected in series, as shown here. The total voltage across the open terminals is simply the sum of the voltage across the inductor and the voltage across the capacitor. The current into the positive terminal of the circuit is equal to the current through both the capacitor and the inductor.
:
 When the inductor (L) and capacitor (C) are connected in parallel as shown here, the voltage across the open terminals is equal to both the voltage across the inductor and the voltage across the capacitor. The total current flowing into the positive terminal of the circuit is equal to the sum of the current flowing through the inductor and the current flowing through the capacitor:
:
When the inductor (L) and capacitor (C) are connected in parallel as shown here, the voltage across the open terminals is equal to both the voltage across the inductor and the voltage across the capacitor. The total current flowing into the positive terminal of the circuit is equal to the sum of the current flowing through the inductor and the current flowing through the capacitor:
:
electric circuit
An electrical network is an interconnection of electrical components (e.g., battery (electricity), batteries, resistors, inductors, capacitors, switches, transistors) or a model of such an interconnection, consisting of electrical elements (e. ...
consisting of an inductor
An inductor, also called a coil, choke, or reactor, is a Passivity (engineering), passive two-terminal electronic component, electrical component that stores energy in a magnetic field when an electric current flows through it. An inductor typic ...
, represented by the letter L, and a capacitor
In electrical engineering, a capacitor is a device that stores electrical energy by accumulating electric charges on two closely spaced surfaces that are insulated from each other. The capacitor was originally known as the condenser, a term st ...
, represented by the letter C, connected together. The circuit can act as an electrical resonator
A resonator is a device or system that exhibits resonance or resonant behavior. That is, it naturally oscillates with greater amplitude at some frequencies, called resonant frequencies, than at other frequencies. The oscillations in a reso ...
, an electrical analogue of a tuning fork
A tuning fork is an acoustic resonator in the form of a two-pronged fork with the prongs ( ''tines'') formed from a U-shaped bar of elastic metal (usually steel). It resonates at a specific constant pitch when set vibrating by striking it ag ...
, storing energy oscillating at the circuit's resonant frequency
Resonance is a phenomenon that occurs when an object or system is subjected to an external force or vibration whose frequency matches a resonant frequency (or resonance frequency) of the system, defined as a frequency that generates a maximu ...
.
LC circuits are used either for generating signals at a particular frequency, or picking out a signal at a particular frequency from a more complex signal; this function is called a bandpass filter
A band-pass filter or bandpass filter (BPF) is a device that passes frequencies within a certain range and rejects ( attenuates) frequencies outside that range.
It is the inverse of a '' band-stop filter''.
Description
In electronics and s ...
. They are key components in many electronic devices, particularly radio equipment, used in circuits such as oscillators
Oscillation is the repetitive or periodic variation, typically in time, of some measure about a central value (often a point of equilibrium) or between two or more different states. Familiar examples of oscillation include a swinging pendulum ...
, filters
Filtration is a physical process that separates solid matter and fluid from a mixture.
Filter, filtering, filters or filtration may also refer to:
Science and technology
Computing
* Filter (higher-order function), in functional programming
* Fil ...
, tuners and frequency mixer
In electronics, a mixer, or frequency mixer, is an electrical circuit that creates new frequencies from two signals applied to it. In its most common application, two signals are applied to a mixer, and it produces new signals at the sum and di ...
s.
An LC circuit is an idealized model since it assumes there is no dissipation of energy due to resistance. Any practical implementation of an LC circuit will always include loss resulting from small but non-zero resistance within the components and connecting wires. The purpose of an LC circuit is usually to oscillate with minimal damping
In physical systems, damping is the loss of energy of an oscillating system by dissipation. Damping is an influence within or upon an oscillatory system that has the effect of reducing or preventing its oscillation. Examples of damping include ...
, so the resistance is made as low as possible. While no practical circuit is without losses, it is nonetheless instructive to study this ideal form of the circuit to gain understanding and physical intuition. For a circuit model incorporating resistance, see RLC circuit
An RLC circuit is an electrical circuit consisting of a electrical resistance, resistor (R), an inductor (L), and a capacitor (C), connected in series or in parallel. The name of the circuit is derived from the letters that are used to denote ...
.
Terminology
The two-element LC circuit described above is the simplest type of inductor-capacitor network (or LC network). It is also referred to as a ''second order LC circuit'' to distinguish it from more complicated (higher order) LC networks with more inductors and capacitors. Such LC networks with more than two reactances may have more than oneresonant frequency
Resonance is a phenomenon that occurs when an object or system is subjected to an external force or vibration whose frequency matches a resonant frequency (or resonance frequency) of the system, defined as a frequency that generates a maximu ...
.
The order of the network is the order of the rational function
In mathematics, a rational function is any function that can be defined by a rational fraction, which is an algebraic fraction such that both the numerator and the denominator are polynomials. The coefficients of the polynomials need not be ...
describing the network in the complex frequency variable . Generally, the order is equal to the number of L and C elements in the circuit and in any event cannot exceed this number.
Operation
An LC circuit, oscillating at its naturalresonant frequency
Resonance is a phenomenon that occurs when an object or system is subjected to an external force or vibration whose frequency matches a resonant frequency (or resonance frequency) of the system, defined as a frequency that generates a maximu ...
, can store electrical energy
Electrical energy is the energy transferred as electric charges move between points with different electric potential, that is, as they move across a voltage, potential difference. As electric potential is lost or gained, work is done changing the ...
. See the animation. A capacitor stores energy in the electric field
An electric field (sometimes called E-field) is a field (physics), physical field that surrounds electrically charged particles such as electrons. In classical electromagnetism, the electric field of a single charge (or group of charges) descri ...
() between its plates, depending on the voltage
Voltage, also known as (electrical) potential difference, electric pressure, or electric tension, is the difference in electric potential between two points. In a Electrostatics, static electric field, it corresponds to the Work (electrical), ...
across it, and an inductor stores energy in its magnetic field
A magnetic field (sometimes called B-field) is a physical field that describes the magnetic influence on moving electric charges, electric currents, and magnetic materials. A moving charge in a magnetic field experiences a force perpendicular ...
(), depending on the current through it.
If an inductor is connected across a charged capacitor, the voltage across the capacitor will drive a current through the inductor, building up a magnetic field around it. The voltage across the capacitor falls to zero as the charge is used up by the current flow. At this point, the energy stored in the coil's magnetic field induces a voltage across the coil, because inductors oppose changes in current. This induced voltage causes a current to begin to recharge the capacitor with a voltage of opposite polarity to its original charge. Due to Faraday's law, the EMF which drives the current is caused by a decrease in the magnetic field, thus the energy required to charge the capacitor is extracted from the magnetic field. When the magnetic field is completely dissipated the current will stop and the charge will again be stored in the capacitor, with the opposite polarity as before. Then the cycle will begin again, with the current flowing in the opposite direction through the inductor.
The charge flows back and forth between the plates of the capacitor, through the inductor. The energy oscillates back and forth between the capacitor and the inductor until (if not replenished from an external circuit) internal resistance makes the oscillations die out. The tuned circuit's action, known mathematically as a harmonic oscillator
In classical mechanics, a harmonic oscillator is a system that, when displaced from its equilibrium position, experiences a restoring force ''F'' proportional to the displacement ''x'':
\vec F = -k \vec x,
where ''k'' is a positive const ...
, is similar to a pendulum
A pendulum is a device made of a weight suspended from a pivot so that it can swing freely. When a pendulum is displaced sideways from its resting, equilibrium position, it is subject to a restoring force due to gravity that will accelerate i ...
swinging back and forth, or water sloshing back and forth in a tank; for this reason the circuit is also called a ''tank circuit''. The natural frequency
Natural frequency, measured in terms of '' eigenfrequency'', is the rate at which an oscillatory system tends to oscillate in the absence of disturbance. A foundational example pertains to simple harmonic oscillators, such as an idealized spring ...
(that is, the frequency at which it will oscillate when isolated from any other system, as described above) is determined by the capacitance and inductance values. In most applications the tuned circuit is part of a larger circuit which applies alternating current
Alternating current (AC) is an electric current that periodically reverses direction and changes its magnitude continuously with time, in contrast to direct current (DC), which flows only in one direction. Alternating current is the form in w ...
to it, driving continuous oscillations. If the frequency of the applied current is the circuit's natural resonant frequency (natural frequency
Natural frequency, measured in terms of '' eigenfrequency'', is the rate at which an oscillatory system tends to oscillate in the absence of disturbance. A foundational example pertains to simple harmonic oscillators, such as an idealized spring ...
below), resonance
Resonance is a phenomenon that occurs when an object or system is subjected to an external force or vibration whose frequency matches a resonant frequency (or resonance frequency) of the system, defined as a frequency that generates a maximu ...
will occur, and a small driving current can excite large amplitude oscillating voltages and currents. In typical tuned circuits in electronic equipment the oscillations are very fast, from thousands to billions of times per second.
Resonance effect
Resonance
Resonance is a phenomenon that occurs when an object or system is subjected to an external force or vibration whose frequency matches a resonant frequency (or resonance frequency) of the system, defined as a frequency that generates a maximu ...
occurs when an LC circuit is driven from an external source at an angular frequency at which the inductive and capacitive reactances are equal in magnitude. The frequency at which this equality holds for the particular circuit is called the resonant frequency. The resonant frequency
Resonance is a phenomenon that occurs when an object or system is subjected to an external force or vibration whose frequency matches a resonant frequency (or resonance frequency) of the system, defined as a frequency that generates a maximu ...
of the LC circuit is
:
where is the inductance
Inductance is the tendency of an electrical conductor to oppose a change in the electric current flowing through it. The electric current produces a magnetic field around the conductor. The magnetic field strength depends on the magnitude of the ...
in henries, and is the capacitance
Capacitance is the ability of an object to store electric charge. It is measured by the change in charge in response to a difference in electric potential, expressed as the ratio of those quantities. Commonly recognized are two closely related ...
in farad
The farad (symbol: F) is the unit of electrical capacitance, the ability of a body to store an electrical charge, in the International System of Units, International System of Units (SI), equivalent to 1 coulomb per volt (C/V). It is named afte ...
s. The angular frequency
In physics, angular frequency (symbol ''ω''), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase argument of a sinusoidal waveform or sine ...
has units of radian
The radian, denoted by the symbol rad, is the unit of angle in the International System of Units (SI) and is the standard unit of angular measure used in many areas of mathematics. It is defined such that one radian is the angle subtended at ...
s per second.
The equivalent frequency in units of hertz
The hertz (symbol: Hz) is the unit of frequency in the International System of Units (SI), often described as being equivalent to one event (or Cycle per second, cycle) per second. The hertz is an SI derived unit whose formal expression in ter ...
is
:
Applications

 The resonance effect of the LC circuit has many important applications in signal processing and communications systems.
* The most common application of tank circuits is tuning radio transmitters and receivers. For example, when tuning a radio to a particular station, the LC circuits are set at resonance for that particular
The resonance effect of the LC circuit has many important applications in signal processing and communications systems.
* The most common application of tank circuits is tuning radio transmitters and receivers. For example, when tuning a radio to a particular station, the LC circuits are set at resonance for that particular carrier frequency
In telecommunications, a carrier wave, carrier signal, or just carrier, is a periodic waveform (usually sinusoidal) that conveys information through a process called ''modulation''. One or more of the wave's properties, such as amplitude or fre ...
.
* A series
Series may refer to:
People with the name
* Caroline Series (born 1951), English mathematician, daughter of George Series
* George Series (1920–1995), English physicist
Arts, entertainment, and media
Music
* Series, the ordered sets used i ...
resonant circuit provides voltage magnification.
* A parallel resonant circuit provides current magnification.
* A parallel resonant circuit can be used as load impedance in output circuits of RF amplifiers. Due to high impedance, the gain of amplifier is maximum at resonant frequency.
* Both parallel and series resonant circuits are used in induction heating
Induction heating is the process of heating electrically conductive materials, namely metals or semi-conductors, by electromagnetic induction, through heat transfer passing through an inductor that creates an electromagnetic field within the coi ...
.
LC circuits behave as electronic resonators
A resonator is a device or system that exhibits resonance or resonant behavior. That is, it naturally oscillates with greater amplitude at some frequencies, called resonant frequencies, than at other frequencies. The oscillations in a resonat ...
, which are a key component in many applications:
* Amplifiers
An amplifier, electronic amplifier or (informally) amp is an electronic device that can increase the magnitude of a signal (a time-varying voltage or current). It is a two-port electronic circuit that uses electric power from a power suppl ...
* Oscillators
Oscillation is the repetitive or periodic variation, typically in time, of some measure about a central value (often a point of equilibrium) or between two or more different states. Familiar examples of oscillation include a swinging pendulum ...
* Filters
Filtration is a physical process that separates solid matter and fluid from a mixture.
Filter, filtering, filters or filtration may also refer to:
Science and technology
Computing
* Filter (higher-order function), in functional programming
* Fil ...
* Tuners
* Mixers
* Foster–Seeley discriminator
The Foster–Seeley discriminator is a common type of FM detector circuit, invented in 1936 by Dudley E. Foster and Stuart William Seeley. The Foster–Seeley discriminator was envisioned for automatic frequency control of receivers, but also ...
* Contactless cards
* Graphics tablet
A graphics tablet (also known as a digitizer, digital graphic tablet, pen tablet, drawing tablet, external drawing pad or digital art board) is a computer input device that enables a user to hand draw or paint images, animations and graphics, w ...
s
* Electronic article surveillance (security tags)
Time domain solution
Kirchhoff's laws
ByKirchhoff's voltage law
Kirchhoff's circuit laws are two equalities that deal with the current and potential difference (commonly known as voltage) in the lumped element model of electrical circuits. They were first described in 1845 by German physicist Gustav Kirchh ...
, the voltage across the capacitor plus the voltage across the inductor must equal zero:
:
Likewise, by Kirchhoff's current law
Kirchhoff's circuit laws are two Equality (mathematics), equalities that deal with the Electric current, current and potential difference (commonly known as voltage) in the lumped element model of electrical circuits. They were first described in ...
, the current through the capacitor equals the current through the inductor:
:
From the constitutive relations for the circuit elements, we also know that
:
Differential equation
Rearranging and substituting gives the second order differential equation : The parameter , the resonantangular frequency
In physics, angular frequency (symbol ''ω''), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase argument of a sinusoidal waveform or sine ...
, is defined as
:
Using this can simplify the differential equation:
:
The associated Laplace transform
In mathematics, the Laplace transform, named after Pierre-Simon Laplace (), is an integral transform that converts a Function (mathematics), function of a Real number, real Variable (mathematics), variable (usually t, in the ''time domain'') to a f ...
is
:
thus
:
where is the imaginary unit
The imaginary unit or unit imaginary number () is a mathematical constant that is a solution to the quadratic equation Although there is no real number with this property, can be used to extend the real numbers to what are called complex num ...
.
Solution
Thus, the complete solution to the differential equation is : and can be solved for and by considering the initial conditions. Since the exponential iscomplex
Complex commonly refers to:
* Complexity, the behaviour of a system whose components interact in multiple ways so possible interactions are difficult to describe
** Complex system, a system composed of many components which may interact with each ...
, the solution represents a sinusoidal alternating current
Alternating current (AC) is an electric current that periodically reverses direction and changes its magnitude continuously with time, in contrast to direct current (DC), which flows only in one direction. Alternating current is the form in w ...
. Since the electric current is a physical quantity, it must be real-valued. As a result, it can be shown that the constants and must be complex conjugate
In mathematics, the complex conjugate of a complex number is the number with an equal real part and an imaginary part equal in magnitude but opposite in sign. That is, if a and b are real numbers, then the complex conjugate of a + bi is a - ...
s:
:
Now let
:
Therefore,
:
Next, we can use Euler's formula
Euler's formula, named after Leonhard Euler, is a mathematical formula in complex analysis that establishes the fundamental relationship between the trigonometric functions and the complex exponential function. Euler's formula states that, for ...
to obtain a real sinusoid
A sine wave, sinusoidal wave, or sinusoid (symbol: ∿) is a periodic wave whose waveform (shape) is the trigonometric sine function. In mechanics, as a linear motion over time, this is '' simple harmonic motion''; as rotation, it correspond ...
with amplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of am ...
, angular frequency
In physics, angular frequency (symbol ''ω''), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase argument of a sinusoidal waveform or sine ...
, and phase angle .
Thus, the resulting solution becomes
:
:
Initial conditions
The initial conditions that would satisfy this result are : :Series circuit
Resonance
Inductive reactance
In electrical circuits, reactance is the opposition presented to alternating current by inductance and capacitance. It's measured in Ω (Ohms). Along with resistance, it is one of two elements of impedance; however, while both elements involve ...
increases as frequency increases, while capacitive reactance
In electrical circuits, reactance is the opposition presented to alternating current by inductance and capacitance. It's measured in Ω (Ohms). Along with resistance, it is one of two elements of impedance; however, while both elements involve ...
decreases with increase in frequency (defined here as a positive number). At one particular frequency, these two reactances are equal and the voltages across them are equal and opposite in sign; that frequency is called the resonant frequency for the given circuit.
Hence, at resonance,
:
Solving for , we have
:
which is defined as the resonant angular frequency of the circuit. Converting angular frequency (in radians per second) into frequency (in Hertz
The hertz (symbol: Hz) is the unit of frequency in the International System of Units (SI), often described as being equivalent to one event (or Cycle per second, cycle) per second. The hertz is an SI derived unit whose formal expression in ter ...
), one has
:
and
:
at .
In a series configuration, and cancel each other out. In real, rather than idealised, components, the current is opposed, mostly by the resistance of the coil windings. Thus, the current supplied to a series resonant circuit is maximal at resonance.
* In the limit as current is maximal. Circuit impedance is minimal. In this state, a circuit is called an ''acceptor circuit''
* For , ; hence, the circuit is capacitive.
* For , ; hence, the circuit is inductive.
Impedance
In the series configuration, resonance occurs when the complex electrical impedance of the circuit approaches zero. First consider the impedance of the series LC circuit. The total impedance is given by the sum of the inductive and capacitive impedances: : Writing the inductive impedance as and capacitive impedance as and substituting gives : Writing this expression under a common denominator gives : Finally, defining the natural angular frequency as : the impedance becomes : where gives the reactance of the inductor at resonance. The numerator implies that in the limit as , the total impedance will be zero and otherwise non-zero. Therefore the series LC circuit, when connected in series with a load, will act as aband-pass filter
A band-pass filter or bandpass filter (BPF) is a device that passes frequencies within a certain range and rejects ( attenuates) frequencies outside that range.
It is the inverse of a '' band-stop filter''.
Description
In electronics and s ...
having zero impedance at the resonant frequency of the LC circuit.
Parallel circuit
Resonance
When equals , the two branch currents are equal and opposite. They cancel each other out to give minimal current in the main line (in principle, for a finite voltage , there is zero current). Since total current in the main line is minimal, in this state the total impedance is maximal. There is also a larger current circulating in the loop formed by the capacitor and inductor. For a finite voltage , this circulating current is finite, with value given by the respective voltage-current relationships of the capacitor and inductor. However, for a finite total current in the main line, in principle, the circulating current would be infinite. In reality, the circulating current in this case is limited by resistance in the circuit, particularly resistance in the inductor windings. The resonant frequency is given by : Any branch current is not minimal at resonance, but each is given separately by dividing source voltage () by reactance (). Hence , as perOhm's law
Ohm's law states that the electric current through a Electrical conductor, conductor between two Node (circuits), points is directly Proportionality (mathematics), proportional to the voltage across the two points. Introducing the constant of ...
.
* At , the line current is minimal. The total impedance is maximal. In this state a circuit is called a ''rejector circuit''.
* Below , the circuit is inductive.
* Above , the circuit is capacitive.
Impedance
The same analysis may be applied to the parallel LC circuit. The total impedance is then given by : and after substitution of and and simplification, gives : Using : it further simplifies to : Note that : but for all other values of the impedance is finite. Thus, the parallel LC circuit connected in series with a load will act asband-stop filter
In signal processing, a band-stop filter or band-rejection filter is a filter that passes most frequencies unaltered, but attenuates those in a specific range to very low levels. It is the inverse of a ''band-pass filter''. A notch filter is ...
having infinite impedance at the resonant frequency of the LC circuit, while the parallel LC circuit connected in parallel with a load will act as band-pass filter
A band-pass filter or bandpass filter (BPF) is a device that passes frequencies within a certain range and rejects ( attenuates) frequencies outside that range.
It is the inverse of a '' band-stop filter''.
Description
In electronics and s ...
.
Laplace solution
The LC circuit can be solved using theLaplace transform
In mathematics, the Laplace transform, named after Pierre-Simon Laplace (), is an integral transform that converts a Function (mathematics), function of a Real number, real Variable (mathematics), variable (usually t, in the ''time domain'') to a f ...
.
We begin by defining the relation between current and voltage across the capacitor and inductor in the usual way:
: and
Then by application of Kirchhoff's laws, we may arrive at the system's governing differential equations
:
With initial conditions and
Making the following definitions,
: and
gives
:
Now we apply the Laplace transform.
:
:
The Laplace transform has turned our differential equation into an algebraic equation. Solving for in the domain (frequency domain) is much simpler viz.
:
:
Which can be transformed back to the time domain via the inverse Laplace transform:
: