Resonance Frequency on:
[Wikipedia]
[Google]
[Amazon]
 Resonance describes the phenomenon of increased
Resonance describes the phenomenon of increased
 A familiar example is a playground swing, which acts as a
A familiar example is a playground swing, which acts as a
 Resonance occurs when, at certain driving frequencies, the steady-state amplitude of ''x''(''t'') is large compared to its amplitude at other driving frequencies. For the mass on a spring, resonance corresponds physically to the mass's oscillations having large displacements from the spring's equilibrium position at certain driving frequencies. Looking at the amplitude of ''x''(''t'') as a function of the driving frequency ''ω'', the amplitude is maximal at the driving frequency
''ω''''r'' is the resonant frequency for this system. Again, note that the resonant frequency does not equal the undamped angular frequency ''ω''0 of the oscillator. They are proportional, and if the damping ratio goes to zero they are the same, but for non-zero damping they are not the same frequency. As shown in the figure, resonance may also occur at other frequencies near the resonant frequency, including ''ω''0, but the maximum response is at the resonant frequency.
Also note that ''ω''''r'' is only real and non-zero if , so this system can only resonate when the harmonic oscillator is significantly underdamped. For systems with a very small damping ratio and a driving frequency near the resonant frequency, the steady state oscillations can become very large.
Resonance occurs when, at certain driving frequencies, the steady-state amplitude of ''x''(''t'') is large compared to its amplitude at other driving frequencies. For the mass on a spring, resonance corresponds physically to the mass's oscillations having large displacements from the spring's equilibrium position at certain driving frequencies. Looking at the amplitude of ''x''(''t'') as a function of the driving frequency ''ω'', the amplitude is maximal at the driving frequency
''ω''''r'' is the resonant frequency for this system. Again, note that the resonant frequency does not equal the undamped angular frequency ''ω''0 of the oscillator. They are proportional, and if the damping ratio goes to zero they are the same, but for non-zero damping they are not the same frequency. As shown in the figure, resonance may also occur at other frequencies near the resonant frequency, including ''ω''0, but the maximum response is at the resonant frequency.
Also note that ''ω''''r'' is only real and non-zero if , so this system can only resonate when the harmonic oscillator is significantly underdamped. For systems with a very small damping ratio and a driving frequency near the resonant frequency, the steady state oscillations can become very large.
 Consider a circuit consisting of a
Consider a circuit consisting of a
 A sinusoidal input voltage at frequency ''ω'' results in an output voltage at the same frequency that has been scaled by ''G''(''ω'') and has a phase shift ''Φ''(''ω''). The gain and phase can be plotted versus frequency on a Bode plot. For the RLC circuit's capacitor voltage, the gain of the transfer function ''H''(''iω'') is
Note the similarity between the gain here and the amplitude in Equation (). Once again, the gain is maximized at the resonant frequency
Here, the resonance corresponds physically to having a relatively large amplitude for the steady state oscillations of the voltage across the capacitor compared to its amplitude at other driving frequencies.
A sinusoidal input voltage at frequency ''ω'' results in an output voltage at the same frequency that has been scaled by ''G''(''ω'') and has a phase shift ''Φ''(''ω''). The gain and phase can be plotted versus frequency on a Bode plot. For the RLC circuit's capacitor voltage, the gain of the transfer function ''H''(''iω'') is
Note the similarity between the gain here and the amplitude in Equation (). Once again, the gain is maximized at the resonant frequency
Here, the resonance corresponds physically to having a relatively large amplitude for the steady state oscillations of the voltage across the capacitor compared to its amplitude at other driving frequencies.
 A physical system can have as many natural frequencies as it has
A physical system can have as many natural frequencies as it has
 When a string of fixed length is driven at a particular frequency, a wave propagates along the string at the same frequency. The waves reflect off the ends of the string, and eventually a
When a string of fixed length is driven at a particular frequency, a wave propagates along the string at the same frequency. The waves reflect off the ends of the string, and eventually a


 The ''Q'' factor or ''quality factor'' is a
The ''Q'' factor or ''quality factor'' is a
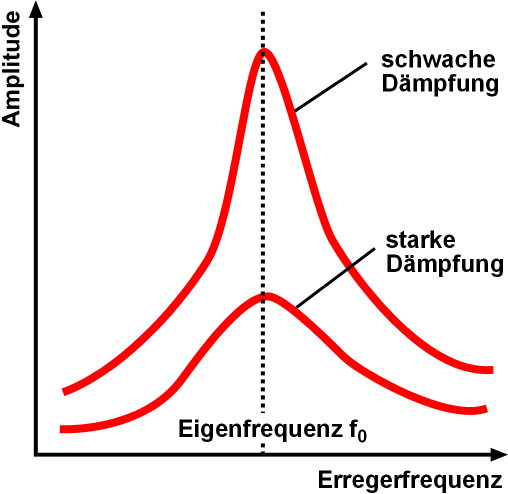
 The exact response of a resonance, especially for frequencies far from the resonant frequency, depends on the details of the physical system, and is usually not exactly symmetric about the resonant frequency, as illustrated for the
The exact response of a resonance, especially for frequencies far from the resonant frequency, depends on the details of the physical system, and is usually not exactly symmetric about the resonant frequency, as illustrated for the
The Feynman Lectures on Physics Vol. I Ch. 23: Resonance
- a chapter from an online textbook *Brian Greene, Greene, Brian, "
Resonance in strings
'". The Elegant Universe, Nova (American TV program), NOVA (Public Broadcasting Service, PBS)
Hyperphysics section on resonance concepts
(usage of terms)
demonstrating resonances on a string when the frequency of the driving force is varied
demonstrating the occurrence of resonance when the driving frequency matches with the natural frequency of an oscillator
Breaking glass with sound
including high-speed footage of glass breaking {{Authority control Resonance, Antennas (radio) Oscillation
 Resonance describes the phenomenon of increased
Resonance describes the phenomenon of increased amplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of amplit ...
that occurs when the frequency
Frequency is the number of occurrences of a repeating event per unit of time. It is also occasionally referred to as ''temporal frequency'' for clarity, and is distinct from ''angular frequency''. Frequency is measured in hertz (Hz) which is eq ...
of an applied periodic force
In physics, a force is an influence that can change the motion of an object. A force can cause an object with mass to change its velocity (e.g. moving from a state of rest), i.e., to accelerate. Force can also be described intuitively as a p ...
(or a Fourier component
A Fourier transform (FT) is a mathematical transform that decomposes functions into frequency components, which are represented by the output of the transform as a function of frequency. Most commonly functions of time or space are transformed, ...
of it) is equal or close to a natural frequency
Natural frequency, also known as eigenfrequency, is the frequency at which a system tends to oscillate in the absence of any driving force.
The motion pattern of a system oscillating at its natural frequency is called the normal mode (if all par ...
of the system on which it acts. When an oscillating
Oscillation is the repetitive or periodic variation, typically in time, of some measure about a central value (often a point of equilibrium) or between two or more different states. Familiar examples of oscillation include a swinging pendulum ...
force is applied at a resonant frequency of a dynamic system, the system will oscillate at a higher amplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of amplit ...
than when the same force is applied at other, non-resonant frequencies.
Frequencies at which the response amplitude is a relative maximum
In mathematical analysis, the maxima and minima (the respective plurals of maximum and minimum) of a function (mathematics), function, known collectively as extrema (the plural of extremum), are the largest and smallest value of the function, e ...
are also known as resonant frequencies or resonance frequencies of the system. Small periodic forces that are near a resonant frequency of the system have the ability to produce large amplitude oscillation
Oscillation is the repetitive or periodic variation, typically in time, of some measure about a central value (often a point of equilibrium) or between two or more different states. Familiar examples of oscillation include a swinging pendulum ...
s in the system due to the storage of vibrational energy
In physics, sound energy is a form of energy that can be heard by living things. Only those waves that have a frequency of 16 Hz to 20 kHz are audible to humans. However, this range is an average and will slightly change from individ ...
.
Resonance phenomena occur with all types of vibrations or wave
In physics, mathematics, and related fields, a wave is a propagating dynamic disturbance (change from equilibrium) of one or more quantities. Waves can be periodic, in which case those quantities oscillate repeatedly about an equilibrium (res ...
s: there is mechanical resonance
Mechanical resonance is the tendency of a mechanical system to respond at greater amplitude when the frequency of its oscillations matches the system's natural frequency of vibration (its ''resonance frequency'' or ''resonant frequency'') close ...
, orbital resonance
In celestial mechanics, orbital resonance occurs when orbiting bodies exert regular, periodic gravitational influence on each other, usually because their orbital periods are related by a ratio of small integers. Most commonly, this relationsh ...
, acoustic resonance
Acoustic resonance is a phenomenon in which an acoustic system amplifies sound waves whose frequency matches one of its own natural frequencies of vibration (its ''resonance frequencies'').
The term "acoustic resonance" is sometimes used to nar ...
, electromagnetic
In physics, electromagnetism is an interaction that occurs between particles with electric charge. It is the second-strongest of the four fundamental interactions, after the strong force, and it is the dominant force in the interactions of a ...
resonance, nuclear magnetic resonance
Nuclear magnetic resonance (NMR) is a physical phenomenon in which nuclei in a strong constant magnetic field are perturbed by a weak oscillating magnetic field (in the near field) and respond by producing an electromagnetic signal with a ...
(NMR), electron spin resonance
Electron paramagnetic resonance (EPR) or electron spin resonance (ESR) spectroscopy is a method for studying materials that have unpaired electrons. The basic concepts of EPR are analogous to those of nuclear magnetic resonance (NMR), but the spi ...
(ESR) and resonance of quantum wave function
A wave function in quantum physics is a mathematical description of the quantum state of an isolated quantum system. The wave function is a complex-valued probability amplitude, and the probabilities for the possible results of measurements mad ...
s. Resonant systems can be used to generate vibrations of a specific frequency (e.g., musical instrument
A musical instrument is a device created or adapted to make musical sounds. In principle, any object that produces sound can be considered a musical instrument—it is through purpose that the object becomes a musical instrument. A person who pl ...
s), or pick out specific frequencies from a complex vibration containing many frequencies (e.g., filters).
The term ''resonance'' (from Latin
Latin (, or , ) is a classical language belonging to the Italic branch of the Indo-European languages. Latin was originally a dialect spoken in the lower Tiber area (then known as Latium) around present-day Rome, but through the power of the ...
''resonantia'', 'echo', from ''resonare'', 'resound') originated from the field of acoustics, particularly the sympathetic resonance
Sympathetic resonance or sympathetic vibration is a harmonic phenomenon wherein a passive string or vibratory body responds to external vibrations to which it has a harmonic likeness. The classic example is demonstrated with two similarly-tuned ...
observed in musical instruments, e.g., when one string starts to vibrate and produce sound after a different one is struck.
Overview
Resonance occurs when a system is able to store and easily transfer energy between two or more differentstorage mode
Storage may refer to:
Goods Containers
* Dry cask storage, for storing high-level radioactive waste
* Food storage
* Intermodal container, cargo shipping
* Storage tank
Facilities
* Garage (residential), a storage space normally used to store car ...
s (such as kinetic energy and potential energy in the case of a simple pendulum). However, there are some losses from cycle to cycle, called damping
Damping is an influence within or upon an oscillatory system that has the effect of reducing or preventing its oscillation. In physical systems, damping is produced by processes that dissipate the energy stored in the oscillation. Examples incl ...
. When damping is small, the resonant frequency is approximately equal to the natural frequency
Natural frequency, also known as eigenfrequency, is the frequency at which a system tends to oscillate in the absence of any driving force.
The motion pattern of a system oscillating at its natural frequency is called the normal mode (if all par ...
of the system, which is a frequency of unforced vibrations. Some systems have multiple, distinct, resonant frequencies.
Examples
 A familiar example is a playground swing, which acts as a
A familiar example is a playground swing, which acts as a pendulum
A pendulum is a weight suspended from a pivot so that it can swing freely. When a pendulum is displaced sideways from its resting, equilibrium position, it is subject to a restoring force due to gravity that will accelerate it back toward the ...
. Pushing a person in a swing in time with the natural interval of the swing (its resonant frequency) makes the swing go higher and higher (maximum amplitude), while attempts to push the swing at a faster or slower tempo produce smaller arcs. This is because the energy the swing absorbs is maximized when the pushes match the swing's natural oscillations.
Resonance occurs widely in nature, and is exploited in many devices. It is the mechanism by which virtually all sinusoidal
A sine wave, sinusoidal wave, or just sinusoid is a mathematical curve defined in terms of the '' sine'' trigonometric function, of which it is the graph. It is a type of continuous wave and also a smooth periodic function. It occurs often in m ...
waves and vibrations are generated. Many sounds we hear, such as when hard objects of metal
A metal (from Greek μέταλλον ''métallon'', "mine, quarry, metal") is a material that, when freshly prepared, polished, or fractured, shows a lustrous appearance, and conducts electricity and heat relatively well. Metals are typicall ...
, glass
Glass is a non-crystalline, often transparent, amorphous solid that has widespread practical, technological, and decorative use in, for example, window panes, tableware, and optics. Glass is most often formed by rapid cooling (quenching) of ...
, or wood
Wood is a porous and fibrous structural tissue found in the stems and roots of trees and other woody plants. It is an organic materiala natural composite of cellulose fibers that are strong in tension and embedded in a matrix of lignin th ...
are struck, are caused by brief resonant vibrations in the object. Light and other short wavelength electromagnetic radiation
In physics, electromagnetic radiation (EMR) consists of waves of the electromagnetic field, electromagnetic (EM) field, which propagate through space and carry momentum and electromagnetic radiant energy. It includes radio waves, microwaves, inf ...
is produced by resonance on an atomic scale
Atomic spacing refers to the distance between the nuclei of atoms in a material. This space is extremely large compared to the size of the atomic nucleus, and is related to the chemical bonds which bind atoms together. In solid materials, the ato ...
, such as electron
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have no kn ...
s in atoms. Other examples of resonance:
* Timekeeping mechanisms of modern clocks and watches, e.g., the balance wheel
A balance wheel, or balance, is the timekeeping device used in mechanical watches and small clocks, analogous to the pendulum in a pendulum clock. It is a weighted wheel that rotates back and forth, being returned toward its center position by a ...
in a mechanical watch
A watch is a portable timepiece intended to be carried or worn by a person. It is designed to keep a consistent movement despite the motions caused by the person's activities. A wristwatch is designed to be worn around the wrist, attached by ...
and the quartz crystal
Quartz is a hard, crystalline mineral composed of silica (silicon dioxide). The atoms are linked in a continuous framework of SiO4 silicon-oxygen tetrahedra, with each oxygen being shared between two tetrahedra, giving an overall chemical form ...
in a quartz watch
Quartz is a hard, crystalline mineral composed of silica (silicon dioxide). The atoms are linked in a continuous framework of SiO4 silicon-oxygen Tetrahedral molecular geometry, tetrahedra, with each oxygen being shared between two tetrahedra, ...
* Tidal resonance
In oceanography, a tidal resonance occurs when the tide excites one of the resonant modes of the ocean.
The effect is most striking when a continental shelf is about a quarter wavelength wide. Then an incident tidal wave can be reinforced b ...
of the Bay of Fundy
The Bay of Fundy (french: Baie de Fundy) is a bay between the Canadian provinces of New Brunswick and Nova Scotia, with a small portion touching the U.S. state of Maine. It is an arm of the Gulf of Maine. Its extremely high tidal range is the hi ...
* Acoustic resonance
Acoustic resonance is a phenomenon in which an acoustic system amplifies sound waves whose frequency matches one of its own natural frequencies of vibration (its ''resonance frequencies'').
The term "acoustic resonance" is sometimes used to nar ...
s of musical instruments
A musical instrument is a device created or adapted to make musical sounds. In principle, any object that produces sound can be considered a musical instrument—it is through purpose that the object becomes a musical instrument. A person who pl ...
and the human vocal tract
The vocal tract is the cavity in human bodies and in animals where the sound produced at the sound source (larynx in mammals; syrinx (biology), syrinx in birds) is filtered.
In birds it consists of the Vertebrate trachea, trachea, the Syrinx (bio ...
* Shattering of a crystal wineglass when exposed to a musical tone of the right pitch (its resonant frequency)
* Friction idiophone
Friction idiophones is designation 13 in the Hornbostel-Sachs system of musical instrument classification. These idiophones produce sound by being rubbed either against each other or by means of a non-sounding object. Instruments of this type a ...
s, such as making a glass object (glass, bottle, vase) vibrate
Vibration is a mechanical phenomenon whereby oscillations occur about an equilibrium point. The word comes from Latin ''vibrationem'' ("shaking, brandishing"). The oscillations may be periodic function, periodic, such as the motion of a pendulum ...
by rubbing around its rim with a fingertip
* Electrical resonance
Electrical resonance occurs in an electric circuit at a particular '' resonant frequency'' when the impedances or admittances of circuit elements cancel each other. In some circuits, this happens when the impedance between the input and output of ...
of tuned circuit
An LC circuit, also called a resonant circuit, tank circuit, or tuned circuit, is an electric circuit consisting of an inductor, represented by the letter L, and a capacitor, represented by the letter C, connected together. The circuit can ac ...
s in radio
Radio is the technology of signaling and communicating using radio waves. Radio waves are electromagnetic waves of frequency between 30 hertz (Hz) and 300 gigahertz (GHz). They are generated by an electronic device called a transmit ...
s and TVs that allow radio frequencies to be selectively received
* Creation of coherent
Coherence, coherency, or coherent may refer to the following:
Physics
* Coherence (physics), an ideal property of waves that enables stationary (i.e. temporally and spatially constant) interference
* Coherence (units of measurement), a deri ...
light by optical resonance in a laser
A laser is a device that emits light through a process of optical amplification based on the stimulated emission of electromagnetic radiation. The word "laser" is an acronym for "light amplification by stimulated emission of radiation". The fir ...
cavity
Cavity may refer to:
Biology and healthcare
*Body cavity, a fluid-filled space in many animals where organs typically develop
**Gastrovascular cavity, the primary organ of digestion and circulation in cnidarians and flatworms
*Dental cavity or too ...
* Orbital resonance
In celestial mechanics, orbital resonance occurs when orbiting bodies exert regular, periodic gravitational influence on each other, usually because their orbital periods are related by a ratio of small integers. Most commonly, this relationsh ...
as exemplified by some moons
A natural satellite is, in the most common usage, an astronomical body that orbits a planet, dwarf planet, or small Solar System body (or sometimes another natural satellite). Natural satellites are often colloquially referred to as ''moons'' ...
of the Solar System
The Solar SystemCapitalization of the name varies. The International Astronomical Union, the authoritative body regarding astronomical nomenclature, specifies capitalizing the names of all individual astronomical objects but uses mixed "Solar S ...
's gas giants
A gas giant is a giant planet composed mainly of hydrogen and helium. Gas giants are also called failed stars because they contain the same basic elements as a star. Jupiter and Saturn are the gas giants of the Solar System. The term "gas giant ...
* Material resonances in atomic scale are the basis of several spectroscopic
Spectroscopy is the field of study that measures and interprets the electromagnetic spectra that result from the interaction between electromagnetic radiation and matter as a function of the wavelength or frequency of the radiation. Matter wav ...
techniques that are used in condensed matter physics
Condensed matter physics is the field of physics that deals with the macroscopic and microscopic physical properties of matter, especially the solid and liquid phases which arise from electromagnetic forces between atoms. More generally, the sub ...
**Electron spin resonance
Electron paramagnetic resonance (EPR) or electron spin resonance (ESR) spectroscopy is a method for studying materials that have unpaired electrons. The basic concepts of EPR are analogous to those of nuclear magnetic resonance (NMR), but the spi ...
**Mössbauer effect
The Mössbauer effect, or recoilless nuclear resonance fluorescence, is a physical phenomenon discovered by Rudolf Mössbauer in 1958. It involves the resonant and recoil-free emission and absorption of gamma radiation by atomic nuclei bound in ...
**Nuclear magnetic resonance
Nuclear magnetic resonance (NMR) is a physical phenomenon in which nuclei in a strong constant magnetic field are perturbed by a weak oscillating magnetic field (in the near field) and respond by producing an electromagnetic signal with a ...
Linear systems
Resonance manifests itself in many linear and nonlinear systems as oscillations around an equilibrium point. When the system is driven by a sinusoidal external input, a measured output of the system may oscillate in response. The ratio of the amplitude of the output's steady-state oscillations to the input's oscillations is called the gain, and the gain can be a function of the frequency of the sinusoidal external input. Peaks in the gain at certain frequencies correspond to resonances, where the amplitude of the measured output's oscillations are disproportionately large. Since many linear and nonlinear systems that oscillate are modeled asharmonic oscillator
In classical mechanics, a harmonic oscillator is a system that, when displaced from its Mechanical equilibrium, equilibrium position, experiences a restoring force ''F'' Proportionality (mathematics), proportional to the displacement ''x'':
\v ...
s near their equilibria, this section begins with a derivation of the resonant frequency for a driven, damped harmonic oscillator. The section then uses an RLC circuit
An RLC circuit is an electrical circuit consisting of a electrical resistance, resistor (R), an inductor (L), and a capacitor (C), connected in series or in parallel. The name of the circuit is derived from the letters that are used to denote the ...
to illustrate connections between resonance and a system's transfer function, frequency response, poles, and zeroes. Building off the RLC circuit example, the section then generalizes these relationships for higher-order linear systems with multiple inputs and outputs.
The driven, damped harmonic oscillator
Consider a damped mass on a spring driven by a sinusoidal, externally applied force.Newton's second law
Newton's laws of motion are three basic laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at rest, or in motion ...
takes the form
where ''m'' is the mass, ''x'' is the displacement of the mass from the equilibrium point, ''F''0 is the driving amplitude, ''ω'' is the driving angular frequency, ''k'' is the spring constant, and ''c'' is the viscous damping coefficient. This can be rewritten in the form
where
* is called the ''undamped angular frequency
In physics, angular frequency "''ω''" (also referred to by the terms angular speed, circular frequency, orbital frequency, radian frequency, and pulsatance) is a scalar measure of rotation rate. It refers to the angular displacement per unit tim ...
of the oscillator'' or the ''natural frequency'',
* is called the ''damping ratio''.
Many sources also refer to ''ω''0 as the ''resonant frequency''. However, as shown below, when analyzing oscillations of the displacement ''x''(''t''), the resonant frequency is close to but not the same as ''ω''0. In general the resonant frequency is close to but not necessarily the same as the natural frequency. The RLC circuit example in the next section gives examples of different resonant frequencies for the same system.
The general solution of Equation () is the sum of a transient solution that depends on initial conditions and a steady state
In systems theory, a system or a Process theory, process is in a steady state if the variables (called state variables) which define the behavior of the system or the process are unchanging in time. In continuous time, this means that for those p ...
solution that is independent of initial conditions and depends only on the driving amplitude ''F''0, driving frequency ''ω'', undamped angular frequency ''ω''0, and the damping ratio ''ζ''. The transient solution decays in a relatively short amount of time, so to study resonance it is sufficient to consider the steady state solution.
It is possible to write the steady-state solution for ''x''(''t'') as a function proportional to the driving force with an induced phase
Phase or phases may refer to:
Science
*State of matter, or phase, one of the distinct forms in which matter can exist
*Phase (matter), a region of space throughout which all physical properties are essentially uniform
* Phase space, a mathematic ...
change ''φ'',
where
The phase value is usually taken to be between −180° and 0 so it represents a phase lag for both positive and negative values of the arctan argument.
The pendulum
For other driven, damped harmonic oscillators whose equations of motion do not look exactly like the mass on a spring example, the resonant frequency remains but the definitions of ''ω''0 and ''ζ'' change based on the physics of the system. For a pendulum of length ''ℓ'' and small displacement angle ''θ'', Equation () becomes and therefore * *RLC series circuits
resistor
A resistor is a passive two-terminal electrical component that implements electrical resistance as a circuit element. In electronic circuits, resistors are used to reduce current flow, adjust signal levels, to divide voltages, bias active el ...
with resistance ''R'', an inductor
An inductor, also called a coil, choke, or reactor, is a passive two-terminal electrical component that stores energy in a magnetic field when electric current flows through it. An inductor typically consists of an insulated wire wound into a c ...
with inductance ''L'', and a capacitor
A capacitor is a device that stores electrical energy in an electric field by virtue of accumulating electric charges on two close surfaces insulated from each other. It is a passive electronic component with two terminals.
The effect of ...
with capacitance ''C'' connected in series with current ''i''(''t'') and driven by a voltage
Voltage, also known as electric pressure, electric tension, or (electric) potential difference, is the difference in electric potential between two points. In a static electric field, it corresponds to the work needed per unit of charge to m ...
source with voltage ''v''''in''(''t''). The voltage drop around the circuit is
Rather than analyzing a candidate solution to this equation like in the mass on a spring example above, this section will analyze the frequency response of this circuit. Taking the Laplace transform
In mathematics, the Laplace transform, named after its discoverer Pierre-Simon Laplace (), is an integral transform
In mathematics, an integral transform maps a function from its original function space into another function space via integra ...
of Equation (),
where ''I''(''s'') and ''V''''in''(''s'') are the Laplace transform of the current and input voltage, respectively, and ''s'' is a complex
Complex commonly refers to:
* Complexity, the behaviour of a system whose components interact in multiple ways so possible interactions are difficult to describe
** Complex system, a system composed of many components which may interact with each ...
frequency parameter in the Laplace domain. Rearranging terms,
Voltage across the capacitor
An RLC circuit in series presents several options for where to measure an output voltage. Suppose the output voltage of interest is the voltage drop across the capacitor. As shown above, in the Laplace domain this voltage is or Define for this circuit a natural frequency and a damping ratio, The ratio of the output voltage to the input voltage becomes ''H''(''s'') is thetransfer function
In engineering, a transfer function (also known as system function or network function) of a system, sub-system, or component is a function (mathematics), mathematical function that mathematical model, theoretically models the system's output for ...
between the input voltage and the output voltage. Note that this transfer function has two poles
Poles,, ; singular masculine: ''Polak'', singular feminine: ''Polka'' or Polish people, are a West Slavic nation and ethnic group, who share a common history, culture, the Polish language and are identified with the country of Poland in Ce ...
–roots of the polynomial in the transfer function's denominator–at
and no zeros–roots of the polynomial in the transfer function's numerator. Moreover, note that for , the magnitude of these poles is the natural frequency ''ω''0 and that for , our condition for resonance in the harmonic oscillator example, the poles are closer to the imaginary axis than to the real axis.
Evaluating ''H''(''s'') along the imaginary axis , the transfer function describes the frequency response of this circuit. Equivalently, the frequency response can be analyzed by taking the Fourier transform
A Fourier transform (FT) is a mathematical transform that decomposes functions into frequency components, which are represented by the output of the transform as a function of frequency. Most commonly functions of time or space are transformed, ...
of Equation () instead of the Laplace transform. The transfer function, which is also complex, can be written as a gain and phase,
Voltage across the inductor
The resonant frequency need not always take the form given in the examples above. For the RLC circuit, suppose instead that the output voltage of interest is the voltage across the inductor. As shown above, in the Laplace domain the voltage across the inductor is using the same definitions for ''ω''0 and ''ζ'' as in the previous example. The transfer function between ''V''in(''s'') and this new ''V''out(''s'') across the inductor is Note that this transfer function has the same poles as the transfer function in the previous example, but it also has two zeroes in the numerator at . Evaluating ''H''(''s'') along the imaginary axis, its gain becomes Compared to the gain in Equation () using the capacitor voltage as the output, this gain has a factor of ''ω''2 in the numerator and will therefore have a different resonant frequency that maximizes the gain. That frequency is So for the same RLC circuit but with the voltage across the inductor as the output, the resonant frequency is now ''larger'' than the natural frequency, though it still tends towards the natural frequency as the damping ratio goes to zero. That the same circuit can have different resonant frequencies for different choices of output is not contradictory. As shown in Equation (), the voltage drop across the circuit is divided among the three circuit elements, and each element has different dynamics. The capacitor's voltage grows slowly by integrating the current over time and is therefore more sensitive to lower frequencies, whereas the inductor's voltage grows when the current changes rapidly and is therefore more sensitive to higher frequencies. While the circuit as a whole has a natural frequency where it tends to oscillate, the different dynamics of each circuit element make each element resonate at a slightly different frequency.Voltage across the resistor
Suppose that the output voltage of interest is the voltage across the resistor. In the Laplace domain the voltage across the resistor is and using the same natural frequency and damping ratio as in the capacitor example the transfer function is Note that this transfer function also has the same poles as the previous RLC circuit examples, but it only has one zero in the numerator at ''s'' = 0. For this transfer function, its gain is The resonant frequency that maximizes this gain is and the gain is one at this frequency, so the voltage across the resistor resonates ''at'' the circuit's natural frequency and at this frequency the amplitude of the voltage across the resistor equals the input voltage's amplitude.Antiresonance
Some systems exhibitantiresonance In the physics of coupled oscillators, antiresonance, by analogy with resonance, is a pronounced minimum in the amplitude of an oscillator at a particular frequency, accompanied by a large, abrupt shift in its oscillation phase. Such frequencies ...
that can be analyzed in the same way as resonance. For antiresonance, the amplitude of the response of the system at certain frequencies is disproportionately ''small'' rather than being disproportionately large. In the RLC circuit example, this phenomenon can be observed by analyzing both the inductor and the capacitor combined.
Suppose that the output voltage of interest in the RLC circuit is the voltage across the inductor ''and'' the capacitor combined in series. Equation () showed that the sum of the voltages across the three circuit elements sums to the input voltage, so measuring the output voltage as the sum of the inductor and capacitor voltages combined is the same as ''v''''in'' minus the voltage drop across the resistor. The previous example showed that at the natural frequency of the system, the amplitude of the voltage drop across the resistor ''equals'' the amplitude of ''v''''in'', and therefore the voltage across the inductor and capacitor combined has zero amplitude. We can show this with the transfer function.
The sum of the inductor and capacitor voltages is
Using the same natural frequency and damping ratios as the previous examples, the transfer function is
Note that this transfer has the same poles as the previous examples but has zeroes at
Evaluating the transfer function along the imaginary axis, its gain is
Rather than look for resonance, i.e., peaks of the gain, notice that the gain goes to zero at ''ω'' = ''ω''0, which complements our analysis of the resistor's voltage. This is called antiresonance, which has the opposite effect of resonance. Rather than result in outputs that are disproportionately large at this frequency, this circuit with this choice of output has no response at all at this frequency. The frequency that is filtered out corresponds exactly to the zeroes of the transfer function, which were shown in Equation () and were on the imaginary axis.
Relationships between resonance and frequency response in the RLC series circuit example
These RLC circuit examples illustrate how resonance is related to the frequency response of the system. Specifically, these examples illustrate: * How resonant frequencies can be found by looking for peaks in the gain of the transfer function between the input and output of the system, for example in a Bode magnitude plot * How the resonant frequency for a single system can be different for different choices of system output * The connection between the system's natural frequency, the system's damping ratio, and the system's resonant frequency * The connection between the system's natural frequency and the magnitude of the transfer function's poles, pointed out in Equation (), and therefore a connection between the poles and the resonant frequency * A connection between the transfer function's zeroes and the shape of the gain as a function of frequency, and therefore a connection between the zeroes and the resonant frequency that maximizes gain * A connection between the transfer function's zeroes and antiresonance The next section extends these concepts to resonance in a general linear system.Generalizing resonance and antiresonance for linear systems
Next consider an arbitrary linear system with multiple inputs and outputs. For example, instate-space representation
In control engineering, a state-space representation is a mathematical model of a physical system as a set of input, output and state variables related by first-order differential equations or difference equations. State variables are variables wh ...
a third order linear time-invariant system
In system analysis, among other fields of study, a linear time-invariant (LTI) system is a system that produces an output signal from any input signal subject to the constraints of linearity and time-invariance; these terms are briefly define ...
with three inputs and two outputs might be written as
where ''u''''i''(''t'') are the inputs, ''x''''i''(t) are the state variables, ''y''''i''(''t'') are the outputs, and ''A'', ''B'', ''C'', and ''D'' are matrices describing the dynamics between the variables.
This system has a transfer function matrix
In control system theory, and various branches of engineering, a transfer function matrix, or just transfer matrix is a generalisation of the transfer functions of single-input single-output (SISO) systems to multiple-input and multiple-output ( ...
whose elements are the transfer functions between the various inputs and outputs. For example,
Each ''H''''ij''(''s'') is a scalar transfer function linking one of the inputs to one of the outputs. The RLC circuit examples above had one input voltage and showed four possible output voltages–across the capacitor, across the inductor, across the resistor, and across the capacitor and inductor combined in series–each with its own transfer function. If the RLC circuit were set up to measure all four of these output voltages, that system would have a 4×1 transfer function matrix linking the single input to each of the four outputs.
Evaluated along the imaginary axis, each ''H''''ij''(''iω'') can be written as a gain and phase shift,
Peaks in the gain at certain frequencies correspond to resonances between that transfer function's input and output, assuming the system is stable
A stable is a building in which livestock, especially horses, are kept. It most commonly means a building that is divided into separate stalls for individual animals and livestock. There are many different types of stables in use today; the ...
.
Each transfer function ''H''''ij''(''s'') can also be written as a fraction whose numerator and denominator are polynomials of ''s''.
The complex roots of the numerator are called zeroes, and the complex roots of the denominator are called poles. For a stable system, the positions of these poles and zeroes on the complex plane give some indication of whether the system can resonate or antiresonate and at which frequencies. In particular, any stable or marginally stable, complex conjugate pair of poles with imaginary components can be written in terms of a natural frequency and a damping ratio as
as in Equation (). The natural frequency ''ω''0 of that pole is the magnitude of the position of the pole on the complex plane and the damping ratio of that pole determines how quickly that oscillation decays. In general,
* Complex conjugate pairs of ''poles'' near the imaginary axis correspond to a peak or resonance in the frequency response in the vicinity of the pole's natural frequency. If the pair of poles is ''on'' the imaginary axis, the gain is infinite at that frequency.
* Complex conjugate pairs of ''zeroes'' near the imaginary axis correspond to a notch or antiresonance in the frequency response in the vicinity of the zero's frequency, i.e., the frequency equal to the magnitude of the zero. If the pair of zeroes is ''on'' the imaginary axis, the gain is zero at that frequency.
In the RLC circuit example, the first generalization relating poles to resonance is observed in Equation (). The second generalization relating zeroes to antiresonance is observed in Equation (). In the examples of the harmonic oscillator, the RLC circuit capacitor voltage, and the RLC circuit inductor voltage, "poles near the imaginary axis" corresponds to the significantly underdamped condition ζ < 1/.
Standing waves
 A physical system can have as many natural frequencies as it has
A physical system can have as many natural frequencies as it has degrees of freedom
Degrees of freedom (often abbreviated df or DOF) refers to the number of independent variables or parameters of a thermodynamic system. In various scientific fields, the word "freedom" is used to describe the limits to which physical movement or ...
and can resonate near each of those natural frequencies. A mass on a spring, which has one degree of freedom, has one natural frequency. A double pendulum
In physics and mathematics, in the area of dynamical systems, a double pendulum also known as a chaos pendulum is a pendulum with another pendulum attached to its end, forming a simple physical system that exhibits rich dynamic behavior with a ...
, which has two degrees of freedom, can have two natural frequencies. As the number of coupled harmonic oscillators increases, the time it takes to transfer energy from one to the next becomes significant. Systems with very large numbers of degrees of freedom can be thought of as continuous
Continuity or continuous may refer to:
Mathematics
* Continuity (mathematics), the opposing concept to discreteness; common examples include
** Continuous probability distribution or random variable in probability and statistics
** Continuous ...
rather than as having discrete oscillators.
Energy transfers from one oscillator to the next in the form of waves. For example, the string of a guitar or the surface of water in a bowl can be modeled as a continuum of small coupled oscillators and waves can travel along them. In many cases these systems have the potential to resonate at certain frequencies, forming standing wave
In physics, a standing wave, also known as a stationary wave, is a wave that oscillates in time but whose peak amplitude profile does not move in space. The peak amplitude of the wave oscillations at any point in space is constant with respect ...
s with large-amplitude oscillations at fixed positions. Resonance in the form of standing waves underlies many familiar phenomena, such as the sound produced by musical instruments, electromagnetic cavities used in lasers and microwave ovens, and energy levels of atoms.
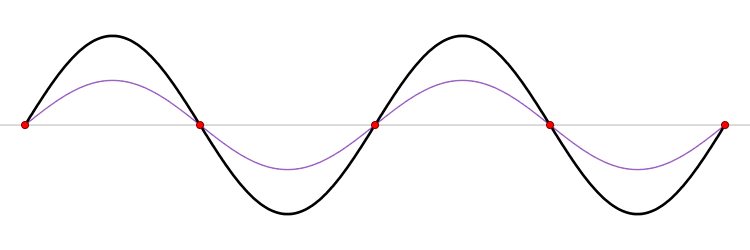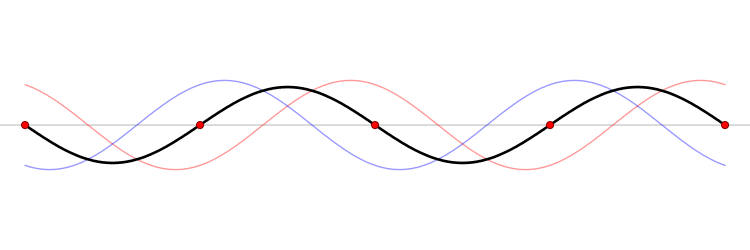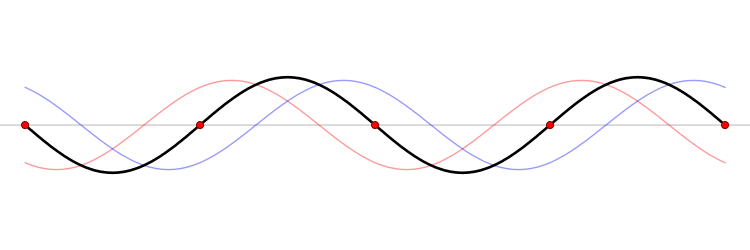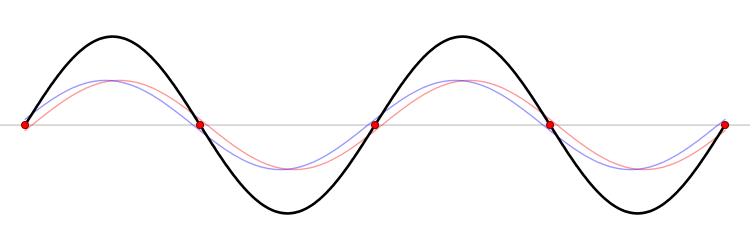
Standing waves on a string
 When a string of fixed length is driven at a particular frequency, a wave propagates along the string at the same frequency. The waves reflect off the ends of the string, and eventually a
When a string of fixed length is driven at a particular frequency, a wave propagates along the string at the same frequency. The waves reflect off the ends of the string, and eventually a steady state
In systems theory, a system or a Process theory, process is in a steady state if the variables (called state variables) which define the behavior of the system or the process are unchanging in time. In continuous time, this means that for those p ...
is reached with waves traveling in both directions. The waveform is the superposition of the waves.
At certain frequencies, the steady state waveform does not appear to travel along the string. At fixed positions called nodes
In general, a node is a localized swelling (a "knot") or a point of intersection (a Vertex (graph theory), vertex).
Node may refer to:
In mathematics
*Vertex (graph theory), a vertex in a mathematical graph
*Vertex (geometry), a point where two ...
, the string is never displaced. Between the nodes the string oscillates and exactly halfway between the nodes–at positions called anti-nodes–the oscillations have their largest amplitude.
For a string of length with fixed ends, the displacement of the string perpendicular to the -axis at time is
where
* is the amplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of amplit ...
of the left- and right-traveling waves interfering to form the standing wave,
* is the wave number
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temp ...
,
* is the frequency
Frequency is the number of occurrences of a repeating event per unit of time. It is also occasionally referred to as ''temporal frequency'' for clarity, and is distinct from ''angular frequency''. Frequency is measured in hertz (Hz) which is eq ...
.
The frequencies that resonate and form standing waves relate to the length of the string as
where is the speed of the wave and the integer denotes different modes or harmonic
A harmonic is a wave with a frequency that is a positive integer multiple of the ''fundamental frequency'', the frequency of the original periodic signal, such as a sinusoidal wave. The original signal is also called the ''1st harmonic'', the ...
s. The standing wave with oscillates at the fundamental frequency
The fundamental frequency, often referred to simply as the ''fundamental'', is defined as the lowest frequency of a periodic waveform. In music, the fundamental is the musical pitch of a note that is perceived as the lowest partial present. In ...
and has a wavelength that is twice the length of the string. The possible modes of oscillation form a harmonic series.
Types
Mechanical and acoustic

Mechanical resonance
Mechanical resonance is the tendency of a mechanical system to respond at greater amplitude when the frequency of its oscillations matches the system's natural frequency of vibration (its ''resonance frequency'' or ''resonant frequency'') close ...
is the tendency of a mechanical system
A machine is a physical system using Power (physics), power to apply Force, forces and control Motion, movement to perform an action. The term is commonly applied to artificial devices, such as those employing engines or motors, but also to na ...
to absorb more energy when the frequency
Frequency is the number of occurrences of a repeating event per unit of time. It is also occasionally referred to as ''temporal frequency'' for clarity, and is distinct from ''angular frequency''. Frequency is measured in hertz (Hz) which is eq ...
of its oscillations matches the system's natural frequency of vibration
Vibration is a mechanical phenomenon whereby oscillations occur about an equilibrium point. The word comes from Latin ''vibrationem'' ("shaking, brandishing"). The oscillations may be periodic function, periodic, such as the motion of a pendulum ...
than it does at other frequencies. It may cause violent swaying motions and even catastrophic failure in improperly constructed structures including bridges, buildings, trains, and aircraft. When designing objects, engineers
Engineers, as practitioners of engineering, are professionals who invent, design, analyze, build and test machines, complex systems, structures, gadgets and materials to fulfill functional objectives and requirements while considering the limit ...
must ensure the mechanical resonance frequencies of the component parts do not match driving vibrational frequencies of motors or other oscillating parts, a phenomenon known as resonance disaster.
Avoiding resonance disasters is a major concern in every building, tower, and bridge
A bridge is a structure built to span a physical obstacle (such as a body of water, valley, road, or rail) without blocking the way underneath. It is constructed for the purpose of providing passage over the obstacle, which is usually somethi ...
construction
Construction is a general term meaning the art and science to form objects, systems, or organizations,"Construction" def. 1.a. 1.b. and 1.c. ''Oxford English Dictionary'' Second Edition on CD-ROM (v. 4.0) Oxford University Press 2009 and com ...
project. As a countermeasure, shock mount
A shock mount or isolation mount is a mechanical fastener that connects two parts elastically. They are used for shock and vibration isolation.
Isolation mounts allow a piece of equipment to be securely mounted to a foundation and/or frame and, a ...
s can be installed to absorb resonant frequencies and thus dissipate the absorbed energy. The Taipei 101
Taipei 101 (; stylized as TAIPEI 101), formerly known as the Taipei World Financial Center, is a supertall skyscraper in Taipei, Taiwan. This building was officially classified as the world's tallest from its opening in 2004 until the 2009 ...
building relies on a —a tuned mass damper
A tuned mass damper (TMD), also known as a harmonic absorber or seismic damper, is a device mounted in structures to reduce mechanical vibrations, consisting of a mass mounted on one or more damped springs. Its oscillation frequency is tuned ...
—to cancel resonance. Furthermore, the structure is designed to resonate at a frequency that does not typically occur. Buildings in seismic
Seismology (; from Ancient Greek σεισμός (''seismós'') meaning "earthquake" and -λογία (''-logía'') meaning "study of") is the scientific study of earthquakes and the propagation of elastic waves through the Earth or through other ...
zones are often constructed to take into account the oscillating frequencies of expected ground motion
Ground motion is the movement of the earth's surface from earthquakes or explosions. Ground motion is produced by seismic waves that are generated by sudden slip on a fault or sudden pressure at the explosive source and travel through the earth a ...
. In addition, engineer
Engineers, as practitioners of engineering, are professionals who invent, design, analyze, build and test machines, complex systems, structures, gadgets and materials to fulfill functional objectives and requirements while considering the l ...
s designing objects having engines must ensure that the mechanical resonant frequencies of the component parts do not match driving vibrational frequencies of the motors or other strongly oscillating parts.
Clock
A clock or a timepiece is a device used to measure and indicate time. The clock is one of the oldest human inventions, meeting the need to measure intervals of time shorter than the natural units such as the day, the lunar month and the ...
s keep time by mechanical resonance in a balance wheel
A balance wheel, or balance, is the timekeeping device used in mechanical watches and small clocks, analogous to the pendulum in a pendulum clock. It is a weighted wheel that rotates back and forth, being returned toward its center position by a ...
, pendulum, or quartz crystal
Quartz is a hard, crystalline mineral composed of silica (silicon dioxide). The atoms are linked in a continuous framework of SiO4 silicon-oxygen tetrahedra, with each oxygen being shared between two tetrahedra, giving an overall chemical form ...
.
The cadence of runners has been hypothesized to be energetically favorable due to resonance between the elastic energy stored in the lower limb and the mass of the runner.
Acoustic resonance
Acoustic resonance is a phenomenon in which an acoustic system amplifies sound waves whose frequency matches one of its own natural frequencies of vibration (its ''resonance frequencies'').
The term "acoustic resonance" is sometimes used to nar ...
is a branch of mechanical resonance that is concerned with the mechanical vibrations across the frequency range of human hearing, in other words sound
In physics, sound is a vibration that propagates as an acoustic wave, through a transmission medium such as a gas, liquid or solid.
In human physiology and psychology, sound is the ''reception'' of such waves and their ''perception'' by the ...
. For humans, hearing is normally limited to frequencies between about 20 Hz and 20,000 Hz (20 kHz
The hertz (symbol: Hz) is the unit of frequency in the International System of Units (SI), equivalent to one event (or cycle) per second. The hertz is an SI derived unit whose expression in terms of SI base units is s−1, meaning that on ...
), Many objects and materials act as resonators with resonant frequencies within this range, and when struck vibrate mechanically, pushing on the surrounding air to create sound waves. This is the source of many percussive sounds we hear.
Acoustic resonance is an important consideration for instrument builders, as most acoustic instruments
Instrument may refer to:
Science and technology
* Flight instruments, the devices used to measure the speed, altitude, and pertinent flight angles of various kinds of aircraft
* Laboratory equipment, the measuring tools used in a scientific lab ...
use resonator
A resonator is a device or system that exhibits resonance or resonant behavior. That is, it naturally oscillates with greater amplitude at some frequencies, called resonant frequencies, than at other frequencies. The oscillations in a resonator ...
s, such as the strings
String or strings may refer to:
*String (structure), a long flexible structure made from threads twisted together, which is used to tie, bind, or hang other objects
Arts, entertainment, and media Films
* ''Strings'' (1991 film), a Canadian anim ...
and body of a violin
The violin, sometimes known as a ''fiddle'', is a wooden chordophone (string instrument) in the violin family. Most violins have a hollow wooden body. It is the smallest and thus highest-pitched instrument (soprano) in the family in regular ...
, the length of tube in a flute
The flute is a family of classical music instrument in the woodwind group. Like all woodwinds, flutes are aerophones, meaning they make sound by vibrating a column of air. However, unlike woodwind instruments with reeds, a flute is a reedless ...
, and the shape of, and tension on, a drum membrane.
Like mechanical resonance, acoustic resonance can result in catastrophic failure of the object at resonance. The classic example of this is breaking a wine glass with sound at the precise resonant frequency of the glass, although this is difficult in practice.
International Space Station
Therocket engine
A rocket engine uses stored rocket propellants as the reaction mass for forming a high-speed propulsive jet of fluid, usually high-temperature gas. Rocket engines are reaction engines, producing thrust by ejecting mass rearward, in accordanc ...
s for the International Space Station
The International Space Station (ISS) is the largest modular space station currently in low Earth orbit. It is a multinational collaborative project involving five participating space agencies: NASA (United States), Roscosmos (Russia), JAXA ...
(ISS) are controlled by an autopilot
An autopilot is a system used to control the path of an aircraft, marine craft or spacecraft without requiring constant manual control by a human operator. Autopilots do not replace human operators. Instead, the autopilot assists the operator' ...
. Ordinarily, uploaded parameters for controlling the engine control system for the Zvezda module make the rocket engines boost the International Space Station to a higher orbit. The rocket engines are hinge
A hinge is a mechanical bearing that connects two solid objects, typically allowing only a limited angle of rotation between them. Two objects connected by an ideal hinge rotate relative to each other about a fixed axis of rotation: all other ...
-mounted, and ordinarily the crew doesn't notice the operation. On January 14, 2009, however, the uploaded parameters made the autopilot swing the rocket engines in larger and larger oscillations, at a frequency of 0.5 Hz. These oscillations were captured on video, and lasted for 142 seconds.
Electrical
Electrical resonance
Electrical resonance occurs in an electric circuit at a particular '' resonant frequency'' when the impedances or admittances of circuit elements cancel each other. In some circuits, this happens when the impedance between the input and output of ...
occurs in an electric circuit at a particular ''resonant frequency'' when the impedance of the circuit is at a minimum in a series circuit or at maximum in a parallel circuit (usually when the transfer function peaks in absolute value). Resonance in circuits are used for both transmitting and receiving wireless communications such as television, cell phones and radio.
Optical
Anoptical cavity An optical cavity, resonating cavity or optical resonator is an arrangement of mirrors or other optical elements that forms a cavity resonator for light waves. Optical cavities are a major component of lasers, surrounding the gain medium and provi ...
, also called an ''optical resonator'', is an arrangement of mirror
A mirror or looking glass is an object that Reflection (physics), reflects an image. Light that bounces off a mirror will show an image of whatever is in front of it, when focused through the lens of the eye or a camera. Mirrors reverse the ...
s that forms a standing wave cavity resonator
A resonator is a device or system that exhibits resonance or resonant behavior. That is, it naturally oscillates with greater amplitude at some frequencies, called resonant frequencies, than at other frequencies. The oscillations in a resonator ...
for light wave
In physics, electromagnetic radiation (EMR) consists of waves of the electromagnetic (EM) field, which propagate through space and carry momentum and electromagnetic radiant energy. It includes radio waves, microwaves, infrared, (visible) ligh ...
s. Optical cavities are a major component of laser
A laser is a device that emits light through a process of optical amplification based on the stimulated emission of electromagnetic radiation. The word "laser" is an acronym for "light amplification by stimulated emission of radiation". The fir ...
s, surrounding the gain medium
The active laser medium (also called gain medium or lasing medium) is the source of optical gain within a laser. The gain results from the stimulated emission of photons through electronic or molecular transitions to a lower energy state from a ...
and providing feedback
Feedback occurs when outputs of a system are routed back as inputs as part of a chain of cause-and-effect that forms a circuit or loop. The system can then be said to ''feed back'' into itself. The notion of cause-and-effect has to be handled ...
of the laser light. They are also used in optical parametric oscillator
An optical parametric oscillator (OPO) is a parametric oscillator that oscillates at optical frequencies. It converts an input laser wave (called "pump") with frequency \omega_p into two output waves of lower frequency (\omega_s, \omega_i) by means ...
s and some interferometer
Interferometry is a technique which uses the ''interference'' of superimposed waves to extract information. Interferometry typically uses electromagnetic waves and is an important investigative technique in the fields of astronomy, fiber op ...
s. Light confined in the cavity reflects multiple times producing standing waves for certain resonant frequencies. The standing wave patterns produced are called "modes". Longitudinal mode
A longitudinal mode of a resonant cavity is a particular standing wave pattern formed by waves confined in the cavity. The longitudinal modes correspond to the wavelengths of the wave which are reinforced by constructive interference after many ...
s differ only in frequency while transverse mode
A transverse mode of electromagnetic radiation is a particular electromagnetic field pattern of the radiation in the plane perpendicular (i.e., transverse) to the radiation's propagation direction. Transverse modes occur in radio waves and microwav ...
s differ for different frequencies and have different intensity patterns across the cross-section of the beam. Ring resonators and whispering galleries are examples of optical resonators that do not form standing waves.
Different resonator types are distinguished by the focal lengths of the two mirrors and the distance between them; flat mirrors are not often used because of the difficulty of aligning them precisely. The geometry (resonator type) must be chosen so the beam remains stable, i.e., the beam size does not continue to grow with each reflection. Resonator types are also designed to meet other criteria such as minimum beam waist or having no focal point (and therefore intense light at that point) inside the cavity.
Optical cavities are designed to have a very large ''Q'' factor. A beam reflects a large number of times with little attenuation
In physics, attenuation (in some contexts, extinction) is the gradual loss of flux intensity through a medium. For instance, dark glasses attenuate sunlight, lead attenuates X-rays, and water and air attenuate both light and sound at variable att ...
—therefore the frequency line width
A spectral line is a dark or bright line in an otherwise uniform and continuous spectrum, resulting from emission (electromagnetic radiation), emission or absorption (electromagnetic radiation), absorption of light in a narrow frequency range, ...
of the beam is small compared to the frequency of the laser.
Additional optical resonances are guided-mode resonance
Guided-mode resonance or waveguide-mode resonance is a phenomenon wherein the guided modes of an optical waveguide can be excited and simultaneously extracted by the introduction of a phase-matching element, such as a diffraction grating or prism. ...
s and surface plasmon resonance
Surface plasmon resonance (SPR) is the resonant oscillation of conduction electrons at the interface between negative and positive permittivity material in a particle stimulated by incident light. SPR is the basis of many standard tools for measu ...
, which result in anomalous reflection and high evanescent fields at resonance. In this case, the resonant modes are guided modes of a waveguide or surface plasmon modes of a dielectric-metallic interface. These modes are usually excited by a subwavelength grating.
Orbital
Incelestial mechanics
Celestial mechanics is the branch of astronomy that deals with the motions of objects in outer space. Historically, celestial mechanics applies principles of physics (classical mechanics) to astronomical objects, such as stars and planets, to ...
, an orbital resonance
In celestial mechanics, orbital resonance occurs when orbiting bodies exert regular, periodic gravitational influence on each other, usually because their orbital periods are related by a ratio of small integers. Most commonly, this relationsh ...
occurs when two orbit
In celestial mechanics, an orbit is the curved trajectory of an object such as the trajectory of a planet around a star, or of a natural satellite around a planet, or of an artificial satellite around an object or position in space such as a p ...
ing bodies exert a regular, periodic gravitational influence on each other, usually due to their orbital period
The orbital period (also revolution period) is the amount of time a given astronomical object takes to complete one orbit around another object. In astronomy, it usually applies to planets or asteroids orbiting the Sun, moons orbiting planets ...
s being related by a ratio of two small integers. Orbital resonances greatly enhance the mutual gravitational influence of the bodies. In most cases, this results in an ''unstable'' interaction, in which the bodies exchange momentum and shift orbits until the resonance no longer exists. Under some circumstances, a resonant system can be stable and self-correcting, so that the bodies remain in resonance. Examples are the 1:2:4 resonance of Jupiter
Jupiter is the fifth planet from the Sun and the List of Solar System objects by size, largest in the Solar System. It is a gas giant with a mass more than two and a half times that of all the other planets in the Solar System combined, but ...
's moons Ganymede, Europa
Europa may refer to:
Places
* Europe
* Europa (Roman province), a province within the Diocese of Thrace
* Europa (Seville Metro), Seville, Spain; a station on the Seville Metro
* Europa City, Paris, France; a planned development
* Europa Cliff ...
, and Io, and the 2:3 resonance between Pluto
Pluto (minor-planet designation: 134340 Pluto) is a dwarf planet in the Kuiper belt, a ring of trans-Neptunian object, bodies beyond the orbit of Neptune. It is the ninth-largest and tenth-most-massive known object to directly orbit the S ...
and Neptune
Neptune is the eighth planet from the Sun and the farthest known planet in the Solar System. It is the fourth-largest planet in the Solar System by diameter, the third-most-massive planet, and the densest giant planet. It is 17 times ...
. Unstable resonances with Saturn
Saturn is the sixth planet from the Sun and the second-largest in the Solar System, after Jupiter. It is a gas giant with an average radius of about nine and a half times that of Earth. It has only one-eighth the average density of Earth; h ...
's inner moons give rise to gaps in the rings of Saturn
The rings of Saturn are the most extensive ring system of any planet in the Solar System. They consist of countless small particles, ranging in size from micrometers to meters, that orbit around Saturn. The ring particles are made almost entirel ...
. The special case of 1:1 resonance (between bodies with similar orbital radii) causes large Solar System bodies to clear the neighborhood around their orbits by ejecting nearly everything else around them; this effect is used in the current definition of a planet.
Atomic, particle, and molecular

Nuclear magnetic resonance
Nuclear magnetic resonance (NMR) is a physical phenomenon in which nuclei in a strong constant magnetic field are perturbed by a weak oscillating magnetic field (in the near field) and respond by producing an electromagnetic signal with a ...
(NMR) is the name given to a physical resonance phenomenon involving the observation of specific quantum mechanical
Quantum mechanics is a fundamental theory in physics that provides a description of the physical properties of nature at the scale of atoms and subatomic particles. It is the foundation of all quantum physics including quantum chemistry, qua ...
magnetic
Magnetism is the class of physical attributes that are mediated by a magnetic field, which refers to the capacity to induce attractive and repulsive phenomena in other entities. Electric currents and the magnetic moments of elementary particle ...
properties of an atom
Every atom is composed of a nucleus and one or more electrons bound to the nucleus. The nucleus is made of one or more protons and a number of neutrons. Only the most common variety of hydrogen has no neutrons.
Every solid, liquid, gas, and ...
ic nucleus
Nucleus ( : nuclei) is a Latin word for the seed inside a fruit. It most often refers to:
*Atomic nucleus, the very dense central region of an atom
*Cell nucleus, a central organelle of a eukaryotic cell, containing most of the cell's DNA
Nucle ...
in the presence of an applied, external magnetic field. Many scientific techniques exploit NMR phenomena to study molecular physics
Molecular physics is the study of the physical properties of molecules and molecular dynamics. The field overlaps significantly with physical chemistry, chemical physics, and quantum chemistry. It is often considered as a sub-field of atomic, mo ...
, crystal
A crystal or crystalline solid is a solid material whose constituents (such as atoms, molecules, or ions) are arranged in a highly ordered microscopic structure, forming a crystal lattice that extends in all directions. In addition, macros ...
s, and non-crystalline materials through NMR spectroscopy
Nuclear magnetic resonance spectroscopy, most commonly known as NMR spectroscopy or magnetic resonance spectroscopy (MRS), is a spectroscopic technique to observe local magnetic fields around atomic nuclei. The sample is placed in a magnetic fiel ...
. NMR is also routinely used in advanced medical imaging techniques, such as in magnetic resonance imaging
Magnetic resonance imaging (MRI) is a medical imaging technique used in radiology to form pictures of the anatomy and the physiological processes of the body. MRI scanners use strong magnetic fields, magnetic field gradients, and radio wave ...
(MRI).
All nuclei containing odd numbers of nucleon
In physics and chemistry, a nucleon is either a proton or a neutron, considered in its role as a component of an atomic nucleus. The number of nucleons in a nucleus defines the atom's mass number (nucleon number).
Until the 1960s, nucleons were ...
s have an intrinsic magnetic moment
In electromagnetism, the magnetic moment is the magnetic strength and orientation of a magnet or other object that produces a magnetic field. Examples of objects that have magnetic moments include loops of electric current (such as electromagnets ...
and angular momentum
In physics, angular momentum (rarely, moment of momentum or rotational momentum) is the rotational analog of linear momentum. It is an important physical quantity because it is a conserved quantity—the total angular momentum of a closed syst ...
. A key feature of NMR is that the resonant frequency of a particular substance is directly proportional to the strength of the applied magnetic field. It is this feature that is exploited in imaging techniques; if a sample is placed in a non-uniform magnetic field then the resonant frequencies of the sample's nuclei depend on where in the field they are located. Therefore, the particle can be located quite precisely by its resonant frequency.
Electron paramagnetic resonance
Electron paramagnetic resonance (EPR) or electron spin resonance (ESR) spectroscopy is a method for studying materials that have unpaired electrons. The basic concepts of EPR are analogous to those of nuclear magnetic resonance (NMR), but the spin ...
, otherwise known as ''electron spin resonance'' (ESR), is a spectroscopic technique similar to NMR, but uses unpaired electrons instead. Materials for which this can be applied are much more limited since the material needs to both have an unpaired spin and be paramagnetic
Paramagnetism is a form of magnetism whereby some materials are weakly attracted by an externally applied magnetic field, and form internal, induced magnetic fields in the direction of the applied magnetic field. In contrast with this behavior, d ...
.
The Mössbauer effect
The Mössbauer effect, or recoilless nuclear resonance fluorescence, is a physical phenomenon discovered by Rudolf Mössbauer in 1958. It involves the resonant and recoil-free emission and absorption of gamma radiation by atomic nuclei bound in ...
is the resonant and recoil
Recoil (often called knockback, kickback or simply kick) is the rearward thrust generated when a gun is being discharged. In technical terms, the recoil is a result of conservation of momentum, as according to Newton's third law the force requ ...
-free emission and absorption of gamma ray
A gamma ray, also known as gamma radiation (symbol γ or \gamma), is a penetrating form of electromagnetic radiation arising from the radioactive decay of atomic nuclei. It consists of the shortest wavelength electromagnetic waves, typically ...
photons by atoms bound in a solid form.
Resonance in particle physics appears in similar circumstances to classical physics
Classical physics is a group of physics theories that predate modern, more complete, or more widely applicable theories. If a currently accepted theory is considered to be modern, and its introduction represented a major paradigm shift, then the ...
at the level of quantum mechanics and quantum field theory
In theoretical physics, quantum field theory (QFT) is a theoretical framework that combines classical field theory, special relativity, and quantum mechanics. QFT is used in particle physics to construct physical models of subatomic particles and ...
. Resonances can also be thought of as unstable particles, with the formula in the Universal resonance curve section of this article applying if ''Γ'' is the particle's decay rate
Radioactive decay (also known as nuclear decay, radioactivity, radioactive disintegration, or nuclear disintegration) is the process by which an unstable atomic nucleus loses energy by radiation. A material containing unstable nuclei is consid ...
and ''Ω'' is the particle's mass ''M''. In that case, the formula comes from the particle's propagator
In quantum mechanics and quantum field theory, the propagator is a function that specifies the probability amplitude for a particle to travel from one place to another in a given period of time, or to travel with a certain energy and momentum. In ...
, with its mass replaced by the complex number ''M'' + ''iΓ''. The formula is further related to the particle's decay rate by the optical theorem
In physics, the optical theorem is a general law of wave scattering theory, which relates the forward scattering amplitude to the total cross section of the scatterer. It is usually written in the form
:\sigma_\mathrm=\frac~\mathrm\,f(0),
where (0 ...
.
Disadvantages
A column of soldiers marching in regular step on a narrow and structurally flexible bridge can set it into dangerously largeamplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of amplit ...
oscillation
Oscillation is the repetitive or periodic variation, typically in time, of some measure about a central value (often a point of equilibrium) or between two or more different states. Familiar examples of oscillation include a swinging pendulum ...
s. April 12, 1831, the Broughton Suspension Bridge
Broughton Suspension Bridge was an iron chain suspension bridge built in 1826 to span the River Irwell between Broughton and Pendleton, now in Salford, Greater Manchester, England. One of Europe's first suspension bridges, it has been attrib ...
near Salford, England
Salford () is a city and the largest settlement in the City of Salford metropolitan borough in Greater Manchester, England. In 2011, Salford had a population of 103,886. It is also the second and only other city in the metropolitan county afte ...
collapsed while a group of British soldiers were marching across. Since then, the British Army has had a standing order for soldiers to break stride when marching across bridges, to avoid resonance from their regular marching pattern affecting the bridge.
Vibrations of a motor or engine can induce resonant vibration in its supporting structures if their natural frequency
Natural frequency, also known as eigenfrequency, is the frequency at which a system tends to oscillate in the absence of any driving force.
The motion pattern of a system oscillating at its natural frequency is called the normal mode (if all par ...
is close to that of the vibrations of the engine. A common example is the rattling sound of a bus body when the engine is left idling.
Structural resonance of a suspension bridge induced by winds can lead to its catastrophic collapse. Several early suspension bridges in Europe
Europe is a large peninsula conventionally considered a continent in its own right because of its great physical size and the weight of its history and traditions. Europe is also considered a Continent#Subcontinents, subcontinent of Eurasia ...
and USA
The United States of America (U.S.A. or USA), commonly known as the United States (U.S. or US) or America, is a country primarily located in North America. It consists of 50 states, a federal district, five major unincorporated territori ...
were destroyed by structural resonance induced by modest winds. The collapse of the Tacoma Narrows Bridge
The Tacoma Narrows Bridge is a pair of twin suspension bridges that span the Tacoma Narrows strait of Puget Sound in Pierce County, Washington. The bridges connect the city of Tacoma with the Kitsap Peninsula and carry State Route 16 (know ...
on 7 November 1940 is characterized in physics as a classic example of resonance. It has been argued by Robert H. Scanlan and others that the destruction was instead caused by aeroelastic flutter
Aeroelasticity is the branch of physics and engineering studying the interactions between the inertial, elastic, and aerodynamic forces occurring while an elastic body is exposed to a fluid flow. The study of aeroelasticity may be broadly classif ...
, a complicated interaction between the bridge and the winds passing through it—an example of a self oscillation
Self-oscillation is the generation and maintenance of a periodic motion by a source of power that lacks any corresponding periodicity. The oscillator itself controls the phase with which the external power acts on it. Self-oscillators are therefor ...
, or a kind of "self-sustaining vibration" as referred to in the nonlinear theory of vibrations.
Q factor
dimensionless
A dimensionless quantity (also known as a bare quantity, pure quantity, or scalar quantity as well as quantity of dimension one) is a quantity to which no physical dimension is assigned, with a corresponding SI unit of measurement of one (or 1) ...
parameter that describes how under-damped an oscillator
Oscillation is the repetitive or periodic variation, typically in time, of some measure about a central value (often a point of equilibrium) or between two or more different states. Familiar examples of oscillation include a swinging pendulum ...
or resonator is, and characterizes the bandwidth
Bandwidth commonly refers to:
* Bandwidth (signal processing) or ''analog bandwidth'', ''frequency bandwidth'', or ''radio bandwidth'', a measure of the width of a frequency range
* Bandwidth (computing), the rate of data transfer, bit rate or thr ...
of a resonator relative to its center frequency.
A high value for ''Q'' indicates a lower rate of energy loss relative to the stored energy, i.e., the system is lightly damped. The parameter is defined by the equation:
.
The higher the Q factor, the greater the amplitude at the resonant frequency, and the smaller the ''bandwidth'', or range of frequencies around resonance occurs. In electrical resonance, a high-''Q'' circuit in a radio receiver is more difficult to tune, but has greater selectivity
Selectivity may refer to:
Psychology and behaviour
* Choice, making a selection among options
* Discrimination, the ability to recognize differences
* Socioemotional selectivity theory, in social psychology
Engineering
* Selectivity (radio), a ...
, and so would be better at filtering out signals from other stations. High Q oscillators are more stable.
Examples that normally have a low Q factor include door closer
A door closer is defined as any mechanical device that closes a door in a controlled manner, preventing it from slamming, in general after someone opens it, or after it was automatically opened. The force used to open the door is stored in some ...
s (Q=0.5). Systems with high Q factors include tuning fork
A tuning fork is an acoustic resonator in the form of a two-pronged fork with the prongs (tines) formed from a U-shaped bar of elastic metal (usually steel). It resonates at a specific constant pitch when set vibrating by striking it against ...
s (Q=1000), atomic clock
An atomic clock is a clock that measures time by monitoring the resonant frequency of atoms. It is based on atoms having different energy levels. Electron states in an atom are associated with different energy levels, and in transitions betwee ...
s and lasers (Q≈1011).
Universal resonance curve
simple harmonic oscillator
In mechanics and physics, simple harmonic motion (sometimes abbreviated ) is a special type of periodic motion of a body resulting from a dynamic equilibrium between an inertial force, proportional to the acceleration of the body away from the ...
above.
For a lightly damped linear oscillator with a resonance frequency ''Ω'', the ''intensity'' of oscillations ''I'' when the system is driven with a driving frequency ''ω'' is typically approximated by a formula that is symmetric about the resonance frequency:
Where the susceptibility links the amplitude of the oscillator to the driving force in frequency space:
The intensity is defined as the square of the amplitude of the oscillations. This is a Lorentzian function
The Cauchy distribution, named after Augustin Cauchy, is a continuous probability distribution. It is also known, especially among physicists, as the Lorentz distribution (after Hendrik Lorentz), Cauchy–Lorentz distribution, Lorentz(ian) funct ...
, or Cauchy distribution, and this response is found in many physical situations involving resonant systems. is a parameter dependent on the damping of the oscillator, and is known as the ''linewidth'' of the resonance. Heavily damped oscillators tend to have broad linewidths, and respond to a wider range of driving frequencies around the resonant frequency. The linewidth is Proportionality (mathematics), inversely proportional to the ''Q'' factor, which is a measure of the sharpness of the resonance.
In radio engineering and electronics engineering, this approximate symmetric response is known as the ''universal resonance curve'', a concept introduced by Frederick E. Terman in 1932 to simplify the approximate analysis of radio circuits with a range of center frequencies and ''Q'' values.
See also
* Cymatics * Driven harmonic motion * Earthquake engineering * Electric dipole spin resonance * Formant * Limbic resonance * Nonlinear resonance * Normal mode * Positive feedback * Schumann resonance * Simple harmonic motion * Stochastic resonance * Sympathetic stringNotes
References
* * * * * * * * * * * * * *External links
The Feynman Lectures on Physics Vol. I Ch. 23: Resonance
- a chapter from an online textbook *Brian Greene, Greene, Brian, "
Resonance in strings
'". The Elegant Universe, Nova (American TV program), NOVA (Public Broadcasting Service, PBS)
Hyperphysics section on resonance concepts
(usage of terms)
demonstrating resonances on a string when the frequency of the driving force is varied
demonstrating the occurrence of resonance when the driving frequency matches with the natural frequency of an oscillator
Breaking glass with sound
including high-speed footage of glass breaking {{Authority control Resonance, Antennas (radio) Oscillation