Pseudomanifold on:
[Wikipedia]
[Google]
[Amazon]
In 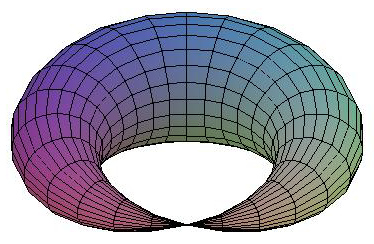 A pseudomanifold can be regarded as a combinatorial realisation of the general idea of a
A pseudomanifold can be regarded as a combinatorial realisation of the general idea of a
 Nevertheless it is always possible to decompose a non-pseudomanifold surface into manifold parts cutting only at singular edges and vertices (see Figure 2 in blue). For some surfaces several non-equivalent options are possible (see Figure 3).
Nevertheless it is always possible to decompose a non-pseudomanifold surface into manifold parts cutting only at singular edges and vertices (see Figure 2 in blue). For some surfaces several non-equivalent options are possible (see Figure 3).
 On the other hand, in higher dimension, for n>2, the situation becomes rather tricky.
* In general, for n≥3, n-pseudomanifolds cannot be decomposed into manifold parts only by cutting at singularities (see Figure 4).
On the other hand, in higher dimension, for n>2, the situation becomes rather tricky.
* In general, for n≥3, n-pseudomanifolds cannot be decomposed into manifold parts only by cutting at singularities (see Figure 4).
 * For n≥3, there are n-complexes that cannot be decomposed, even into pseudomanifold parts, only by cutting at singularities.
* For n≥3, there are n-complexes that cannot be decomposed, even into pseudomanifold parts, only by cutting at singularities.
mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
, a pseudomanifold is a special type of topological space. It looks like a manifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimensional manifold, or ''n-manifold'' for short, is a topological space with the property that each point has a n ...
at most of its points, but it may contain singularities. For example, the cone of solutions of forms a pseudomanifold.
 A pseudomanifold can be regarded as a combinatorial realisation of the general idea of a
A pseudomanifold can be regarded as a combinatorial realisation of the general idea of a manifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimensional manifold, or ''n-manifold'' for short, is a topological space with the property that each point has a n ...
with singularities. The concepts of orientability, orientation and degree of a mapping
In topology, the degree of a continuous mapping between two compact oriented manifolds of the same dimension is a number that represents the number of times that the domain manifold wraps around the range manifold under the mapping. The de ...
make sense for pseudomanifolds and moreover, within the combinatorial approach, pseudomanifolds form the natural domain of definition for these concepts.
Definition
A topological space ''X'' endowed with atriangulation
In trigonometry and geometry, triangulation is the process of determining the location of a point by forming triangles to the point from known points.
Applications
In surveying
Specifically in surveying, triangulation involves only angle me ...
''K'' is an ''n''-dimensional pseudomanifold if the following conditions hold:
# (''pure'') is the union of all ''n''- simplices.
# Every is a face of exactly one or two ''n''-simplices for ''n > 1''.
# For every pair of ''n''-simplices σ and σ' in ''K'', there is a sequence of ''n''-simplices such that the intersection
In mathematics, the intersection of two or more objects is another object consisting of everything that is contained in all of the objects simultaneously. For example, in Euclidean geometry, when two lines in a plane are not parallel, their i ...
is an for all ''i'' = 0, ..., ''k''−1.
Implications of the definition
*Condition 2 means that ''X'' is a non-branchingsimplicial complex
In mathematics, a simplicial complex is a set composed of points, line segments, triangles, and their ''n''-dimensional counterparts (see illustration). Simplicial complexes should not be confused with the more abstract notion of a simplicial set ...
.
*Condition 3 means that ''X'' is a strongly connected simplicial complex.
*If we require Condition 2 to hold only for in sequences of in Condition 3, we obtain an equivalent definition only for n=2. For n≥3 there are examples of combinatorial non-pseudomanifolds that are strongly connected through sequences of satisfying Condition 2.
Decomposition
Strongly connected n-complexes can always be assembled from gluing just two of them at . However, in general, construction by gluing can lead to non-pseudomanifoldness (see Figure 2).Related definitions
*A pseudomanifold is called ''normal'' if the link of each simplex withcodimension
In mathematics, codimension is a basic geometric idea that applies to subspaces in vector spaces, to submanifolds in manifolds, and suitable subsets of algebraic varieties.
For affine and projective algebraic varieties, the codimension equals the ...
≥ 2 is a pseudomanifold.
Examples
*A pinched torus (see Figure 1) is an example of an orientable, compact 2-dimensional pseudomanifold. (Note that a pinched torus is not a normal pseudomanifold, since the link of a vertex is not connected.) * Complex algebraic varieties (even with singularities) are examples of pseudomanifolds. (Note that real algebraic varieties aren't always pseudomanifolds, since their singularities can be of codimension 1, take xy=0 for example.) * Thom spaces of vector bundles over triangulable compact manifolds are examples of pseudomanifolds. * Triangulable, compact, connected, homology manifolds over Z are examples of pseudomanifolds. * Complexes obtained gluing two 4-simplices at a common tetrahedron are a proper superset of 4-pseudomanifolds used in spin foam formulation ofloop quantum gravity
Loop quantum gravity (LQG) is a theory of quantum gravity, which aims to merge quantum mechanics and general relativity, incorporating matter of the Standard Model into the framework established for the pure quantum gravity case. It is an attem ...
.
* Combinatorial n-complexes defined by gluing two at a are not always n-pseudomanifolds. Gluing can induce non-pseudomanifoldness.
See also
* Stratified spaceReferences
{{Reflist Topological spaces Manifolds