A logarithmic spiral, equiangular spiral, or growth spiral is a

self-similar __NOTOC__ In mathematics, a self-similar object is exactly or approximately similar to a part of itself (i.e., the whole has the same shape as one or more of the parts). Many objects in the real world, such as coastlines, are statistically se ...

spiral

curve In mathematics, a curve (also called a curved line in older texts) is an object similar to a line (geometry), line, but that does not have to be Linearity, straight. Intuitively, a curve may be thought of as the trace left by a moving point (ge ...

that often appears in nature. The first to describe a logarithmic

spiral In mathematics, a spiral is a curve which emanates from a point, moving farther away as it revolves around the point. Helices Two major definitions of "spiral" in the American Heritage Dictionary are:Albrecht Dürer Albrecht Dürer (; ; hu, Ajtósi Adalbert; 21 May 1471 – 6 April 1528),Müller, Peter O. (1993) ''Substantiv-Derivation in Den Schriften Albrecht Dürers'', Walter de Gruyter. . sometimes spelled in English as Durer (without an umlaut) or Due ...

(1525) who called it an "eternal line" ("ewige Linie"). More than a century later, the curve was discussed by Descartes (1638), and later extensively investigated by Jacob Bernoulli, who called it ''Spira mirabilis'', "the marvelous spiral". The logarithmic spiral can be distinguished from the

Archimedean spiral The Archimedean spiral (also known as the arithmetic spiral) is a spiral named after the 3rd-century BC Greek mathematician Archimedes. It is the locus corresponding to the locations over time of a point moving away from a fixed point with a con ...

by the fact that the distances between the turnings of a logarithmic spiral increase in geometric progression, while in an Archimedean spiral these distances are constant.

Definition

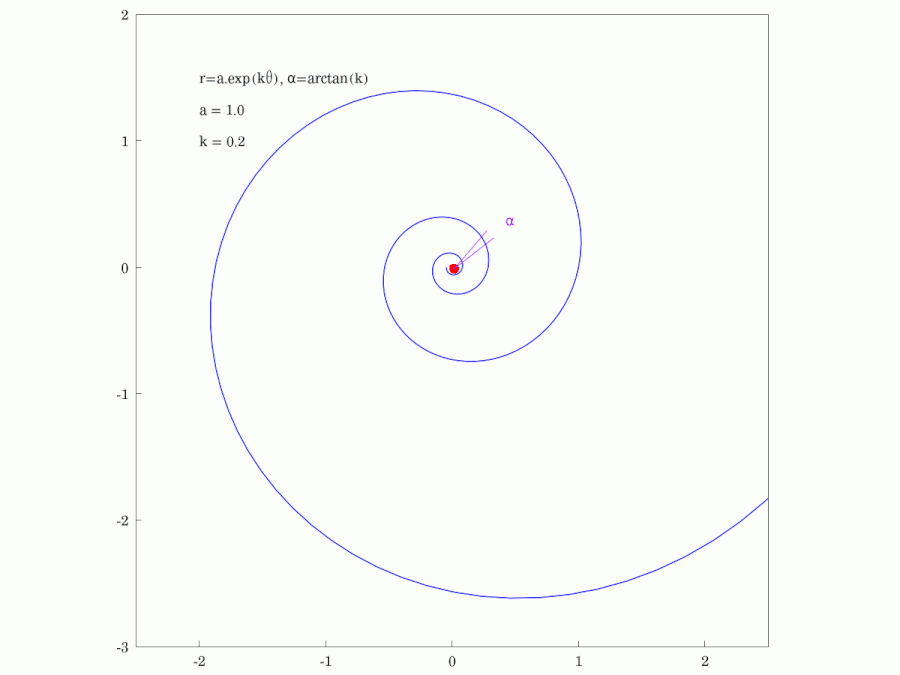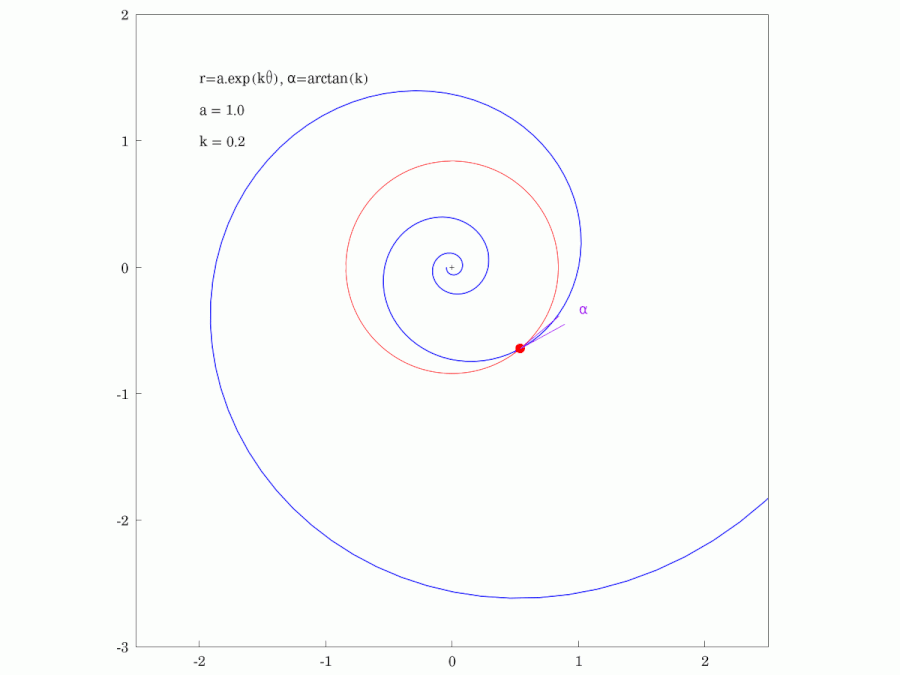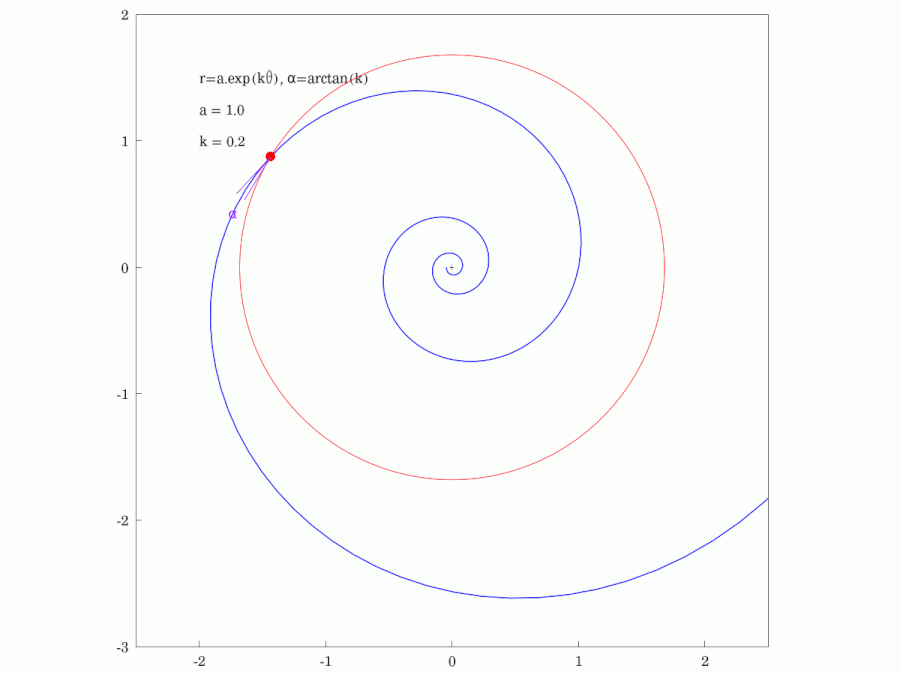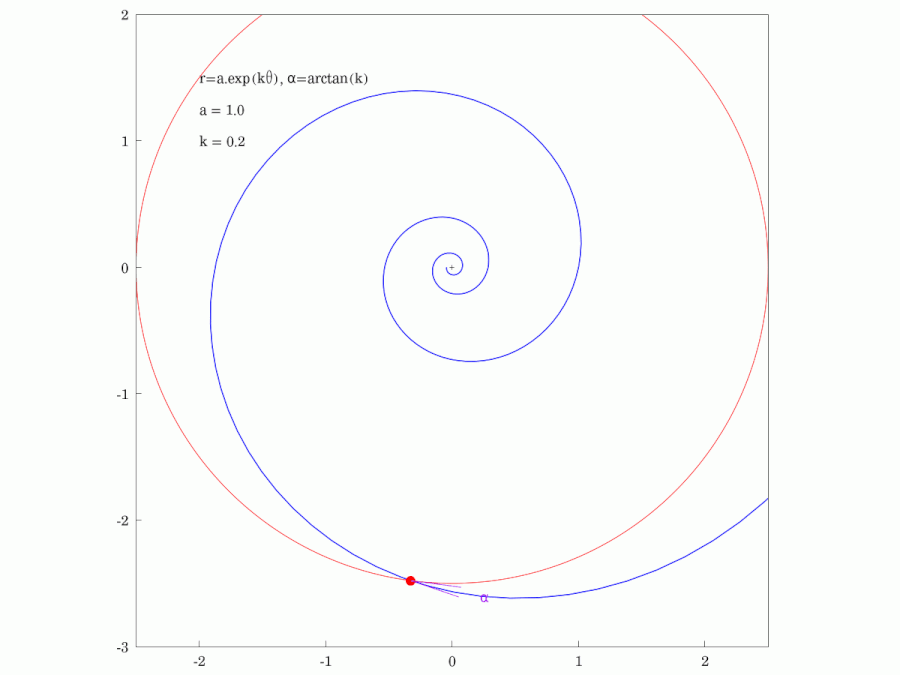
In polar coordinates

(r, \varphi)

the logarithmic spiral can be written as

r = ae^,\quad \varphi  \in \R,

\varphi = \frac \ln \frac,

with

e

being the base of

natural logarithm The natural logarithm of a number is its logarithm to the base of the mathematical constant , which is an irrational and transcendental number approximately equal to . The natural logarithm of is generally written as , , or sometimes, if ...

s, and

a > 0

k\ne 0

being real constants.

In Cartesian coordinates

The logarithmic spiral with the polar equation

r = a e^

can be represented in Cartesian coordinates

(x=r\cos\varphi,\, y=r\sin\varphi)

x = a e^\cos \varphi, \qquad y = a e^\sin \varphi.

In the

complex plane In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by the ...

(z=x+iy,\, e^=\cos\varphi + i\sin\varphi)

z=ae^.

''Spira mirabilis'' and Jacob Bernoulli

''Spira mirabilis'', Latin for "miraculous spiral", is another name for the logarithmic spiral. Although this curve had already been named by other mathematicians, the specific name ("miraculous" or "marvelous" spiral) was given to this curve by Jacob Bernoulli, because he was fascinated by one of its unique mathematical properties: the size of the spiral increases but its shape is unaltered with each successive curve, a property known as self-similarity. Possibly as a result of this unique property, the spira mirabilis has evolved in nature, appearing in certain growing forms such as nautilus shells and

sunflower The common sunflower (''Helianthus annuus'') is a large annual forb of the genus ''Helianthus'' grown as a crop for its edible oily seeds. Apart from cooking oil production, it is also used as livestock forage (as a meal or a silage plant), as ...

heads. Jacob Bernoulli wanted such a spiral engraved on his

headstone A headstone, tombstone, or gravestone is a stele or marker, usually stone, that is placed over a grave. It is traditional for burials in the Christian, Jewish, and Muslim religions, among others. In most cases, it has the deceased's name, da ...

along with the phrase " Eadem mutata resurgo" ("Although changed, I shall arise the same."), but, by error, an

was placed there instead.

Properties

The logarithmic spiral

r=a e^ \;,\; k\ne 0,

has the following properties (see

A=\frac

* Inversion: Circle inversion (

r\to 1/r

) maps the logarithmic spiral

r=a e^

onto the logarithmic spiral

r=\tfrac e^ \, .

* Rotating, scaling: Rotating the spiral by angle

\varphi_0

yields the spiral

r=ae^e^

, which is the original spiral uniformly scaled (at the origin) by

e^

. Scaling by

\;e^\; , n=\pm 1,\pm2,...,\;

gives the ''same'' curve. * Self-similarity: A result of the previous property: A scaled logarithmic spiral is congruent (by rotation) to the original curve. ''Example:'' The diagram shows spirals with slope angle

\alpha=20^\circ

and

a=1,2,3,4,5

. Hence they are all scaled copies of the red one. But they can also be generated by rotating the red one by angles

-109^\circ,-173^\circ,-218^\circ,-253^\circ

resp.. All spirals have no points in common (see property on ''complex exponential function''). * Relation to other curves: Logarithmic spirals are congruent to their own involutes, evolutes, and the

pedal curve A pedal (from the Latin '' pes'' ''pedis'', "foot") is a lever designed to be operated by foot and may refer to: Computers and other equipment * Footmouse, a foot-operated computer mouse * In medical transcription, a pedal is used to control p ...

s based on their centers. * Complex exponential function: The exponential function exactly maps all lines not parallel with the real or imaginary axis in the complex plane, to all logarithmic spirals in the complex plane with centre at

0

z(t)=\underbrace_\quad  \to\quad  e^=e^\cdot e^= \underbrace_

The polar slope angle

\alpha

of the logarithmic spiral is the angle between the line and the imaginary axis.

Special cases and approximations

The golden spiral is a logarithmic spiral that grows outward by a factor of the golden ratio for every 90 degrees of rotation (polar slope angle about 17.03239 degrees). It can be approximated by a "Fibonacci spiral", made of a sequence of quarter circles with radii proportional to Fibonacci numbers.

In nature

Nautilus Cutaway with Logarithmic Spiral

In several natural phenomena one may find curves that are close to being logarithmic spirals. Here follow some examples and reasons: *The approach of a

hawk Hawks are bird of prey, birds of prey of the family Accipitridae. They are widely distributed and are found on all continents except Antarctica. * The subfamily Accipitrinae includes goshawks, sparrowhawks, sharp-shinned hawks and others. Th ...

to its prey in classical pursuit, assuming the prey travels in a straight line. Their sharpest view is at an angle to their direction of flight; this angle is the same as the spiral's pitch. *The approach of an insect to a light source. They are used to having the light source at a constant angle to their flight path. Usually the sun (or moon for nocturnal species) is the only light source and flying that way will result in a practically straight line. *The arms of spiral

galaxies A galaxy is a system of stars, stellar remnants, interstellar gas, dust, dark matter, bound together by gravity. The word is derived from the Greek ' (), literally 'milky', a reference to the Milky Way galaxy that contains the Solar System. ...

. Our own galaxy, the Milky Way, has several spiral arms, each of which is roughly a logarithmic spiral with pitch of about 12 degrees. However, although spiral galaxies have often been modeled as logarithmic spirals,

s, or hyperbolic spirals, their pitch angles vary with distance from the galactic center, unlike logarithmic spirals (for which this angle does not vary), and also at variance with the other mathematical spirals used to model them. *The nerves of the cornea (this is, corneal nerves of the subepithelial layer terminate near superficial epithelial layer of the cornea in a logarithmic spiral pattern).C. Q. Yu CQ and M. I. Rosenblatt, "Transgenic corneal neurofluorescence in mice: a new model for in vivo investigation of nerve structure and regeneration," Invest Ophthalmol Vis Sci. 2007 Apr;48(4):1535-42. *The bands of tropical cyclones, such as hurricanes. *Many

biological Biology is the scientific study of life. It is a natural science with a broad scope but has several unifying themes that tie it together as a single, coherent field. For instance, all organisms are made up of cells that process hereditary in ...

structures including the shells of mollusks. In these cases, the reason may be construction from expanding similar shapes, as is the case for polygonal figures. *

Logarithmic spiral beaches A logarithmic spiral beach is a type of beach which develops in the direction under which it is sheltered by a headland, in an area called the ''shadow zone''. It is shaped like a logarithmic spiral when seen in a map, plan view, or aerial photog ...

can form as the result of wave refraction and diffraction by the coast.

Half Moon Bay (California) Half Moon Bay is a bay of the Pacific Ocean on the coast of San Mateo County, California. The bay is approximately semi-circular, hence the name half moon, with sea access to the south. Coastal towns located there are Princeton-by-the-Sea, ...

is an example of such a type of beach.

In engineering applications

* Logarithmic spiral antennas are frequency-independent antennas, that is, antennas whose radiation pattern, impedance and polarization remain largely unmodified over a wide bandwidth. * When manufacturing mechanisms by subtractive fabrication machines (such as

laser cutters Laser cutting is a technology that uses a laser to vaporize materials, resulting in a cut edge. While typically used for industrial manufacturing applications, it is now used by schools, small businesses, architecture, and hobbyists. Laser cutt ...

), there can be a loss of precision when the mechanism is fabricated on a different machine due to the difference of material removed (that is, the kerf) by each machine in the cutting process. To adjust for this variation of kerf, the self-similar property of the logarithmic spiral has been used to design a kerf cancelling mechanism for laser cutters. *Logarithmic spiral bevel gears are a type of spiral bevel gear whose gear tooth centerline is a logarithmic spiral. A logarithmic spiral has the advantage of providing equal angles between the tooth centerline and the radial lines, which gives the meshing transmission more stability.

References

* * Jim Wilson
Equiangular Spiral (or Logarithmic Spiral) and Its Related Curves
University of Georgia (1999) * Alexander Bogomolny
Spira Mirabilis - Wonderful Spiral
at cut-the-knot

External links

history and math * *
''SpiralZoom.com''
an educational website about the science of pattern formation, spirals in nature, and spirals in the mythic imagination.
Online exploration using JSXGraph (JavaScript)

YouTube lecture on Zeno's mice problem and logarithmic spirals
{{Spirals Spirals

Spiral In mathematics, a spiral is a curve which emanates from a point, moving farther away as it revolves around the point. Helices Two major definitions of "spiral" in the American Heritage Dictionary are:Spiral In mathematics, a spiral is a curve which emanates from a point, moving farther away as it revolves around the point. Helices Two major definitions of "spiral" in the American Heritage Dictionary are:Plane curves