Kronig-Penney Model

In quantum mechanics, the particle in a one-dimensional lattice is a problem that occurs in the model of a periodic crystal lattice. The potential is caused by ions in the periodic structure of the crystal creating an electromagnetic field so electrons are subject to a regular potential inside the lattice. It is a generalization of the free electron model, which assumes zero potential inside the lattice.

Problem definition

When talking about solid materials, the discussion is mainly around crystals – periodic lattices. Here we will discuss a 1D lattice of positive ions. Assuming the spacing between two ions is , the potential in the lattice will look something like this:

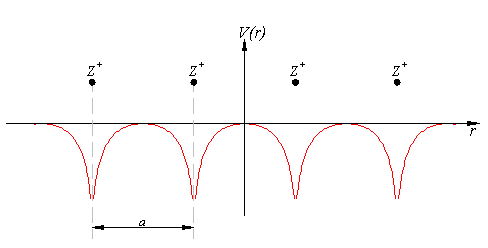
The mathematical representation of the potential is a periodic function with a period . According to Bloch's theorem, the wavefunction solution of the Schrödinger equation when the potential is periodic, can be written as:

$\psi (x) = e^ u(x),$

where is a periodic function which satisfies . It is the Bloch factor with Floquet exponent $k$ which gives rise to the band structure of the energy spectrum of the Schrödinger equation with a periodic potential like the Kronig–Penney potential or a cosine function as in the Mathieu equation.

When nearing the edges of the lattice, there are problems with the boundary condition. Therefore, we can represent the ion lattice as a ring following the Born–von Karman boundary conditions. If is the length of the lattice so that , then the number of ions in the lattice is so big, that when considering one ion, its surrounding is almost linear, and the wavefunction of the electron is unchanged. So now, instead of two boundary conditions we get one circular boundary condition:
$\psi (0)=\psi (L).$

If is the number of ions in the lattice, then we have the relation: . Replacing in the boundary condition and applying Bloch's theorem will result in a quantization for :
$\psi (0) = e^ u(0) = e^ u(L) = \psi (L)$
$u(0) = e^ u(L)=e^ u(N a) \to e^ = 1$
$\Rightarrow kL = 2\pi n \to k = n \qquad \left( n=0, \pm 1, \dots, \pm \frac \right).$

Kronig–Penney model

The Kronig–Penney model (named after Ralph Kronig and William Penney) is a simple, idealized quantum-mechanical system that consists of an infinite periodic array of rectangular potential barriers.

The potential function is approximated by a rectangular potential:

Using Bloch's theorem, we only need to find a solution for a single period, make sure it is continuous and smooth, and to make sure the function is also continuous and smooth.

Considering a single period of the potential:

We have two regions here. We will solve for each independently:
Let ''E'' be an energy value above the well (E>0)
* For $0 < x < (a-b)$: $\begin \frac \psi_ &= E \psi \\ \Rightarrow \psi &= A e^ + A' e^ & \left( \alpha^2 = \right) \end$
*For -b : $\begin \frac \psi_ &= (E+V_0)\psi \\ \Rightarrow \psi &= B e^ + B' e^ & \left( \beta^2 = \right). \end$

To find ''u''(''x'') in each region, we need to manipulate the electron's wavefunction:
\begin
\psi(0

And in the same manner:
u(-b

To complete the solution we need to make sure the probability function is continuous and smooth, i.e.:
$\psi(0^)=\psi(0^) \qquad \psi'(0^)=\psi'(0^).$

And that and are periodic:
$u(-b)=u(a-b) \qquad u'(-b)=u'(a-b).$

These conditions yield the following matrix:
$\begin 1 & 1 & -1 & -1 \\ \alpha & -\alpha & -\beta & \beta \\ e^ & e^ & -e^ & -e^ \\ (\alpha-k)e^ & -(\alpha+k)e^ & -(\beta-k)e^ & (\beta+k)e^ \end \begin A \\ A' \\ B \\ B' \end = \begin 0 \\ 0 \\ 0 \\ 0 \end.$

For us to have a non-trivial solution, the determinant of the matrix must be 0. This leads us to the following expression:
$\cos(k a) = \cos(\beta b) \cos$alpha(a-b) \sin(\beta b) \sinalpha(a-b)

To further simplify the expression, we perform the following approximations:
$b \to 0; \quad V_0 \to \infty; \quad V_0 b = \mathrm$
$\Rightarrow \beta^2 b = \mathrm; \quad \alpha^2 b \to 0$
$\Rightarrow \beta b \to 0; \quad \sin(\beta b) \to \beta b; \quad \cos(\beta b) \to 1.$

The expression will now be:
$\cos(k a) = \cos(\alpha a)+P \frac, \qquad P= \frac.$

For energy values inside the well (''E'' < 0), we get:
$\cos(k a) = \cos(\beta b) \cosh$alpha(a-b) \sin(\beta b) \sinhalpha(a-b)
with $\alpha^2 =$ and $\beta^2 = \frac$.

Following the same approximations as above ($b \to 0; \, V_0 \to \infty; \, V_0 b = \mathrm$), we arrive at
$\cos(k a) = \cosh(\alpha a) + P \frac$
with the same formula for ''P'' as in the previous case $\left(P = \frac\right)$.

Band gaps in the Kronig–Penney model

In the previous paragraph, the only variables not determined by the parameters of the physical system are the energy ''E'' and the crystal momentum ''k''. By picking a value for ''E'', one can compute the right hand side, and then compute ''k'' by taking the $\arccos$ of both sides. Thus, the expression gives rise to the dispersion relation.

The right hand side of the last expression above can sometimes be greater than 1 or less than –1, in which case there is no value of ''k'' that can make the equation true. Since $\alpha a \propto \sqrt$, that means there are certain values of ''E'' for which there are no eigenfunctions of the Schrödinger equation. These values constitute the band gap.

Thus, the Kronig–Penney model is one of the simplest periodic potentials to exhibit a band gap.

Kronig–Penney model: alternative solution

An alternative treatment to a similar problem is given. Here we have a ''delta'' periodic potential:
$V(x) = A\cdot\sum_^\delta(x - n a).$

is some constant, and is the lattice constant (the spacing between each site). Since this potential is periodic, we could expand it as a Fourier series:
$V(x) = \sum_K \tilde(K)\cdot e^,$
where
$\tilde(K) = \frac\int_^dx\,V(x)\,e^ = \frac\int_^ dx \sum_^ A\cdot \delta(x-na)\,e^ = \frac.$

The wave-function, using Bloch's theorem, is equal to $\psi_k(x) = e^ u_k(x)$ where $u_k(x)$ is a function that is periodic in the lattice, which means that we can expand it as a Fourier series as well:
$u_k(x)=\sum_ \tilde_k(K)e^.$

Thus the wave function is:
$\psi_k(x)=\sum_\tilde_k(K)\,e^.$

Putting this into the Schrödinger equation, we get:
\left frac-E_k\right\tilde_k(K)+\sum_\tilde(K-K')\,\tilde_k(K') = 0
or rather:
\left frac-E_k\right\tilde_k(K)+\frac\sum_\tilde_k(K')=0

Now we recognize that:
$u_k(0)=\sum_\tilde_k(K')$

Plug this into the Schrödinger equation:
\left frac-E_k\right\tilde_k(K)+\fracu_k(0)=0

Solving this for $\tilde_k(K)$ we get:
$\tilde_k(K)=\frac=\frac\,u_k(0)$

We sum this last equation over all values of to arrive at:
$\sum_\tilde_k(K)=\sum_\frac\,u_k(0)$

Or:
$u_k(0)=\sum_\frac\,u_k(0)$

Conveniently, $u_k(0)$ cancels out and we get:
$1=\sum_\frac$

Or:
$\frac\frac=\sum_\frac$

To save ourselves some unnecessary notational effort we define a new variable:
$\alpha^2 := \frac$
and finally our expression is:
$\frac\frac=\sum_\frac$

Now, is a reciprocal lattice vector, which means that a sum over is actually a sum over integer multiples of $\frac$:
$\frac\frac=\sum_^\frac$

We can juggle this expression a little bit to make it more suggestive (use Partial fraction decomposition):
\begin
\frac\frac &= \sum_^\frac \\
&=-\frac\sum_^\left frac-\frac\right\\
&=-\frac\sum_^\left frac-\frac \right\\
&=-\frac\left sum_^\frac - \sum_^\frac \right\end

If we use a nice identity of a sum of the cotangent function
Equation 18
which says:
$\cot(x)=\sum_^\frac-\frac$
and plug it into our expression we get to:
\frac\frac = -\frac\left cot\left(\tfrac-\tfrac\right) - \cot\left(\tfrac+\tfrac\right)\right/math>

We use the sum of and then, the product of (which is part of the formula for the sum of ) to arrive at:
$\cos(k a)=\cos(\alpha a)+\frac\sin(\alpha a)$

This equation shows the relation between the energy (through ) and the wave-vector, , and as you can see, since the left hand side of the equation can only range from to then there are some limits on the values that (and thus, the energy) can take, that is, at some ranges of values of the energy, there is no solution according to these equation, and thus, the system will not have those energies: energy gaps. These are the so-called band-gaps, which can be shown to exist in ''any'' shape of periodic potential (not just delta or square barriers).

For a different and detailed calculation of the gap formula (i.e. for the gap between bands) and the level splitting of eigenvalues of the one-dimensional Schrödinger equation see Müller-Kirsten.Harald J. W. Müller-Kirsten, Introduction to Quantum Mechanics: Schrödinger Equation and Path Integral, 2nd ed., World Scientific (Singapore, 2012), 325–329, 458–477. Corresponding results for the cosine potential (Mathieu equation) are also given in detail in this reference.

Particle in a one-dimensional lattice of finite length

Recently, it is found that the problem on particle in an ideal one-dimensional lattice of finite
length $L = N a$ with two ends $\tau$ and $L + \tau$ — where $a$ is the potential period and $N$ is a positive integer —
is another one of the few problems in quantum mechanics which can be solved analytically,



based on the mathematical theory of periodic differential equations.
In the following we briefly describe some major conclusions of the new theory. Readers who are interested in the mathematical reasonings and further details are referred to the original publications.

We are mainly interested in cases where there is always a band gap between two consecutive energy bands.
For such cases, the new theory found that ''there are two different types of states in a one-dimensional lattice of finite length'':
Corresponding to
each energy band of the Bloch wave, there are $N - 1$ states in the finite crystal whose energies are
dependent on $N$ but not on $\tau$ and map the energy band exactly, which are the stationary Bloch states.
There is always one and only one state in the finite crystal corresponding to each band gap of the Bloch wave, whose energy is dependent on $\tau$
but not on $N$. This state is either a constant-energy confined band-edge state or a surface state in the band gap.
''The very existence of such a $\tau$-dependent state is a fundamental distinction of the quantum confinement of Bloch waves''.

See also

* Free electron model
* Empty lattice approximation
* Nearly free electron model
* Crystal structure
* Mathieu function

References

External links

*
The Kronig–Penney Model
by Michael Croucher, an interactive calculation of 1d periodic potential band structure using Mathematica, from The Wolfram Demonstrations Project.

{{DEFAULTSORT:Particle In A One-Dimensional Lattice
Condensed matter physics
Electronics concepts
Quantum models