In-phase and quadrature components

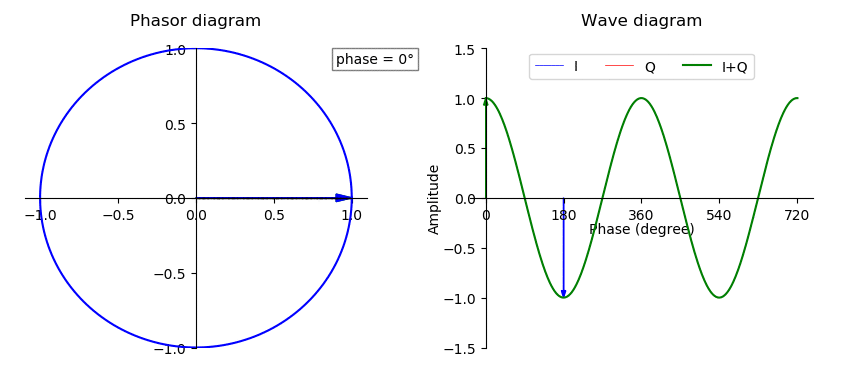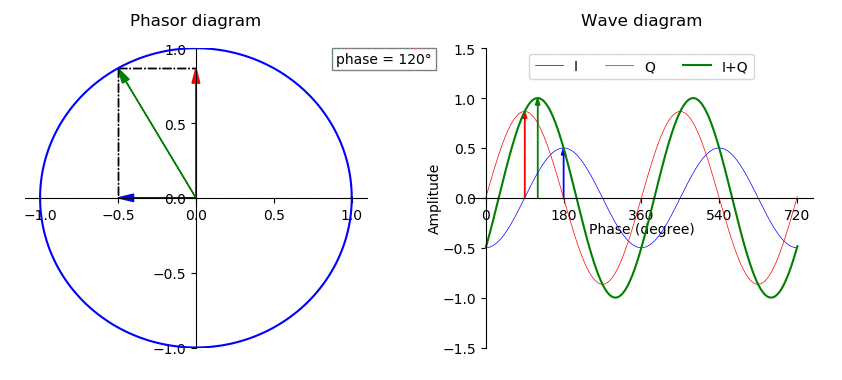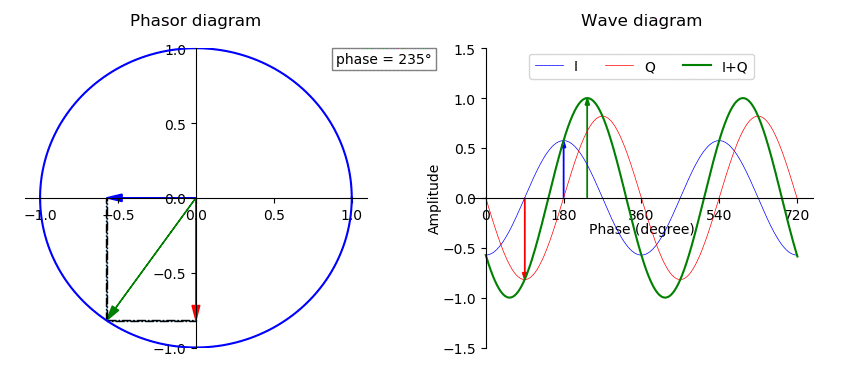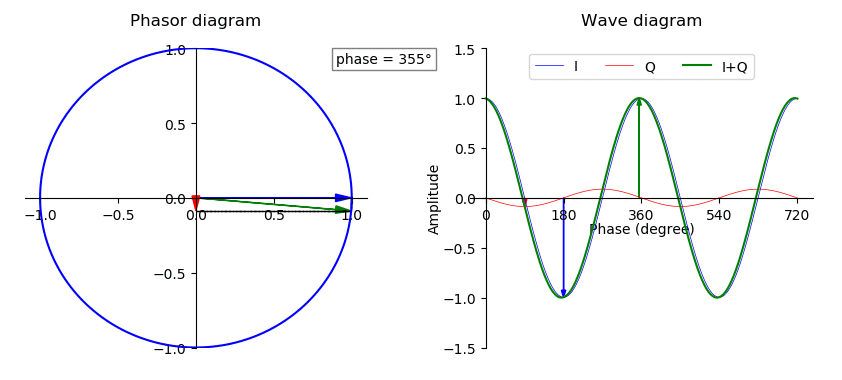
In electrical engineering, a sinusoid with angle modulation can be decomposed into, or synthesized from, two amplitude-modulated sinusoids that are offset in phase by one-quarter cycle (90 degrees or /2 radians). All three functions have the same center frequency. Such amplitude modulated sinusoids are known as the in-phase and quadrature components.
In some contexts it is more convenient to refer to only the amplitude modulation (''baseband'') itself by those terms.

Concept

In vector analysis, a vector with polar coordinates and Cartesian coordinates can be represented as the sum of orthogonal components: Similarly in trigonometry, the angle sum identity expresses:
:

And in functional analysis, when is a linear function of some variable, such as time, these components are sinusoids, and they are orthogonal functions. A phase-shift of changes the identity to:
: ,

in which case is the in-phase component. In both conventions is the in-phase amplitude modulation, which explains why some authors refer to it as the actual in-phase component.

Alternating current (AC) circuits

The term ''alternating current'' applies to a voltage vs. time function that is sinusoidal with a frequency When it is applied to a typical (linear time-invariant) circuit or device, it causes a current that is also sinusoidal. In general there is a constant phase difference, φ, between any two sinusoids. The input sinusoidal voltage is usually defined to have zero phase, meaning that it is arbitrarily chosen as a convenient time reference. So the phase difference is attributed to the current function, e.g.
whose orthogonal components are
and as we have seen. When φ happens to be such that the in-phase component is zero, the current and voltage sinusoids are said to be ''in quadrature'', which means they are orthogonal to each other. In that case, no average (active) electrical power is consumed. Rather power is temporarily stored by the device and given back, once every
seconds. Note that the term ''in quadrature'' only implies that two sinusoids are orthogonal, not that they are ''components'' of another sinusoid.

Narrowband signal model

In an angle modulation application, with carrier frequency φ is also a time-variant function, giving:

:
A(t)\cdot\sin \pi ft + \phi(t) =\ \underbrace_\, +\, \underbrace_.

When all three terms above are multiplied by an optional amplitude function, the left-hand side of the equality is known as the ''amplitude/phase'' form, and the right-hand side is the ''quadrature-carrier'' or ''IQ'' form. Because of the modulation, the components are no longer completely orthogonal functions. But when and are slowly varying functions compared to the assumption of orthogonality is a common one.
Authors often call it a ''narrowband assumption'', or a narrowband signal model.

IQ phase convention

The terms ''I-component'' and ''Q-component'' are common ways of referring to the in-phase and quadrature signals. Both signals comprise a high-frequency sinusoid (or ''carrier'') that is amplitude-modulated by a relatively low-frequency function, usually conveying some sort of information. The two carriers are orthogonal, with ''I'' lagging ''Q'' by cycle, or equivalently leading ''Q'' by cycle. The physical distinction can also be characterized in terms of $\phi(t)$:
*$\phi(t) = 0$: The composite signal reduces to just the ''I''-component, which accounts for the term ''in-phase''.
*$\phi(t) = \pi/2$: The composite signal reduces to just the ''Q''-component.
*$\phi(t) = 2\pi f_m t, \quad f_m > 0$: The amplitude modulations are orthogonal sinusoids, ''I'' leading ''Q'' by cycle.
*$\phi(t) = 2\pi f_m t, \quad f_m < 0$: The amplitude modulations are orthogonal sinusoids, ''Q'' leading ''I'' by cycle.

See also

* IQ imbalance
* Constellation diagram
* Phasor
* Polar modulation
* Quadrature amplitude modulation
* Single-sideband modulation

Notes

References

Further reading

*
*Steinmetz, Charles Proteus (1917). ''Theory and Calculations of Electrical Apparatus'' 6 (1 ed.). New York: McGraw-Hill Book Company
B004G3ZGTM

External links

I/Q Data for Dummies

{{DSP

Signal processing
Radio electronics