Hilbert's eighth problem on:
[Wikipedia]
[Google]
[Amazon]
Hilbert's eighth problem is one of 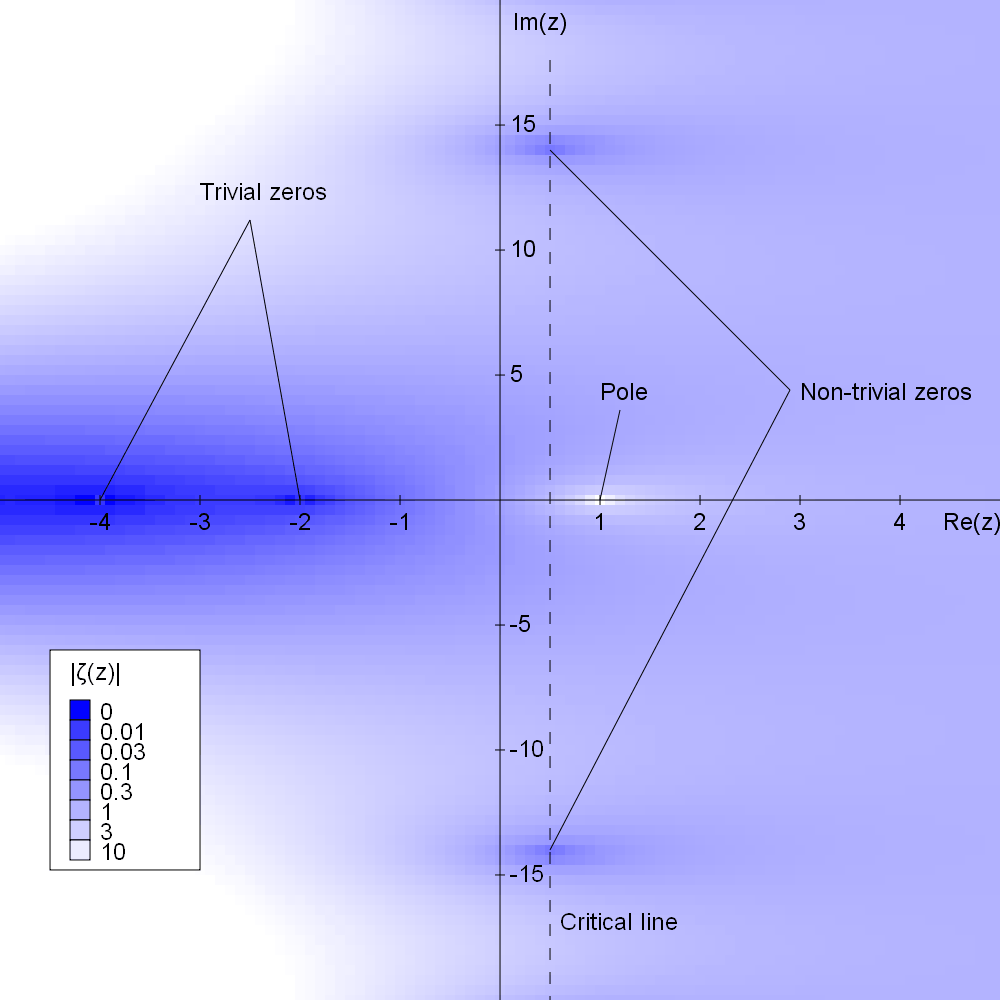
English translation of Hilbert's original address
#08
David Hilbert
David Hilbert (; ; 23 January 1862 – 14 February 1943) was a German mathematician, one of the most influential mathematicians of the 19th and early 20th centuries. Hilbert discovered and developed a broad range of fundamental ideas in many a ...
's list of open mathematical problems posed in 1900. It concerns number theory
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the integers and arithmetic function, integer-valued functions. German mathematician Carl Friedrich Gauss (1777� ...
, and in particular the Riemann hypothesis
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in ...
, although it is also concerned with the Goldbach Conjecture
Goldbach's conjecture is one of the oldest and best-known unsolved problems in number theory and all of mathematics. It states that every even natural number greater than 2 is the sum of two prime numbers.
The conjecture has been shown to hold ...
. The problem as stated asked for more work on the distribution of primes
In mathematics, the prime number theorem (PNT) describes the asymptotic distribution of the prime numbers among the positive integers. It formalizes the intuitive idea that primes become less common as they become larger by precisely quantifying t ...
and generalizations of Riemann hypothesis to other ring
Ring may refer to:
* Ring (jewellery), a round band, usually made of metal, worn as ornamental jewelry
* To make a sound with a bell, and the sound made by a bell
:(hence) to initiate a telephone connection
Arts, entertainment and media Film and ...
s where prime ideal
In algebra, a prime ideal is a subset of a ring that shares many important properties of a prime number in the ring of integers. The prime ideals for the integers are the sets that contain all the multiples of a given prime number, together with ...
s take the place of primes.

Subtopics
Riemann hypothesis and generalizations
Hilbert calls for a solution to the Riemann hypothesis, which has long been regarded as the deepest open problem in mathematics. Given the solution, he calls for more thorough investigation into Riemann'szeta function
In mathematics, a zeta function is (usually) a function analogous to the original example, the Riemann zeta function
: \zeta(s) = \sum_^\infty \frac 1 .
Zeta functions include:
* Airy zeta function, related to the zeros of the Airy function
* A ...
and the prime number theorem
In mathematics, the prime number theorem (PNT) describes the asymptotic distribution of the prime numbers among the positive integers. It formalizes the intuitive idea that primes become less common as they become larger by precisely quantifying ...
.
Goldbach conjecture
He calls for a solution to the Goldbach conjecture, as well as more general problems, such as finding infinitely many pairs of primes solving a fixed lineardiophantine equation
In mathematics, a Diophantine equation is an equation, typically a polynomial equation in two or more unknowns with integer coefficients, such that the only solutions of interest are the integer ones. A linear Diophantine equation equates to a c ...
.
Twin prime conjecture
Generalized Riemann conjecture
Finally, he calls for mathematicians to generalize the ideas of the Riemann hypothesis to counting prime ideals in a number field.External links
English translation of Hilbert's original address
#08
References
* * {{citation, last=Moxley, first= Frederick, title= Complete solutions of inverse quantum orthogonal equivalence classes, journal=Examples and Counterexamples , volume=1, pages= 100003 , year=2021, doi= 10.1016/j.exco.2021.100003, url=https://www.sciencedirect.com/science/article/pii/S2666657X2100001X