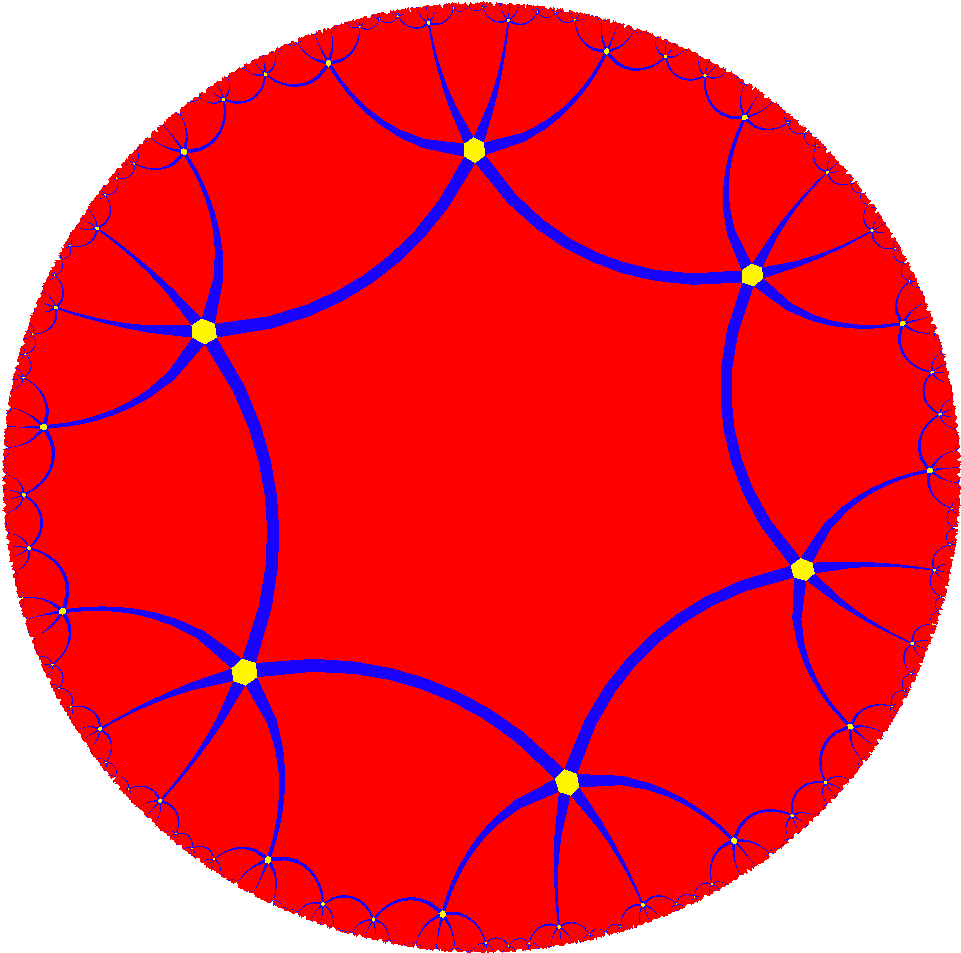
geometry Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...

, the excavated dodecahedron is a

star polyhedron In geometry, a star polyhedron is a polyhedron which has some repetitive quality of nonconvexity giving it a star-like visual quality. There are two general kinds of star polyhedron: *Polyhedra which self-intersect in a repetitive way. *Concave p ...

that looks like a

dodecahedron In geometry, a dodecahedron (Greek , from ''dōdeka'' "twelve" + ''hédra'' "base", "seat" or "face") or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagon ...

with concave

pentagonal pyramid In geometry, a pentagonal pyramid is a pyramid with a pentagonal base upon which are erected five triangular faces that meet at a point (the apex). Like any pyramid, it is self- dual. The ''regular'' pentagonal pyramid has a base that is a regu ...

s in place of its faces. Its exterior surface represents the Ef₁g₁ stellation of the icosahedron. It appears in

Magnus Wenninger Father Magnus J. Wenninger Order of Saint Benedict, OSB (October 31, 1919Banchoff (2002)– February 17, 2017) was an American mathematician who worked on constructing polyhedron models, and wrote the first book on their construction. Early life ...

's book ''Polyhedron Models'' as model 28, the ''third stellation of icosahedron''.

Description

All 20 vertices and 30 of its 60 edges belong to its dodecahedral hull. The 30 other internal edges are longer and belong to a great stellated dodecahedron. (Each contains one of the 30 edges of the

icosahedral In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons". There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrica ...

core.) There are 20 faces corresponding to the 20 vertices. Each face is a self-intersecting

hexagon In geometry, a hexagon (from Ancient Greek, Greek , , meaning "six", and , , meaning "corner, angle") is a six-sided polygon. The total of the internal angles of any simple polygon, simple (non-self-intersecting) hexagon is 720°. Regular hexa ...

with alternating long and short edges and 60° angles. The equilateral triangles touching a short edge are part of the face. (The smaller one between the long edges is a face of the icosahedral core.)

Faceting of the dodecahedron

It has the same external form as a certain facetting of the dodecahedron having 20 self-intersecting

s as faces. The non-convex hexagon face can be broken up into four equilateral triangles, three of which are the same size. A true excavated dodecahedron has the three congruent equilateral triangles as true faces of the polyhedron, while the interior equilateral triangle is not present. The 20 vertices of the

convex hull In geometry, the convex hull or convex envelope or convex closure of a shape is the smallest convex set that contains it. The convex hull may be defined either as the intersection of all convex sets containing a given subset of a Euclidean space ...

match the vertex arrangement of the dodecahedron. File:AD2.png, One of the star hexagon faces highlighted. File:Excavated dodecahedron face.png, Its face as a facet of the dodecahedron. The faceting is a

noble polyhedron A noble polyhedron is one which is isohedral (all faces the same) and isogonal (all vertices the same). They were first studied in any depth by Hess and Bruckner in the late 19th century, and later by Grünbaum. Classes of noble polyhedra Those ...

. With six six-sided faces around each vertex, it is topologically equivalent to a quotient space of the hyperbolic

order-6 hexagonal tiling In geometry, the order-6 hexagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of and is self-dual. Symmetry This tiling represents a hyperbolic kaleidoscope of 6 mirrors defining a regular hexagon fundamental d ...

, and is an abstract type ₆. It is one of ten abstract regular polyhedra of index two with vertices on one orbit.Regular Polyhedra of Index Two, II
Beitrage zur Algebra und Geometrie 52(2):357-387 · November 2010, Table 3, p.27 Uniform tiling 66-t2

Related polyhedra

References

H.S.M. Coxeter Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century. Biography Coxeter was born in Kensington t ...

, '' Regular Polytopes'', (3rd edition, 1973), Dover edition, , 3.6 6.2 ''Stellating the Platonic solids'', pp.96-104 Polyhedral stellation {{polyhedron-stub