Constructions in hyperbolic geometry on:
[Wikipedia]
[Google]
[Amazon]

 The entire hyperbolic plane can also be placed on a Poincaré disk and maintain its angles. However, the lines will turn into circular arcs, which warps them.
The entire hyperbolic plane can also be placed on a Poincaré disk and maintain its angles. However, the lines will turn into circular arcs, which warps them.
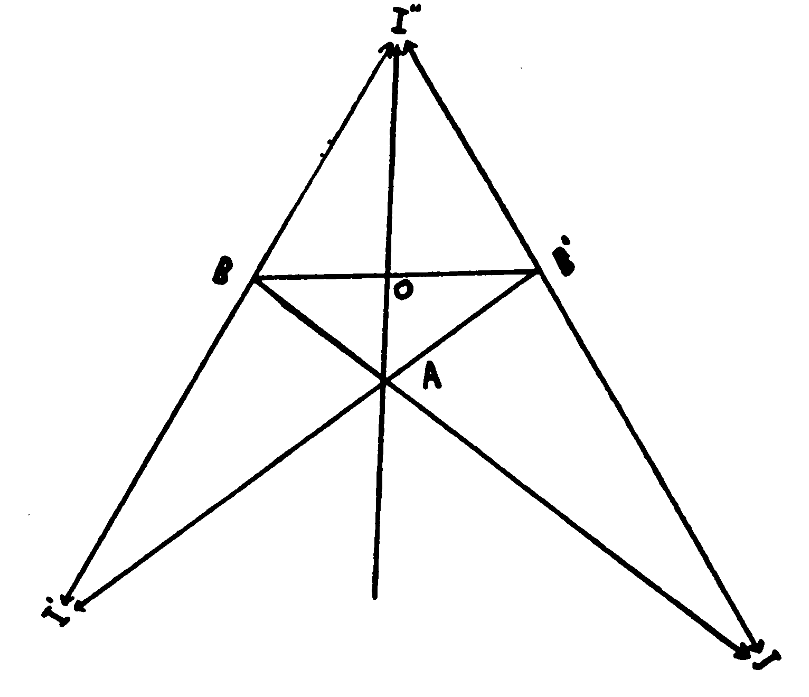 Case 2: ᗉ IAI' > /2 radians
This case is further broken down into three sub-cases:
* Case 2a: IB' intersects I'B
** Let A' be the intersection of IB' and I'B. Then AA' is the angle bisector of ᗉ IAI'.
* Case 2b: IB' is parallel to I'B
** Construct the line segment BB' and using a hyperbolic ruler, construct the line OI" such that OI" is perpendicular to BB' and parallel to B'I". Then, line OA is the angle bisector for ᗉ IAI'.
* Case 2c: IB' is
Case 2: ᗉ IAI' > /2 radians
This case is further broken down into three sub-cases:
* Case 2a: IB' intersects I'B
** Let A' be the intersection of IB' and I'B. Then AA' is the angle bisector of ᗉ IAI'.
* Case 2b: IB' is parallel to I'B
** Construct the line segment BB' and using a hyperbolic ruler, construct the line OI" such that OI" is perpendicular to BB' and parallel to B'I". Then, line OA is the angle bisector for ᗉ IAI'.
* Case 2c: IB' is
 For the purposes of the following definitions, the following assumptions will be made, which usually cannot be made in hyperbolic geometry
* Three distinct points create a unique circle
* Given any two lines, they meet at a unique point (normally, this would contradict the parallel axiom of hyperbolic geometry, since there can be many different lines parallel to the same line)
* Angle measures have signs. Here, they will be defined in the following way: Consider a triangle XYZ. The sign of angle ᗉ XYZ is positive if and only if the direction of the path along the shortest arc from side XY to side YZ is counterclockwise. The picture of the triangle on the right describes this. To make a comparison, when working with the
For the purposes of the following definitions, the following assumptions will be made, which usually cannot be made in hyperbolic geometry
* Three distinct points create a unique circle
* Given any two lines, they meet at a unique point (normally, this would contradict the parallel axiom of hyperbolic geometry, since there can be many different lines parallel to the same line)
* Angle measures have signs. Here, they will be defined in the following way: Consider a triangle XYZ. The sign of angle ᗉ XYZ is positive if and only if the direction of the path along the shortest arc from side XY to side YZ is counterclockwise. The picture of the triangle on the right describes this. To make a comparison, when working with the

Hyperbolic geometry
In mathematics, hyperbolic geometry (also called Lobachevskian geometry or János Bolyai, Bolyai–Nikolai Lobachevsky, Lobachevskian geometry) is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with:
:For a ...
is a non-Euclidean geometry
In mathematics, non-Euclidean geometry consists of two geometries based on axioms closely related to those that specify Euclidean geometry. As Euclidean geometry lies at the intersection of metric geometry and affine geometry, non-Euclidean ge ...
where the first four axioms of Euclidean geometry
Euclidean geometry is a mathematical system attributed to ancient Greek mathematics, Greek mathematician Euclid, which he described in his textbook on geometry, ''Euclid's Elements, Elements''. Euclid's approach consists in assuming a small set ...
are kept but the fifth axiom, the parallel postulate
In geometry, the parallel postulate is the fifth postulate in Euclid's ''Elements'' and a distinctive axiom in Euclidean geometry. It states that, in two-dimensional geometry:
If a line segment intersects two straight lines forming two interior ...
, is changed. The fifth axiom of hyperbolic geometry says that given a line ''L'' and a point ''P'' not on that line, there are at least two lines passing through ''P'' that are parallel to ''L''. As in Euclidean geometry, where ancient Greek mathematicians
Ancient Greek mathematics refers to the history of mathematical ideas and texts in Ancient Greece during classical and late antiquity, mostly from the 5th century BC to the 6th century AD. Greek mathematicians lived in cities spread around the s ...
used a compass and idealized ruler for constructions of lengths, angles, and other geometric figures, constructions can also be made in hyperbolic geometry.

Models of hyperbolic geometry
There are a couple of models for hyperbolic geometry, which can make performing and visualizing constructions easier. Portions of the hyperbolic plane can be placed onto apseudosphere
In geometry, a pseudosphere is a surface with constant negative Gaussian curvature.
A pseudosphere of radius is a surface in \mathbb^3 having Gaussian curvature, curvature −1/''R''2 at each point. Its name comes from the analogy with the sphere ...
and maintain angles and hyperbolic distances, as well as be bent around the pseudosphere and still keep its properties. However, not the entire hyperbolic plane can be placed onto the pseudosphere as a model, only a portion of the hyperbolic plane.
Tools
Inhyperbolic geometry
In mathematics, hyperbolic geometry (also called Lobachevskian geometry or János Bolyai, Bolyai–Nikolai Lobachevsky, Lobachevskian geometry) is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with:
:For a ...
, one can use the standard ruler and compass that is often used in Euclidean plane geometry
Euclidean geometry is a mathematical system attributed to ancient Greek mathematician Euclid, which he described in his textbook on geometry, '' Elements''. Euclid's approach consists in assuming a small set of intuitively appealing axioms (pos ...
. However, there are a variety of compasses and rulers developed for hyperbolic constructions.
A hypercompass can be used to construct a hypercycle given the central line and radius. A horocompass can be used to construct a horocycle
In hyperbolic geometry, a horocycle ( from Greek roots meaning "boundary circle"), sometimes called an oricycle or limit circle, is a curve of constant curvature where all the perpendicular geodesics ( normals) through a point on a horocycle are ...
through a specific point if the diameter and direction are also provided. Both of these also require a straight edge, like the standard ruler
A ruler, sometimes called a rule, scale, line gauge, or metre/meter stick, is an instrument used to make length measurements, whereby a length is read from a series of markings called "rules" along an edge of the device. Usually, the instr ...
. When doing constructions in hyperbolic geometry, as long as you are using the proper ruler for the construction, the three compasses (meaning the horocompass, hypercompass, and the standard compass
A compass is a device that shows the cardinal directions used for navigation and geographic orientation. It commonly consists of a magnetized needle or other element, such as a compass card or compass rose, which can pivot to align itself with No ...
) can all perform the same constructions.
A parallel ruler can be used to draw a line through a given point A and parallel to a given ray ''a''''.'' For any two lines, a hyperbolic ruler can be used to construct a line that is parallel to the first line and perpendicular to the second.
A few notes on the uses of rulers are:
* A parallel ruler can be used to construct anything that a standard ruler and the three compasses can also construct
* A parallel ruler can act as a ruler in Euclidean geometry
* A hyperbolic ruler cannot perform Euclidean geometry constructions
* In hyperbolic geometry, constructions that can be done using any one of the three compasses listed above and the parallel ruler can also be done using the hyperbolic ruler
Simple constructions
Angle bisector
Consider a given angle ᗉ IAI' ≠ /2 radians whoseangle bisector
In geometry, bisection is the division of something into two equal or congruent parts (having the same shape and size). Usually it involves a bisecting line, also called a ''bisector''. The most often considered types of bisectors are the ''se ...
is sought. This results in two different cases: either ᗉ IAI' < /2 radians or ᗉ IAI' > /2 radians. For both cases a hyperbolic ruler is needed to construct a line BI' where BI' is perpendicular
In geometry, two geometric objects are perpendicular if they intersect at right angles, i.e. at an angle of 90 degrees or π/2 radians. The condition of perpendicularity may be represented graphically using the '' perpendicular symbol'', � ...
to AI and parallel to AI'. Also, construct a line B'I where B'I is perpendicular to AI' and parallel to AI.
Case 1: ᗉ IAI'< /2 radians
Let C be the intersection of BI' and B'I. The result of this is that the line AC bisects ᗉ IAI'.
 Case 2: ᗉ IAI' > /2 radians
This case is further broken down into three sub-cases:
* Case 2a: IB' intersects I'B
** Let A' be the intersection of IB' and I'B. Then AA' is the angle bisector of ᗉ IAI'.
* Case 2b: IB' is parallel to I'B
** Construct the line segment BB' and using a hyperbolic ruler, construct the line OI" such that OI" is perpendicular to BB' and parallel to B'I". Then, line OA is the angle bisector for ᗉ IAI'.
* Case 2c: IB' is
Case 2: ᗉ IAI' > /2 radians
This case is further broken down into three sub-cases:
* Case 2a: IB' intersects I'B
** Let A' be the intersection of IB' and I'B. Then AA' is the angle bisector of ᗉ IAI'.
* Case 2b: IB' is parallel to I'B
** Construct the line segment BB' and using a hyperbolic ruler, construct the line OI" such that OI" is perpendicular to BB' and parallel to B'I". Then, line OA is the angle bisector for ᗉ IAI'.
* Case 2c: IB' is ultraparallel
In mathematics, hyperbolic geometry (also called Lobachevskian geometry or Bolyai– Lobachevskian geometry) is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with:
:For any given line ''R'' and point ''P' ...
to I'B.
** Using the ultraparallel theorem
In hyperbolic geometry, two lines are said to be ultraparallel if they do not intersect and are not limiting parallel.
The ultraparallel theorem states that every pair of (distinct) ultraparallel lines has a unique common perpendicular (a hyper ...
, construct the common perpendicular of IB' and I'B, CC'. Let the intersection of CB" and BC' be D. As a result, AD will be the angle bisector of ᗉ BDB'. We then find that the line through OD is also the angle bisector of ᗉ IAI'.
Common parallel line to two lines
We consider the problem of finding a line parallel to two given lines, ''a'' and ''a'ultraparallel theorem
In hyperbolic geometry, two lines are said to be ultraparallel if they do not intersect and are not limiting parallel.
The ultraparallel theorem states that every pair of (distinct) ultraparallel lines has a unique common perpendicular (a hyper ...
. Let the intersection of BI' and B'I be C. Then ᗉ ICI' ≠ /2 radians, which allows you to finish the construction like the other two cases.
Line perpendicular to another line at a point
Suppose you have a line ''a'' and a point A on that line, and you want to construct a line perpendicular to ''a'' and through A. Then let ''a'ultraparallel theorem
In hyperbolic geometry, two lines are said to be ultraparallel if they do not intersect and are not limiting parallel.
The ultraparallel theorem states that every pair of (distinct) ultraparallel lines has a unique common perpendicular (a hyper ...
now allows us to create the common perpendicular to II" and ''a'' because these two lines are ultraparallel. This common perpendicular is now the line perpendicular to ''a'' and through A.
Midpoint of a line segment
Suppose you are trying to find the midpoint of a line segment AB. Then construct the line AI such that AI is through A and perpendicular to AB. Also, construct a line BI' such that BI' intersects AB at B and is perpendicular to AB. Now construct the line II' such that II' is the common parallel to AI and BI'. Construct the common perpendicular to II' and AB, which can be done using theultraparallel theorem
In hyperbolic geometry, two lines are said to be ultraparallel if they do not intersect and are not limiting parallel.
The ultraparallel theorem states that every pair of (distinct) ultraparallel lines has a unique common perpendicular (a hyper ...
because II' and AB are ultraparallel to each other. Name this line CC'. C now ends up being the midpoint of AB.
Definitions for complex constructions
 For the purposes of the following definitions, the following assumptions will be made, which usually cannot be made in hyperbolic geometry
* Three distinct points create a unique circle
* Given any two lines, they meet at a unique point (normally, this would contradict the parallel axiom of hyperbolic geometry, since there can be many different lines parallel to the same line)
* Angle measures have signs. Here, they will be defined in the following way: Consider a triangle XYZ. The sign of angle ᗉ XYZ is positive if and only if the direction of the path along the shortest arc from side XY to side YZ is counterclockwise. The picture of the triangle on the right describes this. To make a comparison, when working with the
For the purposes of the following definitions, the following assumptions will be made, which usually cannot be made in hyperbolic geometry
* Three distinct points create a unique circle
* Given any two lines, they meet at a unique point (normally, this would contradict the parallel axiom of hyperbolic geometry, since there can be many different lines parallel to the same line)
* Angle measures have signs. Here, they will be defined in the following way: Consider a triangle XYZ. The sign of angle ᗉ XYZ is positive if and only if the direction of the path along the shortest arc from side XY to side YZ is counterclockwise. The picture of the triangle on the right describes this. To make a comparison, when working with the unit circle
In mathematics, a unit circle is a circle of unit radius—that is, a radius of 1. Frequently, especially in trigonometry, the unit circle is the circle of radius 1 centered at the origin (0, 0) in the Cartesian coordinate system in the Eucli ...
, the angle measure is positive when going counterclockwise and negative when going clockwise.
Cyclic quadrilaterals
A quadrilateral is cyclic if the two opposite vertices add up to pi radians or 180 degrees. Also, if a quadrilateral is inscribed in a circle in a way that all of its vertexes lie on the circle, it is cyclic.Pseudoaltitudes
Consider triangle ABC where the points are labeled in a clockwise manner so all angles are positive. Let X be a point moving along BC from B to C. As X moves closer to C, angle ᗉAXB will decrease and angle ᗉ AXC will increase. When X is close enough to B, ᗉ AXB > ᗉ AXC. When X is close enough to C, ᗉ AXB < ᗉ AXC. This means that at some point, X will be in a position where ᗉ AXB = ᗉ AXC. When X is in this position, it is defined as the foot of the pseudoaltitude from vertex A. The pseudoaltitude would then be the line segment AX.Pseudolengths
Let dE(A,B) denote the pseudolength for a given hyperbolic line segment AB. Let a transformation move A to the center of a Poincaré disk with a radius equalling 1. The pseudolength dE(A,B) is the length of this segment in Euclidean geometry.Homothety
Given a point P, a point A where A is the center of the homothety, and a number k that represents the ratio of the homothety, a homothety is a transformation that will move P to a point P' where P' is on the ray AP and dE(A,P') = k·dE(A,P).Three dunce cap theorem
Consider the three circles ''ω''1, ''ω''2, and ''ω''3 in a common plane. Let P1 be the intersection of the two external tangent lines of ''ω''2 and ''ω''3. Let P2 and P3 be found the same way. The Three Dunce Caps Theorem then says that P1, P2, and P3 all lie on the same line. ''Proof:'' Construct a sphere on top of each circle and then construct a plane tangent to these three spheres. The plane intersects the plane that the circles lies on at a straight line containing P1, P2, and P3. These points are also the centers of homothety for the circles that they were derived from.Applying to spherical geometry
Algebraically, hyperbolic andspherical geometry
300px, A sphere with a spherical triangle on it.
Spherical geometry or spherics () is the geometry of the two-dimensional surface of a sphere or the -dimensional surface of higher dimensional spheres.
Long studied for its practical applicati ...
have the same structure. This allows us to apply concepts and theorems to one geometry to the other. Applying hyperbolic geometry to spherical geometry can make it easier to understand because spheres are much more concrete, which then makes spherical geometry easier to conceptualize.
References
{{reflist Hyperbolic geometry