Coulomb Repulsion on:
[Wikipedia]
[Google]
[Amazon]
Coulomb's inverse-square law, or simply Coulomb's law, is an experimental
 Ancient cultures around the
Ancient cultures around the
 Coulomb's law in vector form states that the electrostatic force experienced by a charge, at position , in the vicinity of another charge, at position , in a vacuum is equal to
where is the vectorial distance between the charges, a unit vector pointing from to and the
Coulomb's law in vector form states that the electrostatic force experienced by a charge, at position , in the vicinity of another charge, at position , in a vacuum is equal to
where is the vectorial distance between the charges, a unit vector pointing from to and the
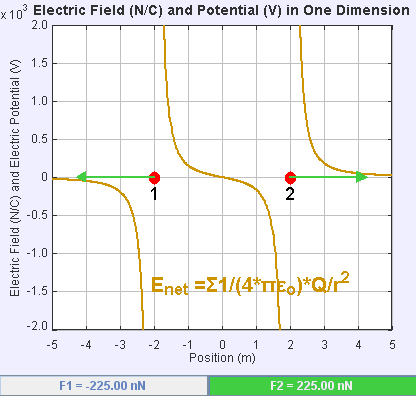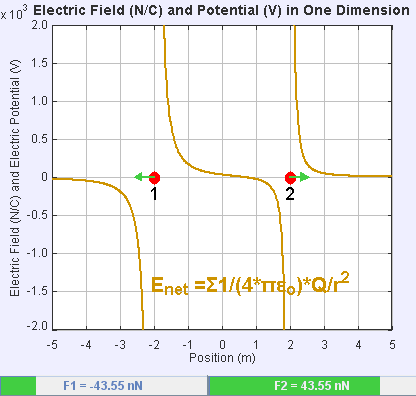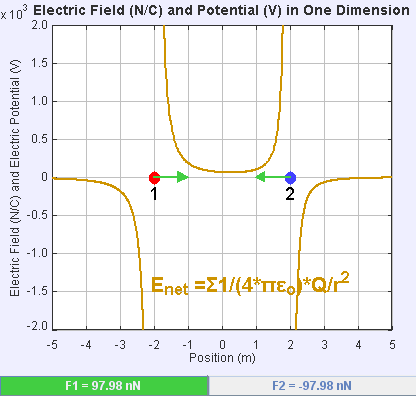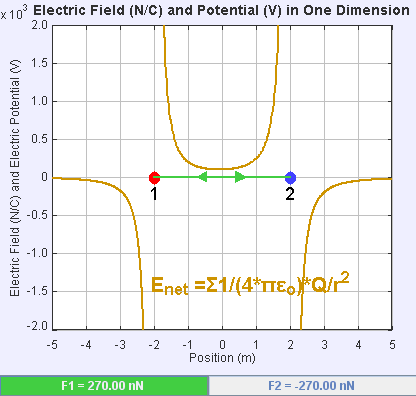 An electric field is a vector field that associates to each point in space the Coulomb force experienced by a unit test charge. The strength and direction of the Coulomb force on a charge depends on the electric field established by other charges that it finds itself in, such that . In the simplest case, the field is considered to be generated solely by a single source
An electric field is a vector field that associates to each point in space the Coulomb force experienced by a unit test charge. The strength and direction of the Coulomb force on a charge depends on the electric field established by other charges that it finds itself in, such that . In the simplest case, the field is considered to be generated solely by a single source
 The Coulomb potential admits continuum states (with ''E'' > 0), describing electron-proton scattering, as well as discrete bound states, representing the hydrogen atom. It can also be derived within the non-relativistic limit between two charged particles, as follows:
Under
The Coulomb potential admits continuum states (with ''E'' > 0), describing electron-proton scattering, as well as discrete bound states, representing the hydrogen atom. It can also be derived within the non-relativistic limit between two charged particles, as follows:
Under
 It is possible to verify Coulomb's law with a simple experiment. Consider two small spheres of mass and same-sign charge , hanging from two ropes of negligible mass of length . The forces acting on each sphere are three: the weight , the rope tension and the electric force . In the equilibrium state:
and
Dividing () by ():
Let be the distance between the charged spheres; the repulsion force between them , assuming Coulomb's law is correct, is equal to
so:
If we now discharge one of the spheres, and we put it in contact with the charged sphere, each one of them acquires a charge . In the equilibrium state, the distance between the charges will be and the repulsion force between them will be:
We know that and:
Dividing () by (), we get:
Measuring the angles and and the distance between the charges and is sufficient to verify that the equality is true taking into account the experimental error. In practice, angles can be difficult to measure, so if the length of the ropes is sufficiently great, the angles will be small enough to make the following approximation:
Using this approximation, the relationship () becomes the much simpler expression:
In this way, the verification is limited to measuring the distance between the charges and check that the division approximates the theoretical value.
It is possible to verify Coulomb's law with a simple experiment. Consider two small spheres of mass and same-sign charge , hanging from two ropes of negligible mass of length . The forces acting on each sphere are three: the weight , the rope tension and the electric force . In the equilibrium state:
and
Dividing () by ():
Let be the distance between the charged spheres; the repulsion force between them , assuming Coulomb's law is correct, is equal to
so:
If we now discharge one of the spheres, and we put it in contact with the charged sphere, each one of them acquires a charge . In the equilibrium state, the distance between the charges will be and the repulsion force between them will be:
We know that and:
Dividing () by (), we get:
Measuring the angles and and the distance between the charges and is sufficient to verify that the equality is true taking into account the experimental error. In practice, angles can be difficult to measure, so if the length of the ropes is sufficiently great, the angles will be small enough to make the following approximation:
Using this approximation, the relationship () becomes the much simpler expression:
In this way, the verification is limited to measuring the distance between the charges and check that the division approximates the theoretical value.
''Coulomb's Law''
o
Project PHYSNET
Ćöa chapter from an online textbook
Ćöa game created by the Molecular Workbench software
Walter Lewin, ''8.02 Electricity and Magnetism, Spring 2002: Lecture 1'' (video). MIT OpenCourseWare. License: Creative Commons Attribution-Noncommercial-Share Alike. {{Authority control Electromagnetism Electrostatics Force Scientific laws
law
Law is a set of rules that are created and are enforceable by social or governmental institutions to regulate behavior,Robertson, ''Crimes against humanity'', 90. with its precise definition a matter of longstanding debate. It has been vario ...
of physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which r ...
that quantifies the amount of force between two stationary, electrically charged
Electric charge is the physical property of matter that causes charged matter to experience a force when placed in an electromagnetic field. Electric charge can be ''positive'' or ''negative'' (commonly carried by protons and electrons respe ...
particles. The electric force between charged bodies at rest is conventionally called ''electrostatic force'' or Coulomb force. Although the law was known earlier, it was first published in 1785 by French physicist Charles-Augustin de Coulomb
Charles-Augustin de Coulomb (; ; 14 June 1736 ŌĆō 23 August 1806) was a French officer, engineer, and physicist. He is best known as the eponymous discoverer of what is now called Coulomb's law, the description of the electrostatic force of attra ...
, hence the name. Coulomb's law was essential to the development of the theory of electromagnetism, maybe even its starting point, as it made it possible to discuss the quantity of electric charge in a meaningful way.
The law states that the magnitude of the electrostatic force of attraction or repulsion between two point charges
Charge or charged may refer to:
Arts, entertainment, and media Films
* ''Charge, Zero Emissions/Maximum Speed'', a 2011 documentary
Music
* ''Charge'' (David Ford album)
* ''Charge'' (Machel Montano album)
* '' Charge!!'', an album by The Aqu ...
is directly proportional to the product of the magnitudes of charges and inversely proportional to the square of the distance between them. Coulomb studied the repulsive force between bodies having electrical charges of the same sign:
Coulomb also showed that oppositely charged bodies obey an inverse-square law of attraction.
Here, or is the Coulomb constant (), and are the assigned magnitudes of the charges, and the scalar ''r'' is the distance between the charges.
The force is along the straight line joining the two charges. If the charges have the same sign, the electrostatic force between them is repulsive; if they have different signs, the force between them is attractive.
Being an inverse-square law, the law is analogous to Isaac Newton
Sir Isaac Newton (25 December 1642 ŌĆō 20 March 1726/27) was an English mathematician, physicist, astronomer, alchemist, theologian, and author (described in his time as a " natural philosopher"), widely recognised as one of the grea ...
's inverse-square law of universal gravitation, but gravitational forces are always attractive, while electrostatic forces can be attractive or repulsive. Coulomb's law can be used to derive Gauss's law
In physics and electromagnetism, Gauss's law, also known as Gauss's flux theorem, (or sometimes simply called Gauss's theorem) is a law relating the distribution of electric charge to the resulting electric field. In its integral form, it sta ...
, and vice versa. In the case of a single stationary point charge, the two laws are equivalent, expressing the same physical law in different ways. The law has been tested extensively, and observations have upheld the law on the scale from 10ŌłÆ16 m to 108 m.
History
 Ancient cultures around the
Ancient cultures around the Mediterranean
The Mediterranean Sea is a sea connected to the Atlantic Ocean, surrounded by the Mediterranean Basin and almost completely enclosed by land: on the north by Western Europe, Western and Southern Europe and Anatolia, on the south by North Africa ...
knew that certain objects, such as rods of amber
Amber is fossilized tree resin that has been appreciated for its color and natural beauty since Neolithic times. Much valued from antiquity to the present as a gemstone, amber is made into a variety of decorative objects."Amber" (2004). In ...
, could be rubbed with cat's fur to attract light objects like feathers and pieces of paper. Thales of Miletus
Thales of Miletus ( ; grc-gre, ╬ś╬▒╬╗ß┐åŽé; ) was a Greek mathematician, astronomer, statesman, and pre-Socratic philosopher from Miletus in Ionia, Asia Minor. He was one of the Seven Sages of Greece. Many, most notably Aristotle, regarded ...
made the first recorded description of static electricity around 600 BC, when he noticed that friction
Friction is the force resisting the relative motion of solid surfaces, fluid layers, and material elements sliding against each other. There are several types of friction:
*Dry friction is a force that opposes the relative lateral motion of ...
could render a piece of amber magnetic.
In 1600, English scientist William Gilbert made a careful study of electricity and magnetism, distinguishing the lodestone
Lodestones are naturally magnetized pieces of the mineral magnetite. They are naturally occurring magnets, which can attract iron. The property of magnetism was first discovered in antiquity through lodestones. Pieces of lodestone, suspen ...
effect from static electricity produced by rubbing amber. He coined the New Latin
New Latin (also called Neo-Latin or Modern Latin) is the revival of Literary Latin used in original, scholarly, and scientific works since about 1500. Modern scholarly and technical nomenclature, such as in zoological and botanical taxonomy ...
word ''electricus'' ("of amber" or "like amber", from 'elektron'' the Greek word for "amber") to refer to the property of attracting small objects after being rubbed. This association gave rise to the English words "electric" and "electricity", which made their first appearance in print in Thomas Browne's ''Pseudodoxia Epidemica
''Pseudodoxia Epidemica or Enquiries into very many received tenents and commonly presumed truths'', also known simply as ''Pseudodoxia Epidemica'' or ''Vulgar Errors'', is a work by Thomas Browne challenging and refuting the "vulgar" or common ...
'' of 1646.
Early investigators of the 18th century who suspected that the electrical force diminished with distance as the force of gravity
In physics, gravity () is a fundamental interaction which causes mutual attraction between all things with mass or energy. Gravity is, by far, the weakest of the four fundamental interactions, approximately 1038 times weaker than the stro ...
did (i.e., as the inverse square of the distance) included Daniel Bernoulli and Alessandro Volta, both of whom measured the force between plates of a capacitor
A capacitor is a device that stores electrical energy in an electric field by virtue of accumulating electric charges on two close surfaces insulated from each other. It is a passive electronic component with two terminals.
The effect of ...
, and Franz Aepinus
Franz Ulrich Theodor Aepinus (13 December 172410 August 1802) was a German mathematician, scientist, and natural philosopher residing in the Russian Empire. Aepinus is best known for his researches, theoretical and experimental, in electricity a ...
who supposed the inverse-square law in 1758.
Based on experiments with electrically charged
Electric charge is the physical property of matter that causes charged matter to experience a force when placed in an electromagnetic field. Electric charge can be ''positive'' or ''negative'' (commonly carried by protons and electrons respe ...
spheres, Joseph Priestley
Joseph Priestley (; 24 March 1733 ŌĆō 6 February 1804) was an English chemist, natural philosopher, separatist theologian, grammarian, multi-subject educator, and liberal political theorist. He published over 150 works, and conducted exp ...
of England was among the first to propose that electrical force followed an inverse-square law, similar to Newton's law of universal gravitation
Newton's law of universal gravitation is usually stated as that every particle attracts every other particle in the universe with a force that is proportional to the product of their masses and inversely proportional to the square of the distan ...
. However, he did not generalize or elaborate on this. In 1767, he conjectured that the force between charges varied as the inverse square of the distance.
In 1769, Scottish physicist John Robison announced that, according to his measurements, the force of repulsion between two spheres with charges of the same sign varied as .
In the early 1770s, the dependence of the force between charged bodies upon both distance and charge had already been discovered, but not published, by Henry Cavendish
Henry Cavendish ( ; 10 October 1731 ŌĆō 24 February 1810) was an English natural philosopher and scientist who was an important experimental and theoretical chemist and physicist. He is noted for his discovery of hydrogen, which he termed "infl ...
of England. In his notes, Cavendish wrote, "We may therefore conclude that the electric attraction and repulsion must be inversely as some power of the distance between that of the 2 + th and that of the 2 ŌłÆ th, and there is no reason to think that it differs at all from the inverse duplicate ratio".
Finally, in 1785, the French physicist Charles-Augustin de Coulomb
Charles-Augustin de Coulomb (; ; 14 June 1736 ŌĆō 23 August 1806) was a French officer, engineer, and physicist. He is best known as the eponymous discoverer of what is now called Coulomb's law, the description of the electrostatic force of attra ...
published his first three reports of electricity and magnetism where he stated his law. This publication was essential to the development of the theory of electromagnetism. He used a torsion balance
A torsion spring is a spring that works by twisting its end along its axis; that is, a flexible elastic object that stores mechanical energy when it is twisted. When it is twisted, it exerts a torque in the opposite direction, proportional ...
to study the repulsion and attraction forces of charged particle
In physics, a charged particle is a particle with an electric charge. It may be an ion, such as a molecule or atom with a surplus or deficit of electrons relative to protons. It can also be an electron or a proton, or another elementary pa ...
s, and determined that the magnitude of the electric force between two point charge
A point particle (ideal particle or point-like particle, often spelled pointlike particle) is an idealization of particles heavily used in physics. Its defining feature is that it lacks spatial extension; being dimensionless, it does not take u ...
s is directly proportional to the product of the charges and inversely proportional to the square of the distance between them.
The torsion balance consists of a bar suspended from its middle by a thin fiber. The fiber acts as a very weak torsion spring. In Coulomb's experiment, the torsion balance was an insulating rod with a metal
A metal (from Greek ╬╝╬ŁŽä╬▒╬╗╬╗╬┐╬Į ''m├®tallon'', "mine, quarry, metal") is a material that, when freshly prepared, polished, or fractured, shows a lustrous appearance, and conducts electricity and heat relatively well. Metals are typicall ...
-coated ball attached to one end, suspended by a silk
Silk is a natural protein fiber, some forms of which can be woven into textiles. The protein fiber of silk is composed mainly of fibroin and is produced by certain insect larvae to form cocoons. The best-known silk is obtained from the ...
thread. The ball was charged with a known charge of static electricity, and a second charged ball of the same polarity was brought near it. The two charged balls repelled one another, twisting the fiber through a certain angle, which could be read from a scale on the instrument. By knowing how much force it took to twist the fiber through a given angle, Coulomb was able to calculate the force between the balls and derive his inverse-square proportionality law.
Scalar form of the law
Coulomb's law can be stated as a simple mathematical expression. Thescalar
Scalar may refer to:
*Scalar (mathematics), an element of a field, which is used to define a vector space, usually the field of real numbers
* Scalar (physics), a physical quantity that can be described by a single element of a number field such ...
form gives the magnitude of the vector of the electrostatic force between two point charges and , but not its direction. If is the distance between the charges, the magnitude of the force is
The constant is called the Coulomb constant and is equal to , where is the electric constant
Vacuum permittivity, commonly denoted (pronounced "epsilon nought" or "epsilon zero"), is the value of the absolute dielectric permittivity of classical vacuum. It may also be referred to as the permittivity of free space, the electric const ...
; . If the product is positive, the force between the two charges is repulsive; if the product is negative, the force between them is attractive.
Vector form of the law
electric constant
Vacuum permittivity, commonly denoted (pronounced "epsilon nought" or "epsilon zero"), is the value of the absolute dielectric permittivity of classical vacuum. It may also be referred to as the permittivity of free space, the electric const ...
. Here, is used for the vector notation.
The vector form of Coulomb's law is simply the scalar definition of the law with the direction given by the unit vector
In mathematics, a unit vector in a normed vector space is a vector (often a spatial vector) of length 1. A unit vector is often denoted by a lowercase letter with a circumflex, or "hat", as in \hat (pronounced "v-hat").
The term ''direction v ...
, parallel with the line ''from'' charge ''to'' charge . If both charges have the same sign (like charges) then the product
Product may refer to:
Business
* Product (business), an item that serves as a solution to a specific consumer problem.
* Product (project management), a deliverable or set of deliverables that contribute to a business solution
Mathematics
* Produ ...
is positive and the direction of the force on is given by ; the charges repel each other. If the charges have opposite signs then the product is negative and the direction of the force on is the charges attract each other.
The electrostatic force experienced by , according to Newton's third law
Newton's laws of motion are three basic laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at rest, or in moti ...
, is
System of discrete charges
Thelaw of superposition
The law of superposition is an axiom that forms one of the bases of the sciences of geology, archaeology, and other fields pertaining to geological stratigraphy. In its plainest form, it states that in undeformed stratigraphic sequences, the ...
allows Coulomb's law to be extended to include any number of point charges. The force acting on a point charge due to a system of point charges is simply the vector addition
In mathematics, physics, and engineering, a Euclidean vector or simply a vector (sometimes called a geometric vector or spatial vector) is a geometric object that has magnitude (or length) and direction. Vectors can be added to other vectors a ...
of the individual forces acting alone on that point charge due to each one of the charges. The resulting force vector is parallel to the electric field vector at that point, with that point charge removed.
Force on a small charge at position , due to a system of discrete charges in vacuum is
where and are the magnitude and position respectively of the th charge, is a unit vector in the direction of , a vector pointing from charges to .
Continuous charge distribution
In this case, the principle oflinear superposition
The superposition principle, also known as superposition property, states that, for all linear systems, the net response caused by two or more stimuli is the sum of the responses that would have been caused by each stimulus individually. So tha ...
is also used. For a continuous charge distribution, an integral
In mathematics, an integral assigns numbers to functions in a way that describes displacement, area, volume, and other concepts that arise by combining infinitesimal data. The process of finding integrals is called integration. Along wit ...
over the region containing the charge is equivalent to an infinite summation, treating each infinitesimal element of space as a point charge . The distribution of charge is usually linear, surface or volumetric.
For a linear charge distribution (a good approximation for charge in a wire) where gives the charge per unit length at position , and is an infinitesimal element of length,
For a surface charge distribution (a good approximation for charge on a plate in a parallel plate capacitor
A capacitor is a device that stores electrical energy in an electric field by virtue of accumulating electric charges on two close surfaces insulated from each other. It is a passive electronic component with two terminals.
The effect of ...
) where gives the charge per unit area at position , and is an infinitesimal element of area,
For a volume charge distribution (such as charge within a bulk metal) where gives the charge per unit volume at position , and is an infinitesimal element of volume,
The force on a small test charge at position in vacuum is given by the integral over the distribution of charge
where it must be noted that the "continuous charge" version of Coulomb's law is never supposed to be applied to locations for which because that location would directly overlap with the location of a charged particle (e.g. electron or proton) which is not a valid location to analyze the electric field or potential classically. Charge is always discrete in reality, and the "continuous charge" assumption is just an approximation that is not supposed to allow to be analyzed.
Coulomb constant
The Coulomb constant is a proportionality factor that appears in Coulomb's law as well as in other electric-related formulas. Denoted , it is also called the electric force constant or electrostatic constant hence the subscript . When theelectromagnetic theory
In physics, electromagnetism is an interaction that occurs between particles with electric charge. It is the second-strongest of the four fundamental interactions, after the strong force, and it is the dominant force in the interactions of a ...
is expressed in the International System of Units, force is measured in newtons, charge in coulombs and distance in meter
The metre (British spelling) or meter (American spelling; see spelling differences) (from the French unit , from the Greek noun , "measure"), symbol m, is the primary unit of length in the International System of Units (SI), though its pref ...
s. The Coulomb constant is given by . The constant is the vacuum electric permittivity
Vacuum permittivity, commonly denoted (pronounced "epsilon nought" or "epsilon zero"), is the value of the absolute dielectric permittivity of classical vacuum. It may also be referred to as the permittivity of free space, the electric const ...
(also known as "electric constant") in . It should not be confused with , which is the dimensionless relative permittivity
The relative permittivity (in older texts, dielectric constant) is the permittivity of a material expressed as a ratio with the electric permittivity of a vacuum. A dielectric is an insulating material, and the dielectric constant of an insul ...
of the material in which the charges are immersed, or with their product , which is called "absolute permittivity
In electromagnetism, the absolute permittivity, often simply called permittivity and denoted by the Greek letter ''╬Ą'' ( epsilon), is a measure of the electric polarizability of a dielectric. A material with high permittivity polarizes more in ...
of the material" and is still used in electrical engineering.
Prior to the 2019 redefinition of the SI base units, the Coulomb constant was considered to have an exact value:
Since the 2019 redefinition, the Coulomb constant is no longer exactly defined and is subject to the measurement error in the fine structure constant. As calculated from CODATA 2018 recommended values, the Coulomb constant isDerived from ŌĆō
In Gaussian units
Gaussian units constitute a metric system of physical units. This system is the most common of the several electromagnetic unit systems based on cgs (centimetreŌĆōgramŌĆōsecond) units. It is also called the Gaussian unit system, Gaussian-cgs uni ...
and HeavisideŌĆōLorentz units
HeavisideŌĆōLorentz units (or LorentzŌĆōHeaviside units) constitute a system of units (particularly electromagnetic units) within CGS, named for Hendrik Antoon Lorentz and Oliver Heaviside. They share with CGS-Gaussian units the property that th ...
, which are both CGS unit systems, the constant has different, dimensionless
A dimensionless quantity (also known as a bare quantity, pure quantity, or scalar quantity as well as quantity of dimension one) is a quantity to which no physical dimension is assigned, with a corresponding SI unit of measurement of one (or 1) ...
values.
In electrostatic units The electrostatic system of units (CGS-ESU) is a system of units used to measure quantities of electric charge, electric current, and voltage within the centimetreŌĆōgramŌĆōsecond (or "CGS") system of metric units. In electrostatic units, electric ...
or Gaussian units the unit charge (''esu'' or statcoulomb
The franklin (Fr) or statcoulomb (statC) electrostatic unit of charge (esu) is the physical unit for electrical charge used in the cgs-esu and Gaussian units. It is a derived unit given by
: 1 statC = 1 dyn1/2Ōŗģcm = 1 cm3/2Ōŗģg1/2ŌŗģsŌłÆ1.
Tha ...
) is defined in such a way that the Coulomb constant disappears, as it has the value of one and becomes dimensionless.
In HeavisideŌĆōLorentz units, also called ''rationalized'' ''units'', the Coulomb constant is dimensionless and is equal to
Gaussian units are more amenable for microscopic problems such as the electrodynamics of individual electrically charged particles. SI units are more convenient for practical, large-scale phenomena, such as engineering applications.
Limitations
There are three conditions to be fulfilled for the validity of Coulomb's inverse square law: # The charges must have a spherically symmetric distribution (e.g. be point charges, or a charged metal sphere). # The charges must not overlap (e.g. they must be distinct point charges). # The charges must be stationary with respect to each other. The last of these is known as the electrostatic approximation. When movement takes place, Einstein'stheory of relativity
The theory of relativity usually encompasses two interrelated theories by Albert Einstein: special relativity and general relativity, proposed and published in 1905 and 1915, respectively. Special relativity applies to all physical phenomena in ...
must be taken into consideration, and a result, an extra factor is introduced, which alters the force produced on the two objects. This extra part of the force is called the magnetic force, and is described by magnetic fields. For slow movement, the magnetic force is minimal and Coulomb's law can still be considered approximately correct, but when the charges are moving more quickly in relation to each other, the full electrodynamics
In physics, electromagnetism is an interaction that occurs between particles with electric charge. It is the second-strongest of the four fundamental interactions, after the strong force, and it is the dominant force in the interactions o ...
rules (incorporating the magnetic force) must be considered.
Electric field
 An electric field is a vector field that associates to each point in space the Coulomb force experienced by a unit test charge. The strength and direction of the Coulomb force on a charge depends on the electric field established by other charges that it finds itself in, such that . In the simplest case, the field is considered to be generated solely by a single source
An electric field is a vector field that associates to each point in space the Coulomb force experienced by a unit test charge. The strength and direction of the Coulomb force on a charge depends on the electric field established by other charges that it finds itself in, such that . In the simplest case, the field is considered to be generated solely by a single source point charge
A point particle (ideal particle or point-like particle, often spelled pointlike particle) is an idealization of particles heavily used in physics. Its defining feature is that it lacks spatial extension; being dimensionless, it does not take u ...
. More generally, the field can be generated by a distribution of charges who contribute to the overall by the principle of superposition.
If the field is generated by a positive source point charge , the direction of the electric field points along lines directed radially outwards from it, i.e. in the direction that a positive point test charge would move if placed in the field. For a negative point source charge, the direction is radially inwards.
The magnitude of the electric field can be derived from Coulomb's law
Coulomb's inverse-square law, or simply Coulomb's law, is an experimental law of physics that quantifies the amount of force between two stationary, electrically charged particles. The electric force between charged bodies at rest is convention ...
. By choosing one of the point charges to be the source, and the other to be the test charge, it follows from Coulomb's law that the magnitude of the electric field created by a single source point charge
A point particle (ideal particle or point-like particle, often spelled pointlike particle) is an idealization of particles heavily used in physics. Its defining feature is that it lacks spatial extension; being dimensionless, it does not take u ...
''Q'' at a certain distance from it ''r'' in vacuum is given by
A system ''N'' of charges stationed at produces an electric field whose magnitude and direction is, by superposition
Atomic forces
Coulomb's law holds even withinatom
Every atom is composed of a nucleus and one or more electrons bound to the nucleus. The nucleus is made of one or more protons and a number of neutrons. Only the most common variety of hydrogen has no neutrons.
Every solid, liquid, gas, ...
s, correctly describing the force between the positively charged atomic nucleus
The atomic nucleus is the small, dense region consisting of protons and neutrons at the center of an atom, discovered in 1911 by Ernest Rutherford based on the 1909 GeigerŌĆōMarsden gold foil experiment. After the discovery of the neutron ...
and each of the negatively charged electron
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have no ...
s. This simple law also correctly accounts for the forces that bind atoms together to form molecule
A molecule is a group of two or more atoms held together by attractive forces known as chemical bonds; depending on context, the term may or may not include ions which satisfy this criterion. In quantum physics, organic chemistry, and bioche ...
s and for the forces that bind atoms and molecules together to form solids and liquids. Generally, as the distance between ion
An ion () is an atom or molecule with a net electrical charge.
The charge of an electron is considered to be negative by convention and this charge is equal and opposite to the charge of a proton, which is considered to be positive by conve ...
s increases, the force of attraction, and binding energy, approach zero and ionic bonding
Ionic bonding is a type of chemical bonding that involves the electrostatic attraction between oppositely charged ions, or between two atoms with sharply different electronegativities, and is the primary interaction occurring in ionic compounds ...
is less favorable. As the magnitude of opposing charges increases, energy increases and ionic bonding is more favorable.
Relation to Gauss's law
Deriving Gauss's law from Coulomb's law
Strictly speaking,Gauss's law
In physics and electromagnetism, Gauss's law, also known as Gauss's flux theorem, (or sometimes simply called Gauss's theorem) is a law relating the distribution of electric charge to the resulting electric field. In its integral form, it sta ...
cannot be derived from Coulomb's law alone, since Coulomb's law gives the electric field due to an individual point charge
A point particle (ideal particle or point-like particle, often spelled pointlike particle) is an idealization of particles heavily used in physics. Its defining feature is that it lacks spatial extension; being dimensionless, it does not take u ...
only. However, Gauss's law ''can'' be proven from Coulomb's law if it is assumed, in addition, that the electric field obeys the superposition principle. The superposition principle says that the resulting field is the vector sum of fields generated by each particle (or the integral, if the charges are distributed smoothly in space).
Note that since Coulomb's law only applies to stationary charges, there is no reason to expect Gauss's law to hold for moving charges based on this derivation alone. In fact, Gauss's law does hold for moving charges, and in this respect Gauss's law is more general than Coulomb's law.
Deriving Coulomb's law from Gauss's law
Strictly speaking, Coulomb's law cannot be derived from Gauss's law alone, since Gauss's law does not give any information regarding the curl of (seeHelmholtz decomposition
In physics and mathematics, in the area of vector calculus, Helmholtz's theorem, also known as the fundamental theorem of vector calculus, states that any sufficiently smooth, rapidly decaying vector field in three dimensions can be resolved into ...
and Faraday's law). However, Coulomb's law ''can'' be proven from Gauss's law if it is assumed, in addition, that the electric field from a point charge
A point particle (ideal particle or point-like particle, often spelled pointlike particle) is an idealization of particles heavily used in physics. Its defining feature is that it lacks spatial extension; being dimensionless, it does not take u ...
is spherically symmetric (this assumption, like Coulomb's law itself, is exactly true if the charge is stationary, and approximately true if the charge is in motion).
In relativity
Coulomb's law can be used to gain insight of the form of magnetic field generated by moving charges since by special relativity, in certain cases the magnetic field can be shown to be a transformation of forces caused by the electric field. When no acceleration is involved in a particle's history, it can be viewed to obey Coulomb's law on any test particle in its own inertial frame. Coulomb's law can be expanded to moving test particle to be of the same form, which shows discrepancy in Newton's third law as followed but it is not strictly obeyed in the framework of special relativity (yet without violating relativistic-energy conservation). This assumption can be justified by obtaining the correct form of field equations, that is with respect to agreement withmaxwell's equations
Maxwell's equations, or MaxwellŌĆōHeaviside equations, are a set of coupled partial differential equations that, together with the Lorentz force law, form the foundation of classical electromagnetism, classical optics, and electric circuits.
...
. Considering the charge to be invariant of observer, the electric and magnetic fields of a uniformly moving point charge can hence be derived by the Lorentz transformation
In physics, the Lorentz transformations are a six-parameter family of Linear transformation, linear coordinate transformation, transformations from a Frame of Reference, coordinate frame in spacetime to another frame that moves at a constant velo ...
of the four force on test charge given by Coulomb's law in the charge's frame of reference and attributing magnetic and electric fields by their definition given by the form of Lorentz force. The fields hence found for uniformly moving point charges are given by:where is the charge of the point source, is the position vector from the point source to the point in space, is the velocity vector of the charged particle, is the ratio of speed of the charged particle divided by the speed of light and is the angle between and .
Note that the expression for electric field reduces to Coulomb's law for non-relativistic speeds of the point charge and that the magnetic field in non-relativistic limit (approximating ) can be applied to electric currents to get the BiotŌĆōSavart law
In physics, specifically electromagnetism, the BiotŌĆōSavart law ( or ) is an equation describing the magnetic field generated by a constant electric current. It relates the magnetic field to the magnitude, direction, length, and proximity of the ...
. These solutions, when expressed in retarded time also correspond to the general solution of maxwell's equations
Maxwell's equations, or MaxwellŌĆōHeaviside equations, are a set of coupled partial differential equations that, together with the Lorentz force law, form the foundation of classical electromagnetism, classical optics, and electric circuits.
...
given by solutions of Li├®nardŌĆōWiechert potential
The Li├®nardŌĆōWiechert potentials describe the classical electromagnetic effect of a moving electric point charge in terms of a vector potential and a scalar potential in the Lorenz gauge. Stemming directly from Maxwell's equations, these descr ...
, due to the validity of Coulomb's law within its specific range of application. Also note that the spherical symmetry for gauss law on stationary charges is not valid for moving charges owing to the breaking of symmetry by the specification of direction of velocity in the problem. Maxwell's equations can also be manually verified for the above two equations.
Coulomb potential
Quantum field theory
Born approximation
Generally in scattering theory and in particular in quantum mechanics, the Born approximation consists of taking the incident field in place of the total field as the driving field at each point in the scatterer. The Born approximation is named a ...
, in non-relativistic quantum mechanics, the scattering amplitude is:
This is to be compared to the:
where we look at the (connected) S-matrix entry for two electrons scattering off each other, treating one with "fixed" momentum as the source of the potential, and the other scattering off that potential.
Using the Feynman rules to compute the S-matrix element, we obtain in the non-relativistic limit with
Comparing with the QM scattering, we have to discard the as they arise due to differing normalizations of momentum eigenstate in QFT compared to QM and obtain:
where Fourier transforming both sides, solving the integral and taking at the end will yield
as the Coulomb potential.
However, the equivalent results of the classical Born derivations for the Coulomb problem are thought to be strictly accidental.
The Coulomb potential, and its derivation, can be seen as a special case of the Yukawa potential
In particle, atomic and condensed matter physics, a Yukawa potential (also called a screened Coulomb potential) is a potential named after the Japanese physicist Hideki Yukawa. The potential is of the form:
:V_\text(r)= -g^2\frac,
where is a ...
, which is the case where the exchanged boson ŌĆō the photon ŌĆō has no rest mass.
Simple experiment to verify Coulomb's law
 It is possible to verify Coulomb's law with a simple experiment. Consider two small spheres of mass and same-sign charge , hanging from two ropes of negligible mass of length . The forces acting on each sphere are three: the weight , the rope tension and the electric force . In the equilibrium state:
and
Dividing () by ():
Let be the distance between the charged spheres; the repulsion force between them , assuming Coulomb's law is correct, is equal to
so:
If we now discharge one of the spheres, and we put it in contact with the charged sphere, each one of them acquires a charge . In the equilibrium state, the distance between the charges will be and the repulsion force between them will be:
We know that and:
Dividing () by (), we get:
Measuring the angles and and the distance between the charges and is sufficient to verify that the equality is true taking into account the experimental error. In practice, angles can be difficult to measure, so if the length of the ropes is sufficiently great, the angles will be small enough to make the following approximation:
Using this approximation, the relationship () becomes the much simpler expression:
In this way, the verification is limited to measuring the distance between the charges and check that the division approximates the theoretical value.
It is possible to verify Coulomb's law with a simple experiment. Consider two small spheres of mass and same-sign charge , hanging from two ropes of negligible mass of length . The forces acting on each sphere are three: the weight , the rope tension and the electric force . In the equilibrium state:
and
Dividing () by ():
Let be the distance between the charged spheres; the repulsion force between them , assuming Coulomb's law is correct, is equal to
so:
If we now discharge one of the spheres, and we put it in contact with the charged sphere, each one of them acquires a charge . In the equilibrium state, the distance between the charges will be and the repulsion force between them will be:
We know that and:
Dividing () by (), we get:
Measuring the angles and and the distance between the charges and is sufficient to verify that the equality is true taking into account the experimental error. In practice, angles can be difficult to measure, so if the length of the ropes is sufficiently great, the angles will be small enough to make the following approximation:
Using this approximation, the relationship () becomes the much simpler expression:
In this way, the verification is limited to measuring the distance between the charges and check that the division approximates the theoretical value.
See also
*BiotŌĆōSavart law
In physics, specifically electromagnetism, the BiotŌĆōSavart law ( or ) is an equation describing the magnetic field generated by a constant electric current. It relates the magnetic field to the magnitude, direction, length, and proximity of the ...
* Darwin Lagrangian
* Electromagnetic force
In physics, electromagnetism is an interaction that occurs between particles with electric charge. It is the second-strongest of the four fundamental interactions, after the strong force, and it is the dominant force in the interactions o ...
* Gauss's law
In physics and electromagnetism, Gauss's law, also known as Gauss's flux theorem, (or sometimes simply called Gauss's theorem) is a law relating the distribution of electric charge to the resulting electric field. In its integral form, it sta ...
* Method of image charges
* Molecular modelling
Molecular modelling encompasses all methods, theoretical and computational, used to model or mimic the behaviour of molecules. The methods are used in the fields of computational chemistry, drug design, computational biology and materials sci ...
* Newton's law of universal gravitation
Newton's law of universal gravitation is usually stated as that every particle attracts every other particle in the universe with a force that is proportional to the product of their masses and inversely proportional to the square of the distan ...
, which uses a similar structure, but for mass instead of charge
* Static forces and virtual-particle exchange
Static force fields are fields, such as a simple electric, magnetic or gravitational fields, that exist without excitations. The most common approximation method that physicists use for scattering calculations can be interpreted as static forces ...
References
Related reading
* * * * * * *External links
''Coulomb's Law''
o
Project PHYSNET
Ćöa chapter from an online textbook
Ćöa game created by the Molecular Workbench software
Walter Lewin, ''8.02 Electricity and Magnetism, Spring 2002: Lecture 1'' (video). MIT OpenCourseWare. License: Creative Commons Attribution-Noncommercial-Share Alike. {{Authority control Electromagnetism Electrostatics Force Scientific laws