|
Velocity-addition Formula
In relativistic physics, a velocity-addition formula is a three-dimensional equation that relates the velocities of objects in different reference frames. Such formulas apply to successive Lorentz transformations, so they also relate different frames. Accompanying velocity addition is a kinematic effect known as Thomas precession, whereby successive non-collinear Lorentz boosts become equivalent to the composition of a rotation of the coordinate system and a boost. Standard applications of velocity-addition formulas include the Doppler shift, Doppler navigation, the aberration of light, and the dragging of light in moving water observed in the 1851 Fizeau experiment. The notation employs as velocity of a body within a Lorentz frame , and as velocity of a second frame , as measured in , and as the transformed velocity of the body within the second frame. History The speed of light in a fluid is slower than the speed of light in vacuum, and it changes if the fluid is moving a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Albert Einstein Head
Albert may refer to: Companies * Albert (supermarket), a supermarket chain in the Czech Republic * Albert Heijn, a supermarket chain in the Netherlands * Albert Market, a street market in The Gambia * Albert Productions, a record label * Albert Computers, Inc., a computer manufacturer in the 1980s Entertainment * ''Albert'' (1985 film), a Czechoslovak film directed by František Vláčil * ''Albert'' (2015 film), a film by Karsten Kiilerich * ''Albert'' (2016 film), an American TV movie * ''Albert'' (Ed Hall album), 1988 * "Albert" (short story), by Leo Tolstoy * Albert (comics), a character in Marvel Comics * Albert (''Discworld''), a character in Terry Pratchett's ''Discworld'' series * Albert, a character in Dario Argento's 1977 film ''Suspiria'' Military * Battle of Albert (1914), a WWI battle at Albert, Somme, France * Battle of Albert (1916), a WWI battle at Albert, Somme, France * Battle of Albert (1918), a WWI battle at Albert, Somme, France People * Albert (give ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Special Relativity
In physics, the special theory of relativity, or special relativity for short, is a scientific theory regarding the relationship between space and time. In Albert Einstein's original treatment, the theory is based on two postulates: # The laws of physics are invariant (that is, identical) in all inertial frames of reference (that is, frames of reference with no acceleration). # The speed of light in vacuum is the same for all observers, regardless of the motion of the light source or the observer. Origins and significance Special relativity was originally proposed by Albert Einstein in a paper published on 26 September 1905 titled "On the Electrodynamics of Moving Bodies".Albert Einstein (1905)''Zur Elektrodynamik bewegter Körper'', ''Annalen der Physik'' 17: 891; English translatioOn the Electrodynamics of Moving Bodiesby George Barker Jeffery and Wilfrid Perrett (1923); Another English translation On the Electrodynamics of Moving Bodies by Megh Nad Saha (1920). The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Four-vector
In special relativity, a four-vector (or 4-vector) is an object with four components, which transform in a specific way under Lorentz transformations. Specifically, a four-vector is an element of a four-dimensional vector space considered as a representation space of the standard representation of the Lorentz group, the (,) representation. It differs from a Euclidean vector in how its magnitude is determined. The transformations that preserve this magnitude are the Lorentz transformations, which include spatial rotations and boosts (a change by a constant velocity to another inertial reference frame). Four-vectors describe, for instance, position in spacetime modeled as Minkowski space, a particle's four-momentum , the amplitude of the electromagnetic four-potential at a point in spacetime, and the elements of the subspace spanned by the gamma matrices inside the Dirac algebra. The Lorentz group may be represented by 4×4 matrices . The action of a Lorentz transfor ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Four Vector
In special relativity, a four-vector (or 4-vector) is an object with four components, which transform in a specific way under Lorentz transformations. Specifically, a four-vector is an element of a four-dimensional vector space considered as a representation space of the standard representation of the Lorentz group, the (,) representation. It differs from a Euclidean vector in how its magnitude is determined. The transformations that preserve this magnitude are the Lorentz transformations, which include spatial rotations and boosts (a change by a constant velocity to another inertial reference frame). Four-vectors describe, for instance, position in spacetime modeled as Minkowski space, a particle's four-momentum , the amplitude of the electromagnetic four-potential at a point in spacetime, and the elements of the subspace spanned by the gamma matrices inside the Dirac algebra. The Lorentz group may be represented by 4×4 matrices . The action of a Lorentz transformation on ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polar Coordinates
In mathematics, the polar coordinate system is a two-dimensional coordinate system in which each point on a plane is determined by a distance from a reference point and an angle from a reference direction. The reference point (analogous to the origin of a Cartesian coordinate system) is called the ''pole'', and the ray from the pole in the reference direction is the ''polar axis''. The distance from the pole is called the ''radial coordinate'', ''radial distance'' or simply ''radius'', and the angle is called the ''angular coordinate'', ''polar angle'', or ''azimuth''. Angles in polar notation are generally expressed in either degrees or radians (2 rad being equal to 360°). Grégoire de Saint-Vincent and Bonaventura Cavalieri independently introduced the concepts in the mid-17th century, though the actual term "polar coordinates" has been attributed to Gregorio Fontana in the 18th century. The initial motivation for the introduction of the polar system was the study of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lorentz Factor
The Lorentz factor or Lorentz term is a quantity expressing how much the measurements of time, length, and other physical properties change for an object while that object is moving. The expression appears in several equations in special relativity, and it arises in derivations of the Lorentz transformations. The name originates from its earlier appearance in Lorentzian electrodynamics – named after the Dutch physicist Hendrik Lorentz. It is generally denoted (the Greek lowercase letter gamma). Sometimes (especially in discussion of superluminal motion) the factor is written as (Greek uppercase-gamma) rather than . Definition The Lorentz factor is defined as :\gamma = \frac = \frac = \frac , where: *''v'' is the relative velocity between inertial reference frames, *''c'' is the ''speed of light in a vacuum'', * is the ratio of ''v'' to ''c'', *''t'' is coordinate time, * is the proper time for an observer (measuring time intervals in the observer's own frame). This is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Derivations Of The Lorentz Transformations
There are many ways to derive the Lorentz transformations utilizing a variety of physical principles, ranging from Maxwell's equations to Einstein's postulates of special relativity, and mathematical tools, spanning from elementary algebra and hyperbolic functions, to linear algebra and group theory. This article provides a few of the easier ones to follow in the context of special relativity, for the simplest case of a Lorentz boost in standard configuration, i.e. two inertial frames moving relative to each other at constant (uniform) relative velocity less than the speed of light, and using Cartesian coordinates so that the ''x'' and ''x''′ axes are collinear. Lorentz transformation In the fundamental branches of modern physics, namely general relativity and its widely applicable subset special relativity, as well as relativistic quantum mechanics and relativistic quantum field theory, the Lorentz transformation is the transformation rule under which all four-vectors and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Relativistic Mechanics
In physics, relativistic mechanics refers to mechanics compatible with special relativity (SR) and general relativity (GR). It provides a non-quantum mechanical description of a system of particles, or of a fluid, in cases where the velocities of moving objects are comparable to the speed of light ''c''. As a result, classical mechanics is extended correctly to particles traveling at high velocities and energies, and provides a consistent inclusion of electromagnetism with the mechanics of particles. This was not possible in Galilean relativity, where it would be permitted for particles and light to travel at ''any'' speed, including faster than light. The foundations of relativistic mechanics are the postulates of special relativity and general relativity. The unification of SR with quantum mechanics is relativistic quantum mechanics, while attempts for that of GR is quantum gravity, an unsolved problem in physics. As with classical mechanics, the subject can be divided into " ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
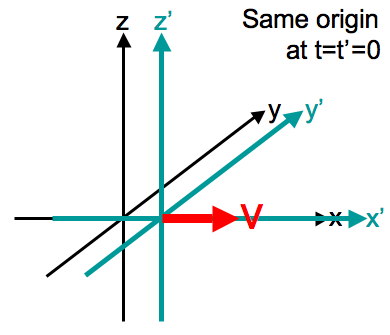
Lorentz Transformation
In physics, the Lorentz transformations are a six-parameter family of linear transformations from a coordinate frame in spacetime to another frame that moves at a constant velocity relative to the former. The respective inverse transformation is then parameterized by the negative of this velocity. The transformations are named after the Dutch physicist Hendrik Lorentz. The most common form of the transformation, parametrized by the real constant v, representing a velocity confined to the -direction, is expressed as \begin t' &= \gamma \left( t - \frac \right) \\ x' &= \gamma \left( x - v t \right)\\ y' &= y \\ z' &= z \end where and are the coordinates of an event in two frames with the origins coinciding at 0, where the primed frame is seen from the unprimed frame as moving with speed along the -axis, where is the speed of light, and \gamma = \left ( \sqrt\right )^ is the Lorentz factor. When speed is much smaller than , the Lorentz factor is negligibly different from ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Minkowski Spacetime
In mathematical physics, Minkowski space (or Minkowski spacetime) () is a combination of three-dimensional Euclidean space and time into a four-dimensional manifold where the spacetime interval between any two events is independent of the inertial frame of reference in which they are recorded. Although initially developed by mathematician Hermann Minkowski for Maxwell's equations of electromagnetism, the mathematical structure of Minkowski spacetime was shown to be implied by the postulates of special relativity. Minkowski space is closely associated with Einstein's theories of special relativity and general relativity and is the most common mathematical structure on which special relativity is formulated. While the individual components in Euclidean space and time may differ due to length contraction and time dilation, in Minkowski spacetime, all frames of reference will agree on the total distance in spacetime between events.This makes spacetime distance an invar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Newtonian Mechanics
Newton's laws of motion are three basic Scientific law, laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows: # A body remains at rest, or in motion at a constant speed in a straight line, unless acted upon by a force. # When a body is acted upon by a force, the time rate of change of its momentum equals the force. # If two bodies exert forces on each other, these forces have the same magnitude but opposite directions. The three laws of motion were first stated by Isaac Newton in his ''Philosophiæ Naturalis Principia Mathematica'' (''Mathematical Principles of Natural Philosophy''), originally published in 1687. Newton used them to investigate and explain the motion of many physical objects and systems, which laid the foundation for classical mechanics. In the time since Newton, the conceptual content of classical physics has been reformulated in alternative ways, inv ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Galilean Relativity
Galilean invariance or Galilean relativity states that the laws of motion are the same in all inertial frames of reference. Galileo Galilei first described this principle in 1632 in his '' Dialogue Concerning the Two Chief World Systems'' using the example of a ship travelling at constant velocity, without rocking, on a smooth sea; any observer below the deck would not be able to tell whether the ship was moving or stationary. The young Albert Einstein "was engrossed in analyzing Galileo's principle of inertia (Galilean relativity)". Formulation Specifically, the term ''Galilean invariance'' today usually refers to this principle as applied to Newtonian mechanics, that is, Newton's laws of motion hold in all frames related to one another by a Galilean transformation. In other words, all frames related to one another by such a transformation are inertial (meaning, Newton's equation of motion is valid in these frames). In this context it is sometimes called ''Newtonian relativity'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |