|
Toroid
In mathematics, a toroid is a surface of revolution with a hole in the middle. The axis of revolution passes through the hole and so does not intersect the surface. For example, when a rectangle is rotated around an axis parallel to one of its edges, then a hollow rectangle-section ring is produced. If the revolved figure is a circle, then the object is called a torus. The term ''toroid'' is also used to describe a toroidal polyhedron. In this context a toroid need not be circular and may have any number of holes. A ''g''-holed ''toroid'' can be seen as approximating the surface of a torus having a topological genus, ''g'', of 1 or greater. The Euler characteristic χ of a ''g'' holed toroid is 2(1-''g'').Stewart, B.; "Adventures Among the Toroids:A Study of Orientable Polyhedra with Regular Faces", 2nd Edition, Stewart (1980). The torus is an example of a toroid, which is the surface of a doughnut. Doughnuts are an example of a solid torus created by rotating a disk, and s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Toroidal Inductors And Transformers
Toroidal inductors and transformers are inductors and transformers which use magnetic cores with a toroidal (ring or donut) shape. They are passive electronic components, consisting of a circular ring or donut shaped magnetic core of ferromagnetic material such as laminated iron, iron powder, or ferrite, around which wire is wound. Although closed-core inductors and transformers often used cores with a square shape in the past, the use of toroidal-shaped cores has significantly increased because of their superior electrical performance. The advantage of the toroidal shape is that, due to its symmetry, the amount of magnetic flux that escapes outside the core (leakage flux) is low, therefore it is more efficient and thus radiates less electromagnetic interference (EMI). Toroidal inductors and transformers are used in a wide range of electronic circuits: power supplies, inverters, and amplifiers, which in turn are used in the vast majority of electrical equipment: TVs, rad ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Toroidal Polyhedron
In geometry, a toroidal polyhedron is a polyhedron which is also a toroid (a -holed torus), having a topological genus () of 1 or greater. Notable examples include the Császár and Szilassi polyhedra. Variations in definition Toroidal polyhedra are defined as collections of polygons that meet at their edges and vertices, forming a manifold as they do. That is, each edge should be shared by exactly two polygons, and at each vertex the edges and faces that meet at the vertex should be linked together in a single cycle of alternating edges and faces, the link of the vertex. For toroidal polyhedra, this manifold is an orientable surface. Some authors restrict the phrase "toroidal polyhedra" to mean more specifically polyhedra topologically equivalent to the (genus 1) torus. In this area, it is important to distinguish embedded toroidal polyhedra, whose faces are flat polygons in three-dimensional Euclidean space that do not cross themselves or each other, from abstract po ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Toroid By Zureks
In mathematics, a toroid is a surface of revolution with a hole in the middle. The axis of revolution passes through the hole and so does not intersect the surface. For example, when a rectangle is rotated around an axis parallel to one of its edges, then a hollow rectangle-section ring is produced. If the revolved figure is a circle, then the object is called a torus. The term ''toroid'' is also used to describe a toroidal polyhedron. In this context a toroid need not be circular and may have any number of holes. A ''g''-holed ''toroid'' can be seen as approximating the surface of a torus having a topological genus, ''g'', of 1 or greater. The Euler characteristic χ of a ''g'' holed toroid is 2(1-''g'').Stewart, B.; "Adventures Among the Toroids:A Study of Orientable Polyhedra with Regular Faces", 2nd Edition, Stewart (1980). The torus is an example of a toroid, which is the surface of a doughnut. Doughnuts are an example of a solid torus created by rotating a disk, and s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
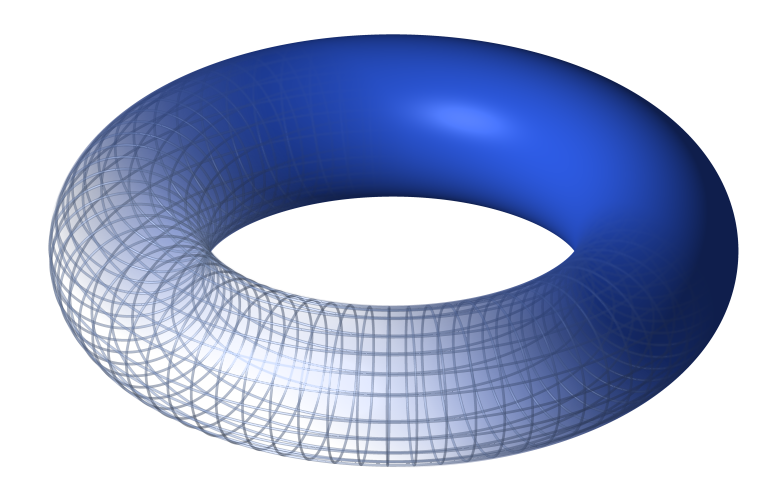
Torus
In geometry, a torus (plural tori, colloquially donut or doughnut) is a surface of revolution generated by revolving a circle in three-dimensional space about an axis that is coplanar with the circle. If the axis of revolution does not touch the circle, the surface has a ring shape and is called a torus of revolution. If the axis of revolution is tangent to the circle, the surface is a horn torus. If the axis of revolution passes twice through the circle, the surface is a spindle torus. If the axis of revolution passes through the center of the circle, the surface is a degenerate torus, a double-covered sphere. If the revolved curve is not a circle, the surface is called a '' toroid'', as in a square toroid. Real-world objects that approximate a torus of revolution include swim rings, inner tubes and ringette rings. Eyeglass lenses that combine spherical and cylindrical correction are toric lenses. A torus should not be confused with a ''solid torus'', which is form ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Solid Torus
In mathematics, a solid torus is the topological space formed by sweeping a disk around a circle. It is homeomorphic to the Cartesian product S^1 \times D^2 of the disk and the circle, endowed with the product topology. A standard way to visualize a solid torus is as a toroid, embedded in 3-space. However, it should be distinguished from a torus, which has the same visual appearance: the torus is the two-dimensional space on the boundary of a toroid, while the solid torus includes also the compact interior space enclosed by the torus. Topological properties The solid torus is a connected, compact, orientable 3-dimensional manifold with boundary. The boundary is homeomorphic to S^1 \times S^1, the ordinary torus. Since the disk D^2 is contractible, the solid torus has the homotopy type of a circle, S^1.. Therefore the fundamental group and homology groups are isomorphic to those of the circle: \begin \pi_1\left(S^1 \times D^2\right) &\cong \pi_1\left(S^1\right) \cong \m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Surface Of Revolution
A surface of revolution is a surface in Euclidean space created by rotating a curve (the generatrix) around an axis of rotation. Examples of surfaces of revolution generated by a straight line are cylindrical and conical surfaces depending on whether or not the line is parallel to the axis. A circle that is rotated around any diameter generates a sphere of which it is then a great circle, and if the circle is rotated around an axis that does not intersect the interior of a circle, then it generates a torus which does not intersect itself (a ring torus). Properties The sections of the surface of revolution made by planes through the axis are called ''meridional sections''. Any meridional section can be considered to be the generatrix in the plane determined by it and the axis. The sections of the surface of revolution made by planes that are perpendicular to the axis are circles. Some special cases of hyperboloids (of either one or two sheets) and elliptic paraboloids are s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Topology (Mathematics)
In mathematics, topology (from the Greek words , and ) is concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling, and bending; that is, without closing holes, opening holes, tearing, gluing, or passing through itself. A topological space is a set endowed with a structure, called a '' topology'', which allows defining continuous deformation of subspaces, and, more generally, all kinds of continuity. Euclidean spaces, and, more generally, metric spaces are examples of a topological space, as any distance or metric defines a topology. The deformations that are considered in topology are homeomorphisms and homotopies. A property that is invariant under such deformations is a topological property. Basic examples of topological properties are: the dimension, which allows distinguishing between a line and a surface; compactness, which allows distinguishing between a line and a circle; ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euler Characteristic
In mathematics, and more specifically in algebraic topology and polyhedral combinatorics, the Euler characteristic (or Euler number, or Euler–Poincaré characteristic) is a topological invariant, a number that describes a topological space's shape or structure regardless of the way it is bent. It is commonly denoted by \chi ( Greek lower-case letter chi). The Euler characteristic was originally defined for polyhedra and used to prove various theorems about them, including the classification of the Platonic solids. It was stated for Platonic solids in 1537 in an unpublished manuscript by Francesco Maurolico. Leonhard Euler, for whom the concept is named, introduced it for convex polyhedra more generally but failed to rigorously prove that it is an invariant. In modern mathematics, the Euler characteristic arises from homology and, more abstractly, homological algebra. Polyhedra The Euler characteristic \chi was classically defined for the surfaces of polyhedra, acc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Topology
In mathematics, topology (from the Greek words , and ) is concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling, and bending; that is, without closing holes, opening holes, tearing, gluing, or passing through itself. A topological space is a set endowed with a structure, called a ''topology'', which allows defining continuous deformation of subspaces, and, more generally, all kinds of continuity. Euclidean spaces, and, more generally, metric spaces are examples of a topological space, as any distance or metric defines a topology. The deformations that are considered in topology are homeomorphisms and homotopies. A property that is invariant under such deformations is a topological property. Basic examples of topological properties are: the dimension, which allows distinguishing between a line and a surface; compactness, which allows distinguishing between a line and a circle; connectedne ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is constant. The distance between any point of the circle and the centre is called the radius. Usually, the radius is required to be a positive number. A circle with r=0 (a single point) is a degenerate case. This article is about circles in Euclidean geometry, and, in particular, the Euclidean plane, except where otherwise noted. Specifically, a circle is a simple closed curve that divides the plane into two regions: an interior and an exterior. In everyday use, the term "circle" may be used interchangeably to refer to either the boundary of the figure, or to the whole figure including its interior; in strict technical usage, the circle is only the boundary and the whole figure is called a '' disc''. A circle may also be defined as a special ki ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Genus (mathematics)
In mathematics, genus (plural genera) has a few different, but closely related, meanings. Intuitively, the genus is the number of "holes" of a surface. A sphere has genus 0, while a torus has genus 1. Topology Orientable surfaces The genus of a connected, orientable surface is an integer representing the maximum number of cuttings along non-intersecting closed simple curves without rendering the resultant manifold disconnected. It is equal to the number of handles on it. Alternatively, it can be defined in terms of the Euler characteristic ''χ'', via the relationship ''χ'' = 2 − 2''g'' for closed surfaces, where ''g'' is the genus. For surfaces with ''b'' boundary components, the equation reads ''χ'' = 2 − 2''g'' − ''b''. In layman's terms, it's the number of "holes" an object has ("holes" interpreted in the sense of doughnut holes; a hollow sphere would be considered as having zero holes in this sense). A torus has ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |