|
Tiān Yuán Shù
''Tian yuan shu'' () is a Chinese system of algebra for polynomial equations. Some of the earliest existing writings were created in the 13th century during the Yuan dynasty. However, the tianyuanshu method was known much earlier, in the Song dynasty and possibly before. History The Tianyuanshu was explained in the writings of Zhu Shijie (''Jade Mirror of the Four Unknowns'') and Li Zhi (''Ceyuan haijing''), two Chinese mathematicians during the Mongol Yuan dynasty. However, after the Ming overthrew the Mongol Yuan, Zhu and Li's mathematical works went into disuse as the Ming literati became suspicious of knowledge imported from Mongol Yuan times. Only recently, with the advent of modern mathematics in China has the tianyuanshu been re-deciphered. Meanwhile, ''tian yuan shu'' arrived in Japan, where it is called ''tengen-jutsu''. Zhu's text '' Suanxue qimeng'' was deciphered and was important in the development of Japanese mathematics (''wasan'') in the 17th and 18th centuri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rod Numeral
Counting rods () are small bars, typically 3–14 cm long, that were used by mathematicians for calculation in ancient East Asia. They are placed either horizontally or vertically to represent any integer or rational number. The written forms based on them are called rod numerals. They are a true positional numeral system with digits for 1–9 and a blank for 0, from the Warring states period (circa 475 BCE) to the 16th century. History Chinese arithmeticians used counting rods well over two thousand years ago. In 1954 forty-odd counting rods of the Warring States period (5th century BCE to 221 BCE) were found in Zuǒjiāgōngshān (左家公山) Chu Grave No.15 in Changsha, Hunan. In 1973 archeologists unearthed a number of wood scripts from a tomb in Hubei dating from the period of the Han dynasty (206 BCE to 220 CE). On one of the wooden scripts was written: "当利二月定算𝍥". This is one of the earliest examples of using counting-rod numerals in writing. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
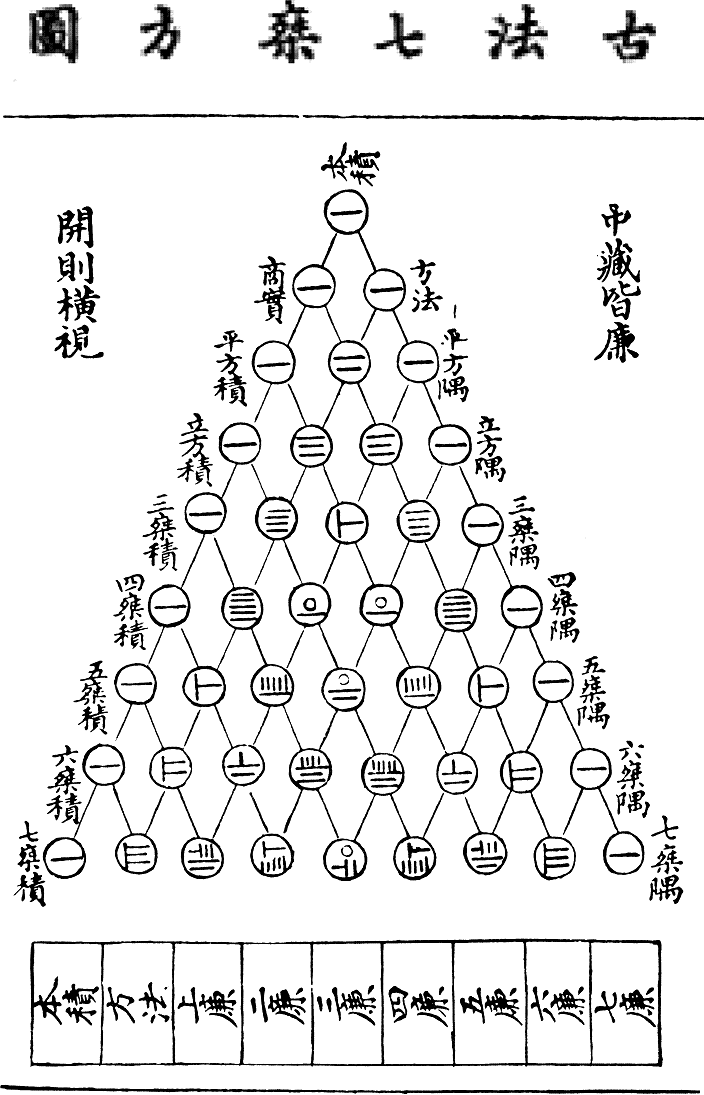
Chinese Mathematics
Mathematics in China emerged independently by the 11th century BCE. The Chinese independently developed a real number system that includes significantly large and negative numbers, more than one numeral system ( base 2 and base 10), algebra, geometry, number theory and trigonometry. Since the Han Dynasty, as diophantine approximation being a prominent numerical method, the Chinese made substantial progress on polynomial evaluation. Algorithms like regula falsi and expressions like continued fractions are widely used and have been well-documented ever-since. They deliberately find the principal ''n''th root of positive numbers and the roots of equations. The major texts from the period, ''The Nine Chapters on the Mathematical Art'' and the ''Book on Numbers and Computation'' gave detailed processes for solving various mathematical problems in daily life. All procedures were computed using a counting board in both texts, and they included inverse elements as well as Euclidean divi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yigu Yanduan
''Yigu yanduan'' (益古演段 Old Mathematics in Expanded Sections) is a 13th-century mathematical work by Yuan dynasty mathematician Li Zhi. Overview ''Yigu yanduan'' was based on Northern Song mathematician Jiang Zhou's (蒋周) ''Yigu Ji'' (益古集 Collection of Old Mathematics) which is not extant. However, from fragments quoted in Yang Hui's work ''The Complete Algorithms of Acreage'' (田亩比类算法大全), this lost mathematical treatise ''Yigu Ji'' was about solving area problems with geometry. Li Zhi used the examples of ''Yigu Ji'' to introduce the art of Tian yuan shu to newcomers to this field. Although Li Zhi's previous monograph ''Ceyuan haijing'' also used Tian yuan shu, it is harder to understand than ''Yigu yanduan''. ''Yigu yanduan'' was later collected into '' Siku Quanshu''. ''Yigu yanduan'' consists of three volumes with 64 problems solved using Tian yuan sh] in parallel with the geometrical method. Li Zhi intended to introduce students to th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Exponent
Exponentiation is a mathematical operation, written as , involving two numbers, the '' base'' and the ''exponent'' or ''power'' , and pronounced as " (raised) to the (power of) ". When is a positive integer, exponentiation corresponds to repeated multiplication of the base: that is, is the product of multiplying bases: b^n = \underbrace_. The exponent is usually shown as a superscript to the right of the base. In that case, is called "''b'' raised to the ''n''th power", "''b'' (raised) to the power of ''n''", "the ''n''th power of ''b''", "''b'' to the ''n''th power", or most briefly as "''b'' to the ''n''th". Starting from the basic fact stated above that, for any positive integer n, b^n is n occurrences of b all multiplied by each other, several other properties of exponentiation directly follow. In particular: \begin b^ & = \underbrace_ \\ ex& = \underbrace_ \times \underbrace_ \\ ex& = b^n \times b^m \end In other words, when multiplying a base raised to one exp ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quadratic Function
In mathematics, a quadratic polynomial is a polynomial of degree two in one or more variables. A quadratic function is the polynomial function defined by a quadratic polynomial. Before 20th century, the distinction was unclear between a polynomial and its associated polynomial function; so "quadratic polynomial" and "quadratic function" were almost synonymous. This is still the case in many elementary courses, where both terms are often abbreviated as "quadratic". For example, a univariate (single-variable) quadratic function has the form :f(x)=ax^2+bx+c,\quad a \ne 0, where is its variable. The graph of a function, graph of a univariate quadratic function is a parabola, a curve that has an axis of symmetry parallel to the -axis. If a quadratic function is equation, equated with zero, then the result is a quadratic equation. The solutions of a quadratic equation are the zero of a function, zeros of the corresponding quadratic function. The bivariate function, bivariate case ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Coefficient
In mathematics, a coefficient is a multiplicative factor in some term of a polynomial, a series, or an expression; it is usually a number, but may be any expression (including variables such as , and ). When the coefficients are themselves variables, they may also be called parameters. For example, the polynomial 2x^2-x+3 has coefficients 2, −1, and 3, and the powers of the variable x in the polynomial ax^2+bx+c have coefficient parameters a, b, and c. The constant coefficient is the coefficient not attached to variables in an expression. For example, the constant coefficients of the expressions above are the number 3 and the parameter ''c'', respectively. The coefficient attached to the highest degree of the variable in a polynomial is referred to as the leading coefficient. For example, in the expressions above, the leading coefficients are 2 and ''a'', respectively. Terminology and definition In mathematics, a coefficient is a multiplicative factor in some term of a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polynomial Equation In Tian Yuan Shu With Arabic Numerals
In mathematics, a polynomial is an expression consisting of indeterminates (also called variables) and coefficients, that involves only the operations of addition, subtraction, multiplication, and positive-integer powers of variables. An example of a polynomial of a single indeterminate is . An example with three indeterminates is . Polynomials appear in many areas of mathematics and science. For example, they are used to form polynomial equations, which encode a wide range of problems, from elementary word problems to complicated scientific problems; they are used to define polynomial functions, which appear in settings ranging from basic chemistry and physics to economics and social science; they are used in calculus and numerical analysis to approximate other functions. In advanced mathematics, polynomials are used to construct polynomial rings and algebraic varieties, which are central concepts in algebra and algebraic geometry. Etymology The word ''polynomial'' j ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polynomial Equation With Rod Numerals
In mathematics, a polynomial is an expression consisting of indeterminates (also called variables) and coefficients, that involves only the operations of addition, subtraction, multiplication, and positive-integer powers of variables. An example of a polynomial of a single indeterminate is . An example with three indeterminates is . Polynomials appear in many areas of mathematics and science. For example, they are used to form polynomial equations, which encode a wide range of problems, from elementary word problems to complicated scientific problems; they are used to define polynomial functions, which appear in settings ranging from basic chemistry and physics to economics and social science; they are used in calculus and numerical analysis to approximate other functions. In advanced mathematics, polynomials are used to construct polynomial rings and algebraic varieties, which are central concepts in algebra and algebraic geometry. Etymology The word ''polynomial'' joins tw ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polynomial Equation
In mathematics, an algebraic equation or polynomial equation is an equation of the form :P = 0 where ''P'' is a polynomial with coefficients in some field, often the field of the rational numbers. For many authors, the term ''algebraic equation'' refers only to ''univariate equations'', that is polynomial equations that involve only one variable. On the other hand, a polynomial equation may involve several variables. In the case of several variables (the ''multivariate'' case), the term ''polynomial equation'' is usually preferred to ''algebraic equation''. For example, :x^5-3x+1=0 is an algebraic equation with integer coefficients and :y^4 + \frac - \frac + xy^2 + y^2 + \frac = 0 is a multivariate polynomial equation over the rationals. Some but not all polynomial equations with rational coefficients have a solution that is an algebraic expression that can be found using a finite number of operations that involve only those same types of coefficients (that is, can be solved a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Variable (mathematics)
In mathematics, a variable (from Latin '' variabilis'', "changeable") is a symbol that represents a mathematical object. A variable may represent a number, a vector, a matrix, a function, the argument of a function, a set, or an element of a set. Algebraic computations with variables as if they were explicit numbers solve a range of problems in a single computation. For example, the quadratic formula solves any quadratic equation by substituting the numeric values of the coefficients of that equation for the variables that represent them in the quadratic formula. In mathematical logic, a ''variable'' is either a symbol representing an unspecified term of the theory (a meta-variable), or a basic object of the theory that is manipulated without referring to its possible intuitive interpretation. History In ancient works such as Euclid's ''Elements'', single letters refer to geometric points and shapes. In the 7th century, Brahmagupta used different colours to represent the u ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wylie On Tian Yuen
Wylie is an English name meaning "well-watered meadow", and may also refer to: People * Wylie (surname) * Wylie (Australian explorer), Aboriginal companion of Edward John Eyre during his crossing of the Nullarbor Plain in Australia * Wylie Breckenridge (1903–1991), rugby union player who represented Australia * Wylie Gibbs (born 1922), Australian politician * Wylie Cameron Grant (1879–1968), American tennis champion * Wylie Human (born 1979), South African rugby union winger * Wylie Stateman, American supervising sound editor * Wylie Sypher (1905–1987), American non-fiction writer and professor * Wylie Watson (1889–1966), British actor * Wylie G. Woodruff (1866–1930), American football player and coach Fictional characters * Wile E. Coyote, a cartoon character whose name sounds similar to "Wily" * Wylie Burp, a character from the film ''An American Tail: Fievel Goes West'' * Wylie Endal, a character from ''Keeper Of The Lost Cities'' by Shannon Messenger Places U ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
