|
Trochoid
In geometry, a trochoid () is a roulette curve formed by a circle rolling along a line. It is the curve traced out by a point fixed to a circle (where the point may be on, inside, or outside the circle) as it rolls along a straight line. If the point is on the circle, the trochoid is called ''common'' (also known as a cycloid); if the point is inside the circle, the trochoid is ''curtate''; and if the point is outside the circle, the trochoid is ''prolate''. The word "trochoid" was coined by Gilles de Roberval. Basic description As a circle of radius rolls without slipping along a line , the center moves parallel to , and every other point in the rotating plane rigidly attached to the circle traces the curve called the trochoid. Let . Parametric equations of the trochoid for which is the -axis are :\begin & x = a\theta - b \sin \theta \\ & y = a - b \cos \theta \end where is the variable angle through which the circle rolls. Curtate, common, prolate If lies inside the ci ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
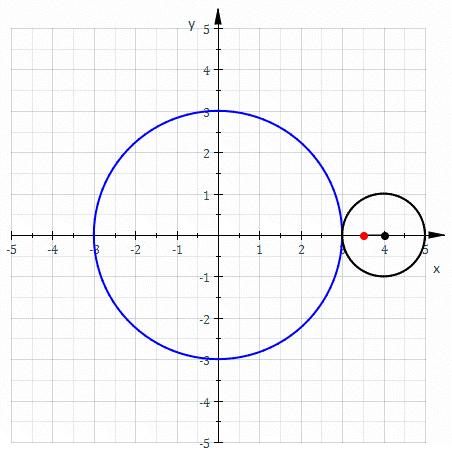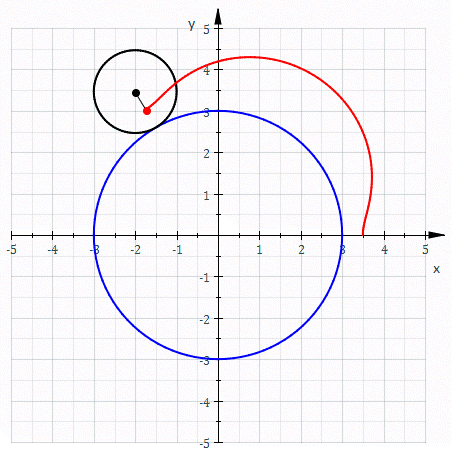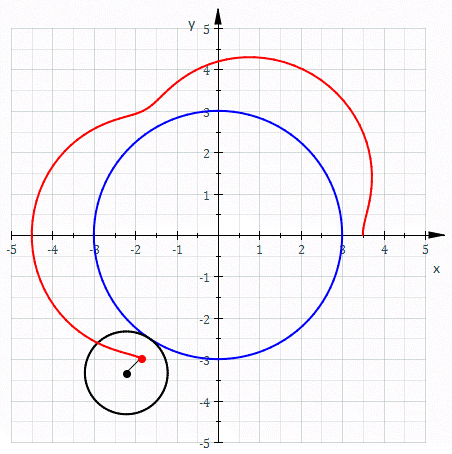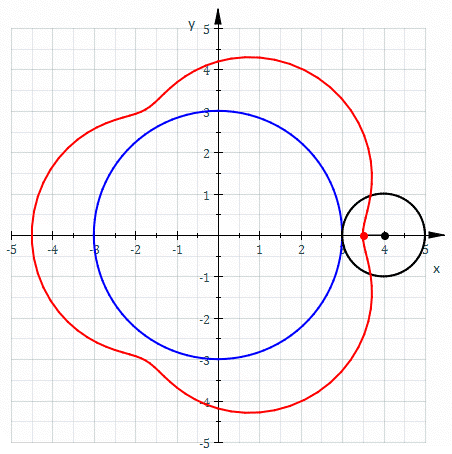
Epitrochoid
In geometry, an epitrochoid ( or ) is a roulette traced by a point attached to a circle of radius rolling around the outside of a fixed circle of radius , where the point is at a distance from the center of the exterior circle. The parametric equations for an epitrochoid are :\begin & x (\theta) = (R + r)\cos\theta - d\cos\left(\theta\right) \\ & y (\theta) = (R + r)\sin\theta - d\sin\left(\theta\right) \end The parameter is geometrically the polar angle of the center of the exterior circle. (However, is not the polar angle of the point (x(\theta),y(\theta)) on the epitrochoid.) Special cases include the limaçon with and the epicycloid with . The classic Spirograph toy traces out epitrochoid and hypotrochoid curves. The orbits of planets in the once popular geocentric Ptolemaic system are epitrochoids (see deferent and epicycle). The orbit of the moon, when centered around the sun, approximates an epitrochoid. The combustion chamber of the Wankel engine is an epitro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hypotrochoid
In geometry, a hypotrochoid is a roulette traced by a point attached to a circle of radius rolling around the inside of a fixed circle of radius , where the point is a distance from the center of the interior circle. The parametric equations for a hypotrochoid are: :\begin & x (\theta) = (R - r)\cos\theta + d\cos\left(\theta\right) \\ & y (\theta) = (R - r)\sin\theta - d\sin\left(\theta\right) \end where is the angle formed by the horizontal and the center of the rolling circle (these are not polar equations because is not the polar angle). When measured in radian, takes values from 0 to 2 \pi \times \tfrac (where is least common multiple). Special cases include the hypocycloid with and the ellipse with and . The eccentricity of the ellipse is :e=\frac becoming 1 when d=r (see Tusi couple). The classic Spirograph toy traces out hypotrochoid and epitrochoid curves. Hypotrochoids describe the support of the eigenvalues of some random matrices with cyclic correlatio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trochoidal Wave
In fluid dynamics, a trochoidal wave or Gerstner wave is an exact solution of the Euler equations for periodic surface gravity waves. It describes a progressive wave of permanent form on the surface of an incompressible fluid of infinite depth. The free surface of this wave solution is an inverted (upside-down) trochoid – with sharper crests and flat troughs. This wave solution was discovered by Gerstner in 1802, and rediscovered independently by Rankine in 1863. The flow field associated with the trochoidal wave is not irrotational: it has vorticity. The vorticity is of such a specific strength and vertical distribution that the trajectories of the fluid parcels are closed circles. This is in contrast with the usual experimental observation of Stokes drift associated with the wave motion. Also the phase speed is independent of the trochoidal wave's amplitude, unlike other nonlinear wave-theories (like those of the Stokes wave and cnoidal wave) and observations. For these ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Roulette (curve)
In the differential geometry of curves, a roulette is a kind of curve, generalizing cycloids, epicycloids, hypocycloids, trochoids, epitrochoids, hypotrochoids, and involutes. Definition Informal definition Roughly speaking, a roulette is the curve described by a point (called the ''generator'' or ''pole'') attached to a given curve as that curve rolls without slipping, along a second given curve that is fixed. More precisely, given a curve attached to a plane which is moving so that the curve rolls, without slipping, along a given curve attached to a fixed plane occupying the same space, then a point attached to the moving plane describes a curve, in the fixed plane called a roulette. Special cases and related concepts In the case where the rolling curve is a line and the generator is a point on the line, the roulette is called an involute of the fixed curve. If the rolling curve is a circle and the fixed curve is a line then the roulette is a trochoid. If, in this case, t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Parametric Equation
In mathematics, a parametric equation defines a group of quantities as functions of one or more independent variables called parameters. Parametric equations are commonly used to express the coordinates of the points that make up a geometric object such as a curve or surface, in which case the equations are collectively called a parametric representation or parameterization (alternatively spelled as parametrisation) of the object. For example, the equations :\begin x &= \cos t \\ y &= \sin t \end form a parametric representation of the unit circle, where ''t'' is the parameter: A point (''x'', ''y'') is on the unit circle if and only if there is a value of ''t'' such that these two equations generate that point. Sometimes the parametric equations for the individual scalar output variables are combined into a single parametric equation in vectors: :(x, y)=(\cos t, \sin t). Parametric representations are generally nonunique (see the "Examples in two dimensions" section ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Periodic Functions
This is a list of some well-known periodic functions. The constant function , where is independent of , is periodic with any period, but lacks a ''fundamental period''. A definition is given for some of the following functions, though each function may have many equivalent definitions. Smooth functions All trigonometric functions listed have period 2\pi, unless otherwise stated. For the following trigonometric functions: : is the th up/down number, : is the th Bernoulli number Non-smooth functions The following functions have period p and take x as their argument. The symbol \lfloor n \rfloor is the floor function of n and \sgn is the sign function. Vector-valued functions * Epitrochoid * Epicycloid (special case of the epitrochoid) * Limaçon (special case of the epitrochoid) * Hypotrochoid * Hypocycloid In geometry, a hypocycloid is a special plane curve generated by the trace of a fixed point on a small circle that rolls within a larger circle. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hypocycloid
In geometry, a hypocycloid is a special plane curve generated by the trace of a fixed point on a small circle that rolls within a larger circle. As the radius of the larger circle is increased, the hypocycloid becomes more like the cycloid created by rolling a circle on a line. Properties If the smaller circle has radius , and the larger circle has radius , then the parametric equations for the curve can be given by either: :\begin & x (\theta) = (R - r) \cos \theta + r \cos \left(\frac \theta \right) \\ & y (\theta) = (R - r) \sin \theta - r \sin \left( \frac \theta \right) \end or: :\begin & x (\theta) = r (k - 1) \cos \theta + r \cos \left( (k - 1) \theta \right) \\ & y (\theta) = r (k - 1) \sin \theta - r \sin \left( (k - 1) \theta \right) \end If is an integer, then the curve is closed, and has cusps (i.e., sharp corners, where the curve is not differentiable). Specially for the curve is a straight line and the circles are called Cardano circles. Girolamo Car ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
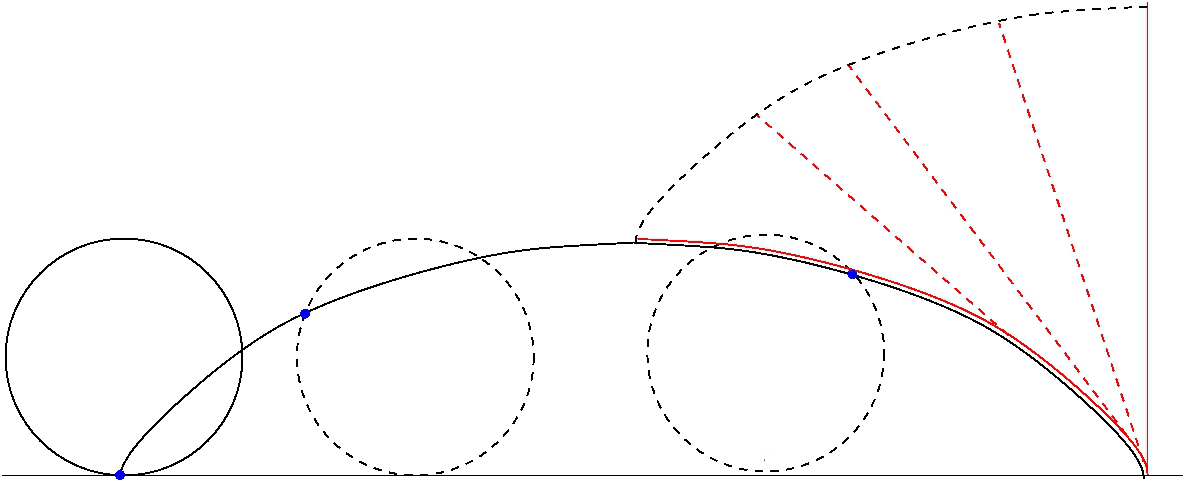
Cycloid
In geometry, a cycloid is the curve traced by a point on a circle as it rolls along a straight line without slipping. A cycloid is a specific form of trochoid and is an example of a roulette, a curve generated by a curve rolling on another curve. The cycloid, with the cusps pointing upward, is the curve of fastest descent under uniform gravity (the brachistochrone curve). It is also the form of a curve for which the period of an object in simple harmonic motion (rolling up and down repetitively) along the curve does not depend on the object's starting position (the tautochrone curve). History The cycloid has been called "The Helen of Geometers" as it caused frequent quarrels among 17th-century mathematicians. Historians of mathematics have proposed several candidates for the discoverer of the cycloid. Mathematical historian Paul Tannery cited similar work by the Syrian philosopher Iamblichus as evidence that the curve was known in antiquity. English mathemati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Epicycloid
In geometry, an epicycloid is a plane curve produced by tracing the path of a chosen point on the circumference of a circle—called an ''epicycle''—which rolls without slipping around a fixed circle. It is a particular kind of roulette. Equations If the smaller circle has radius , and the larger circle has radius , then the parametric equations for the curve can be given by either: :\begin & x (\theta) = (R + r) \cos \theta \ - r \cos \left( \frac \theta \right) \\ & y (\theta) = (R + r) \sin \theta \ - r \sin \left( \frac \theta \right) \end or: :\begin & x (\theta) = r (k + 1) \cos \theta - r \cos \left( (k + 1) \theta \right) \\ & y (\theta) = r (k + 1) \sin \theta - r \sin \left( (k + 1) \theta \right) \end in a more concise and complex form :z(\theta) = r \left(e^ - (k + 1)e^ \right) where * angle is in turns: \theta \in , 2\pi * smaller circle has radius * the larger circle has radius Area (Assuming the initial point lies on the larger circle.) When is a pos ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cycloid F
In geometry, a cycloid is the curve traced by a point on a circle as it rolls along a straight line without slipping. A cycloid is a specific form of trochoid and is an example of a roulette, a curve generated by a curve rolling on another curve. The cycloid, with the cusps pointing upward, is the curve of fastest descent under uniform gravity (the brachistochrone curve). It is also the form of a curve for which the period of an object in simple harmonic motion (rolling up and down repetitively) along the curve does not depend on the object's starting position (the tautochrone curve). History The cycloid has been called "The Helen of Geometers" as it caused frequent quarrels among 17th-century mathematicians. Historians of mathematics have proposed several candidates for the discoverer of the cycloid. Mathematical historian Paul Tannery cited similar work by the Syrian philosopher Iamblichus as evidence that the curve was known in antiquity. English mathematici ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |