|
Scale (spatial)
Spatial scale is a specific application of the term scale for describing or categorizing (e.g. into orders of magnitude) the size of a space (hence ''spatial''), or the extent of it at which a phenomenon or process occurs. For instance, in physics an object or phenomenon can be called microscopic if too small to be visible. In climatology, a micro-climate is a climate which might occur in a mountain, valley or near a lake shore. In statistics, a megatrend is a political, social, economical, environmental or technological trend which involves the whole planet or is supposed to last a very large amount of time. The concept is also used in geography, astronomy, and meteorology. These divisions are somewhat arbitrary; where, on this table, ''mega-'' is assigned global scope, it may only apply continentally or even regionally in other contexts. The interpretations of ''meso-'' and ''macro-'' must then be adjusted accordingly. See also * Astronomical units of length * Cosmi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Scale (other)
Scale or scales may refer to: Mathematics * Scale (descriptive set theory), an object defined on a set of points * Scale (ratio), the ratio of a linear dimension of a model to the corresponding dimension of the original * Scale factor, a number which scales, or multiplies, some quantity * Long and short scales, how powers of ten are named and grouped in large numbers * Scale parameter, a description of the spread or dispersion of a probability distribution * Feature scaling, a method used to normalize the range of independent variables or features of data * Scale (analytical tool) Measurements * Scale (map), the ratio of the distance on a map to the corresponding actual distance * Weighing scale, an instrument used to measure mass * Scale (ratio), the ratio of the linear dimension of the model to the same dimension of the original * Spatial scale, a classification of sizes * Scale ruler, a tool for measuring lengths and transferring measurements at a fixed ratio of le ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
A Cosmic Sense Of Scale
A, or a, is the first letter and the first vowel of the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is ''a'' (pronounced ), plural ''aes''. It is similar in shape to the Ancient Greek letter alpha, from which it derives. The uppercase version consists of the two slanting sides of a triangle, crossed in the middle by a horizontal bar. The lowercase version can be written in two forms: the double-storey a and single-storey ɑ. The latter is commonly used in handwriting and fonts based on it, especially fonts intended to be read by children, and is also found in italic type. In English grammar, " a", and its variant " an", are indefinite articles. History The earliest certain ancestor of "A" is aleph (also written 'aleph), the first letter of the Phoenician alphabet, which consisted entirely of consonants (for that reason, it is also called an abjad to distinguish ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geography Terminology
Geography (from Greek: , ''geographia''. Combination of Greek words ‘Geo’ (The Earth) and ‘Graphien’ (to describe), literally "earth description") is a field of science devoted to the study of the lands, features, inhabitants, and phenomena of Earth. The first recorded use of the word γεωγραφία was as a title of a book by Greek scholar Eratosthenes (276–194 BC). Geography is an all-encompassing discipline that seeks an understanding of Earth and its human and natural complexities—not merely where objects are, but also how they have changed and come to be. While geography is specific to Earth, many concepts can be applied more broadly to other celestial bodies in the field of planetary science. One such concept, the first law of geography, proposed by Waldo Tobler, is "everything is related to everything else, but near things are more related than distant things." Geography has been called "the world discipline" and "the bridge between the human and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Concepts In Physics
Concepts are defined as abstract ideas. They are understood to be the fundamental building blocks of the concept behind principles, thoughts and beliefs. They play an important role in all aspects of cognition. As such, concepts are studied by several disciplines, such as linguistics, psychology, and philosophy, and these disciplines are interested in the logical and psychological structure of concepts, and how they are put together to form thoughts and sentences. The study of concepts has served as an important flagship of an emerging interdisciplinary approach called cognitive science. In contemporary philosophy, there are at least three prevailing ways to understand what a concept is: * Concepts as mental representations, where concepts are entities that exist in the mind (mental objects) * Concepts as abilities, where concepts are abilities peculiar to cognitive agents (mental states) * Concepts as Fregean senses, where concepts are abstract objects, as opposed to mental obj ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Location Of Earth
Knowledge of the location of Earth has been shaped by 400 years of telescopic observations, and has expanded radically since the start of the 20th century. Initially, Earth was believed to be the center of the Universe, which consisted only of those planets visible with the naked eye and an outlying sphere of fixed stars. After the acceptance of the heliocentric model in the 17th century, observations by William Herschel and others showed that the Sun lay within a vast, disc-shaped galaxy of stars. By the 20th century, observations of spiral nebulae revealed that the Milky Way galaxy was one of billions in an expanding universe, grouped into clusters and superclusters. By the end of the 20th century, the overall structure of the visible universe was becoming clearer, with superclusters forming into a vast web of filaments and voids. Superclusters, filaments and voids are the largest coherent structures in the Universe that we can observe. At still larger scales (over 1000 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
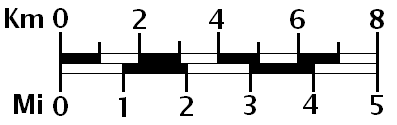
Scale (ratio)
The scale ratio of a model represents the proportional ratio of a linear dimension of the model to the same feature of the original. Examples include a 3-dimensional scale model of a building or the scale drawings of the elevations or plans of a building. In such cases the scale is dimensionless and exact throughout the model or drawing. The scale can be expressed in four ways: in words (a lexical scale), as a ratio, as a fraction and as a graphical (bar) scale. Thus on an architect's drawing one might read 'one centimeter to one meter', 1:100, 1/100, or . A bar scale would also normally appear on the drawing. Colon may also be substituted with a specific, slightly raised ratio symbol , ie. . General representation In general a representation may involve more than one scale at the same time. For example, a drawing showing a new road in elevation might use different horizontal and vertical scales. An elevation of a bridge might be annotated with arrows with a length proport ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Scale (map)
The scale of a map is the ratio of a distance on the map to the corresponding distance on the ground. This simple concept is complicated by the curvature of the Earth's surface, which forces scale to vary across a map. Because of this variation, the concept of scale becomes meaningful in two distinct ways. The first way is the ratio of the size of the generating globe to the size of the Earth. The generating globe is a conceptual model to which the Earth is shrunk and from which the map is projected. The ratio of the Earth's size to the generating globe's size is called the nominal scale (= principal scale = representative fraction). Many maps state the nominal scale and may even display a bar scale (sometimes merely called a 'scale') to represent it. The second distinct concept of scale applies to the variation in scale across a map. It is the ratio of the mapped point's scale to the nominal scale. In this case 'scale' means the scale factor (= point scale = particular scal ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Scale (analytical Tool)
In the study of complex systems and hierarchy theory, the concept of scale refers to the combination of (1) the level of analysis (for example, analyzing the whole or a specific component of the system); and (2) the level of observation (for example, observing a system as an external viewer or as an internal participant). The scale of analysis encompasses both the analytical choice of how to observe a given system or object of study, and the role of the observer in determining the identity of the system. This analytical tool is central to multi-scale analysis (see for example, MuSIASEM, land-use analysis). For example, on at the scale of analysis of a given population of zebras, the number of predators (e.g. lions) determines the number of preys that survives after hunting, while at the scale of analysis of the ecosystem, the availability of preys determines how many predators can survive in a given area. The semantic categories of "prey" and "predator" are not given, but are de ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
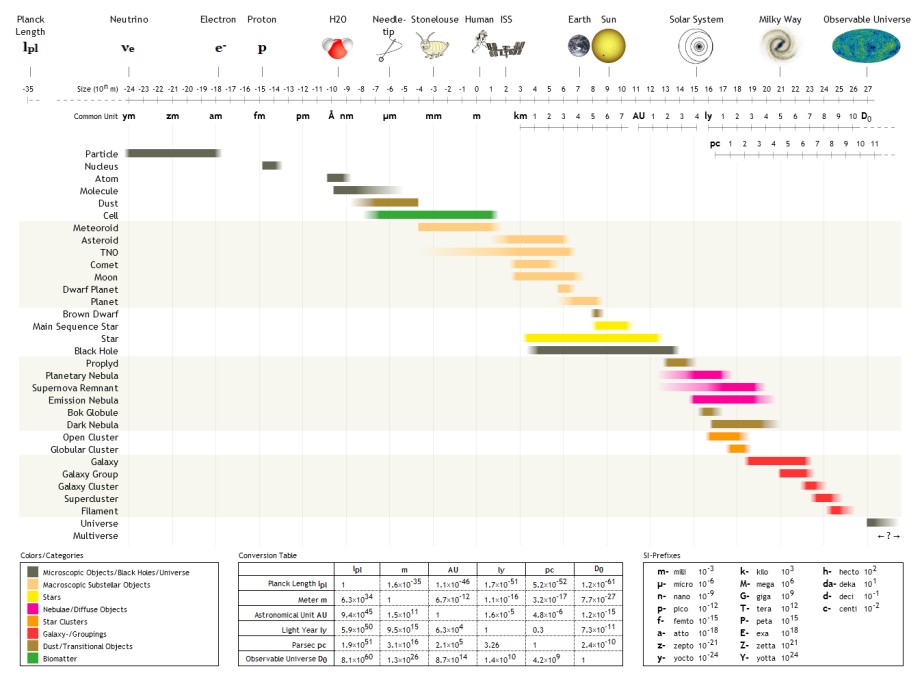
Orders Of Magnitude (length)
The following are examples of orders of magnitude for different lengths. __TOC__ Overview Detailed list To help compare different orders of magnitude, the following list describes various lengths between 1.6 \times 10^ metres and 10^metres. Subatomic scale Atomic to cellular scale Cellular to human scale Human to astronomical scale Astronomical scale Less than 1 zeptometre The ' ( SI symbol: ') is a unit of length in the metric system equal to . To help compare different orders of magnitude, this section lists lengths shorter than 10−21 m (1 zm). *1.6 × 10−5 quectometres (1.6 × 10−35 metres) – the Planck length (Measures of distance shorter than this do not make physical sense, according to current theories of physics.) *1 qm – 1 quectometre, the smallest named subdivision of the metre in the SI base unit of length, one nonillionth of a metre *1 rm – 1 rontometre, a subdivision of the metre in the SI base unit of length, one octillio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Examples Of Lengths
This is a list of examples of lengths, in metres in order to give an understanding of lengths. Shorter than 1 ym * metres = qm = the Planck length * metres = 1 qm = 1 quectometre, the smallest named subdivision of the metre in the SI base unit of length. * metres = 1 rm = 1 rontometre = quectometres 1 ym to 1 zm * metres = 1 ym = 1 yoctometre = rontometres * metres = 10 ym * metres = 20 ym, the effective cross-section radius of 1 MeV neutrinos as measured by Clyde Cowan and Frederick Reines 1 zm to 1 am * metres = 1 zm = 1 zeptometre = yoctometres * metres = radius of effective cross section for a 20 GeV neutrino scattering off a nucleon (area for 20 GeV about gives effective radius of about ; for 250 GeV about gives effective radius of about ) * metres = radius of effective cross section for a 250 GeV neutrino scattering off a nucleon * metres = 10 zm * metres = 100 zm * 310 zm � ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cosmic Distance Ladder
The cosmic distance ladder (also known as the extragalactic distance scale) is the succession of methods by which astronomers determine the distances to celestial objects. A ''direct'' distance measurement of an astronomical object is possible only for those objects that are "close enough" (within about a thousand parsecs) to Earth. The techniques for determining distances to more distant objects are all based on various measured correlations between methods that work at close distances and methods that work at larger distances. Several methods rely on a standard candle, which is an astronomical object that has a known luminosity. The ladder analogy arises because no single technique can measure distances at all ranges encountered in astronomy. Instead, one method can be used to measure nearby distances, a second can be used to measure nearby to intermediate distances, and so on. Each rung of the ladder provides information that can be used to determine the distances at the ne ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Astronomical Units Of Length
The astronomical system of units, formerly called the IAU (1976) System of Astronomical Constants, is a system of measurement developed for use in astronomy. It was adopted by the International Astronomical Union (IAU) in 1976 via Resolution No. 1, and has been significantly updated in 1994 and 2009 (see astronomical constant). The system was developed because of the difficulties in measuring and expressing astronomical data in International System of Units ( SI units). In particular, there is a huge quantity of very precise data relating to the positions of objects within the Solar System which cannot conveniently be expressed or processed in SI units. Through a number of modifications, the astronomical system of units now explicitly recognizes the consequences of general relativity, which is a necessary addition to the International System of Units in order to accurately treat astronomical data. The astronomical system of units is a tridimensional system, in that it defi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |