|
Resection (orientation)
Position resection and intersection are methods for determining an unknown geographic position ( position finding) by measuring angles with respect to known positions. In ''resection'', the one point with unknown coordinates is occupied and sightings are taken to the known points; in ''intersection'', the two points with known coordinates are occupied and sightings are taken to the unknown point. Measurements can be made with a compass and topographic map (or nautical chart), theodolite or with a total station using known points of a geodetic network or landmarks of a map. Resection versus intersection Resection and its related method, ''intersection'', are used in surveying as well as in general land navigation (including inshore marine navigation using shore-based landmarks). Both methods involve taking azimuths or bearings to two or more objects, then drawing ''lines of position'' along those recorded bearings or azimuths. When intersecting, lines of position are used to fix th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geographic Position
The geographic coordinate system (GCS) is a spherical or ellipsoidal coordinate system for measuring and communicating positions directly on the Earth as latitude and longitude. It is the simplest, oldest and most widely used of the various spatial reference systems that are in use, and forms the basis for most others. Although latitude and longitude form a coordinate tuple like a cartesian coordinate system, the geographic coordinate system is not cartesian because the measurements are angles and are not on a planar surface. A full GCS specification, such as those listed in the EPSG and ISO 19111 standards, also includes a choice of geodetic datum (including an Earth ellipsoid), as different datums will yield different latitude and longitude values for the same location. History The invention of a geographic coordinate system is generally credited to Eratosthenes of Cyrene, who composed his now-lost ''Geography'' at the Library of Alexandria in the 3rd century BC. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
John Collins (mathematician)
John Collins FRS (25 March 1625 – 10 November 1683) was an English mathematician. He is most known for his extensive correspondence with leading scientists and mathematicians such as Giovanni Alfonso Borelli, Gottfried Leibniz, Isaac Newton, and John Wallis. His correspondence provides details of many of the discoveries and developments made in his time, and shows his activity as an 'intelligencer'. Life He was the son of a nonconformist minister, and was born at Wood Eaton in Oxfordshire, 5 March 1625. Apprenticed at the age of sixteen to Thomas Allam, a bookseller, living outside the Turl Gate of Oxford, he was driven to quit the trade by the troubles of the time, and accepted a clerkship in the employment of John Marr, clerk of the kitchen to the Prince of Wales. From him he derived some instruction in mathematics, but the outbreak of the First English Civil War drove him to sea for seven years, 1642-9, most of which time he spent on board an English merchantman, engaged b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orienteering
Orienteering is a group of sports that require navigational skills using a map and compass to navigate from point to point in diverse and usually unfamiliar terrain whilst moving at speed. Participants are given a topographical map, usually a specially prepared orienteering map, which they use to find control points. Originally a training exercise in land navigation for military officers, orienteering has developed many variations. Among these, the oldest and the most popular is foot orienteering. For the purposes of this article, foot orienteering serves as a point of departure for discussion of all other variations, but almost any sport that involves racing against a clock and requires navigation with a map is a type of orienteering. Orienteering is included in the programs of world sporting events including the World Games (see Orienteering at the World Games) and World Police and Fire Games. History The history of orienteering begins in the late 19th century in Swede ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Position Line
A position line or line of position (LOP) is a line (or, on the surface of the earth, a curve) that can be both identified on a chart (nautical chart or aeronautical chart) and translated to the surface of the earth. The intersection of a minimum of two position lines is a fix that is used in position fixing to identify a navigator's location. There are several types of position line: * Compass bearing – the angle between north and the line passing through the compass and the point of interest * Transit – a line passing through the observer and two other reference points * Leading line – the line passing through two marks indicating a safe channel * Leading lights – the line passing through two beacons indicating a safe channel * Sector lights – the lines created by masked colored lights that indicate a safe channel See also * Coordinate line * Intersection (aeronautics) * Navigation * Position circle * Sight reduction In astronavigation, sight reduction is the proce ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orienteering Compass
A compass is a device that shows the cardinal directions used for navigation and geographic orientation. It commonly consists of a magnetized needle or other element, such as a compass card or compass rose, which can pivot to align itself with magnetic north. Other methods may be used, including gyroscopes, magnetometers, and GPS receivers. Compasses often show angles in degrees: north corresponds to 0°, and the angles increase clockwise, so east is 90°, south is 180°, and west is 270°. These numbers allow the compass to show azimuths or bearings which are commonly stated in degrees. If local variation between magnetic north and true north is known, then direction of magnetic north also gives direction of true north. Among the Four Great Inventions, the magnetic compass was first invented as a device for divination as early as the Chinese Han Dynasty (since c. 206 BC),Li Shu-hua, p. 176 and later adopted for navigation by the Song Dynasty Chinese during the 11th century. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Intersection (aeronautics)
In aviation, an intersection is a virtual navigational fix that helps aircraft maintain their flight plan. It is usually defined as the intersection (in the geometrical sense) of two VOR radials. They are usually identified as major airway intersections where aircraft, operating under instrument flight rules, often change direction of flight while en route. According to the Federal Aviation Regulations, some intersections are designated as mandatory reporting points for pilots who are not in radar contact with air traffic control. Intersections also play an important role in departure and approach procedures. All intersections have an alphabetical or alphanumeric designation. Near major airports, the intersection designation code typically consists of three letters followed by the runway number. Most other intersection designations consist of five-letter combinations that are either pronounceable or chosen for their mnemonic value, since either air traffic control or the flight ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hansen's Problem
Hansen's problem is a problem in planar surveying, named after the astronomer Peter Andreas Hansen (1795–1874), who worked on the geodetic survey of Denmark. There are two known points ''A'' and ''B'', and two unknown points ''P''1 and ''P''2. From ''P''1 and ''P''2 an observer measures the angles made by the lines of sight to each of the other three points. The problem is to find the positions of ''P''1 and ''P''2. See figure; the angles measured are (''α''1, ''β''1, ''α''2, ''β''2). Since it involves observations of angles made at unknown points, the problem is an example of Resection (orientation), resection (as opposed to intersection). Solution method overview Define the following angles: ''γ'' = ''P''1''AP''2, ''δ'' = ''P''1''BP''2, ''φ'' = ''P''2''AB'', ''ψ'' = ''P''1''BA''. As a first step we will solve for ''φ'' and ''ψ''. The sum of these two unknown ang ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hand Compass
A hand compass (also hand bearing compass or sighting compass) is a compact magnetic compass capable of one-hand use and fitted with a sighting device to record a precise bearing or azimuth to a given target or to determine a location. Hand or sighting compasses include instruments with simple notch-and-post alignment ("gunsights"), prismatic sights, direct or lensatic sights, and mirror/vee (reflected-image) sights. With the additional precision offered by the sighting arrangement, and depending upon construction, sighting compasses provide increased accuracy when measuring precise bearings to an objective. The term ''hand compass'' is used by some in the forestry and surveying professions to refer to a certain type of hand compass optimized for use in those fields, also known as a forester or cruiser compass. A ''hand compass'' may also include the various one-hand or 'pocket' versions of the surveyor's or geologist's transit. History and use While small portable compass ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projection Variation
Projection, projections or projective may refer to: Physics * Projection (physics), the action/process of light, heat, or sound reflecting from a surface to another in a different direction * The display of images by a projector Optics, graphics, and cartography * Map projection, reducing the surface of a three-dimensional planet to a flat map * Graphical projection, the production of a two-dimensional image of a three-dimensional object Chemistry * Fischer projection, a two-dimensional representation of a three-dimensional organic molecule * Haworth projection, a way of writing a structural formula to represent the cyclic structure of monosaccharides * Natta projection, a way to depict molecules with complete stereochemistry in two dimensions in a skeletal formula * Newman projection, a visual representation of a chemical bond from front to back Mathematics * Projection (mathematics), any of several different types of geometrical mappings ** Projection (linear algebra), a l ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
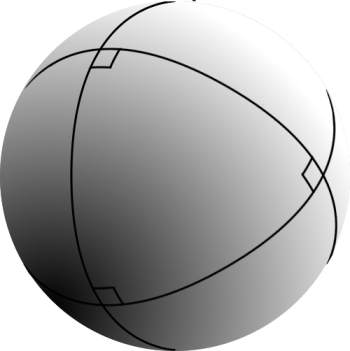
Spherical Excess
Spherical trigonometry is the branch of spherical geometry that deals with the metrical relationships between the sides and angles of spherical triangles, traditionally expressed using trigonometric functions. On the sphere, geodesics are great circles. Spherical trigonometry is of great importance for calculations in astronomy, geodesy, and navigation. The origins of spherical trigonometry in Greek mathematics and the major developments in Islamic mathematics are discussed fully in History of trigonometry and Mathematics in medieval Islam. The subject came to fruition in Early Modern times with important developments by John Napier, Delambre and others, and attained an essentially complete form by the end of the nineteenth century with the publication of Todhunter's textbook ''Spherical trigonometry for the use of colleges and Schools''. Since then, significant developments have been the application of vector methods, quaternion methods, and the use of numerical methods. Pr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geodesy
Geodesy ( ) is the Earth science of accurately measuring and understanding Earth's figure (geometric shape and size), orientation in space, and gravity. The field also incorporates studies of how these properties change over time and equivalent measurements for other planets (known as '' planetary geodesy''). Geodynamical phenomena, including crustal motion, tides and polar motion, can be studied by designing global and national control networks, applying space geodesy and terrestrial geodetic techniques and relying on datums and coordinate systems. The job title is geodesist or geodetic surveyor. History Definition The word geodesy comes from the Ancient Greek word ''geodaisia'' (literally, "division of Earth"). It is primarily concerned with positioning within the temporally varying gravitational field. Geodesy in the German-speaking world is divided into "higher geodesy" ( or ), which is concerned with measuring Earth on the global scale, and "practical geodes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Snellius–Pothenot Problem
The Snellius–Pothenot problem is a problem in planar surveying. Given three known points A, B and C, an observer at an unknown point P observes that the segment AC subtends an angle \alpha and the segment CB subtends an angle \beta; the problem is to determine the position of the point P. (See figure; the point denoted C is between A and B as seen from P). Since it involves the observation of known points from an unknown point, the problem is an example of resection. Historically it was first studied by Snellius, who found a solution around 1615. Formulating the equations First equation Denoting the (unknown) angles ''CAP'' as ''x'' and ''CBP'' as ''y'' we get: :x+y = 2 \pi - \alpha - \beta - C by using the sum of the angles formula for the quadrilateral ''PACB''. The variable ''C'' represents the (known) internal angle in this quadrilateral at point ''C''. (Note that in the case where the points ''C'' and ''P'' are on the same side of the line ''AB'', the angle C will b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |