|
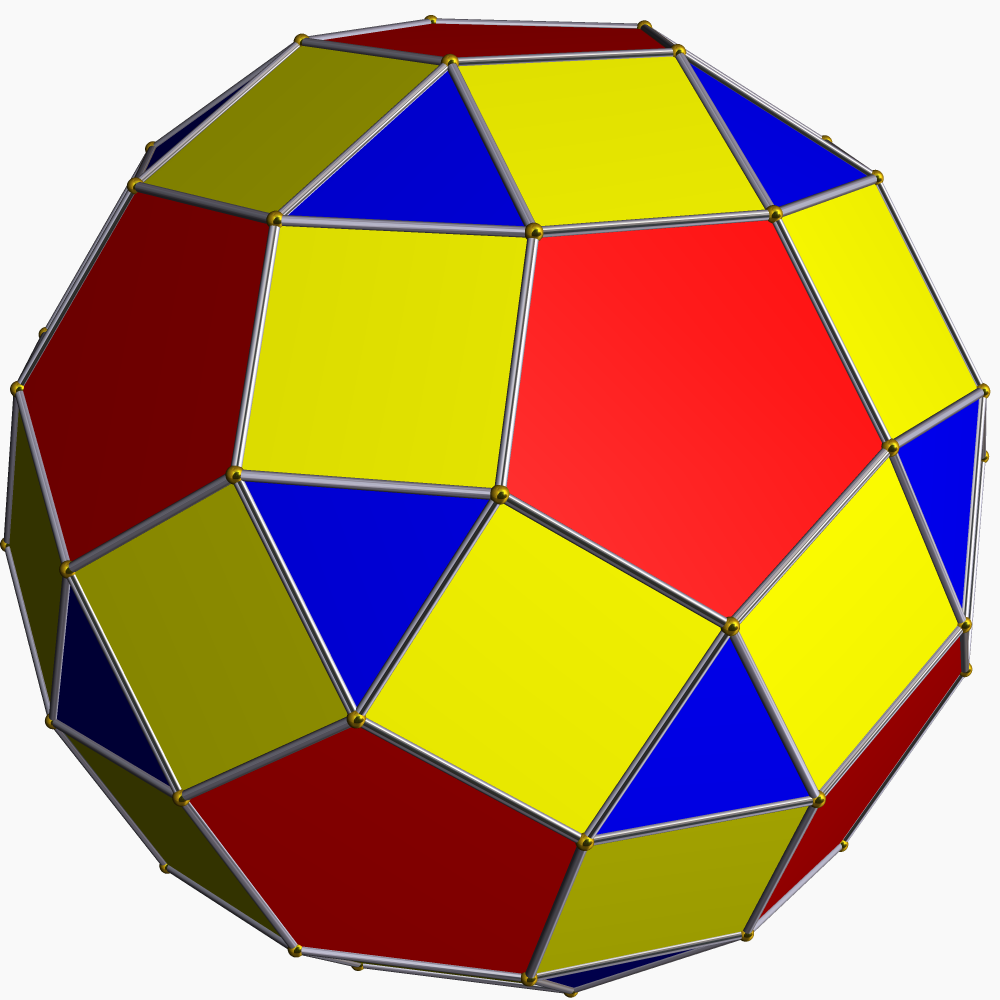
Pseudorhombicuboctahedron
In geometry, the elongated square gyrobicupola or pseudo-rhombicuboctahedron is one of the Johnson solids (). It is not usually considered to be an Archimedean solid, even though its faces consist of regular polygons that meet in the same pattern at each of its vertices, because unlike the 13 Archimedean solids, it lacks a set of global symmetries that map every vertex to every other vertex (though Grünbaum has suggested it should be added to the traditional list of Archimedean solids as a 14th example). It strongly resembles, but should not be mistaken for, the rhombicuboctahedron, which ''is'' an Archimedean solid. It is also a canonical polyhedron. This shape may have been discovered by Johannes Kepler in his enumeration of the Archimedean solids, but its first clear appearance in print appears to be the work of Duncan Sommerville in 1905. It was independently rediscovered by J. C. P. Miller by 1930 (by mistake while attempting to construct a model of the rhombicuboctah ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pseudo-deltoidal Icositetrahedron
The pseudo-deltoidal icositetrahedron is a convex polyhedron with congruent kites as its faces. It is the dual of the elongated square gyrobicupola, also known as the pseudorhombicuboctahedron. As the pseudorhombicuboctahedron is tightly related to the rhombicuboctahedron, but has a twist along an equatorial belt of faces (and edges), the pseudo-deltoidal icositetrahedron is tightly related to the deltoidal icositetrahedron, but has a twist along an equator of (vertices and) edges. Properties Vertices As the faces of the pseudorhombicuboctahedron are regular, the vertices of the pseudo-deltoidal icositetrahedron are regular. But due to the twist, these vertices are of four different kinds: *eight vertices connecting three short edges (yellow vertices in 1st figure below), *two apices connecting four long edges (top and bottom vertices, light red in 1st figure below), *eight vertices connecting four alternate edges: short-long-short-long (dark red vertices in 1st figure below) ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Archimedean Solid
In geometry, an Archimedean solid is one of the 13 solids first enumerated by Archimedes. They are the convex uniform polyhedra composed of regular polygons meeting in identical vertices, excluding the five Platonic solids (which are composed of only one type of polygon), excluding the prisms and antiprisms, and excluding the pseudorhombicuboctahedron. They are a subset of the Johnson solids, whose regular polygonal faces do not need to meet in identical vertices. "Identical vertices" means that each two vertices are symmetric to each other: A global isometry of the entire solid takes one vertex to the other while laying the solid directly on its initial position. observed that a 14th polyhedron, the elongated square gyrobicupola (or pseudo-rhombicuboctahedron), meets a weaker definition of an Archimedean solid, in which "identical vertices" means merely that the faces surrounding each vertex are of the same types (i.e. each vertex looks the same from close up), so only a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Small Rhombicuboctahedron
In geometry, the rhombicuboctahedron, or small rhombicuboctahedron, is a polyhedron with eight triangular, six square, and twelve rectangular faces. There are 24 identical vertices, with one triangle, one square, and two rectangles meeting at each one. If all the rectangles are themselves square (equivalently, all the edges are the same length, ensuring the triangles are equilateral), it is an Archimedean solid. The polyhedron has octahedral symmetry, like the cube and octahedron. Its dual is called the deltoidal icositetrahedron or trapezoidal icositetrahedron, although its faces are not really true trapezoids. Names Johannes Kepler in Harmonices Mundi (1618) named this polyhedron a ''rhombicuboctahedron'', being short for ''truncated cuboctahedral rhombus'', with ''cuboctahedral rhombus'' being his name for a rhombic dodecahedron. There are different truncations of a rhombic dodecahedron into a topological rhombicuboctahedron: Prominently its rectification (left), the one ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rhombicuboctahedron
In geometry, the rhombicuboctahedron, or small rhombicuboctahedron, is a polyhedron with eight triangular, six square, and twelve rectangular faces. There are 24 identical vertices, with one triangle, one square, and two rectangles meeting at each one. If all the rectangles are themselves square (equivalently, all the edges are the same length, ensuring the triangles are equilateral), it is an Archimedean solid. The polyhedron has octahedral symmetry, like the cube and octahedron. Its dual is called the deltoidal icositetrahedron or trapezoidal icositetrahedron, although its faces are not really true trapezoids. Names Johannes Kepler in Harmonices Mundi (1618) named this polyhedron a ''rhombicuboctahedron'', being short for ''truncated cuboctahedral rhombus'', with ''cuboctahedral rhombus'' being his name for a rhombic dodecahedron. There are different truncations of a rhombic dodecahedron into a topological rhombicuboctahedron: Prominently its rectification (left ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Johnson Solid
In geometry, a Johnson solid is a strictly convex polyhedron each face of which is a regular polygon. There is no requirement that each face must be the same polygon, or that the same polygons join around each vertex. An example of a Johnson solid is the square-based pyramid with equilateral sides ( ); it has 1 square face and 4 triangular faces. Some authors require that the solid not be uniform (i.e., not Platonic solid, Archimedean solid, uniform prism, or uniform antiprism) before they refer to it as a “Johnson solid”. As in any strictly convex solid, at least three faces meet at every vertex, and the total of their angles is less than 360 degrees. Since a regular polygon has angles at least 60 degrees, it follows that at most five faces meet at any vertex. The pentagonal pyramid () is an example that has a degree-5 vertex. Although there is no obvious restriction that any given regular polygon cannot be a face of a Johnson solid, it turns out that the face ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pseudorhombicuboctahedron
In geometry, the elongated square gyrobicupola or pseudo-rhombicuboctahedron is one of the Johnson solids (). It is not usually considered to be an Archimedean solid, even though its faces consist of regular polygons that meet in the same pattern at each of its vertices, because unlike the 13 Archimedean solids, it lacks a set of global symmetries that map every vertex to every other vertex (though Grünbaum has suggested it should be added to the traditional list of Archimedean solids as a 14th example). It strongly resembles, but should not be mistaken for, the rhombicuboctahedron, which ''is'' an Archimedean solid. It is also a canonical polyhedron. This shape may have been discovered by Johannes Kepler in his enumeration of the Archimedean solids, but its first clear appearance in print appears to be the work of Duncan Sommerville in 1905. It was independently rediscovered by J. C. P. Miller by 1930 (by mistake while attempting to construct a model of the rhombicuboctah ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vertex-transitive
In geometry, a polytope (e.g. a polygon or polyhedron) or a tiling is isogonal or vertex-transitive if all its vertices are equivalent under the symmetries of the figure. This implies that each vertex is surrounded by the same kinds of face in the same or reverse order, and with the same angles between corresponding faces. Technically, one says that for any two vertices there exists a symmetry of the polytope mapping the first isometrically onto the second. Other ways of saying this are that the group of automorphisms of the polytope '' acts transitively'' on its vertices, or that the vertices lie within a single ''symmetry orbit''. All vertices of a finite -dimensional isogonal figure exist on an -sphere. The term isogonal has long been used for polyhedra. Vertex-transitive is a synonym borrowed from modern ideas such as symmetry groups and graph theory. The pseudorhombicuboctahedronwhich is ''not'' isogonaldemonstrates that simply asserting that "all vertices l ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vertex Figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off. Definitions Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines across the connected faces, joining adjacent points around the face. When done, these lines form a complete circuit, i.e. a polygon, around the vertex. This polygon is the vertex figure. More precise formal definitions can vary quite widely, according to circumstance. For example Coxeter (e.g. 1948, 1954) varies his definition as convenient for the current area of discussion. Most of the following definitions of a vertex figure apply equally well to infinite tilings or, by extension, to space-filling tessellation with polytope cells and other higher-dimensional polytopes. As a flat slice Make a slice through the corner of the polyhedron, cutting through all the edges connected to the vertex. The cut surface is the vertex figure. This i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Johannes Kepler
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws of planetary motion, and his books '' Astronomia nova'', '' Harmonice Mundi'', and '' Epitome Astronomiae Copernicanae''. These works also provided one of the foundations for Newton's theory of universal gravitation. Kepler was a mathematics teacher at a seminary school in Graz, where he became an associate of Prince Hans Ulrich von Eggenberg. Later he became an assistant to the astronomer Tycho Brahe in Prague, and eventually the imperial mathematician to Emperor Rudolf II and his two successors Matthias and Ferdinand II. He also taught mathematics in Linz, and was an adviser to General Wallenstein. Additionally, he did fundamental work in the field of optics, invented an improved version of the refracting (or Keplerian) tele ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gyrate Rhombicosidodecahedron
In geometry, the gyrate rhombicosidodecahedron is one of the Johnson solids (). It is also a canonical polyhedron. Related polyhedron It can be constructed as a rhombicosidodecahedron with one pentagonal cupola rotated through 36 degrees. They have the same faces around each vertex, but vertex configurations along the rotation become a different order, . Alternative Johnson solids, constructed by rotating different cupolae of a rhombicosidodecahedron, are: * The parabigyrate rhombicosidodecahedron () where two opposing cupolae are rotated; * The metabigyrate rhombicosidodecahedron () where two non-opposing cupolae are rotated; * And the trigyrate rhombicosidodecahedron In geometry, the trigyrate rhombicosidodecahedron is one of the Johnson solids (). It contains 20 triangles, 30 squares and 12 pentagons. It is also a canonical polyhedron. It can be constructed as a rhombicosidodecahedron with three pentag ... () where three cupolae are rotated. External links * ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Square Cupola
In geometry, the square cupola, sometimes called lesser dome, is one of the Johnson solids (). It can be obtained as a slice of the rhombicuboctahedron. As in all cupolae, the base polygon has twice as many edges and vertices as the top; in this case the base polygon is an octagon. Formulae The following formulae for the circumradius, surface area, volume, and height can be used if all faces are regular, with edge length ''a'': :C=\left(\frac\sqrt\right)a\approx1.39897a, :A=\left(7+2\sqrt+\sqrt\right)a^2\approx11.56048a^2, :V=\left(1+\frac\right)a^3\approx1.94281a^3. :h = \fraca \approx 0.70711a Related polyhedra and honeycombs Other convex cupolae Dual polyhedron The dual of the square cupola has 8 triangular and 4 kite faces: Crossed square cupola The crossed square cupola is one of the nonconvex Johnson solid isomorphs, being topologically identical to the convex square cupola. It can be obtained as a slice of the nonconvex great rhombicuboctahedron or ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.png)