|
Pyritohedron
In geometry, a dodecahedron (; ) or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagons as faces, which is a Platonic solid. There are also three regular star dodecahedra, which are constructed as stellations of the convex form. All of these have icosahedral symmetry, order 120. Some dodecahedra have the same combinatorial structure as the regular dodecahedron (in terms of the graph formed by its vertices and edges), but their pentagonal faces are not regular: The pyritohedron, a common crystal form in pyrite, has pyritohedral symmetry, while the tetartoid has tetrahedral symmetry. The rhombic dodecahedron can be seen as a limiting case of the pyritohedron, and it has octahedral symmetry. The elongated dodecahedron and trapezo-rhombic dodecahedron variations, along with the rhombic dodecahedra, are space-filling. There are numerous other dodecahedra. While the regular dodecahedron sha ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tetartoid
In geometry, a dodecahedron (; ) or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagons as faces, which is a Platonic solid. There are also three regular star dodecahedra, which are constructed as stellations of the convex form. All of these have icosahedral symmetry, order 120. Some dodecahedra have the same combinatorial structure as the regular dodecahedron (in terms of the graph formed by its vertices and edges), but their pentagonal faces are not regular: The pyritohedron, a common crystal form in pyrite, has pyritohedral symmetry, while the tetartoid has tetrahedral symmetry. The rhombic dodecahedron can be seen as a limiting case of the pyritohedron, and it has octahedral symmetry. The elongated dodecahedron and trapezo-rhombic dodecahedron variations, along with the rhombic dodecahedra, are space-filling. There are numerous other dodecahedra. While the regular dodecahed ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Platonic Solid
In geometry, a Platonic solid is a Convex polytope, convex, regular polyhedron in three-dimensional space, three-dimensional Euclidean space. Being a regular polyhedron means that the face (geometry), faces are congruence (geometry), congruent (identical in shape and size) regular polygons (all angles congruent and all edge (geometry), edges congruent), and the same number of faces meet at each Vertex (geometry), vertex. There are only five such polyhedra: Geometers have studied the Platonic solids for thousands of years. They are named for the ancient Greek philosopher Plato, who hypothesized in one of his dialogues, the ''Timaeus (dialogue), Timaeus'', that the classical elements were made of these regular solids. History The Platonic solids have been known since antiquity. It has been suggested that certain carved stone balls created by the late Neolithic people of Scotland represent these shapes; however, these balls have rounded knobs rather than being polyhedral, the num ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rhombic Dodecahedron
In geometry, the rhombic dodecahedron is a Polyhedron#Convex_polyhedra, convex polyhedron with 12 congruence (geometry), congruent rhombus, rhombic face (geometry), faces. It has 24 edge (geometry), edges, and 14 vertex (geometry), vertices of 2 types. As a Catalan solid, it is the dual polyhedron of the cuboctahedron. As a parallelohedron, the rhombic dodecahedron can be used to Honeycomb (geometry), tesselate its copies in space creating a rhombic dodecahedral honeycomb. There are some variations of the rhombic dodecahedron, one of which is the Bilinski dodecahedron. There are some stellations of the rhombic dodecahedron, one of which is the Escher's solid. The rhombic dodecahedron may also appear in nature (such as in the garnet crystal), the architectural philosophies, practical usages, and toys. As a Catalan solid Metric properties The rhombic dodecahedron is a polyhedron with twelve rhombus, rhombi, each of which long face-diagonal length is exactly \sqrt times the sho ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polyhedron
In geometry, a polyhedron (: polyhedra or polyhedrons; ) is a three-dimensional figure with flat polygonal Face (geometry), faces, straight Edge (geometry), edges and sharp corners or Vertex (geometry), vertices. The term "polyhedron" may refer either to a solid figure or to its boundary surface (mathematics), surface. The terms solid polyhedron and polyhedral surface are commonly used to distinguish the two concepts. Also, the term ''polyhedron'' is often used to refer implicitly to the whole structure (mathematics), structure formed by a solid polyhedron, its polyhedral surface, its faces, its edges, and its vertices. There are many definitions of polyhedron. Nevertheless, the polyhedron is typically understood as a generalization of a two-dimensional polygon and a three-dimensional specialization of a polytope, a more general concept in any number of dimensions. Polyhedra have several general characteristics that include the number of faces, topological classification by Eule ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tetrahedral Symmetry
image:tetrahedron.svg, 150px, A regular tetrahedron, an example of a solid with full tetrahedral symmetry A regular tetrahedron has 12 rotational (or orientation-preserving) symmetries, and a symmetry order of 24 including transformations that combine a reflection and a rotation. The group of all (not necessarily orientation preserving) symmetries is isomorphic to the group S4, the symmetric group of permutations of four objects, since there is exactly one such symmetry for each permutation of the vertices of the tetrahedron. The set of orientation-preserving symmetries forms a group referred to as the alternating group, alternating subgroup A4 of S4. Details Chiral and full (or achiral tetrahedral symmetry and pyritohedral symmetry) are Point groups in three dimensions, discrete point symmetries (or equivalently, List of spherical symmetry groups, symmetries on the sphere). They are among the Crystal system#Overview of point groups by crystal system, crystallographic point gro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pyritohedral Symmetry
150px, A regular tetrahedron, an example of a solid with full tetrahedral symmetry A regular tetrahedron has 12 rotational (or orientation-preserving) symmetries, and a symmetry order of 24 including transformations that combine a reflection and a rotation. The group of all (not necessarily orientation preserving) symmetries is isomorphic to the group S4, the symmetric group of permutations of four objects, since there is exactly one such symmetry for each permutation of the vertices of the tetrahedron. The set of orientation-preserving symmetries forms a group referred to as the alternating subgroup A4 of S4. Details Chiral and full (or achiral tetrahedral symmetry and pyritohedral symmetry) are discrete point symmetries (or equivalently, symmetries on the sphere). They are among the crystallographic point groups of the cubic crystal system. Seen in stereographic projection the edges of the tetrakis hexahedron form 6 circles (or centrally radial lines) in the plane. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Icosahedral Symmetry
In mathematics, and especially in geometry, an object has icosahedral symmetry if it has the same symmetries as a regular icosahedron. Examples of other polyhedra with icosahedral symmetry include the regular dodecahedron (the dual polyhedron, dual of the icosahedron) and the rhombic triacontahedron. Every polyhedron with icosahedral symmetry has 60 Rotational symmetry, rotational (or orientation-preserving) symmetries and 60 orientation-reversing symmetries (that combine a rotation and a Reflection symmetry, reflection), for a total symmetry order of 120. The full symmetry group is the Coxeter group of type . It may be represented by Coxeter notation and Coxeter diagram . The set of rotational symmetries forms a subgroup that is isomorphic to the alternating group on 5 letters. As point group Apart from the two infinite series of prismatic and antiprismatic symmetry, rotational icosahedral symmetry or chiral icosahedral symmetry of chiral objects and full icosahedra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
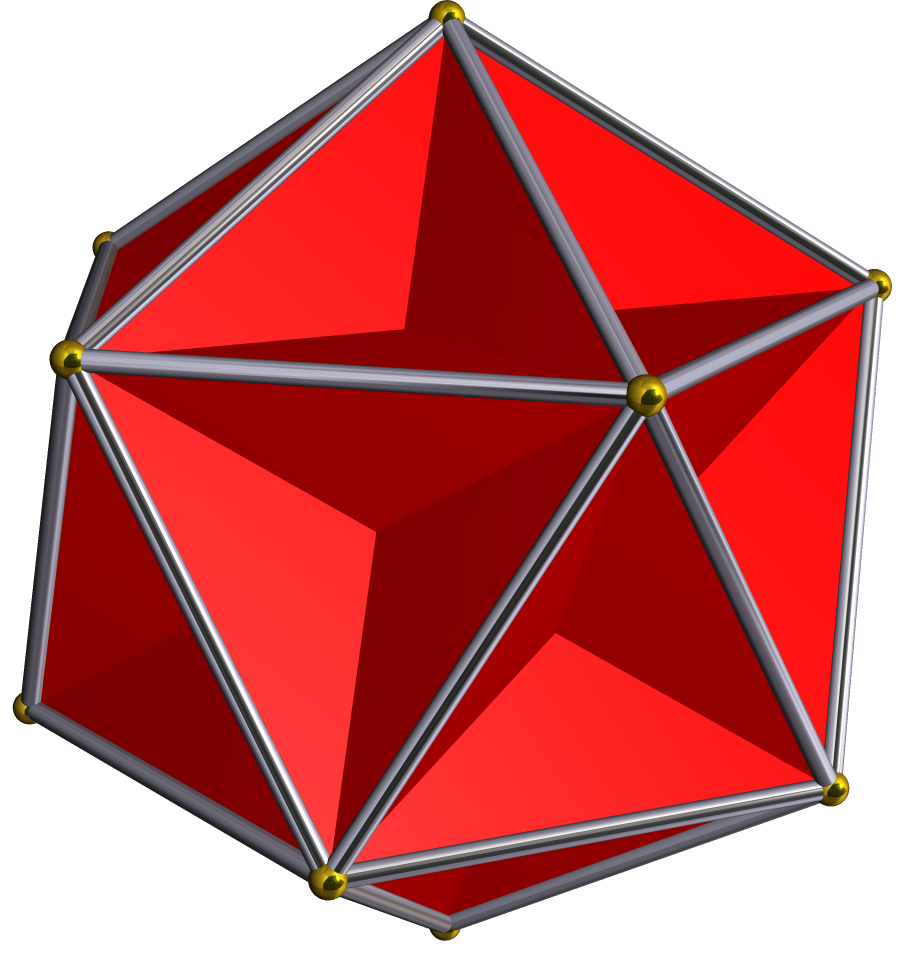
Kepler–Poinsot Polyhedron
In geometry, a Kepler–Poinsot polyhedron is any of four Regular polyhedron, regular Star polyhedron, star polyhedra. They may be obtained by stellation, stellating the regular Convex polyhedron, convex dodecahedron and icosahedron, and differ from these in having regular pentagrammic face (geometry), faces or vertex figures. They can all be seen as three-dimensional analogues of the pentagram in one way or another. Characteristics Sizes The great icosahedron edge length is \phi^4 = \tfrac12\bigl(7+3\sqrt5\,\bigr) times the original icosahedron edge length. The small stellated dodecahedron, great dodecahedron, and great stellated dodecahedron edge lengths are respectively \phi^3 = 2+\sqrt5, \phi^2 = \tfrac12\bigl(3+\sqrt5\,\bigr), and \phi^5 = \tfrac12\bigl(11+5\sqrt5\,\bigr) times the original dodecahedron edge length. Non-convexity These figures have pentagrams (star pentagons) as faces or vertex figures. The small stellated dodecahedron, small and great stellated dodec ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
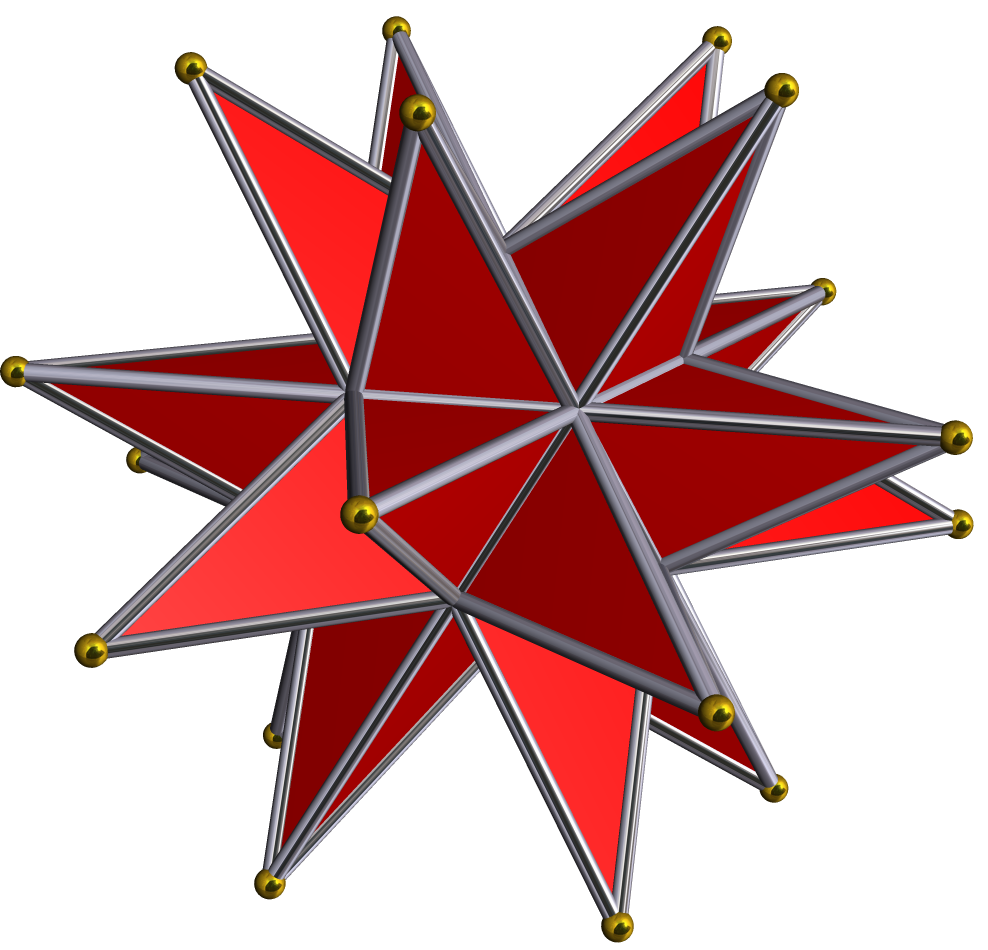
Stellation
In geometry, stellation is the process of extending a polygon in two dimensions, a polyhedron in three dimensions, or, in general, a polytope in ''n'' dimensions to form a new figure. Starting with an original figure, the process extends specific elements such as its edges or face planes, usually in a symmetrical way, until they meet each other again to form the closed boundary of a new figure. The new figure is a stellation of the original. The word ''stellation'' comes from the Latin ''stellātus'', "starred", which in turn comes from the Latin ''stella'', "star". Stellation is the reciprocal or dual process to '' faceting''. Kepler's definition In 1619 Kepler defined stellation for polygons and polyhedra as the process of extending edges or faces until they meet to form a new polygon or polyhedron. He stellated the regular dodecahedron to obtain two regular star polyhedra, the small stellated dodecahedron and the great stellated dodecahedron. He also stellated the regular oct ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
