|
Pseudo-uniform Polyhedron
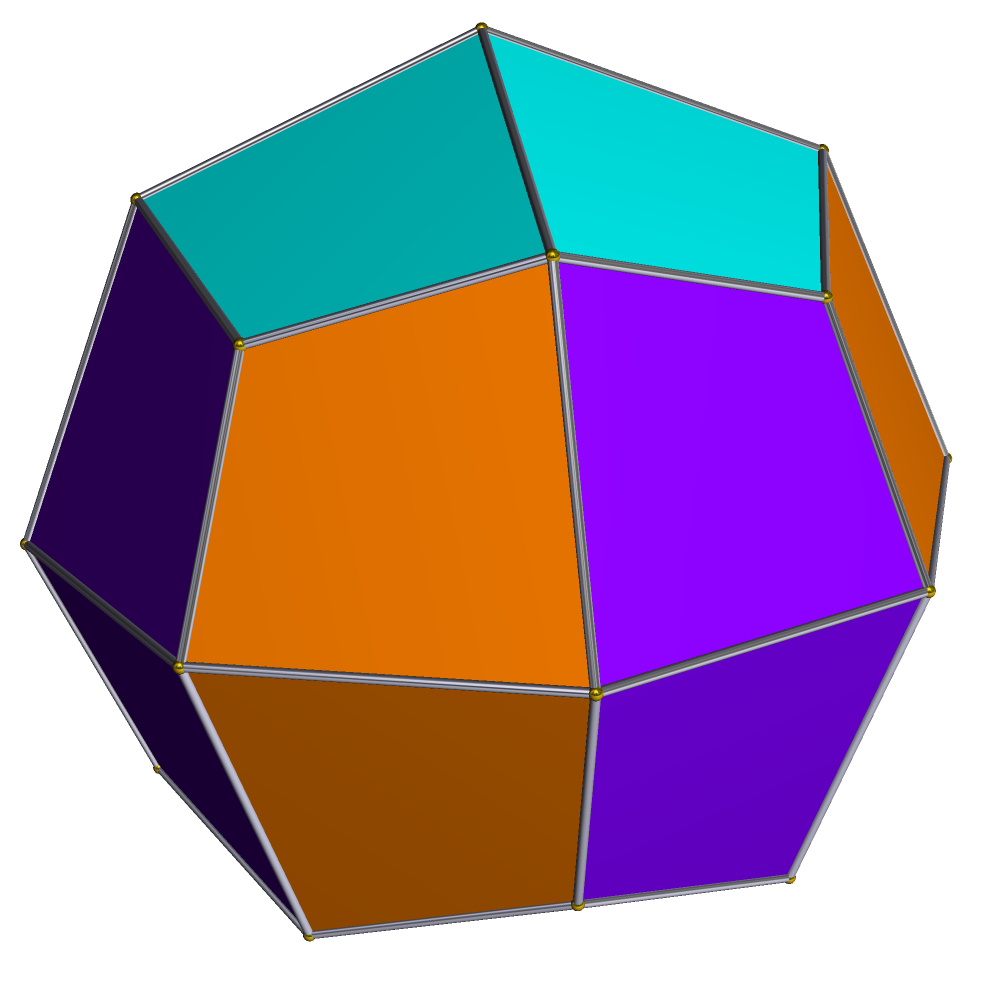
A pseudo-uniform polyhedron is a polyhedron which has regular polygons as faces and has the same vertex configuration at all vertices but is not vertex-transitive: it is not true that for any two vertices, there exists a symmetry of the polyhedron mapping the first isometrically onto the second. Thus, although all the vertices of a pseudo-uniform polyhedron appear the same, it is not isogonal. They are called pseudo-uniform polyhedra due to their resemblance to some true uniform polyhedra. There are two known pseudo-uniform polyhedra: the pseudorhombicuboctahedron and the pseudo-great rhombicuboctahedron. It is not known if there are any others; Branko Grünbaum conjectured that there are not, but thought that a proof would be "probably quite complicated".. Reprinted in . They both have D4d symmetry, the same symmetry as a square antiprism. They can both be constructed from a uniform polyhedron by twisting one cupola-shaped cap. The pseudo-uniform polyhedra Pseudorhombicuboct ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Uniform Polyhedron
In geometry, a uniform polyhedron has regular polygons as Face (geometry), faces and is vertex-transitive—there is an isometry mapping any vertex onto any other. It follows that all vertices are congruence (geometry), congruent. Uniform polyhedra may be Regular polyhedron, regular (if also Isohedral figure, face- and Isotoxal figure, edge-transitive), Quasiregular polyhedron, quasi-regular (if also edge-transitive but not face-transitive), or Semiregular polyhedron, semi-regular (if neither edge- nor face-transitive). The faces and vertices don't need to be Convex polyhedron, convex, so many of the uniform polyhedra are also Star polyhedron, star polyhedra. There are two infinite classes of uniform polyhedra, together with 75 other polyhedra. They are 2 infinite classes of Prism (geometry), prisms and antiprisms, the convex polyhedrons as in 5 Platonic solids and 13 Archimedean solids—2 Quasiregular polyhedron, quasiregular and 11 Semiregular polyhedron, semiregular&m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Elongated Square Gyrobicupola
In geometry, the elongated square gyrobicupola is a polyhedron constructed by two square cupolas attaching onto the bases of octagonal prism, with one of them rotated. It is a Midsphere#Canonical polyhedron, canonical polyhedron. It is not considered to be an Archimedean solid because it lacks a set of global symmetries that map every vertex to every other vertex, unlike the 13 Archimedean solids. However, it was once mistakenly considered a rhombicuboctahedron by many mathematicians. For this reason, it is also known as the pseudo-rhombicuboctahedron, Miller solid, or Miller–Askinuze solid. Construction The elongated square gyrobicupola can be constructed similarly to the rhombicuboctahedron, by attaching two regular square cupolas onto the bases of an octagonal prism, a process known as Elongation (geometry), elongation. The difference between these two polyhedrons is that one of the two square cupolas is twisted by 45 degrees, a process known as ''gyration'', makin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Johnson Solid 37
Johnson may refer to: People and fictional characters *Johnson (surname), a common surname in English *Johnson (given name), a list of people * List of people with surname Johnson, including fictional characters *Johnson (composer) (1953–2011), Indian film score composer *Johnson (rapper) (born 1979), Danish rapper * Mr. Johnson (born 1966), Nigerian singer Places * Mount Johnson (other) Canada * Johnson, Ontario, township * Johnson (electoral district), provincial electoral district in Quebec * Johnson Point (British Columbia), a headland on the north side of the entrance to Belize Inlet United States * Johnson, Arizona * Johnson, Arkansas, a town * Johnson, Delaware * Johnson, Indiana, an unincorporated town * Johnson, Kentucky * Johnson, Minnesota * Johnson, Nebraska * Johnson, New York * Johnson, Ohio, an unincorporated community * Johnson, Oklahoma * Johnson, Utah * Johnson, Vermont, a town ** Johnson (village), Vermont * Johnson, Washington * Johnson, Wisc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Polyhedral Net
In geometry, a net of a polyhedron is an arrangement of non-overlapping edge-joined polygons in the plane which can be folded (along edges) to become the faces of the polyhedron. Polyhedral nets are a useful aid to the study of polyhedra and solid geometry in general, as they allow for physical models of polyhedra to be constructed from material such as thin cardboard. An early instance of polyhedral nets appears in the works of Albrecht Dürer, whose 1525 book ''A Course in the Art of Measurement with Compass and Ruler'' (''Unterweysung der Messung mit dem Zyrkel und Rychtscheyd '') included nets for the Platonic solids and several of the Archimedean solids. These constructions were first called nets in 1543 by Augustin Hirschvogel. Existence and uniqueness Many different nets can exist for a given polyhedron, depending on the choices of which edges are joined and which are separated. The edges that are cut from a convex polyhedron to form a net must form a spanning tree of th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Johnson Solid 37 Net
Johnson may refer to: People and fictional characters *Johnson (surname), a common surname in English *Johnson (given name), a list of people * List of people with surname Johnson, including fictional characters *Johnson (composer) (1953–2011), Indian film score composer *Johnson (rapper) (born 1979), Danish rapper * Mr. Johnson (born 1966), Nigerian singer Places * Mount Johnson (other) Canada * Johnson, Ontario, township * Johnson (electoral district), provincial electoral district in Quebec * Johnson Point (British Columbia), a headland on the north side of the entrance to Belize Inlet United States * Johnson, Arizona * Johnson, Arkansas, a town * Johnson, Delaware * Johnson, Indiana, an unincorporated town * Johnson, Kentucky * Johnson, Minnesota * Johnson, Nebraska * Johnson, New York * Johnson, Ohio, an unincorporated community * Johnson, Oklahoma * Johnson, Utah * Johnson, Vermont, a town ** Johnson (village), Vermont * Johnson, Washington * Johnson, Wisc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Dual Polyhedron
In geometry, every polyhedron is associated with a second dual structure, where the vertices of one correspond to the faces of the other, and the edges between pairs of vertices of one correspond to the edges between pairs of faces of the other. Such dual figures remain combinatorial or abstract polyhedra, but not all can also be constructed as geometric polyhedra. Starting with any given polyhedron, the dual of its dual is the original polyhedron. Duality preserves the symmetries of a polyhedron. Therefore, for many classes of polyhedra defined by their symmetries, the duals belong to a corresponding symmetry class. For example, the regular polyhedrathe (convex) Platonic solids and (star) Kepler–Poinsot polyhedraform dual pairs, where the regular tetrahedron is self-dual. The dual of an isogonal polyhedron (one in which any two vertices are equivalent under symmetries of the polyhedron) is an isohedral polyhedron (one in which any two faces are equivalent .., and vice ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Rhombicuboctahedron
In geometry, the rhombicuboctahedron is an Archimedean solid with 26 faces, consisting of 8 equilateral triangles and 18 squares. It was named by Johannes Kepler in his 1618 Harmonices Mundi, being short for ''truncated cuboctahedral rhombus'', with cuboctahedral rhombus being his name for a rhombic dodecahedron. The rhombicuboctahedron is an Archimedean solid, and its dual is a Catalan solid, the deltoidal icositetrahedron. The elongated square gyrobicupola is a polyhedron that is similar to a rhombicuboctahedron, but it is not an Archimedean solid because it is not vertex-transitive. The rhombicuboctahedron is found in diverse cultures in architecture, toys, the arts, and elsewhere. Construction The rhombicuboctahedron may be constructed from a cube by drawing a smaller one in the middle of each face, parallel to the cube's edges. After removing the edges of a cube, the squares may be joined by adding more squares adjacent between them, and the corners may be filled by th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Square Cupola
In geometry, the square cupola (sometimes called lesser dome) is a cupola with an octagonal In geometry, an octagon () is an eight-sided polygon or 8-gon. A '' regular octagon'' has Schläfli symbol and can also be constructed as a quasiregular truncated square, t, which alternates two types of edges. A truncated octagon, t is a hex ... base. In the case of all edges being equal in length, it is a Johnson solid, a Convex set, convex polyhedron with Regular polygon, regular faces. It can be used to construct many other polyhedrons, particularly other Johnson solids. Properties The square cupola has 4 triangles, 5 squares, and 1 octagon as their faces; the octagon is the base, and one of the squares is the top. If the edges are equal in length, the triangles and octagon become Regular polygon, regular, and the edge length of the octagon is equal to the edge length of both triangles and squares. The dihedral angle between both square and triangle is approximately 144.7^\cir ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Pseudorhombicuboctahedron
In geometry, the elongated square gyrobicupola is a polyhedron constructed by two square cupolas attaching onto the bases of octagonal prism, with one of them rotated. It is a canonical polyhedron. It is not considered to be an Archimedean solid because it lacks a set of global symmetries that map every vertex to every other vertex, unlike the 13 Archimedean solids. However, it was once mistakenly considered a rhombicuboctahedron by many mathematicians. For this reason, it is also known as the pseudo-rhombicuboctahedron, Miller solid, or Miller–Askinuze solid. Construction The elongated square gyrobicupola can be constructed similarly to the rhombicuboctahedron, by attaching two regular square cupolas onto the bases of an octagonal prism, a process known as elongation. The difference between these two polyhedrons is that one of the two square cupolas is twisted by 45 degrees, a process known as ''gyration'', making the triangular faces staggered vertically. The res ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Exploded Rhombicuboctahedron
An explosion is a rapid expansion in volume of a given amount of matter associated with an extreme outward release of energy, usually with the generation of high temperatures and release of high-pressure gases. Explosions may also be generated by a slower expansion that would normally not be forceful, but is not allowed to expand, so that when whatever is containing the expansion is broken by the pressure that builds as the matter inside tries to expand, the matter expands forcefully. An example of this is a volcanic eruption created by the expansion of magma in a magma chamber as it rises to the surface. Supersonic explosions created by high explosives are known as detonations and travel through shock waves. Subsonic explosions are created by low explosives through a slower combustion process known as deflagration. Causes For an explosion to occur, there must be a rapid, forceful expansion of matter. There are numerous ways this can happen, both naturally and artificially, s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Small Rhombicuboctahedron
In geometry, the rhombicuboctahedron is an Archimedean solid with 26 faces, consisting of 8 equilateral triangles and 18 squares. It was named by Johannes Kepler in his 1618 Harmonices Mundi, being short for ''truncated cuboctahedral rhombus'', with cuboctahedral rhombus being his name for a rhombic dodecahedron. The rhombicuboctahedron is an Archimedean solid, and its dual is a Catalan solid, the deltoidal icositetrahedron. The elongated square gyrobicupola is a polyhedron that is similar to a rhombicuboctahedron, but it is not an Archimedean solid because it is not vertex-transitive. The rhombicuboctahedron is found in diverse cultures in architecture, toys, the arts, and elsewhere. Construction The rhombicuboctahedron may be constructed from a cube by drawing a smaller one in the middle of each face, parallel to the cube's edges. After removing the edges of a cube, the squares may be joined by adding more squares adjacent between them, and the corners may be filled by th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Archimedean Solid
The Archimedean solids are a set of thirteen convex polyhedra whose faces are regular polygon and are vertex-transitive, although they aren't face-transitive. The solids were named after Archimedes, although he did not claim credit for them. They belong to the class of uniform polyhedra, the polyhedra with regular faces and symmetric vertices. Some Archimedean solids were portrayed in the works of artists and mathematicians during the Renaissance. The elongated square gyrobicupola or ' is an extra polyhedron with regular faces and congruent vertices, but it is not generally counted as an Archimedean solid because it is not vertex-transitive. The solids The Archimedean solids have a single vertex configuration and highly symmetric properties. A vertex configuration indicates which regular polygons meet at each vertex. For instance, the configuration 3 \cdot 5 \cdot 3 \cdot 5 indicates a polyhedron in which each vertex is met by alternating two triangles and two pentagons. Highl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |