|
Polynomial System Of Equations
A system of polynomial equations (sometimes simply a polynomial system) is a set of simultaneous equations where the are polynomials in several variables, say , over some field . A ''solution'' of a polynomial system is a set of values for the s which belong to some algebraically closed field extension of , and make all equations true. When is the field of rational numbers, is generally assumed to be the field of complex numbers, because each solution belongs to a field extension of , which is isomorphic to a subfield of the complex numbers. This article is about the methods for solving, that is, finding all solutions or describing them. As these methods are designed for being implemented in a computer, emphasis is given on fields in which computation (including equality testing) is easy and efficient, that is the field of rational numbers and finite fields. Searching for solutions that belong to a specific set is a problem which is generally much more difficult, and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Simultaneous Equations
In mathematics, a set of simultaneous equations, also known as a system of equations or an equation system, is a finite set of equations for which common solutions are sought. An equation system is usually classified in the same manner as single equations, namely as a: * System of linear equations, * System of nonlinear equations, * System of bilinear equations, * System of polynomial equations, * System of differential equations, or a * System of difference equations See also * Simultaneous equations model, a statistical model in the form of simultaneous linear equations * Elementary algebra Elementary algebra encompasses the basic concepts of algebra. It is often contrasted with arithmetic: arithmetic deals with specified numbers, whilst algebra introduces variables (quantities without fixed values). This use of variables entai ..., for elementary methods {{set index article Equations Broad-concept articles de:Gleichung#Gleichungssysteme ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
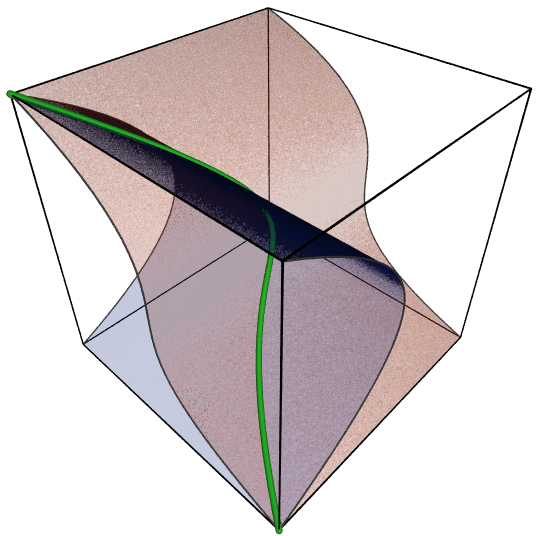
Barth Surface
__NOTOC__ In algebraic geometry, a Barth surface is one of the complex nodal surfaces in 3 dimensions with large numbers of double points found by . Two examples are the Barth sextic of degree 6 with 65 double points, and the Barth decic of degree 10 with 345 double points. For degree 6 surfaces in P3, showed that 65 is the maximum number of double points possible. The Barth sextic is a counterexample to an incorrect claim by Francesco Severi in 1946 that 52 is the maximum number of double points possible. Informal accounting of the 65 ordinary double points of the Barth Sextic The Barth Sextic may be visualized in three dimensions as featuring 50 finite and 15 infinite ordinary double points (nodes). Referring to the figure, the 50 finite ordinary double points are arrayed as the vertices of 20 roughly tetrahedral shapes oriented such that the bases of these four-sided "outward pointing" shapes form the triangular faces of a regular icosidodecahedron. To these 30 icosidodeca ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dimension Of An Algebraic Variety
In mathematics and specifically in algebraic geometry, the dimension of an algebraic variety may be defined in various equivalent ways. Some of these definitions are of geometric nature, while some other are purely algebraic and rely on commutative algebra. Some are restricted to algebraic varieties while others apply also to any algebraic set. Some are intrinsic, as independent of any embedding of the variety into an affine or projective space, while other are related to such an embedding. Dimension of an affine algebraic set Let be a field, and be an algebraically closed extension. An affine algebraic set is the set of the common zeros in of the elements of an ideal in a polynomial ring R=K _1, \ldots, x_n Let A=R/I be the algebra of the polynomial functions over . The dimension of is any of the following integers. It does not change if is enlarged, if is replaced by another algebraically closed extension of and if is replaced by another ideal having the same zer ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebraic Variety
Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex numbers. Modern definitions generalize this concept in several different ways, while attempting to preserve the geometric intuition behind the original definition. Conventions regarding the definition of an algebraic variety differ slightly. For example, some definitions require an algebraic variety to be irreducible, which means that it is not the union of two smaller sets that are closed in the Zariski topology. Under this definition, non-irreducible algebraic varieties are called algebraic sets. Other conventions do not require irreducibility. The fundamental theorem of algebra establishes a link between algebra and geometry by showing that a monic polynomial (an algebraic object) in one variable with complex number coefficients is determined ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Zero-dimensional Space
In mathematics, a zero-dimensional topological space (or nildimensional space) is a topological space that has dimension zero with respect to one of several inequivalent notions of assigning a dimension to a given topological space. A graphical illustration of a nildimensional space is a point. Definition Specifically: * A topological space is zero-dimensional with respect to the Lebesgue covering dimension if every open cover of the space has a refinement which is a cover by disjoint open sets. * A topological space is zero-dimensional with respect to the finite-to-finite covering dimension if every finite open cover of the space has a refinement that is a finite open cover such that any point in the space is contained in exactly one open set of this refinement. * A topological space is zero-dimensional with respect to the small inductive dimension if it has a base consisting of clopen sets. The three notions above agree for separable, metrisable spaces. Properties of spaces ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Krull's Principal Ideal Theorem
In commutative algebra, Krull's principal ideal theorem, named after Wolfgang Krull (1899–1971), gives a bound on the height of a principal ideal in a commutative Noetherian ring. The theorem is sometimes referred to by its German name, ''Krulls Hauptidealsatz'' ('' Satz'' meaning "proposition" or "theorem"). Precisely, if ''R'' is a Noetherian ring and ''I'' is a principal, proper ideal of ''R'', then each minimal prime ideal over ''I'' has height at most one. This theorem can be generalized to ideals that are not principal, and the result is often called Krull's height theorem. This says that if ''R'' is a Noetherian ring and ''I'' is a proper ideal generated by ''n'' elements of ''R'', then each minimal prime over ''I'' has height at most ''n''. The converse is also true: if a prime ideal has height ''n'', then it is a minimal prime ideal over an ideal generated by ''n'' elements. The principal ideal theorem and the generalization, the height theorem, both follow from the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commutative Algebra
Commutative algebra, first known as ideal theory, is the branch of algebra that studies commutative rings, their ideals, and modules over such rings. Both algebraic geometry and algebraic number theory build on commutative algebra. Prominent examples of commutative rings include polynomial rings; rings of algebraic integers, including the ordinary integers \mathbb; and ''p''-adic integers. Commutative algebra is the main technical tool in the local study of schemes. The study of rings that are not necessarily commutative is known as noncommutative algebra; it includes ring theory, representation theory, and the theory of Banach algebras. Overview Commutative algebra is essentially the study of the rings occurring in algebraic number theory and algebraic geometry. In algebraic number theory, the rings of algebraic integers are Dedekind rings, which constitute therefore an important class of commutative rings. Considerations related to modular arithmetic have led to the no ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Underdetermined System
In mathematics, a system of linear equations or a system of polynomial equations is considered underdetermined if there are fewer equations than unknowns (in contrast to an overdetermined system, where there are more equations than unknowns). The terminology can be explained using the concept of constraint counting. Each unknown can be seen as an available degree of freedom. Each equation introduced into the system can be viewed as a constraint that restricts one degree of freedom. Therefore, the critical case (between overdetermined and underdetermined) occurs when the number of equations and the number of free variables are equal. For every variable giving a degree of freedom, there exists a corresponding constraint removing a degree of freedom. The underdetermined case, by contrast, occurs when the system has been underconstrained—that is, when the unknowns outnumber the equations. Solutions of underdetermined systems An underdetermined linear system has either no sol ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hilbert's Nullstellensatz
In mathematics, Hilbert's Nullstellensatz (German for "theorem of zeros," or more literally, "zero-locus-theorem") is a theorem that establishes a fundamental relationship between geometry and algebra. This relationship is the basis of algebraic geometry. It relates algebraic sets to ideals in polynomial rings over algebraically closed fields. This relationship was discovered by David Hilbert, who proved the Nullstellensatz in his second major paper on invariant theory in 1893 (following his seminal 1890 paper in which he proved Hilbert's basis theorem). Formulation Let ''k'' be a field (such as the rational numbers) and ''K'' be an algebraically closed field extension (such as the complex numbers). Consider the polynomial ring k _1, \ldots, X_n/math> and let ''I'' be an ideal in this ring. The algebraic set V(''I'') defined by this ideal consists of all ''n''-tuples x = (''x''1,...,''x''''n'') in ''Kn'' such that ''f''(x) = 0 for all ''f'' in ''I''. Hilbert's Nullstellensatz st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Inconsistent Equations
In mathematics and particularly in algebra, a system of equations (either linear or nonlinear) is called consistent if there is at least one set of values for the unknowns that satisfies each equation in the system—that is, when substituted into each of the equations, they make each equation hold true as an identity. In contrast, a linear or non linear equation system is called inconsistent if there is no set of values for the unknowns that satisfies all of the equations. If a system of equations is inconsistent, then it is possible to manipulate and combine the equations in such a way as to obtain contradictory information, such as , or x^3 + y^5 = 5 and x^3 + y^3 = 6 (which implies ). Both types of equation system, consistent and inconsistent, can be any of overdetermined (having more equations than unknowns), underdetermined (having fewer equations than unknowns), or exactly determined. Simple examples Underdetermined and consistent The system :\begin x+y+z &= 3, \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Overdetermined System
In mathematics, a system of equations is considered overdetermined if there are more equations than unknowns. An overdetermined system is almost always inconsistent (it has no solution) when constructed with random coefficients. However, an overdetermined system will have solutions in some cases, for example if some equation occurs several times in the system, or if some equations are linear combinations of the others. The terminology can be described in terms of the concept of constraint counting. Each unknown can be seen as an available degree of freedom. Each equation introduced into the system can be viewed as a constraint that restricts one degree of freedom. Therefore, the critical case occurs when the number of equations and the number of free variables are equal. For every variable giving a degree of freedom, there exists a corresponding constraint. The ''overdetermined'' case occurs when the system has been overconstrained — that is, when the equations outnumb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |