|
Moment Distribution Method
The moment distribution method is a structural analysis method for statically indeterminate beams and frames developed by Hardy Cross. It was published in 1930 in an ASCE journal. The method only accounts for flexural effects and ignores axial and shear effects. From the 1930s until computers began to be widely used in the design and analysis of structures, the moment distribution method was the most widely practiced method. Introduction In the moment distribution method, every joint of the structure to be analysed is fixed so as to develop the ''fixed-end moments''. Then each fixed joint is sequentially released and the fixed-end moments (which by the time of release are not in equilibrium) are distributed to adjacent members until equilibrium is achieved. The moment distribution method in mathematical terms can be demonstrated as the process of solving a set of simultaneous equations by means of iteration. The moment distribution method falls into the category of displacem ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Structural Analysis
Structural analysis is a branch of solid mechanics which uses simplified models for solids like bars, beams and shells for engineering decision making. Its main objective is to determine the effect of loads on physical structures and their components. In contrast to theory of elasticity, the models used in structural analysis are often differential equations in one spatial variable. Structures subject to this type of analysis include all that must withstand loads, such as buildings, bridges, aircraft and ships. Structural analysis uses ideas from applied mechanics, materials science and applied mathematics to compute a structure's deformations, internal forces, stresses, support reactions, velocity, accelerations, and stability. The results of the analysis are used to verify a structure's fitness for use, often precluding physical tests. Structural analysis is thus a key part of the engineering design of structures. [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Bending Stiffness
The bending stiffness (K) is the resistance of a member against bending deflection/deformation. It is a function of the Young's modulus E, the second moment of area I of the beam cross-section about the axis of interest, length of the beam and beam boundary condition. Bending stiffness of a beam can analytically be derived from the equation of beam deflection when it is applied by a force. :K = \frac where \mathrm is the applied force and \mathrm is the deflection. According to elementary beam theory, the relationship between the applied bending moment M and the resulting curvature \kappa of the beam is: :M = E I \kappa \approx E I \frac where w is the deflection of the beam and x is the distance along the beam. Double integration of the above equation leads to computing the deflection of the beam, and in turn, the bending stiffness of the beam. Bending stiffness in beams is also known as Flexural rigidity. See also * Applied mechanics * Beam theory * Bending *Stiffness ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
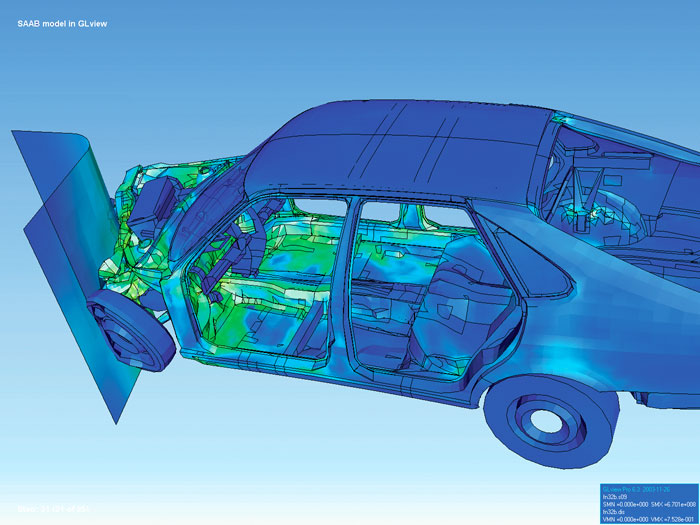
Finite Element Method
Finite element method (FEM) is a popular method for numerically solving differential equations arising in engineering and mathematical modeling. Typical problem areas of interest include the traditional fields of structural analysis, heat transfer, fluid flow, mass transport, and electromagnetic potential. Computers are usually used to perform the calculations required. With high-speed supercomputers, better solutions can be achieved and are often required to solve the largest and most complex problems. FEM is a general numerical method for solving partial differential equations in two- or three-space variables (i.e., some boundary value problems). There are also studies about using FEM to solve high-dimensional problems. To solve a problem, FEM subdivides a large system into smaller, simpler parts called finite elements. This is achieved by a particular space discretization in the space dimensions, which is implemented by the construction of a mesh of the object: the numer ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Direct Stiffness Method
In structural engineering, the direct stiffness method, also known as the matrix stiffness method, is a structural analysis technique particularly suited for computer-automated analysis of complex structures including the statically indeterminate type. It is a matrix method that makes use of the members' stiffness relations for computing member forces and displacements in structures. The direct stiffness method is the most common implementation of the finite element method (FEM). In applying the method, the system must be modeled as a set of simpler, idealized elements interconnected at the nodes. The material stiffness properties of these elements are then, through linear algebra, compiled into a single matrix equation which governs the behaviour of the entire idealized structure. The structure’s unknown displacements and forces can then be determined by solving this equation. The direct stiffness method forms the basis for most commercial and free source finite element so ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Matrix Method
Matrix (: matrices or matrixes) or MATRIX may refer to: Science and mathematics * Matrix (mathematics), a rectangular array of numbers, symbols or expressions * Matrix (logic), part of a formula in prenex normal form * Matrix (biology), the material in between a eukaryotic organism's cells * Matrix (chemical analysis), the non-analyte components of a sample * Matrix (geology), the fine-grained material in which larger objects are embedded * Matrix (composite), the constituent of a composite material * Hair matrix, produces hair * Nail matrix, part of the nail in anatomy Technology * Matrix (mass spectrometry), a compound that promotes the formation of ions * Matrix (numismatics), a tool used in coin manufacturing * Matrix (printing), a mould for casting letters * Matrix (protocol), an open standard for real-time communication * Matrix (record production), or master, a disc used in the production of phonograph records ** Matrix number, of a gramophone record * Diode matrix ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Repeating Decimal
A repeating decimal or recurring decimal is a decimal representation of a number whose digits are eventually periodic (that is, after some place, the same sequence of digits is repeated forever); if this sequence consists only of zeros (that is if there is only a finite number of nonzero digits), the decimal is said to be ''terminating'', and is not considered as repeating. It can be shown that a number is rational if and only if its decimal representation is repeating or terminating. For example, the decimal representation of becomes periodic just after the decimal point, repeating the single digit "3" forever, i.e. 0.333.... A more complicated example is , whose decimal becomes periodic at the ''second'' digit following the decimal point and then repeats the sequence "144" forever, i.e. 5.8144144144.... Another example of this is , which becomes periodic after the decimal point, repeating the 13-digit pattern "1886792452830" forever, i.e. 11.18867924528301886792452830.... ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Span (architecture)
In engineering, span is the distance between two adjacent structural supports (e.g., two piers) of a structural member (e.g., a beam). Span is measured in the horizontal direction either between the faces of the supports (clear span) or between the centers of the bearing surfaces (effective span): A span can be closed by a solid beam or by a rope. The first kind is used for bridges, the second one for power lines, overhead telecommunication lines, some type of antennas or for aerial tramways. Span is a significant factor in finding the strength and size of a beam as it determines the maximum bending moment and deflection. The maximum bending moment M_ and deflection \delta_in the pictured beam is found using: :M_ = \frac :\delta_ = \frac = \frac where :q = Uniformly distributed load :L = Length of the beam between two supports (span) :E = Modulus of elasticity :I = Area moment of inertia The maximum bending moment and deflection occur midway between the two ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Ratio
In mathematics, a ratio () shows how many times one number contains another. For example, if there are eight oranges and six lemons in a bowl of fruit, then the ratio of oranges to lemons is eight to six (that is, 8:6, which is equivalent to the ratio 4:3). Similarly, the ratio of lemons to oranges is 6:8 (or 3:4) and the ratio of oranges to the total amount of fruit is 8:14 (or 4:7). The numbers in a ratio may be quantities of any kind, such as counts of people or objects, or such as measurements of lengths, weights, time, etc. In most contexts, both numbers are restricted to be Positive integer, positive. A ratio may be specified either by giving both constituting numbers, written as "''a'' to ''b''" or "''a'':''b''", or by giving just the value of their quotient Equal quotients correspond to equal ratios. A statement expressing the equality of two ratios is called a ''proportion''. Consequently, a ratio may be considered as an ordered pair of numbers, a Fraction (mathematic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Second Moment Of Area
The second moment of area, or second area moment, or quadratic moment of area and also known as the area moment of inertia, is a geometrical property of an area which reflects how its points are distributed with regard to an arbitrary axis. The second moment of area is typically denoted with either an I (for an axis that lies in the plane of the area) or with a J (for an axis perpendicular to the plane). In both cases, it is calculated with a multiple integral over the object in question. Its dimension is L (length) to the fourth power. Its unit of dimension, when working with the International System of Units, is meters to the fourth power, m4, or inches to the fourth power, in4, when working in the Imperial System of Units or the US customary system. In structural engineering, the second moment of area of a beam is an important property used in the calculation of the beam's deflection and the calculation of stress caused by a moment applied to the beam. In order to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Modulus Of Elasticity
An elastic modulus (also known as modulus of elasticity (MOE)) is a quantity that describes an object's or substance's resistance to being deformed elastically (i.e., non-permanently) when a stress is applied to it. Definition The elastic modulus of an object is defined as the slope of its stress–strain curve in the elastic deformation region: A stiffer material will have a higher elastic modulus. An elastic modulus has the form: :\delta \ \stackrel\ \frac where stress is the force causing the deformation divided by the area to which the force is applied and strain is the ratio of the change in some parameter caused by the deformation to the original value of the parameter. Since strain is a dimensionless quantity, the units of \delta will be the same as the units of stress. Elastic constants and moduli Elastic constants are specific parameters that quantify the stiffness of a material in response to applied stresses and are fundamental in defining the elastic pro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |