|
Magneto-hydrodynamics
Magnetohydrodynamics (MHD; also called magneto-fluid dynamics or hydromagnetics) is the study of the magnetic properties and behaviour of electrically conducting fluids. Examples of such magnetofluids include Plasma (physics), plasmas, liquid metals, Brine, salt water, and electrolytes. The word ''magnetohydrodynamics'' is derived from ' meaning magnetic field, ' meaning water, and ' meaning movement. The field of MHD was initiated by Hannes Alfvén, for which he received the Nobel Prize in Physics in 1970. The fundamental concept behind MHD is that magnetic fields can Electromagnetic induction, induce currents in a moving conductive fluid, which in turn polarizes the fluid and reciprocally changes the magnetic field itself. The set of equations that describe MHD are a combination of the Navier–Stokes equations of fluid dynamics and Maxwell’s equations of electromagnetism, electromagnetism. These differential equations must be solved simultaneous equ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Magnetohydrodynamic Flow Simulation
Magnetohydrodynamics (MHD; also called magneto-fluid dynamics or hydromagnetics) is the study of the magnetic properties and behaviour of electrically conducting fluids. Examples of such magnetofluids include plasmas, liquid metals, salt water, and electrolytes. The word ''magnetohydrodynamics'' is derived from ' meaning magnetic field, ' meaning water, and ' meaning movement. The field of MHD was initiated by Hannes Alfvén, for which he received the Nobel Prize in Physics in 1970. The fundamental concept behind MHD is that magnetic fields can induce currents in a moving conductive fluid, which in turn polarizes the fluid and reciprocally changes the magnetic field itself. The set of equations that describe MHD are a combination of the Navier–Stokes equations of fluid dynamics and Maxwell’s equations of electromagnetism. These differential equations must be solved simultaneously, either analytically or numerically. History The first re ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
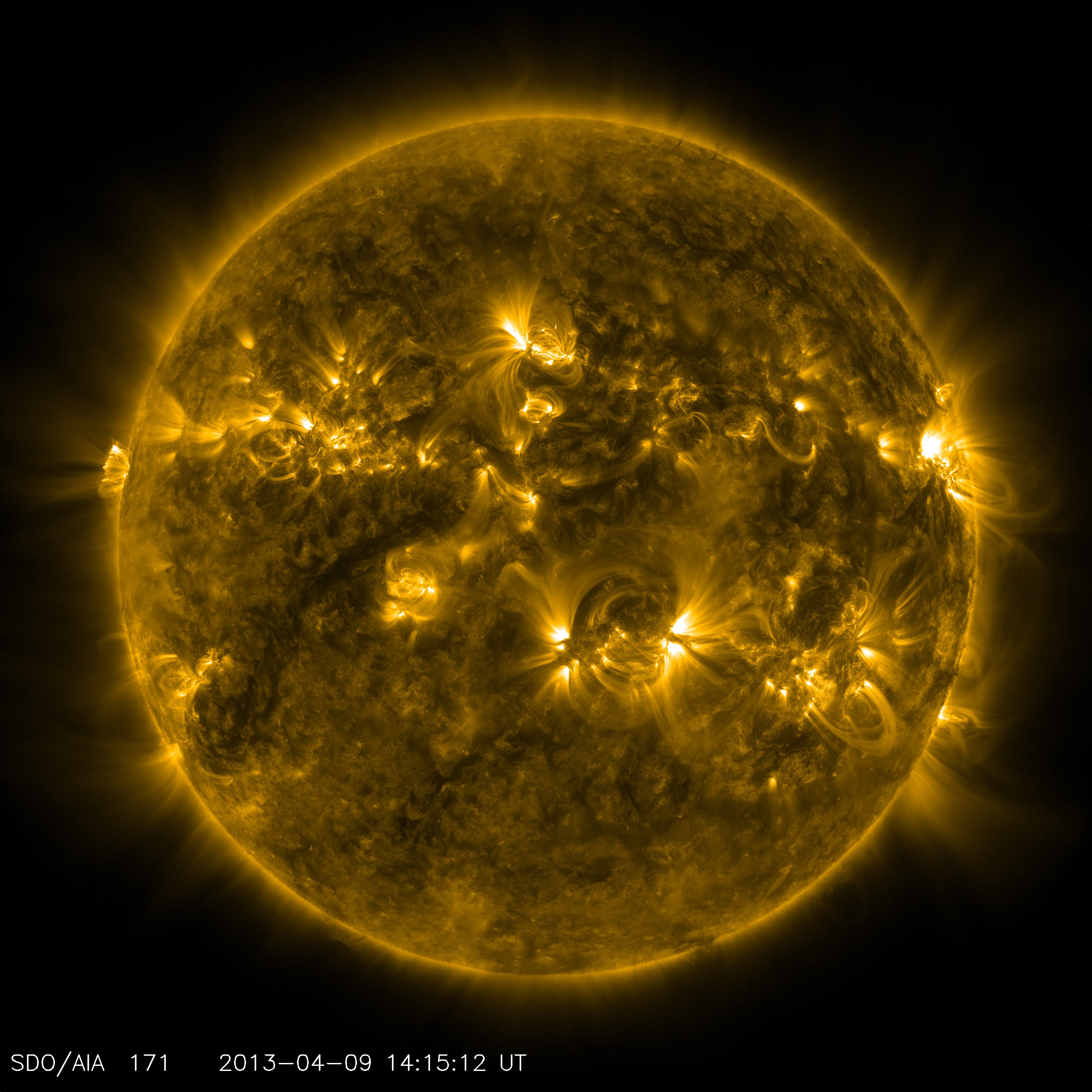
The Sun Is An MHD System That Is Not Well Understood- 2013-04-9 14-29
''The'' () is a grammatical article in English, denoting persons or things that are already or about to be mentioned, under discussion, implied or otherwise presumed familiar to listeners, readers, or speakers. It is the definite article in English. ''The'' is the most frequently used word in the English language; studies and analyses of texts have found it to account for seven percent of all printed English-language words. It is derived from gendered articles in Old English which combined in Middle English and now has a single form used with nouns of any gender. The word can be used with both singular and plural nouns, and with a noun that starts with any letter. This is different from many other languages, which have different forms of the definite article for different genders or numbers. Pronunciation In most dialects, "the" is pronounced as (with the voiced dental fricative followed by a schwa) when followed by a consonant sound, and as (homophone of the archaic pron ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Numerical Analysis
Numerical analysis is the study of algorithms that use numerical approximation (as opposed to symbolic computation, symbolic manipulations) for the problems of mathematical analysis (as distinguished from discrete mathematics). It is the study of numerical methods that attempt at finding approximate solutions of problems rather than the exact ones. Numerical analysis finds application in all fields of engineering and the physical sciences, and in the 21st century also the life and social sciences, medicine, business and even the arts. Current growth in computing power has enabled the use of more complex numerical analysis, providing detailed and realistic mathematical models in science and engineering. Examples of numerical analysis include: ordinary differential equations as found in celestial mechanics (predicting the motions of planets, stars and galaxies), numerical linear algebra in data analysis, and stochastic differential equations and Markov chains for simulating living ce ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Magnetic Reconnection
Magnetic reconnection is a physical process occurring in highly conducting plasmas in which the magnetic topology is rearranged and magnetic energy is converted to kinetic energy, thermal energy, and particle acceleration. Magnetic reconnection occurs on timescales intermediate between slow resistive diffusion of the magnetic field and fast Alfvénic timescales. The concept of magnetic reconnection was first introduced in 1950 in the PhD thesis of James Dungey to explain the coupling of mass, energy and momentum from the solar wind into Earth's magnetosphere and was published for the first time on the open literature in his seminal paper in 1961. Fundamental principles Magnetic reconnection is a breakdown of "ideal-magnetohydrodynamics" and so of "Alfvén's theorem" (also called the "frozen-in flux theorem") which applies to large-scale regions of a highly-conducting magnetoplasma, for which the Magnetic Reynolds Number is very large: this makes the convective term in th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Topology
In mathematics, topology (from the Greek language, Greek words , and ) is concerned with the properties of a mathematical object, geometric object that are preserved under Continuous function, continuous Deformation theory, deformations, such as Stretch factor, stretching, Twist (mathematics), twisting, crumpling, and bending; that is, without closing holes, opening holes, tearing, gluing, or passing through itself. A topological space is a set (mathematics), set endowed with a structure, called a ''Topology (structure), topology'', which allows defining continuous deformation of subspaces, and, more generally, all kinds of continuity (mathematics), continuity. Euclidean spaces, and, more generally, metric spaces are examples of a topological space, as any distance or metric defines a topology. The deformations that are considered in topology are homeomorphisms and homotopy, homotopies. A property that is invariant under such deformations is a topological property. Basic exampl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alfvén's Theorem
In magnetohydrodynamics, Alfvén's theorem, or the frozen-in flux theorem, "states that in a fluid with infinite electric conductivity, the magnetic field is frozen into the fluid and has to move along with it." Hannes Alfvén put the idea forward for the first time in 1942. In his own words: "In view of the infinite conductivity, every motion (perpendicular to the field) of the liquid in relation to the lines of force is forbidden because it would give infinite eddy currents. Thus the matter of the liquid is “fastened” to the lines of force...." In later life, Alfvén changed his mind and advised against use of his own theorem. However, Alfvén's theorem is much used today because of a second mechanism, magnetic reconnection. This is a breakdown of Alfvén's theorem in thin current sheets and is important as it can untangle field lines that would become increasingly tangled by plasma velocity shears and vortices in regions of low plasma beta if Alfvén's theorem applied everywh ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lenz's Law
Lenz's law states that the direction of the electric current induced in a conductor by a changing magnetic field is such that the magnetic field created by the induced current opposes changes in the initial magnetic field. It is named after physicist Emil Lenz, who formulated it in 1834. It is a qualitative law that specifies the direction of induced current, but states nothing about its magnitude. Lenz's law predicts the direction of many effects in electromagnetism, such as the direction of voltage induced in an inductor or wire loop by a changing current, or the drag force of eddy currents exerted on moving objects in a magnetic field. Lenz's law may be seen as analogous to Newton's third law in classical mechanicsSchmitt, Ron ''Electromagnetics explained'' 2002. Retrieved 16 July 2010. and Le Chatelier's principle in chemistry. Definition Lenz's law states that: The current induced in a circuit due to a change in a magnetic field is directed to oppose the change in fl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Magnetic Reynolds Number
In magnetohydrodynamics, the magnetic Reynolds number (Rm) is a dimensionless quantity that estimates the relative effects of advection or induction of a magnetic field by the motion of a conducting medium to the magnetic diffusion. It is the magnetic analogue of the Reynolds number in fluid mechanics and is typically defined by: : \mathrm_\mathrm = \frac ~~ \sim \frac where * U is a typical velocity scale of the flow, * L is a typical length scale of the flow, * \eta is the magnetic diffusivity. The mechanism by which the motion of a conducting fluid generates a magnetic field is the subject of dynamo theory. When the magnetic Reynolds number is very large, however, diffusion and the dynamo are less of a concern, and in this case focus instead often rests on the influence of the magnetic field on the flow. Derivation In the theory of magnetohydrodynamics, the magnetic Reynolds number can be derived from the induction equation: : \frac = \nabla \times (\mathbf \times \mathb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perfect Conductor
A perfect conductor or perfect electric conductor (PEC) is an idealized material exhibiting infinite electrical conductivity or, equivalently, zero resistivity (cf. perfect dielectric). While perfect electrical conductors do not exist in nature, the concept is a useful model when electrical resistance is negligible compared to other effects. One example is ideal magnetohydrodynamics, the study of perfectly conductive fluids. Another example is electrical circuit diagrams, which carry the implicit assumption that the wires connecting the components have no resistance. Yet another example is in computational electromagnetics, where PEC can be simulated faster, since the parts of equations that take finite conductivity into account can be neglected. Properties of perfect conductors Perfect conductors: *have exactly zero electrical resistance - a steady current within a perfect conductor will flow without losing energy to resistance. Resistance is what causes heating in conductors, t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Resistivity
Electrical resistivity (also called specific electrical resistance or volume resistivity) is a fundamental property of a material that measures how strongly it resists electric current. A low resistivity indicates a material that readily allows electric current. Resistivity is commonly represented by the Greek letter (rho). The SI unit of electrical resistivity is the ohm-meter (Ω⋅m). For example, if a solid cube of material has sheet contacts on two opposite faces, and the resistance between these contacts is , then the resistivity of the material is . Electrical conductivity or specific conductance is the reciprocal of electrical resistivity. It represents a material's ability to conduct electric current. It is commonly signified by the Greek letter ( sigma), but (kappa) (especially in electrical engineering) and (gamma) are sometimes used. The SI unit of electrical conductivity is siemens per metre (S/m). Resistivity and conductivity are intensi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
T3e Troy
The Cray T3E was Cray Research's second-generation massively parallel supercomputer architecture, launched in late November 1995. The first T3E was installed at the Pittsburgh Supercomputing Center in 1996. Like the previous Cray T3D, it was a fully distributed memory machine using a 3D torus topology interconnection network. The T3E initially used the DEC Alpha 21164 (EV5) microprocessor and was designed to scale from 8 to 2,176 ''Processing Elements'' (PEs). Each PE had between 64 MB and 2 GB of DRAM and a 6-way interconnect router with a payload bandwidth of 480 MB/s in each direction. Unlike many other MPP systems, including the T3D, the T3E was fully self-hosted and ran the UNICOS/mk distributed operating system with a ''GigaRing'' I/O subsystem integrated into the torus for network, disk and tape I/O. The original T3E (retrospectively known as the T3E-600) had a 300 MHz processor clock. Later variants, using the faster 21164A (EV56) processor, comprised the T3E-900 ( ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Michael Faraday
Michael Faraday (; 22 September 1791 – 25 August 1867) was an English scientist who contributed to the study of electromagnetism and electrochemistry. His main discoveries include the principles underlying electromagnetic induction, diamagnetism and electrolysis. Although Faraday received little formal education, he was one of the most influential scientists in history. It was by his research on the magnetic field around a conductor carrying a direct current that Faraday established the concept of the electromagnetic field in physics. Faraday also established that magnetism could affect rays of light and that there was an underlying relationship between the two phenomena.. the 1911 Encyclopædia Britannica. He similarly discovered the principles of electromagnetic induction, diamagnetism, and the laws of electrolysis. His inventions of electromagnetic rotary devices formed the foundation of electric motor technology, and it was largely due to his efforts t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |

.png)