|
Multivariate Stable Distribution
The multivariate stable distribution is a multivariate probability distribution that is a multivariate generalisation of the univariate stable distribution. The multivariate stable distribution defines linear relations between stable distribution marginals. In the same way as for the univariate case, the distribution is defined in terms of its characteristic function. The multivariate stable distribution can also be thought as an extension of the multivariate normal distribution. It has parameter, ''α'', which is defined over the range 0 < ''α'' ≤ 2, and where the case ''α'' = 2 is equivalent to the multivariate normal distribution. It has an additional skew parameter that allows for non-symmetric distributions, where the multivariate normal distribution is symmetric. Definitio ...
|
Mv Stable
MV may refer to: Businesses and organizations In transportation * Motor vessel, a motorized ship; used as a prefix for ship names * MV Agusta, a motorcycle manufacturer based in Cascina Costa, Italy * Armenian International Airways (IATA code MV) * Metropolitan-Vickers, an electrical equipment and vehicle manufacturer * Midland Valley Railroad, United States (reporting mark MV) * Merchant ship, Merchant vessel, ship prefix Other organizations * Mieterverband, a Swiss tenant organization * Millennium Volunteers, a former UK government initiative * Minnesota Vikings, an American football team * Miss Venezuela, a beauty pageant * Museum Victoria, an organization which operates three major state-owned museums in Melbourne, Victoria, Australia Places * Martha's Vineyard, an island located south of Cape Cod in Massachusetts * Maldives (ISO 3166-1 alpha-2 country code MV) * Mecklenburg-Vorpommern, a German state at the Baltic Sea * Mountain View, California, Mountain View, a city in Cal ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Exponent
In mathematics, exponentiation, denoted , is an operation involving two numbers: the ''base'', , and the ''exponent'' or ''power'', . When is a positive integer, exponentiation corresponds to repeated multiplication of the base: that is, is the product of multiplying bases: b^n = \underbrace_.In particular, b^1=b. The exponent is usually shown as a superscript to the right of the base as or in computer code as b^n. This binary operation is often read as " to the power "; it may also be referred to as " raised to the th power", "the th power of ", or, most briefly, " to the ". The above definition of b^n immediately implies several properties, in particular the multiplication rule:There are three common notations for multiplication: x\times y is most commonly used for explicit numbers and at a very elementary level; xy is most common when variables are used; x\cdot y is used for emphasizing that one talks of multiplication or when omitting the multiplication sign would ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
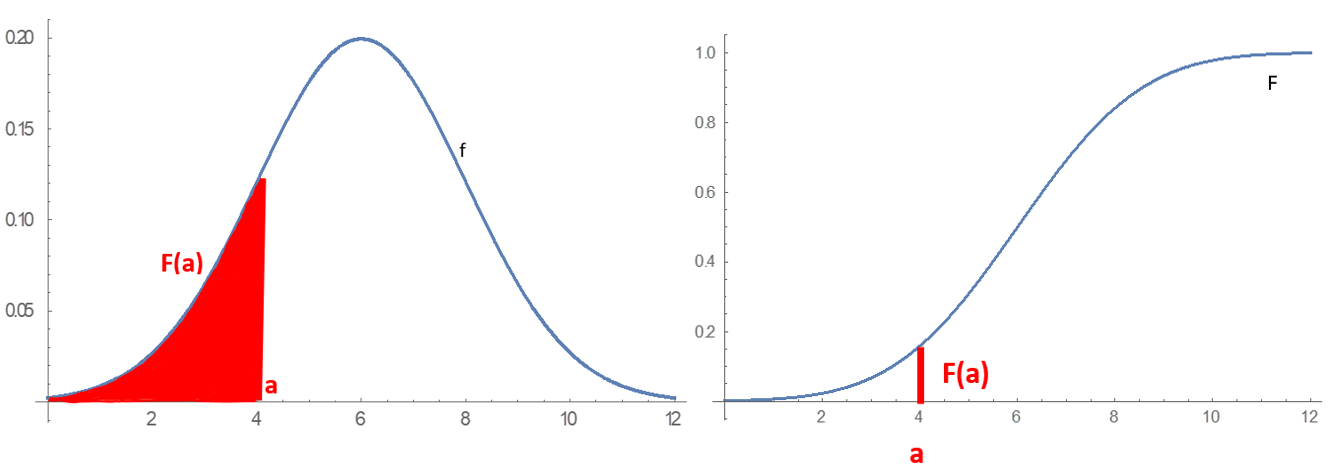
Probability Distribution
In probability theory and statistics, a probability distribution is a Function (mathematics), function that gives the probabilities of occurrence of possible events for an Experiment (probability theory), experiment. It is a mathematical description of a Randomness, random phenomenon in terms of its sample space and the Probability, probabilities of Event (probability theory), events (subsets of the sample space). For instance, if is used to denote the outcome of a coin toss ("the experiment"), then the probability distribution of would take the value 0.5 (1 in 2 or 1/2) for , and 0.5 for (assuming that fair coin, the coin is fair). More commonly, probability distributions are used to compare the relative occurrence of many different random values. Probability distributions can be defined in different ways and for discrete or for continuous variables. Distributions with special properties or for especially important applications are given specific names. Introduction A prob ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stable Distribution
In probability theory, a distribution is said to be stable if a linear combination of two independent random variables with this distribution has the same distribution, up to location and scale parameters. A random variable is said to be stable if its distribution is stable. The stable distribution family is also sometimes referred to as the Lévy alpha-stable distribution, after Paul Lévy, the first mathematician to have studied it. Of the four parameters defining the family, most attention has been focused on the stability parameter, \alpha (see panel). Stable distributions have 0 < \alpha \leq 2, with the upper bound corresponding to the , and to the Cauchy distribution. The distributio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Characteristic Function (probability Theory)
In probability theory and statistics, the characteristic function of any real-valued random variable completely defines its probability distribution. If a random variable admits a probability density function, then the characteristic function is the Fourier transform (with sign reversal) of the probability density function. Thus it provides an alternative route to analytical results compared with working directly with probability density functions or cumulative distribution functions. There are particularly simple results for the characteristic functions of distributions defined by the weighted sums of random variables. In addition to univariate distributions, characteristic functions can be defined for vector- or matrix-valued random variables, and can also be extended to more generic cases. The characteristic function always exists when treated as a function of a real-valued argument, unlike the moment-generating function. There are relations between the behavior of the charact ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Multivariate Normal Distribution
In probability theory and statistics, the multivariate normal distribution, multivariate Gaussian distribution, or joint normal distribution is a generalization of the one-dimensional ( univariate) normal distribution to higher dimensions. One definition is that a random vector is said to be ''k''-variate normally distributed if every linear combination of its ''k'' components has a univariate normal distribution. Its importance derives mainly from the multivariate central limit theorem. The multivariate normal distribution is often used to describe, at least approximately, any set of (possibly) correlated real-valued random variables, each of which clusters around a mean value. Definitions Notation and parametrization The multivariate normal distribution of a ''k''-dimensional random vector \mathbf = (X_1,\ldots,X_k)^ can be written in the following notation: : \mathbf\ \sim\ \mathcal(\boldsymbol\mu,\, \boldsymbol\Sigma), or to make it explicitly known that \mathb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Random Vector
In probability, and statistics, a multivariate random variable or random vector is a list or vector of mathematical variables each of whose value is unknown, either because the value has not yet occurred or because there is imperfect knowledge of its value. The individual variables in a random vector are grouped together because they are all part of a single mathematical system — often they represent different properties of an individual statistical unit. For example, while a given person has a specific age, height and weight, the representation of these features of ''an unspecified person'' from within a group would be a random vector. Normally each element of a random vector is a real number. Random vectors are often used as the underlying implementation of various types of aggregate random variables, e.g. a random matrix, random tree, random sequence, stochastic process, etc. Formally, a multivariate random variable is a column vector \mathbf = (X_1,\dots,X_n)^\maths ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Elliptical Distribution
In probability and statistics, an elliptical distribution is any member of a broad family of probability distributions that generalize the multivariate normal distribution. In the simplified two and three dimensional case, the joint distribution forms an ellipse and an ellipsoid, respectively, in iso-density plots. In statistics, the normal distribution is used in ''classical'' multivariate analysis, while elliptical distributions are used in ''generalized'' multivariate analysis, for the study of symmetric distributions with tails that are heavy, like the multivariate t-distribution, or light (in comparison with the normal distribution). Some statistical methods that were originally motivated by the study of the normal distribution have good performance for general elliptical distributions (with finite variance), particularly for spherical distributions (which are defined below). Elliptical distributions are also used in robust statistics to evaluate proposed multivariate-statis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mv Indp
MV may refer to: Businesses and organizations In transportation * Motor vessel, a motorized ship; used as a prefix for ship names * MV Agusta MV Agusta (, full name: MV AGUSTA Motor S.p.A., original name: Meccanica Verghera Agusta or MV) is an Italian high end motorcycle manufacturer. It was founded by Domenico Agusta, Count Domenico Agusta on 19 January 1945 as one of the branches of ..., a motorcycle manufacturer based in Cascina Costa, Italy * Armenian International Airways (IATA code MV) * Metropolitan-Vickers, an electrical equipment and vehicle manufacturer * Midland Valley Railroad, United States (reporting mark MV) * Merchant ship, Merchant vessel, ship prefix Other organizations * Mieterverband, a Swiss tenant organization * Millennium Volunteers, a former UK government initiative * Minnesota Vikings, an American football team * Miss Venezuela, a beauty pageant * Museum Victoria, an organization which operates three major state-owned museums in Melbourne, Victoria, A ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mv Indp2
MV may refer to: Businesses and organizations In transportation * Motor vessel, a motorized ship; used as a prefix for ship names * MV Agusta, a motorcycle manufacturer based in Cascina Costa, Italy * Armenian International Airways (IATA code MV) * Metropolitan-Vickers, an electrical equipment and vehicle manufacturer * Midland Valley Railroad, United States (reporting mark MV) * Merchant vessel, ship prefix Other organizations * Mieterverband, a Swiss tenant organization * Millennium Volunteers, a former UK government initiative * Minnesota Vikings, an American football team * Miss Venezuela, a beauty pageant * Museum Victoria, an organization which operates three major state-owned museums in Melbourne, Victoria, Australia Places * Martha's Vineyard, an island located south of Cape Cod in Massachusetts * Maldives (ISO 3166-1 alpha-2 country code MV) * Mecklenburg-Vorpommern, a German state at the Baltic Sea * Mountain View, a city in California, US People * M. Visvesvaraya ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Factor Analysis
Factor analysis is a statistical method used to describe variability among observed, correlated variables in terms of a potentially lower number of unobserved variables called factors. For example, it is possible that variations in six observed variables mainly reflect the variations in two unobserved (underlying) variables. Factor analysis searches for such joint variations in response to unobserved latent variables. The observed variables are modelled as linear combinations of the potential factors plus "error" terms, hence factor analysis can be thought of as a special case of errors-in-variables models. Simply put, the factor loading of a variable quantifies the extent to which the variable is related to a given factor. A common rationale behind factor analytic methods is that the information gained about the interdependencies between observed variables can be used later to reduce the set of variables in a dataset. Factor analysis is commonly used in psychometrics, pers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Multiuser Detection
Multiuser detection deals with demodulation of the mutually interfering digital streams of information that occur in areas such as wireless communications, high-speed data transmission, DSL, satellite communication, digital television, and magnetic recording. It is also being currently investigated for demodulation in low-power inter-chip and intra-chip communication. Multiuser detection encompasses both receiver technologies devoted to joint detection of all the interfering signals or to single-user receivers which are interested in recovering only one user but are robustified against multiuser interference and not just background noise. Mutual interference is unavoidable in modern spectrally efficient wireless systems: even when using orthogonal multiplexing systems such as TDMA, synchronous CDMA or OFDMA, multiuser interference originates from channel distortion and from out-of-cell interference. In addition, in multi-antenna (MIMO In radio, multiple-input and multiple-ou ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |