|
Logical Intuition
Logical Intuition, or mathematical intuition or rational intuition, is a series of instinctive foresight, know-how, and savviness often associated with the ability to perceive logical or mathematical truth—and the ability to solve mathematical challenges efficiently. Humans apply logical intuition in proving mathematical theorems, validating logical arguments, developing algorithms and heuristics, and in related contexts where mathematical challenges are involved. The ability to recognize logical or mathematical truth and identify viable methods may vary from person to person, and may even be a result of knowledge and experience, which are subject to cultivation. The ability may not be realizable in a computer program by means other than genetic programming or evolutionary programming. History Plato and Aristotle considered intuition a means for perceiving ideas, significant enough that for Aristotle, intuition comprised the only means of knowing principles that are not subjec ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Logic
Logic is the study of correct reasoning. It includes both formal and informal logic. Formal logic is the science of deductively valid inferences or of logical truths. It is a formal science investigating how conclusions follow from premises in a topic-neutral way. When used as a countable noun, the term "a logic" refers to a logical formal system that articulates a proof system. Formal logic contrasts with informal logic, which is associated with informal fallacies, critical thinking, and argumentation theory. While there is no general agreement on how formal and informal logic are to be distinguished, one prominent approach associates their difference with whether the studied arguments are expressed in formal or informal languages. Logic plays a central role in multiple fields, such as philosophy, mathematics, computer science, and linguistics. Logic studies arguments, which consist of a set of premises together with a conclusion. Premises and conclusions are usually un ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Self-evidence
In epistemology (theory of knowledge), a self-evident proposition is a proposition that is known to be true by understanding its meaning without proof, and/or by ordinary human reason. Some epistemologists deny that any proposition can be self-evident. For most others, one's belief that oneself is conscious is offered as an example of self-evidence. However, one's belief that someone else is conscious is not epistemically self-evident. The following proposition is often said to be self-evident: "A finite whole is greater than, or equal to, any of its parts". A logical argument for a self-evident conclusion would demonstrate only an ignorance of the purpose of persuasively arguing for the conclusion based on one or more premises that differ from it (see ' and begging the question). Analytic propositions It is sometimes said that a self-evident proposition is one whose denial is self-contradictory. It is also sometimes said that an analytic proposition is one whose denial is sel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Intuition
Intuition is the ability to acquire knowledge without recourse to conscious reasoning. Different fields use the word "intuition" in very different ways, including but not limited to: direct access to unconscious knowledge; unconscious cognition; gut feelings; inner sensing; inner insight to unconscious pattern-recognition; and the ability to understand something instinctively, without any need for conscious reasoning.Intuition and consciousness – Rosenblatt AD, Thickstun JT. Psychoanal Q. 1994 Oct;63(4):696-714. Intuitive knowledge tends to be approximate. The word ''intuition'' comes from the Latin verb ''intueri'' translated as "consider" or from the late middle English word ''intuit'', "to contemplate". Use of intuition is sometimes referred to as responding to a "gut feeling" or "trusting your gut".Wilding, M.How to Stop Overthinking and Start Trusting your Gut ''Harvard Business Review'', published 10 March 2022, accessed 21 September 2022 Psychology Freud According to Si ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
David Chalmers
David John Chalmers (; born 20 April 1966) is an Australian philosopher and cognitive scientist specializing in the areas of philosophy of mind and philosophy of language. He is a professor of philosophy and neural science at New York University, as well as co-director of NYU's Center for Mind, Brain and Consciousness (along with Ned Block). In 2006, he was elected a Fellow of the Australian Academy of the Humanities. In 2013, he was elected a Fellow of the American Academy of Arts & Sciences. Chalmers is best known for formulating the hard problem of consciousness. He and David Bourget cofounded PhilPapers, a database of journal articles for philosophers. Early life and education Chalmers was born in Sydney, New South Wales, in 1966, and subsequently grew up in Adelaide, South Australia, where he attended Unley High School. As a child, he experienced synesthesia. He began coding and playing computer games at age 10 on a PDP-10 at a medical center. He also performed excep ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hard Problem Of Consciousness
The hard problem of consciousness is the problem of explaining why and how humans have qualia or phenomenal experiences. This is in contrast to the "easy problems" of explaining the physical systems that give us and other animals the ability to discriminate, integrate information, and so forth. These problems are seen as relatively easy because all that is required for their solution is to specify the mechanisms that perform such functions. Philosopher David Chalmers writes that even once we have solved all such problems about the brain and experience, the hard problem will still persist. The existence of a "hard problem" is controversial. It has been accepted by philosophers of mind such as Joseph Levine, Colin McGinn, and Ned Block and cognitive neuroscientists such as Francisco Varela, Giulio Tononi, and Christof Koch. However, its existence is disputed by philosophers of mind such as Daniel Dennett, Massimo Pigliucci, Thomas Metzinger, Patricia Churchland, and Keith Fr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
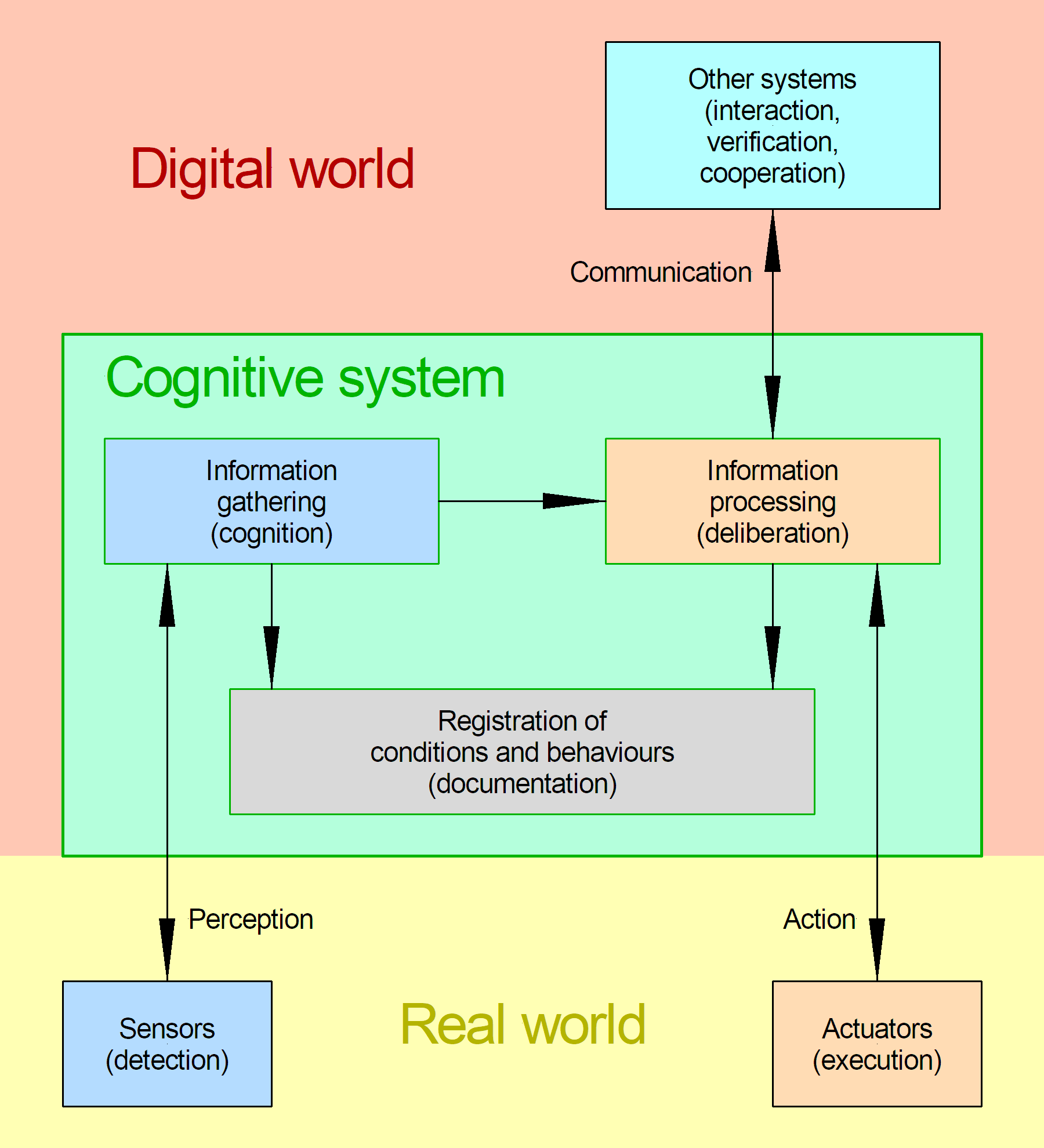
Cognitive Computing
Cognitive computing (CC) refers to technology platforms that, broadly speaking, are based on the scientific disciplines of artificial intelligence and signal processing. These platforms encompass machine learning, reasoning, natural language processing, speech recognition and vision (object recognition), human–computer interaction, dialog and narrative generation, among other technologies. Definition At present, there is no widely agreed upon definition for cognitive computing in either academia or industry. In general, the term cognitive computing has been used to refer to new hardware and/or software that mimics the functioning of the human brain (2004) and helps to improve human decision-making. In this sense, CC is a new type of computing with the goal of more accurate models of how the human brain/mind senses, reasons, and responds to stimulus. CC applications link data analysis and adaptive page displays ( AUI) to adjust content for a particular type of audience ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Artificial Intelligence
Artificial intelligence (AI) is intelligence—perceiving, synthesizing, and inferring information—demonstrated by machines, as opposed to intelligence displayed by animals and humans. Example tasks in which this is done include speech recognition, computer vision, translation between (natural) languages, as well as other mappings of inputs. The ''Oxford English Dictionary'' of Oxford University Press defines artificial intelligence as: the theory and development of computer systems able to perform tasks that normally require human intelligence, such as visual perception, speech recognition, decision-making, and translation between languages. AI applications include advanced web search engines (e.g., Google), recommendation systems (used by YouTube, Amazon and Netflix), understanding human speech (such as Siri and Alexa), self-driving cars (e.g., Tesla), automated decision-making and competing at the highest level in strategic game systems (such as chess and Go). ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Finite Set
In mathematics, particularly set theory, a finite set is a set that has a finite number of elements. Informally, a finite set is a set which one could in principle count and finish counting. For example, :\ is a finite set with five elements. The number of elements of a finite set is a natural number (possibly zero) and is called the '' cardinality (or the cardinal number)'' of the set. A set that is not a finite set is called an ''infinite set''. For example, the set of all positive integers is infinite: :\. Finite sets are particularly important in combinatorics, the mathematical study of counting. Many arguments involving finite sets rely on the pigeonhole principle, which states that there cannot exist an injective function from a larger finite set to a smaller finite set. Definition and terminology Formally, a set is called finite if there exists a bijection :f\colon S\to\ for some natural number . The number is the set's cardinality, denoted as . The empty set o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Philosophical Realism
Philosophical realism is usually not treated as a position of its own but as a stance towards other subject matters. Realism about a certain kind of thing (like numbers or morality) is the thesis that this kind of thing has ''mind-independent existence'', i.e. that it is not just a mere appearance in the eye of the beholder. This includes a number of positions within epistemology and metaphysics which express that a given thing instead exists independently of knowledge, thought, or understanding. This can apply to items such as the physical world, the past and future, other minds, and the self, though may also apply less directly to things such as universals, mathematical truths, moral truths, and thought itself. However, realism may also include various positions which instead reject metaphysical treatments of reality entirely. Realism can also be a view about the properties of reality in general, holding that reality exists independent of the mind, as opposed to non-realist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stanford Encyclopedia Of Philosophy
The ''Stanford Encyclopedia of Philosophy'' (''SEP'') combines an online encyclopedia of philosophy with peer-reviewed publication of original papers in philosophy, freely accessible to Internet users. It is maintained by Stanford University. Each entry is written and maintained by an expert in the field, including professors from many academic institutions worldwide. Authors contributing to the encyclopedia give Stanford University the permission to publish the articles, but retain the copyright to those articles. Approach and history As of August 5th, 2022, the ''SEP'' has 1,774 published entries. Apart from its online status, the encyclopedia uses the traditional academic approach of most encyclopedias and academic journals to achieve quality by means of specialist authors selected by an editor or an editorial committee that is competent (although not necessarily considered specialists) in the field covered by the encyclopedia and peer review. The encyclopedia was created in 1 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Many-valued Logic
Many-valued logic (also multi- or multiple-valued logic) refers to a propositional calculus in which there are more than two truth values. Traditionally, in Aristotle's logical calculus, there were only two possible values (i.e., "true" and "false") for any proposition. Classical two-valued logic may be extended to ''n''-valued logic for ''n'' greater than 2. Those most popular in the literature are three-valued (e.g., Łukasiewicz's and Kleene's, which accept the values "true", "false", and "unknown"), four-valued, nine-valued, the finite-valued (finitely-many valued) with more than three values, and the infinite-valued (infinitely-many-valued), such as fuzzy logic and probability logic. History It is wrong that the first known classical logician who did not fully accept the law of excluded middle was Aristotle (who, ironically, is also generally considered to be the first classical logician and the "father of wo-valuedlogic"). In fact, Aristotle did not contest the univer ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Propositional Calculus
Propositional calculus is a branch of logic. It is also called propositional logic, statement logic, sentential calculus, sentential logic, or sometimes zeroth-order logic. It deals with propositions (which can be true or false) and relations between propositions, including the construction of arguments based on them. Compound propositions are formed by connecting propositions by logical connectives. Propositions that contain no logical connectives are called atomic propositions. Unlike first-order logic, propositional logic does not deal with non-logical objects, predicates about them, or Quantifier (logic), quantifiers. However, all the machinery of propositional logic is included in first-order logic and higher-order logics. In this sense, propositional logic is the foundation of first-order logic and higher-order logic. Explanation Logical connectives are found in natural languages. In English for example, some examples are "and" (logical conjunction, conjunction), "or" (lo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |