|
Kinematic Chain
In mechanical engineering, a kinematic chain is an assembly of rigid bodies connected by joints to provide constrained (or desired) motion that is the mathematical model for a mechanical system. Reuleaux, F., 187''The Kinematics of Machinery,''(trans. and annotated by A. B. W. Kennedy), reprinted by Dover, New York (1963) As in the familiar use of the word chain, the rigid bodies, or links, are constrained by their connections to other links. An example is the simple open chain formed by links connected in series, like the usual chain, which is the kinematic model for a typical robot manipulator.J. M. McCarthy and G. S. Soh, 2010''Geometric Design of Linkages,''Springer, New York. Mathematical models of the connections, or joints, between two links are termed kinematic pairs. Kinematic pairs model the hinged and sliding joints fundamental to robotics, often called ''lower pairs'' and the surface contact joints critical to cams and gearing, called ''higher pairs.'' These ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
ATHLETE Robot Climbing A Hill
An athlete (also sportsman or sportswoman) is a person who competes in one or more sports that involve physical strength, speed, or endurance. Athletes may be professional sports, professionals or amateur sports, amateurs. Most professional athletes have particularly well-developed physiques obtained by extensive physical training and strict exercise accompanied by a strict dietary regimen. Definitions The word "athlete" is a romanization of the el, άθλητὴς, ''athlētēs'', one who participates in a contest; from ἄθλος, ''áthlos'' or ἄθλον, ''áthlon'', a contest or feat. The primary definition of "sportsman" according to Webster's ''Third Unabridged Dictionary'' (1960) is, "a person who is active in sports: as (a): one who engages in the sports of the field and especially in hunting or fishing." Physiology Athletes involved in isotonic exercises have an increased mean left ventricular end-diastolic volume and are less likely to be depressed. Due to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Compliant Mechanism
In mechanical engineering, a compliant mechanism is a flexible mechanism that achieves force and motion transmission through elastic body deformation. It gains some or all of its motion from the relative flexibility of its members rather than from rigid-body joints alone. These may be monolithic (single-piece) or jointless structures. Some common devices that use compliant mechanisms are backpack latches and paper clips. One of the oldest examples of using compliant structures is the bow and arrow. Design methods Compliant mechanisms are usually designed using two techniques: Kinematics approach Kinematic analysis can be used to design a compliant mechanism by creating a pseudo- rigid-body model of the mechanism. In this model, flexible segments are modeled as rigid links connected to revolute joints with torsional springs. Other structures can be modeled as a combination of rigid links, springs, and dampers. Structural optimization approach In this method, computationa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Graph Theory
In mathematics, graph theory is the study of ''graphs'', which are mathematical structures used to model pairwise relations between objects. A graph in this context is made up of '' vertices'' (also called ''nodes'' or ''points'') which are connected by '' edges'' (also called ''links'' or ''lines''). A distinction is made between undirected graphs, where edges link two vertices symmetrically, and directed graphs, where edges link two vertices asymmetrically. Graphs are one of the principal objects of study in discrete mathematics. Definitions Definitions in graph theory vary. The following are some of the more basic ways of defining graphs and related mathematical structures. Graph In one restricted but very common sense of the term, a graph is an ordered pair G=(V,E) comprising: * V, a set of vertices (also called nodes or points); * E \subseteq \, a set of edges (also called links or lines), which are unordered pairs of vertices (that is, an edge is associated with t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tree (graph Theory)
In graph theory In mathematics, graph theory is the study of ''graphs'', which are mathematical structures used to model pairwise relations between objects. A graph in this context is made up of '' vertices'' (also called ''nodes'' or ''points'') which are conne ..., a tree is an undirected graph in which any two Vertex (graph theory), vertices are connected by ''exactly one'' Path (graph theory), path, or equivalently a Connected graph, connected Cycle (graph theory), acyclic undirected graph. A forest is an undirected graph in which any two vertices are connected by ''at most one'' path, or equivalently an acyclic undirected graph, or equivalently a Disjoint union of graphs, disjoint union of trees. A polytreeSee . (or directed tree or oriented treeSee .See . or singly connected networkSee .) is a directed acyclic graph (DAG) whose underlying undirected graph is a tree. A polyforest (or directed forest or oriented forest) is a directed acyclic graph whose underlying undirecte ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
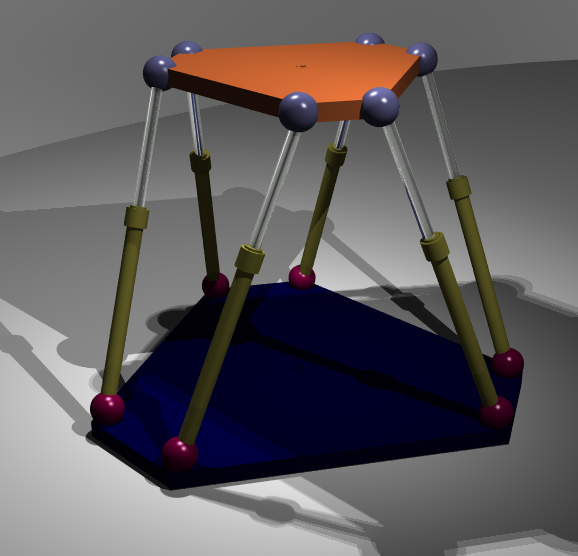
Parallel Manipulator
A parallel manipulator is a mechanical system that uses several computer-controlled serial chains to support a single platform, or end-effector. Perhaps, the best known parallel manipulator is formed from six linear actuators that support a movable base for devices such as flight simulators. This device is called a Stewart platform or the Gough-Stewart platform in recognition of the engineers who first designed and used them. Also known as parallel robots, or generalized Stewart platforms (in the Stewart platform, the actuators are paired together on both the basis and the platform), these systems are articulated robots that use similar mechanisms for the movement of either the robot on its base, or one or more manipulator arms. Their 'parallel' distinction, as opposed to a serial manipulator, is that the end effector (or 'hand') of this linkage (or 'arm') is directly connected to its base by a number of (usually three or six) separate and independent linkages working simu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Topology
In mathematics, topology (from the Greek language, Greek words , and ) is concerned with the properties of a mathematical object, geometric object that are preserved under Continuous function, continuous Deformation theory, deformations, such as Stretch factor, stretching, Twist (mathematics), twisting, crumpling, and bending; that is, without closing holes, opening holes, tearing, gluing, or passing through itself. A topological space is a set (mathematics), set endowed with a structure, called a ''Topology (structure), topology'', which allows defining continuous deformation of subspaces, and, more generally, all kinds of continuity (mathematics), continuity. Euclidean spaces, and, more generally, metric spaces are examples of a topological space, as any distance or metric defines a topology. The deformations that are considered in topology are homeomorphisms and homotopy, homotopies. A property that is invariant under such deformations is a topological property. Basic exampl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Inverse Kinematics
In computer animation and robotics, inverse kinematics is the mathematical process of calculating the variable joint parameters needed to place the end of a kinematic chain, such as a robot manipulator or animation character's skeleton, in a given position and orientation relative to the start of the chain. Given joint parameters, the position and orientation of the chain's end, e.g. the hand of the character or robot, can typically be calculated directly using multiple applications of trigonometric formulas, a process known as forward kinematics. However, the reverse operation is, in general, much more challenging. Inverse kinematics is also used to recover the movements of an object in the world from some other data, such as a film of those movements, or a film of the world as seen by a camera which is itself making those movements. This occurs, for example, where a human actor's filmed movements are to be duplicated by an animated character. Robotics In robotics, inverse ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Forward Kinematics
In robot kinematics, forward kinematics refers to the use of the kinematic equations of a robot to compute the position of the end-effector from specified values for the joint parameters. The kinematics equations of the robot are used in robotics, computer games, and animation. The reverse process, that computes the joint parameters that achieve a specified position of the end-effector, is known as inverse kinematics. Kinematics equations The kinematics equations for the series chain of a robot are obtained using a rigid transformation to characterize the relative movement allowed at each joint and separate rigid transformation to define the dimensions of each link. The result is a sequence of rigid transformations alternating joint and link transformations from the base of the chain to its end link, which is equated to the specified position for the end link, : = _1X_1] _2X_2]\ldots _Z_n],\! where is the transformation locating the end-link. These equations are called ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rigid Transformation
In mathematics, a rigid transformation (also called Euclidean transformation or Euclidean isometry) is a geometric transformation of a Euclidean space that preserves the Euclidean distance between every pair of points. The rigid transformations include rotations, translations, reflections, or any sequence of these. Reflections are sometimes excluded from the definition of a rigid transformation by requiring that the transformation also preserve the handedness of objects in the Euclidean space. (A reflection would not preserve handedness; for instance, it would transform a left hand into a right hand.) To avoid ambiguity, a transformation that preserves handedness is known as a proper rigid transformation, or rototranslation. Any proper rigid transformation can be decomposed into a rotation followed by a translation, while any improper rigid transformation can be decomposed into an improper rotation followed by a translation, or into a sequence of reflections. Any object wil ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebraic Equations
In mathematics Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ..., an algebraic equation or polynomial equation is an equation of the form :P = 0 where ''P'' is a polynomial with coefficients in some field (mathematics), field, often the field of the rational numbers. For many authors, the term ''algebraic equation'' refers only to ''univariate equations'', that is polynomial equations that involve only one variable (mathematics), variable. On the other hand, a polynomial equation may involve several variables. In the case of several variables (the ''multivariate'' case), the term ''polynomial equation'' is usually preferred to ''algebraic equation''. For example, :x^5-3x+1=0 is an algebraic equation with integer coefficients and :y^4 + \frac - \frac + xy^2 + y^2 + \frac = 0 is a m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Prismatic Joint
A prismatic joint is a one- degree-of-freedom kinematic pair which constrains the motion of two bodies to sliding along a common axis, without rotation; for this reason it is often called a slider (as in the slider-crank linkage) or a sliding pair. They are often utilized in hydraulic and pneumatic cylinders. A prismatic joint can be formed with a polygonal cross-section to resist rotation. See for example the dovetail joint and linear bearings. See also * Cylindrical joint * Degrees of freedom (mechanics) * Kinematic pair * Kinematics * Mechanical joint * Revolute joint A revolute joint (also called pin joint or hinge joint) is a one- degree-of-freedom kinematic pair used frequently in mechanisms and machines. The joint constrains the motion of two bodies to pure rotation along a common axis. The joint does ... References Kinematics Rigid bodies {{classicalmechanics-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hinge
A hinge is a mechanical bearing that connects two solid objects, typically allowing only a limited angle of rotation between them. Two objects connected by an ideal hinge rotate relative to each other about a fixed axis of rotation: all other Translation (geometry), translations or rotations being prevented, and thus a hinge has one degree of freedom. Hinges may be made of Flexure bearing, flexible material or of moving components. In biology, many joints function as hinges, like the elbow joint. History Ancient remains of stone, marble, wood, and bronze hinges have been found. Some date back to at least Ancient Egypt. In Ancient Rome, hinges were called wikt:cardo#Latin, cardō and gave name to the goddess Cardea and the main street Cardo. This name cardō lives on figuratively today as "the chief thing (on which something turns or depends)" in words such as ''wikt:cardinal#English, cardinal''. According to the OED, the English word hinge is related to ''wikt:hang#English, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
