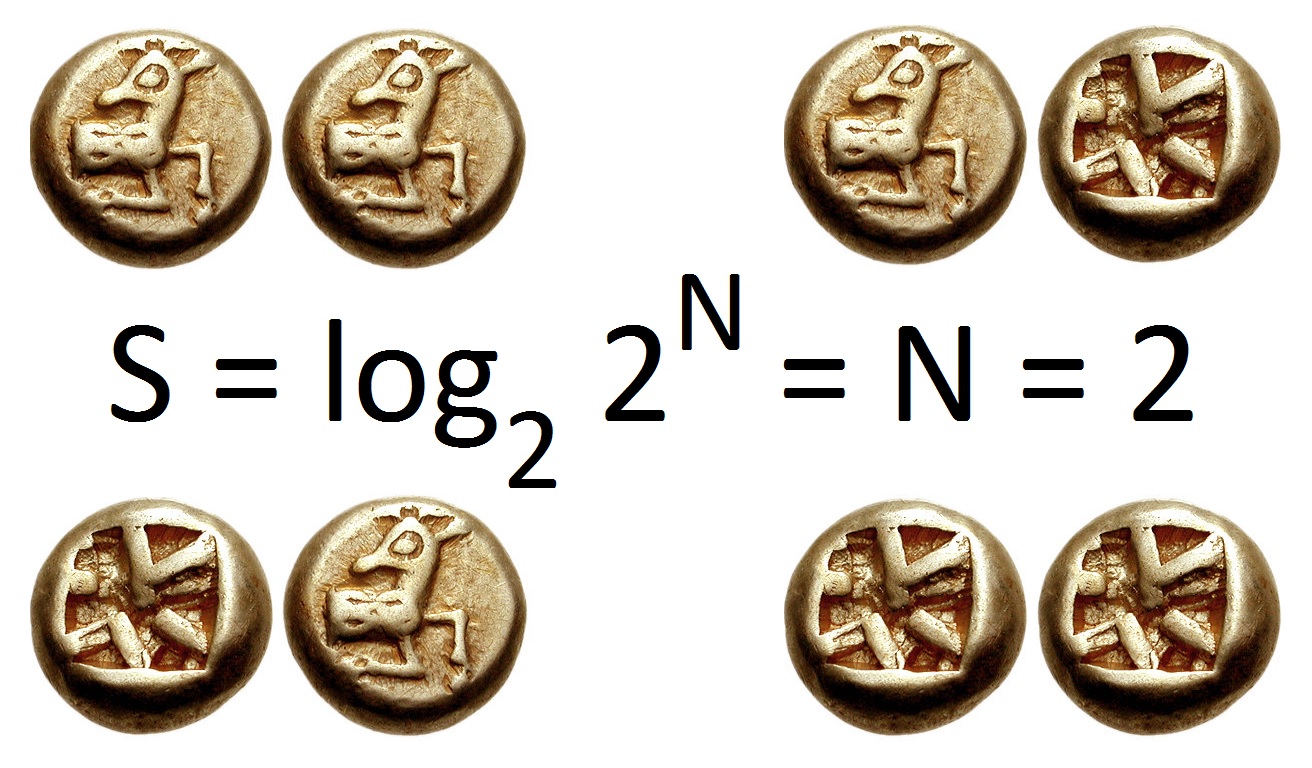|
Indeterminism
Indeterminism is the idea that events (or certain events, or events of certain types) are not caused, or do not cause deterministically. It is the opposite of determinism and related to chance. It is highly relevant to the philosophical problem of free will, particularly in the form of libertarianism. In science, most specifically quantum theory in physics, indeterminism is the belief that no event is certain and the entire outcome of anything is probabilistic. Heisenberg's uncertainty principle and the " Born rule", proposed by Max Born, are often starting points in support of the indeterministic nature of the universe. Indeterminism is also asserted by Sir Arthur Eddington, and Murray Gell-Mann. Indeterminism has been promoted by the French biologist Jacques Monod's essay "'' Chance and Necessity''". The physicist-chemist Ilya Prigogine argued for indeterminism in complex systems. Necessary but insufficient causation Indeterminists do not have to deny that causes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sir Arthur Eddington
Sir Arthur Stanley Eddington (28 December 1882 – 22 November 1944) was an English astronomer, physicist, and mathematician. He was also a philosopher of science and a populariser of science. The Eddington limit, the natural limit to the luminosity of stars, or the radiation generated by accretion onto a compact object, is named in his honour. Around 1920, he foreshadowed the discovery and mechanism of nuclear fusion processes in stars, in his paper "The Internal Constitution of the Stars".The Internal Constitution of the Stars A. S. Eddington The Scientific Monthly Vol. 11, No. 4 (Oct., 1920), pp. 297–303 At that time, the source of stellar energy was a complete mystery; Eddington was the first to correctly speculate that the source was fusion of hydrogen into helium. Eddington wrote a number of articles that announced and explained Einstein's theory of general relativity to the English-speaking world. World War I had severed many lines of scientific communication, and ne ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Causal Determinism
Determinism is a philosophical view, where all events are determined completely by previously existing causes. Deterministic theories throughout the history of philosophy have developed from diverse and sometimes overlapping motives and considerations. The opposite of determinism is some kind of indeterminism (otherwise called nondeterminism) or randomness. Determinism is often contrasted with free will, although some philosophers claim that the two are compatible.For example, see Determinism is often used to mean ''causal determinism'', which in physics is known as cause-and-effect. This is the concept that events within a given paradigm are bound by causality in such a way that any state of an object or event is completely determined by its prior states. This meaning can be distinguished from other varieties of determinism mentioned below. Debates about determinism often concern the scope of determined systems; some maintain that the entire universe is a single determi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Determinism
Determinism is a philosophical view, where all events are determined completely by previously existing causes. Deterministic theories throughout the history of philosophy have developed from diverse and sometimes overlapping motives and considerations. The opposite of determinism is some kind of indeterminism (otherwise called nondeterminism) or randomness. Determinism is often contrasted with free will, although some philosophers claim that the two are compatible.For example, see Determinism is often used to mean ''causal determinism'', which in physics is known as cause-and-effect. This is the concept that events within a given paradigm are bound by causality in such a way that any state of an object or event is completely determined by its prior states. This meaning can be distinguished from other varieties of determinism mentioned below. Debates about determinism often concern the scope of determined systems; some maintain that the entire universe is a single determi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Free Will
Free will is the capacity of agents to choose between different possible courses of action unimpeded. Free will is closely linked to the concepts of moral responsibility, praise, culpability, sin, and other judgements which apply only to actions that are freely chosen. It is also connected with the concepts of advice, persuasion, deliberation, and prohibition. Traditionally, only actions that are freely willed are seen as deserving credit or blame. Whether free will exists, what it is and the implications of whether it exists or not are some of the longest running debates of philosophy and religion. Some conceive of free will as the right to act outside of external influences or wishes. Some conceive free will to be the capacity to make choices undetermined by past events. Determinism suggests that only one course of events is possible, which is inconsistent with a libertarian model of free will. Ancient Greek philosophy identified this issue, which remains a major fo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Libertarianism (metaphysics)
Libertarianism is one of the main philosophical positions related to the problems of free will and determinism which are part of the larger domain of metaphysics. In particular, libertarianism is an incompatibilist position which argues that free will is logically incompatible with a deterministic universe. Libertarianism states that since agents have free will, determinism must be false. One of the first clear formulations of libertarianism is found in John Duns Scotus. In theological context, metaphysical libertarianism was notably defended by Jesuit authors like Luis de Molina and Francisco Suárez against rather compatibilist Thomist Bañecianism. Other important metaphysical libertarians in the early modern period were René Descartes, George Berkeley, Immanuel Kant and Thomas Reid. Roderick Chisholm was a prominent defender of libertarianism in the 20th century and contemporary libertarians include Robert Kane, Peter van Inwagen and Robert Nozick. Overview The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Leucippus
Leucippus (; el, Λεύκιππος, ''Leúkippos''; fl. 5th century BCE) is a pre-Socratic Greek philosopher who has been credited as the first philosopher to develop a theory of atomism. Leucippus' reputation, even in antiquity, was obscured by the reputation of his much more famous pupil, Democritus, who is also credited with the first development of atomic theory. Few details about Leucippus' life are known, and even his historical existence has on occasion been questioned. Biography Leucippus's dates are not recorded and he is often mentioned in conjunction with his more well-known pupil Democritus. Diogenes Laertius lists possible birthplaces as Miletus, Abdera and Elea. Some sources claim that around 440 or 430 BCE Leucippus founded a school at Abdera, with which his pupil, Democritus, was closely associated. There is mention that a Leucippus founded the city of Metapontum, which honored this Leucippus with a coin. Philosophical views Leucippus is typically asso ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Interpretations Of Quantum Mechanics
An interpretation of quantum mechanics is an attempt to explain how the mathematical theory of quantum mechanics might correspond to experienced reality. Although quantum mechanics has held up to rigorous and extremely precise tests in an extraordinarily broad range of experiments, there exist a number of contending schools of thought over their interpretation. These views on interpretation differ on such fundamental questions as whether quantum mechanics is deterministic or stochastic, which elements of quantum mechanics can be considered real, and what the nature of measurement is, among other matters. Despite nearly a century of debate and experiment, no consensus has been reached among physicists and philosophers of physics concerning which interpretation best "represents" reality. History The definition of quantum theorists' terms, such as ''wave function'' and ''matrix mechanics'', progressed through many stages. For instance, Erwin Schrödinger originally viewed the e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Entropy (information Theory)
In information theory, the entropy of a random variable is the average level of "information", "surprise", or "uncertainty" inherent to the variable's possible outcomes. Given a discrete random variable X, which takes values in the alphabet \mathcal and is distributed according to p: \mathcal\to , 1/math>: \Eta(X) := -\sum_ p(x) \log p(x) = \mathbb \log p(X), where \Sigma denotes the sum over the variable's possible values. The choice of base for \log, the logarithm, varies for different applications. Base 2 gives the unit of bits (or " shannons"), while base ''e'' gives "natural units" nat, and base 10 gives units of "dits", "bans", or " hartleys". An equivalent definition of entropy is the expected value of the self-information of a variable. The concept of information entropy was introduced by Claude Shannon in his 1948 paper "A Mathematical Theory of Communication",PDF archived froherePDF archived frohere and is also referred to as Shannon entropy. Shannon's theory def ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Necessary Cause
In logic and mathematics, necessity and sufficiency are terms used to describe a conditional or implicational relationship between two statements. For example, in the conditional statement: "If then ", is necessary for , because the truth of is guaranteed by the truth of (equivalently, it is impossible to have without ). Similarly, is sufficient for , because being true always implies that is true, but not being true does not always imply that is not true. In general, a necessary condition is one that must be present in order for another condition to occur, while a sufficient condition is one that produces the said condition. The assertion that a statement is a "necessary ''and'' sufficient" condition of another means that the former statement is true if and only if the latter is true. That is, the two statements must be either simultaneously true, or simultaneously false. In ordinary English (also natural language) "necessary" and "sufficient" indicate relations betw ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Inverse Distribution Function
In probability and statistics, the quantile function, associated with a probability distribution of a random variable, specifies the value of the random variable such that the probability of the variable being less than or equal to that value equals the given probability. Intuitively, the quantile function associates with a range at and below a probability input the likelihood that a random variable is realized in that range for some probability distribution. It is also called the percentile function, percent-point function or inverse cumulative distribution function. Definition Strictly monotonic distribution function With reference to a continuous and strictly monotonic cumulative distribution function F_X\colon \mathbb \to ,1/math> of a random variable ''X'', the quantile function Q\colon , 1\to \mathbb returns a threshold value ''x'' below which random draws from the given c.d.f. would fall ''100*p'' percent of the time. In terms of the distribution function ''F'', the qu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniform Distribution (continuous)
In probability theory and statistics, the continuous uniform distribution or rectangular distribution is a family of symmetric probability distributions. The distribution describes an experiment where there is an arbitrary outcome that lies between certain bounds. The bounds are defined by the parameters, ''a'' and ''b'', which are the minimum and maximum values. The interval can either be closed (e.g. , b or open (e.g. (a, b)). Therefore, the distribution is often abbreviated ''U'' (''a'', ''b''), where U stands for uniform distribution. The difference between the bounds defines the interval length; all intervals of the same length on the distribution's support are equally probable. It is the maximum entropy probability distribution for a random variable ''X'' under no constraint other than that it is contained in the distribution's support. Definitions Probability density function The probability density function of the continuous uniform distribution is: : f(x)=\be ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Probability Distribution
In probability theory and statistics, a probability distribution is the mathematical function that gives the probabilities of occurrence of different possible outcomes for an experiment. It is a mathematical description of a random phenomenon in terms of its sample space and the probabilities of events (subsets of the sample space). For instance, if is used to denote the outcome of a coin toss ("the experiment"), then the probability distribution of would take the value 0.5 (1 in 2 or 1/2) for , and 0.5 for (assuming that the coin is fair). Examples of random phenomena include the weather conditions at some future date, the height of a randomly selected person, the fraction of male students in a school, the results of a survey to be conducted, etc. Introduction A probability distribution is a mathematical description of the probabilities of events, subsets of the sample space. The sample space, often denoted by \Omega, is the set of all possible outcomes of a ra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |

.jpg)