|
Impedance Control
Impedance control is an approach to dynamic control relating force and position. It is often used in applications where a manipulator interacts with its environment and the force position relation is of concern. Examples of such applications include humans interacting with robots, where the force produced by the human relates to how fast the robot should move/stop. Simpler control methods, such as position control or torque control, perform poorly when the manipulator experiences contacts. Thus impedance control is commonly used in these settings. Mechanical impedance is the ratio of force output to motion input. This is analogous to electrical impedance that is the ratio of voltage output to current input (e.g. resistance is voltage divided by current). A "spring constant" defines the force output for a displacement (extension or compression) of the spring. A "damping constant" defines the force output for a velocity input. If we control the impedance of a mechanism, we are co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Manipulator (device)
In robotics, a manipulator is a device used to manipulate materials without direct physical contact by the operator. The applications were originally for dealing with radioactive or biohazardous materials, using robotic arms, or they were used in inaccessible places. In more recent developments they have been used in diverse range of applications including welding automation, robotic surgery and in space. It is an arm-like mechanism that consists of a series of segments, usually sliding or jointed called cross-slides, which grasp and move objects with a number of degrees of freedom. In industrial ergonomics a manipulator is a lift-assist device used to help workers lift, maneuver and place articles in process that are too heavy, too hot, too large or otherwise too difficult for a single worker to manually handle. As opposed to simply vertical lift assists (cranes, hoists, etc.) manipulators have the ability to reach in to tight spaces and remove workpieces. A good example wo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Admittance
In electrical engineering, admittance is a measure of how easily a circuit or device will allow a current to flow. It is defined as the reciprocal of impedance, analogous to how conductance & resistance are defined. The SI unit of admittance is the siemens (symbol S); the older, synonymous unit is mho, and its symbol is ℧ (an upside-down uppercase omega Ω). Oliver Heaviside coined the term ''admittance'' in December 1887. Heaviside used ''Y'' to represent the magnitude of admittance, but it quickly became the conventional symbol for admittance itself through the publications of Charles Proteus Steinmetz. Heaviside probably chose ''Y'' simply because it is next to ''Z'' in the alphabet, the conventional symbol for impedance. Admittance is defined as :Y \equiv \frac \, where :''Y'' is the admittance, measured in siemens :''Z'' is the impedance, measured in ohms Resistance is a measure of the opposition of a circuit to the flow of a steady current, while impedance ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Electrical Impedance
In electrical engineering, impedance is the opposition to alternating current presented by the combined effect of resistance and reactance in a circuit. Quantitatively, the impedance of a two-terminal circuit element is the ratio of the complex representation of the sinusoidal voltage between its terminals, to the complex representation of the current flowing through it. In general, it depends upon the frequency of the sinusoidal voltage. Impedance extends the concept of resistance to alternating current (AC) circuits, and possesses both magnitude and phase, unlike resistance, which has only magnitude. Impedance can be represented as a complex number, with the same units as resistance, for which the SI unit is the ohm (). Its symbol is usually , and it may be represented by writing its magnitude and phase in the polar form . However, Cartesian complex number representation is often more powerful for circuit analysis purposes. The notion of impedance is useful for pe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Serial Manipulator
Serial manipulators are the most common industrial robots and they are designed as a series of links connected by motor-actuated joints that extend from a base to an end-effector. Often they have an anthropomorphic arm structure described as having a "shoulder", an "elbow", and a "wrist". Serial robots usually have six joints, because it requires at least six degrees of freedom to place a manipulated object in an arbitrary position and orientation in the workspace of the robot. A popular application for serial robots in today's industry is the pick-and-place assembly robot, called a SCARA robot, which has four degrees of freedom. Structure In its most general form, a serial robot consists of a number of rigid links connected with joints. Simplicity considerations in manufacturing and control have led to robots with only revolute or prismatic joints and orthogonal, parallel and/or intersecting joint axes (instead of arbitrarily placed joint axes). Donald L. Pieper derived ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
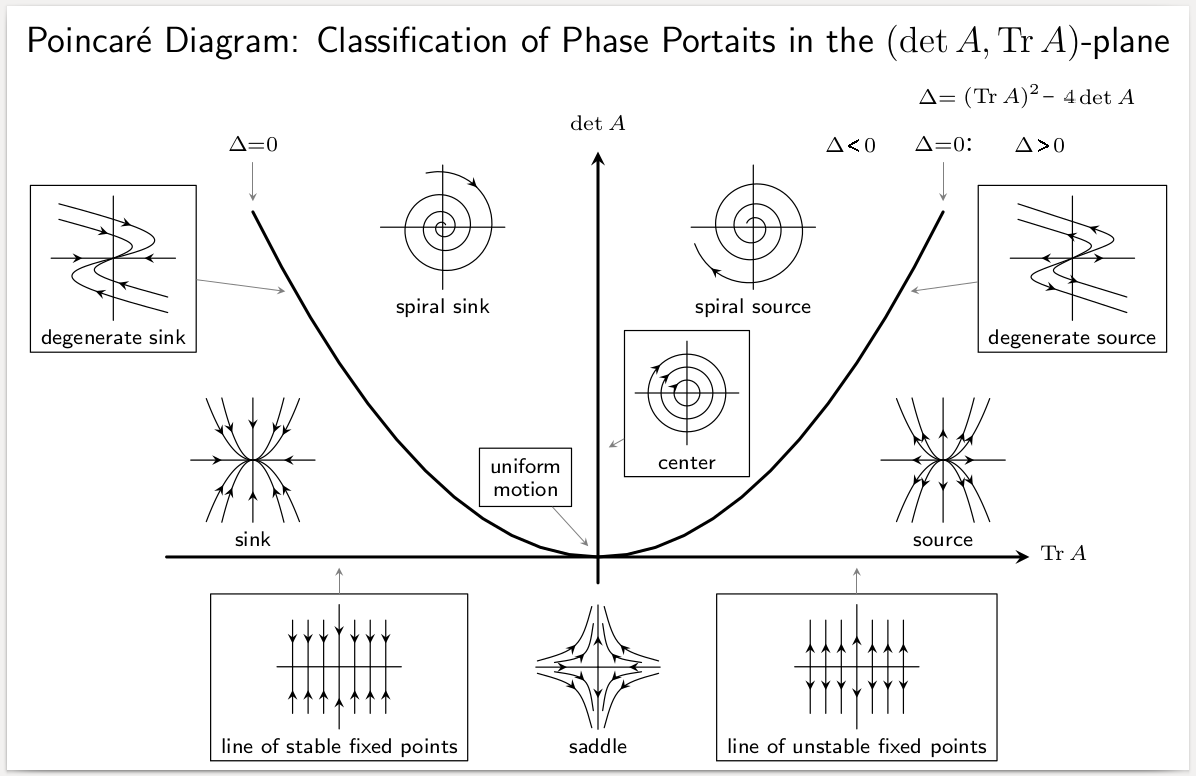
Stability Theory
In mathematics, stability theory addresses the stability of solutions of differential equations and of trajectories of dynamical systems under small perturbations of initial conditions. The heat equation, for example, is a stable partial differential equation because small perturbations of initial data lead to small variations in temperature at a later time as a result of the maximum principle. In partial differential equations one may measure the distances between functions using Lp norms or the sup norm, while in differential geometry one may measure the distance between spaces using the Gromov–Hausdorff distance. In dynamical systems, an orbit is called '' Lyapunov stable'' if the forward orbit of any point is in a small enough neighborhood or it stays in a small (but perhaps, larger) neighborhood. Various criteria have been developed to prove stability or instability of an orbit. Under favorable circumstances, the question may be reduced to a well-studied problem invo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |