|
High-dimensional Model Representation
High-dimensional model representation is a finite expansion for a given ''multivariable'' function. The expansion was first described by Ilya M. Sobol as : f(\mathbf) = f_0+ \sum_^nf_i(x_i)+ \sum_^n f_(x_,x_)+ \cdots + f_(x_1,\ldots,x_n). The method, used to determine the right hand side functions, is given in Sobol's paper. A review can be found hereHigh Dimensional Model Representation (HDMR): Concepts and Applications The underlying logic behind the HDMR is to express all variable interactions in a system in a hierarchical order. For instance f_0 represents the mean response of the model f. It can be considered as measuring what is left from the model after stripping down all variable effects. The uni-variate functions f_i(x_i), however represents the "individual" contributions of the variables. For instance, f_1(x_1) is the portion of the model that can be controlled only by the variable x_1. For this reason, there can not be any constant in f_1(x_1) because all constants ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Finite
Finite may refer to: * Finite set, a set whose cardinality (number of elements) is some natural number * Finite verb, a verb form that has a subject, usually being inflected or marked for person and/or tense or aspect * "Finite", a song by Sara Groves from the album ''Invisible Empires'' See also * Finite number (other) * Finite part (other) * Finite map (other) * Finite presentation (other) * Finite type (other) Finite type refers to several related concepts in mathematics: * Algebra of finite type, an associative algebra with finitely many generators **Morphism of finite type, a morphism of schemes with underlying morphisms on affine opens given by algebr ... * * Nonfinite (other) {{disambiguation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Expansion (geometry)
In geometry, expansion is a polytope operation where Facet (mathematics), facets are separated and moved radially apart, and new facets are formed at separated elements (Vertex (geometry), vertices, Edge (geometry), edges, etc.). Equivalently this operation can be imagined by keeping facets in the same position but reducing their size. The expansion of a Regular polytope, regular convex polytope creates a uniform polytope, uniform convex polytope. For polyhedra, an expanded polyhedron has all the Face (geometry), faces of the original polyhedron, all the faces of the dual polyhedron, and new square faces in place of the original edges. Expansion of regular polytopes According to Coxeter, this multidimensional term was defined by Alicia Boole StottCoxeter, ''Regular Polytopes'' (1973), p. 123. p.210 for creating new polytopes, specifically starting from regular polytopes to construct new uniform polytopes. The ''expansion'' operation is symmetric with respect to a regular p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Function (mathematics)
In mathematics, a function from a set (mathematics), set to a set assigns to each element of exactly one element of .; the words ''map'', ''mapping'', ''transformation'', ''correspondence'', and ''operator'' are sometimes used synonymously. The set is called the Domain of a function, domain of the function and the set is called the codomain of the function. Functions were originally the idealization of how a varying quantity depends on another quantity. For example, the position of a planet is a ''function'' of time. History of the function concept, Historically, the concept was elaborated with the infinitesimal calculus at the end of the 17th century, and, until the 19th century, the functions that were considered were differentiable function, differentiable (that is, they had a high degree of regularity). The concept of a function was formalized at the end of the 19th century in terms of set theory, and this greatly increased the possible applications of the concept. A f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Ilya M
Ilya, Iliya, Ilia, Ilja, Ilija, or Illia ( , or ; ; ) is the East Slavic form of the male Hebrew name Eliyahu (Eliahu), meaning "My God is Yahu/Jah." It comes from the Byzantine Greek pronunciation of the vocative (Ilía) of the Greek Elias (Ηλίας, Ilías). It is pronounced with stress on the second syllable. The diminutive form is Iliusha or Iliushen'ka. The Russian patronymic for a son of Ilya is " Ilyich", and a daughter is "Ilyinichna". People with the name Real people *Ilya (Archbishop of Novgorod), 12th-century Russian Orthodox cleric and saint *Ilya Ivanovitch Alekseyev (1772–1830), commander of the Russian Imperial Army *Ilya Borok (born 1993), Russian jiujitsu fighter *Ilya Bryzgalov (born 1980), Russian ice hockey goalie * Ilya Dzhirkvelov (1927–2006), author and KGB defector *Ilya Ehrenburg (1891–1967), Russian writer and Soviet cultural ambassador *Ilya Frank (1908–1990), Russian physicist *Ilya Glazunov (1930–2017), Russian painter *Ilya Gringolts ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
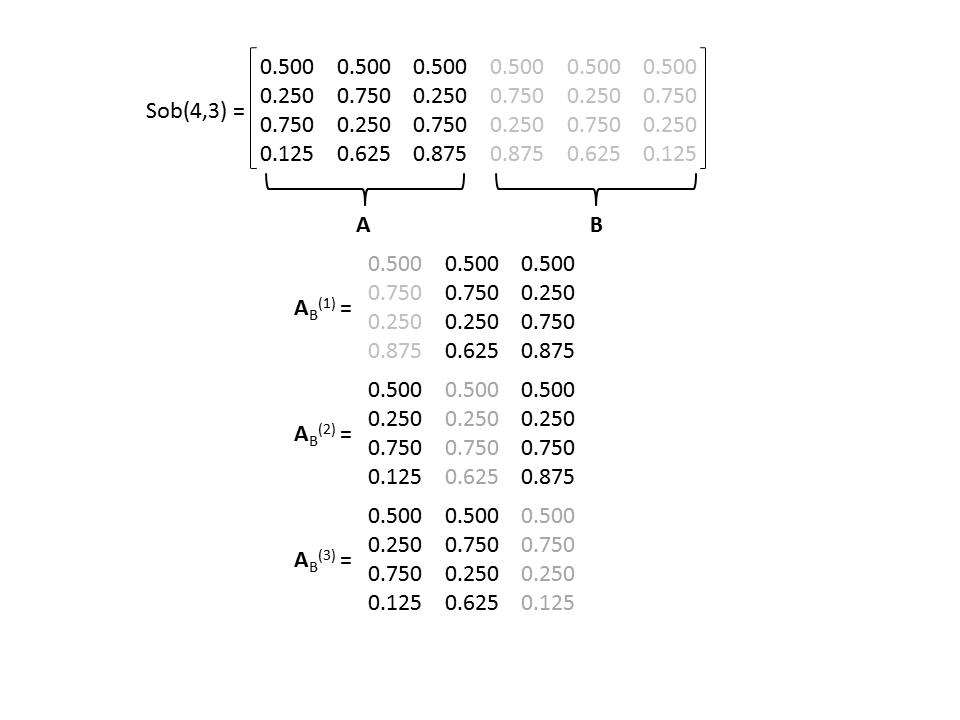
Variance-based Sensitivity Analysis
Variance-based sensitivity analysis (often referred to as the Sobol’ method or Sobol’ indices, after Ilya M. Sobol’) is a form of global sensitivity analysis.Sobol, I.M. (2001), Global sensitivity indices for nonlinear mathematical models and their Monte Carlo estimates. ''MATH COMPUT SIMULAT'',55(1–3),271-280, Saltelli, A., Ratto, M., Andres, T., Campolongo, F., Cariboni, J., Gatelli, D. Saisana, M., and Tarantola, S., 2008, ''Global Sensitivity Analysis. The Primer'', John Wiley & Sons. Working within a probabilistic framework, it decomposes the variance of the output of the model or system into fractions which can be attributed to inputs or sets of inputs. For example, given a model with two inputs and one output, one might find that 70% of the output variance is caused by the variance in the first input, 20% by the variance in the second, and 10% due to interactions between the two. These percentages are directly interpreted as measures of sensitivity. Variance-based me ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Volterra Series
The Volterra series is a model for non-linear behavior similar to the Taylor series. It differs from the Taylor series in its ability to capture "memory" effects. The Taylor series can be used for approximating the response of a nonlinear system to a given input if the output of the system depends strictly on the input at that particular time. In the Volterra series, the output of the nonlinear system depends on the input to the system at ''all'' other times. This provides the ability to capture the "memory" effect of devices like capacitors and inductors. It has been applied in the fields of medicine (biomedical engineering) and biology, especially neuroscience. It is also used in electrical engineering to model intermodulation distortion in many devices, including power amplifiers and frequency mixers. Its main advantage lies in its generalizability: it can represent a wide range of systems. Thus, it is sometimes considered a non-parametric model. In mathematics, a Volterra se ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |