|
Fluctuation-dissipation Theorem
The fluctuation–dissipation theorem (FDT) or fluctuation–dissipation relation (FDR) is a powerful tool in statistical physics for predicting the behavior of systems that obey detailed balance. Given that a system obeys detailed balance, the theorem is a proof that thermodynamic fluctuations in a physical variable predict the response quantified by the admittance or impedance (to be intended in their general sense, not only in electromagnetic terms) of the same physical variable (like voltage, temperature difference, etc.), and vice versa. The fluctuation–dissipation theorem applies both to classical and quantum mechanical systems. The fluctuation–dissipation theorem was proven by Herbert Callen and Theodore Welton in 1951 and expanded by Ryogo Kubo. There are antecedents to the general theorem, including Einstein's explanation of Brownian motion during his ''annus mirabilis'' and Harry Nyquist's explanation in 1928 of Johnson noise in electrical resistors. Qualitat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistical Physics
Statistical physics is a branch of physics that evolved from a foundation of statistical mechanics, which uses methods of probability theory and statistics, and particularly the mathematical tools for dealing with large populations and approximations, in solving physical problems. It can describe a wide variety of fields with an inherently stochastic nature. Its applications include many problems in the fields of physics, biology, chemistry, and neuroscience. Its main purpose is to clarify the properties of matter in aggregate, in terms of physical laws governing atomic motion. Statistical mechanics develop the phenomenological results of thermodynamics from a probabilistic examination of the underlying microscopic systems. Historically, one of the first topics in physics where statistical methods were applied was the field of classical mechanics, which is concerned with the motion of particles or objects when subjected to a force. Scope Statistical physics explains and quant ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Thermal Fluctuation
In statistical mechanics, thermal fluctuations are random deviations of a system from its average state, that occur in a system at equilibrium.In statistical mechanics they are often simply referred to as fluctuations. All thermal fluctuations become larger and more frequent as the temperature increases, and likewise they decrease as temperature approaches absolute zero. Thermal fluctuations are a basic manifestation of the temperature of systems: A system at nonzero temperature does not stay in its equilibrium microscopic state, but instead randomly samples all possible states, with probabilities given by the Boltzmann distribution. Thermal fluctuations generally affect all the degrees of freedom of a system: There can be random vibrations (phonons), random rotations ( rotons), random electronic excitations, and so forth. Thermodynamic variables, such as pressure, temperature, or entropy, likewise undergo thermal fluctuations. For example, for a system that has an equilibri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Terminal Velocity
Terminal velocity is the maximum velocity (speed) attainable by an object as it falls through a fluid ( air is the most common example). It occurs when the sum of the drag force (''Fd'') and the buoyancy is equal to the downward force of gravity (''FG'') acting on the object. Since the net force on the object is zero, the object has zero acceleration. In fluid dynamics an object is moving at its terminal velocity if its speed is constant due to the restraining force exerted by the fluid through which it is moving. As the speed of an object increases, so does the drag force acting on it, which also depends on the substance it is passing through (for example air or water). At some speed, the drag or force of resistance will equal the gravitational pull on the object (buoyancy is considered below). At this point the object stops accelerating and continues falling at a constant speed called the terminal velocity (also called settling velocity). An object moving downward faster than ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fick's Law Of Diffusion
Fick's laws of diffusion describe diffusion and were derived by Adolf Fick in 1855. They can be used to solve for the diffusion coefficient, . Fick's first law can be used to derive his second law which in turn is identical to the diffusion equation. A diffusion process that obeys Fick's laws is called normal or Fickian diffusion; otherwise, it is called anomalous diffusion or non-Fickian diffusion. History In 1855, physiologist Adolf Fick first reported* * his now well-known laws governing the transport of mass through diffusive means. Fick's work was inspired by the earlier experiments of Thomas Graham, which fell short of proposing the fundamental laws for which Fick would become famous. Fick's law is analogous to the relationships discovered at the same epoch by other eminent scientists: Darcy's law (hydraulic flow), Ohm's law (charge transport), and Fourier's Law (heat transport). Fick's experiments (modeled on Graham's) dealt with measuring the concentrations and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Einstein–Smoluchowski Relation
In physics (specifically, the kinetic theory of gases), the Einstein relation is a previously unexpected connection revealed independently by William Sutherland in 1904, Albert Einstein in 1905, and by Marian Smoluchowski in 1906 in their works on Brownian motion. The more general form of the equation is D = \mu \, k_\text T, where * is the diffusion coefficient; * is the "mobility", or the ratio of the particle's terminal drift velocity to an applied force, ; * is the Boltzmann constant; * is the absolute temperature. This equation is an early example of a fluctuation-dissipation relation. Two frequently used important special forms of the relation are: * Einstein–Smoluchowski equation, for diffusion of charged particles: D = \frac * Stokes–Einstein equation, for diffusion of spherical particles through a liquid with low Reynolds number: D = \frac Here * is the electrical charge of a particle; * is the electrical mobility of the charged particle; * is the dy ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistical Mechanics
In physics, statistical mechanics is a mathematical framework that applies statistical methods and probability theory to large assemblies of microscopic entities. It does not assume or postulate any natural laws, but explains the macroscopic behavior of nature from the behavior of such ensembles. Statistical mechanics arose out of the development of classical thermodynamics, a field for which it was successful in explaining macroscopic physical properties—such as temperature, pressure, and heat capacity—in terms of microscopic parameters that fluctuate about average values and are characterized by probability distributions. This established the fields of statistical thermodynamics and statistical physics. The founding of the field of statistical mechanics is generally credited to three physicists: *Ludwig Boltzmann, who developed the fundamental interpretation of entropy in terms of a collection of microstates *James Clerk Maxwell, who developed models of probability ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistical Thermodynamics
In physics, statistical mechanics is a mathematical framework that applies statistical methods and probability theory to large assemblies of microscopic entities. It does not assume or postulate any natural laws, but explains the macroscopic behavior of nature from the behavior of such ensembles. Statistical mechanics arose out of the development of classical thermodynamics, a field for which it was successful in explaining macroscopic physical properties—such as temperature, pressure, and heat capacity—in terms of microscopic parameters that fluctuate about average values and are characterized by probability distributions. This established the fields of statistical thermodynamics and statistical physics. The founding of the field of statistical mechanics is generally credited to three physicists: *Ludwig Boltzmann, who developed the fundamental interpretation of entropy in terms of a collection of microstates *James Clerk Maxwell, who developed models of probability distri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kirchhoff's Law Of Thermal Radiation
In heat transfer, Kirchhoff's law of thermal radiation refers to wavelength-specific radiative emission and absorption by a material body in thermodynamic equilibrium, including radiative exchange equilibrium. It is a special case of Onsager reciprocal relations as a consequence of the time reversibility of microscopic dynamics, also known as microscopic reversibility. A body at temperature radiates electromagnetic energy. A perfect black body in thermodynamic equilibrium absorbs all light that strikes it, and radiates energy according to a unique law of radiative emissive power for temperature ( Stefan–Boltzmann law), universal for all perfect black bodies. Kirchhoff's law states that: Here, the dimensionless coefficient of absorption (or the absorptivity) is the fraction of incident light (power) that is absorbed by the body when it is radiating and absorbing in thermodynamic equilibrium. In slightly different terms, the emissive power of an arbitrary opaque bod ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Thermal Radiation
Thermal radiation is electromagnetic radiation generated by the thermal motion of particles in matter. Thermal radiation is generated when heat from the movement of charges in the material (electrons and protons in common forms of matter) is converted to electromagnetic radiation. All matter with a temperature greater than absolute zero emits thermal radiation. At room temperature, most of the emission is in the infrared (IR) spectrum. Particle motion results in charge-acceleration or dipole oscillation which produces electromagnetic radiation. Infrared radiation emitted by animals (detectable with an infrared camera) and cosmic microwave background radiation are examples of thermal radiation. If a radiation object meets the physical characteristics of a black body in thermodynamic equilibrium, the radiation is called blackbody radiation. Planck's law describes the spectrum of blackbody radiation, which depends solely on the object's temperature. Wien's displacemen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Absorption (electromagnetic Radiation)
In physics, absorption of electromagnetic radiation is how matter (typically electrons bound in atoms) takes up a photon's energy — and so transforms electromagnetic energy into internal energy of the absorber (for example, thermal energy). A notable effect is attenuation, or the gradual reduction of the intensity of light waves as they propagate through a medium. Although the absorption of waves does not usually depend on their intensity (linear absorption), in certain conditions ( optics) the medium's transparency changes by a factor that varies as a function of wave intensity, and saturable absorption (or nonlinear absorption) occurs. Quantifying absorption Many approaches can potentially quantify radiation absorption, with key examples following. * The absorption coefficient along with some closely related derived quantities * The attenuation coefficient (NB used infrequently with meaning synonymous with "absorption coefficient") * The Molar attenuation co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Joule Heating
Joule heating, also known as resistive, resistance, or Ohmic heating, is the process by which the passage of an electric current through a conductor produces heat. Joule's first law (also just Joule's law), also known in countries of former USSR as the Joule–Lenz law, Assuming the element behaves as a perfect resistor and that the power is completely converted into heat, the formula can be re-written by substituting Ohm's law, V = I R , into the generalized power equation: P = IV = I^2R = V^2/R where ''R'' is the resistance. Alternating current When current varies, as it does in AC circuits, P(t) = U(t) I(t) where ''t'' is time and ''P'' is the instantaneous power being converted from electrical energy to heat. Far more often, the ''average'' power is of more interest than the instantaneous power: P_ = U_\text I_\text = I_\text^2 R = U_\text^2 / R where "avg" denotes average (mean) over one or more cycles, and "rms" denotes root mean square. These formulas are valid fo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
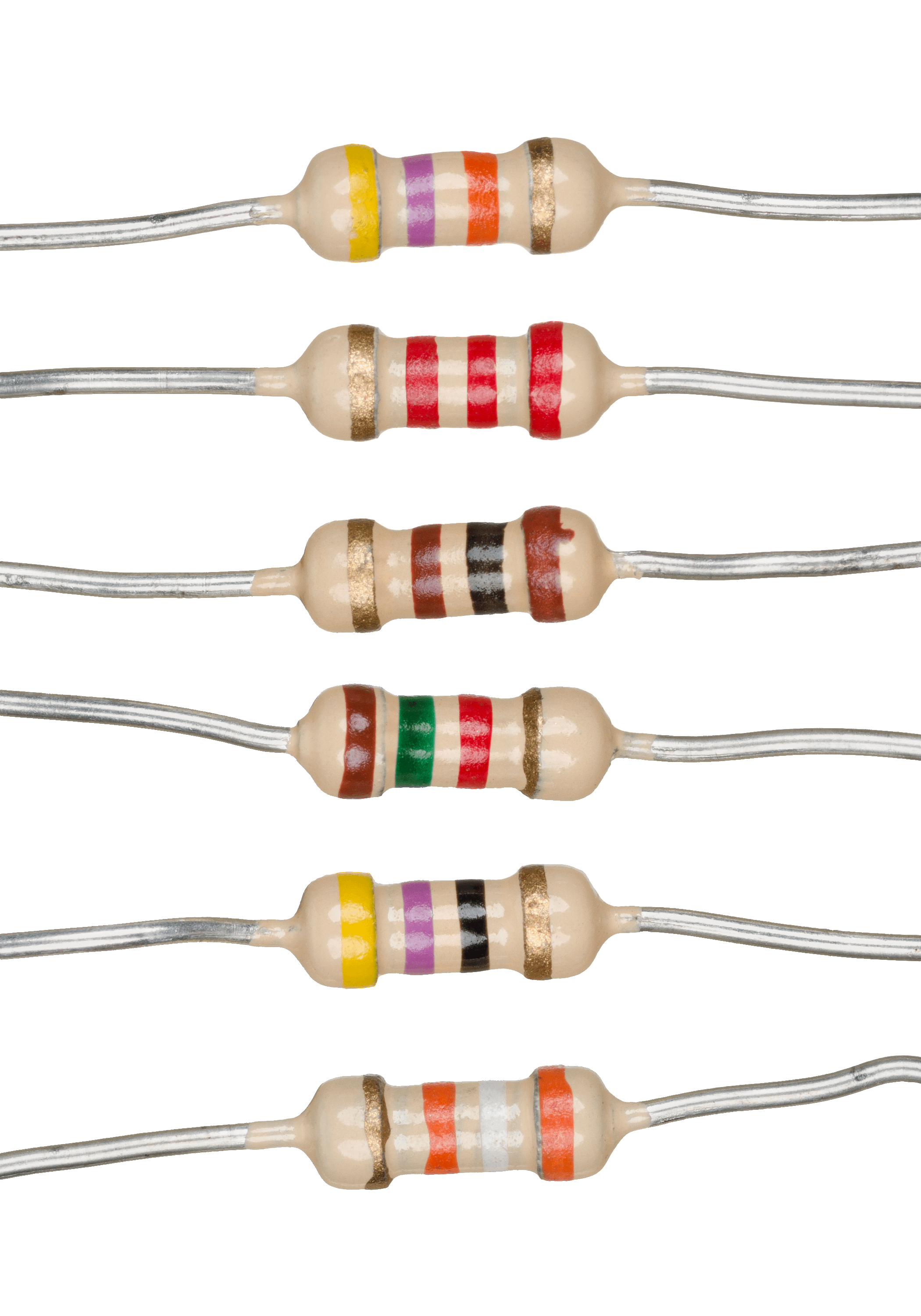
Resistor
A resistor is a passive two-terminal electrical component that implements electrical resistance as a circuit element. In electronic circuits, resistors are used to reduce current flow, adjust signal levels, to divide voltages, bias active elements, and terminate transmission lines, among other uses. High-power resistors that can dissipate many watts of electrical power as heat may be used as part of motor controls, in power distribution systems, or as test loads for generators. Fixed resistors have resistances that only change slightly with temperature, time or operating voltage. Variable resistors can be used to adjust circuit elements (such as a volume control or a lamp dimmer), or as sensing devices for heat, light, humidity, force, or chemical activity. Resistors are common elements of electrical networks and electronic circuits and are ubiquitous in electronic equipment. Practical resistors as discrete components can be composed of various compounds and forms. R ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |