|
Exponent Pair
In mathematics, van der Corput's method generates estimates for exponential sums. The method applies two processes, the van der Corput processes A and B which relate the sums into simpler sums which are easier to estimate. The processes apply to exponential sums of the form : \sum_^b e(f(n)) \ where ''f'' is a sufficiently smooth function and ''e''(''x'') denotes exp(2Ï€i''x''). Process A To apply process A, write the first difference ''f''''h''(''x'') for ''f''(''x''+''h'')−''f''(''x''). Assume there is ''H'' ≤ ''b''−''a'' such that : \sum_^H \left\vert\right\vert \le b-a \ . Then : \left\vert\right\vert \ll \frac \ . Process B Process B transforms the sum involving ''f'' into one involving a function ''g'' defined in terms of the derivative of f. Suppose that ''f is monotone increasing with ''f'''(''a'') = α, ''f'''(''b'') = β. Then ''f''' is invertible on ±,βwith inverse ''u'' say. Further suppose ''f'''' ≥ λ > 0. Write : g(y) = f(u(y)) - y u(y) \ . ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Exponential Sum
In mathematics, an exponential sum may be a finite Fourier series (i.e. a trigonometric polynomial), or other finite sum formed using the exponential function, usually expressed by means of the function :e(x) = \exp(2\pi ix).\, Therefore, a typical exponential sum may take the form :\sum_n e(x_n), summed over a finite sequence of real numbers ''x''''n''. Formulation If we allow some real coefficients ''a''''n'', to get the form :\sum_n a_n e(x_n) it is the same as allowing exponents that are complex numbers. Both forms are certainly useful in applications. A large part of twentieth century analytic number theory was devoted to finding good estimates for these sums, a trend started by basic work of Hermann Weyl in diophantine approximation. Estimates The main thrust of the subject is that a sum :S=\sum_n e(x_n) is ''trivially'' estimated by the number ''N'' of terms. That is, the absolute value :, S, \le N\, by the triangle inequality, since each summand has absolute va ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Smooth Function
In mathematical analysis, the smoothness of a function (mathematics), function is a property measured by the number of Continuous function, continuous Derivative (mathematics), derivatives it has over some domain, called ''differentiability class''. At the very minimum, a function could be considered smooth if it is differentiable everywhere (hence continuous). At the other end, it might also possess derivatives of all Order of derivation, orders in its Domain of a function, domain, in which case it is said to be infinitely differentiable and referred to as a C-infinity function (or C^ function). Differentiability classes Differentiability class is a classification of functions according to the properties of their derivatives. It is a measure of the highest order of derivative that exists and is continuous for a function. Consider an open set U on the real line and a function f defined on U with real values. Let ''k'' be a non-negative integer. The function f is said to be of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Differentiable Function
In mathematics, a differentiable function of one real variable is a function whose derivative exists at each point in its domain. In other words, the graph of a differentiable function has a non-vertical tangent line at each interior point in its domain. A differentiable function is smooth (the function is locally well approximated as a linear function at each interior point) and does not contain any break, angle, or cusp. If is an interior point in the domain of a function , then is said to be ''differentiable at'' if the derivative f'(x_0) exists. In other words, the graph of has a non-vertical tangent line at the point . is said to be differentiable on if it is differentiable at every point of . is said to be ''continuously differentiable'' if its derivative is also a continuous function over the domain of the function f. Generally speaking, is said to be of class if its first k derivatives f^(x), f^(x), \ldots, f^(x) exist and are continuous over the domain of the func ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
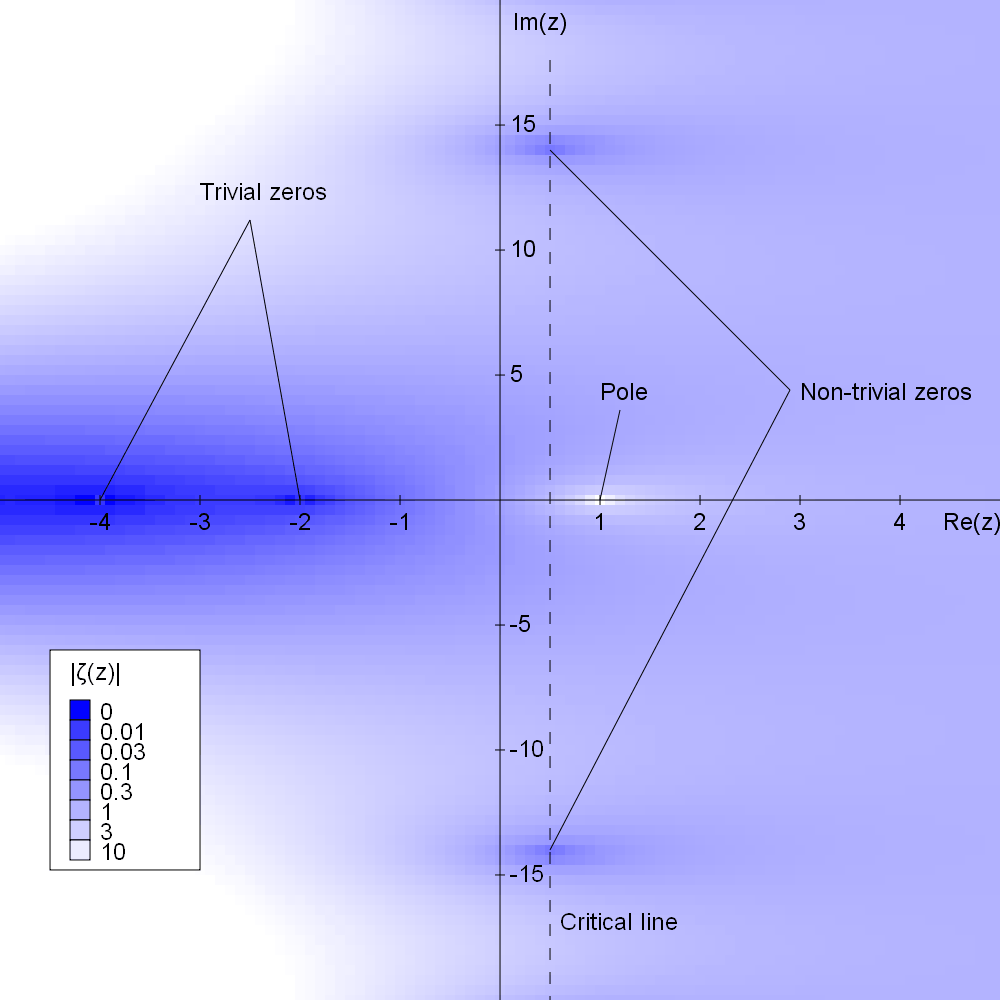
Riemann Zeta Function
The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter (zeta), is a mathematical function of a complex variable defined as \zeta(s) = \sum_^\infty \frac = \frac + \frac + \frac + \cdots for \operatorname(s) > 1 and its analytic continuation elsewhere. The Riemann zeta function plays a pivotal role in analytic number theory, and has applications in physics, probability theory, and applied statistics. Leonhard Euler first introduced and studied the function over the reals in the first half of the eighteenth century. Bernhard Riemann's 1859 article "On the Number of Primes Less Than a Given Magnitude" extended the Euler definition to a complex variable, proved its meromorphic continuation and functional equation, and established a relation between its zeros and the distribution of prime numbers. This paper also contained the Riemann hypothesis, a conjecture about the distribution of complex zeros of the Riemann zeta function that is consid ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Critical Line (mathematics)
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in pure mathematics. It is of great interest in number theory because it implies results about the distribution of prime numbers. It was proposed by , after whom it is named. The Riemann hypothesis and some of its generalizations, along with Goldbach's conjecture and the twin prime conjecture, make up Hilbert's eighth problem in David Hilbert's list of twenty-three unsolved problems; it is also one of the Clay Mathematics Institute's Millennium Prize Problems, which offers a million dollars to anyone who solves any of them. The name is also used for some closely related analogues, such as the Riemann hypothesis for curves over finite fields. The Riemann zeta function ζ(''s'') is a function whose argument ''s'' may be any complex number othe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lindelöf Hypothesis
In mathematics, the Lindelöf hypothesis is a conjecture by Finnish mathematician Ernst Leonard Lindelöf (see ) about the rate of growth of the Riemann zeta function on the critical line. This hypothesis is implied by the Riemann hypothesis. It says that for any ''ε'' > 0, \zeta\!\left(\frac + it\right)\! = O(t^\varepsilon) as ''t'' tends to infinity (see big O notation). Since ''ε'' can be replaced by a smaller value, the conjecture can be restated as follows: for any positive ''ε'', \zeta\!\left(\frac + it\right)\! = o(t^\varepsilon). The μ function If σ is real, then ''μ''(σ) is defined to be the infimum of all real numbers ''a'' such that ζ(σ + ''iT'' ) = O(''T'' ''a''). It is trivial to check that ''μ''(σ) = 0 for σ > 1, and the functional equation of the zeta function implies that ''μ''(σ) = ''μ''(1 − σ) − σ + 1/2. The Phragmén–Lindelöf theorem implies that ''μ'' is a conve ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
American Mathematical Society
The American Mathematical Society (AMS) is an association of professional mathematicians dedicated to the interests of mathematical research and scholarship, and serves the national and international community through its publications, meetings, advocacy and other programs. The society is one of the four parts of the Joint Policy Board for Mathematics and a member of the Conference Board of the Mathematical Sciences. History The AMS was founded in 1888 as the New York Mathematical Society, the brainchild of Thomas Fiske, who was impressed by the London Mathematical Society on a visit to England. John Howard Van Amringe was the first president and Fiske became secretary. The society soon decided to publish a journal, but ran into some resistance, due to concerns about competing with the American Journal of Mathematics. The result was the ''Bulletin of the American Mathematical Society'', with Fiske as editor-in-chief. The de facto journal, as intended, was influential in in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Springer-Verlag
Springer Science+Business Media, commonly known as Springer, is a German multinational publishing company of books, e-books and peer-reviewed journals in science, humanities, technical and medical (STM) publishing. Originally founded in 1842 in Berlin, it expanded internationally in the 1960s, and through mergers in the 1990s and a sale to venture capitalists it fused with Wolters Kluwer and eventually became part of Springer Nature in 2015. Springer has major offices in Berlin, Heidelberg, Dordrecht, and New York City. History Julius Springer founded Springer-Verlag in Berlin in 1842 and his son Ferdinand Springer grew it from a small firm of 4 employees into Germany's then second largest academic publisher with 65 staff in 1872.Chronology ". Springer Science+Business Media. In 1964, Springer expanded its business internationally, o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Exponentials
Exponential may refer to any of several mathematical topics related to exponentiation, including: *Exponential function, also: **Matrix exponential, the matrix analogue to the above * Exponential decay, decrease at a rate proportional to value *Exponential discounting, a specific form of the discount function, used in the analysis of choice over time *Exponential growth, where the growth rate of a mathematical function is proportional to the function's current value *Exponential map (Riemannian geometry), in Riemannian geometry * Exponential map (Lie theory), in Lie theory * Exponential notation, also known as scientific notation, or standard form *Exponential object, in category theory *Exponential time, in complexity theory *in probability and statistics: ** Exponential distribution, a family of continuous probability distributions **Exponentially modified Gaussian distribution, describes the sum of independent normal and exponential random variables **Exponential family, a paramet ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |