|
Digital Sundial
A digital sundial is a clock that indicates the current time with numerals formed by the sunlight striking it. Like a classical sundial, the device contains no moving parts. It uses no electricity nor other manufactured sources of energy. The digital display changes as the sun advances in its daily course. Technique There are two basic types of digital sundials. One type uses optical waveguides, while the other is inspired by fractal geometry. Optical fiber sundial Sunlight enters into the device through a slit and moves as the sun advances. The sun's rays shine on ten linearly distributed sockets of optical waveguides that transport the light to a seven-segment display. Each socket fiber is connected to a few segments forming the digit corresponding to the position of the sun. Fractal sundial The theoretical basis for the other construction comes from fractal geometry. For the sake of simplicity, we describe a two-dimensional (planar) version. Let denote a straight line p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sundial
A sundial is a horological device that tells the time of day (referred to as civil time in modern usage) when direct sunlight shines by the apparent position of the Sun in the sky. In the narrowest sense of the word, it consists of a flat plate (the ''dial'') and a gnomon, which casts a shadow onto the dial. As the Sun appears to move through the sky, the shadow aligns with different hour-lines, which are marked on the dial to indicate the time of day. The ''style'' is the time-telling edge of the gnomon, though a single point or ''nodus'' may be used. The gnomon casts a broad shadow; the shadow of the style shows the time. The gnomon may be a rod, wire, or elaborately decorated metal casting. The style must be parallel to the axis of the Earth's rotation for the sundial to be accurate throughout the year. The style's angle from horizontal is equal to the sundial's geographical latitude. The term ''sundial'' can refer to any device that uses the Sun's altitude or azimut ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Waveguide (optics)
An optical waveguide is a physical structure that guides electromagnetic waves in the optical spectrum. Common types of optical waveguides include optical fiber waveguides, transparent dielectric waveguides made of plastic and glass, liquid light guides, and liquid waveguides. Optical waveguides are used as components in integrated optical circuits or as the transmission medium in local and long-haul optical communication systems. Optical waveguides can be classified according to their geometry (planar, strip, or fiber waveguides), mode structure (single-mode optical fiber, single-mode, multi-mode optical fiber, multi-mode), refractive index distribution (step or gradient index), and material (glass, polymer, semiconductor). Total internal reflection The basic principles behind optical waveguides can be described using the concepts of geometrical optics, geometrical or ray optics, as illustrated in the diagram. Light passing into a medium with higher refractive index bends towa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
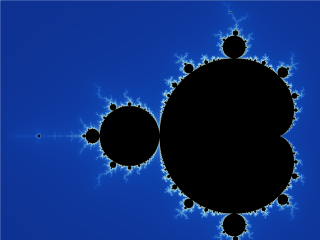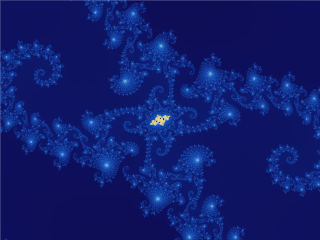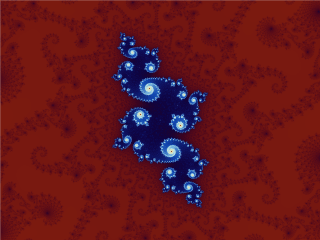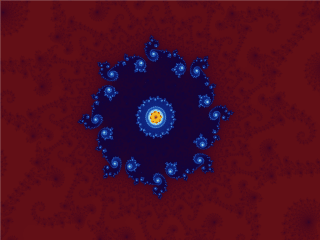
Fractal
In mathematics, a fractal is a geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scales, as illustrated in successive magnifications of the Mandelbrot set. This exhibition of similar patterns at increasingly smaller scales is called self-similarity, also known as expanding symmetry or unfolding symmetry; if this replication is exactly the same at every scale, as in the Menger sponge, the shape is called affine self-similar. Fractal geometry lies within the mathematical branch of measure theory. One way that fractals are different from finite geometric figures is how they scale. Doubling the edge lengths of a filled polygon multiplies its area by four, which is two (the ratio of the new to the old side length) raised to the power of two (the conventional dimension of the filled polygon). Likewise, if the radius of a filled sphere i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Seven-segment Display
A seven-segment display is a form of electronic display device for displaying decimal numerals that is an alternative to the more complex dot matrix displays. Seven-segment displays are widely used in digital clocks, electronic meters, basic calculators, and other electronic devices that display numerical information. History Seven-segment representation of figures can be found in patents as early as 1903 (in ), when Carl Kinsley invented a method of telegraphically transmitting letters and numbers and having them printed on tape in a segmented format. In 1908, F. W. Wood invented an 8-segment display, which displayed the number 4 using a diagonal bar (). In 1910, a seven-segment display illuminated by incandescent bulbs was used on a power-plant boiler room signal panel. They were also used to show the dialed telephone number to operators during the transition from manual to automatic telephone dialing. They did not achieve widespread use until the advent of LEDs in the 1970 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Digital Sundial
A digital sundial is a clock that indicates the current time with numerals formed by the sunlight striking it. Like a classical sundial, the device contains no moving parts. It uses no electricity nor other manufactured sources of energy. The digital display changes as the sun advances in its daily course. Technique There are two basic types of digital sundials. One type uses optical waveguides, while the other is inspired by fractal geometry. Optical fiber sundial Sunlight enters into the device through a slit and moves as the sun advances. The sun's rays shine on ten linearly distributed sockets of optical waveguides that transport the light to a seven-segment display. Each socket fiber is connected to a few segments forming the digit corresponding to the position of the sun. Fractal sundial The theoretical basis for the other construction comes from fractal geometry. For the sake of simplicity, we describe a two-dimensional (planar) version. Let denote a straight line p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fractal Geometry
In mathematics, a fractal is a geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scales, as illustrated in successive magnifications of the Mandelbrot set. This exhibition of similar patterns at increasingly smaller scales is called self-similarity, also known as expanding symmetry or unfolding symmetry; if this replication is exactly the same at every scale, as in the Menger sponge, the shape is called affine self-similar. Fractal geometry lies within the mathematical branch of measure theory. One way that fractals are different from finite geometric figures is how they scale. Doubling the edge lengths of a filled polygon multiplies its area by four, which is two (the ratio of the new to the old side length) raised to the power of two (the conventional dimension of the filled polygon). Likewise, if the radius of a filled sphere is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cartesian Coordinate System
A Cartesian coordinate system (, ) in a plane is a coordinate system that specifies each point uniquely by a pair of numerical coordinates, which are the signed distances to the point from two fixed perpendicular oriented lines, measured in the same unit of length. Each reference coordinate line is called a ''coordinate axis'' or just ''axis'' (plural ''axes'') of the system, and the point where they meet is its ''origin'', at ordered pair . The coordinates can also be defined as the positions of the perpendicular projections of the point onto the two axes, expressed as signed distances from the origin. One can use the same principle to specify the position of any point in three-dimensional space by three Cartesian coordinates, its signed distances to three mutually perpendicular planes (or, equivalently, by its perpendicular projection onto three mutually perpendicular lines). In general, ''n'' Cartesian coordinates (an element of real ''n''-space) specify the point in an ' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Measurable Set
In mathematics, the concept of a measure is a generalization and formalization of geometrical measures (length, area, volume) and other common notions, such as mass and probability of events. These seemingly distinct concepts have many similarities and can often be treated together in a single mathematical context. Measures are foundational in probability theory, integration theory, and can be generalized to assume negative values, as with electrical charge. Far-reaching generalizations (such as spectral measures and projection-valued measures) of measure are widely used in quantum physics and physics in general. The intuition behind this concept dates back to ancient Greece, when Archimedes tried to calculate the area of a circle. But it was not until the late 19th and early 20th centuries that measure theory became a branch of mathematics. The foundations of modern measure theory were laid in the works of Émile Borel, Henri Lebesgue, Nikolai Luzin, Johann Radon, Consta ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kenneth Falconer (mathematician)
Kenneth John Falconer FRSE (born 25 January 1952) is an English mathematician working in mathematical analysis and in particular on fractal geometry. He is Regius Professor of Mathematics in the School of Mathematics and Statistics at the University of St Andrews. He is known for his work on the mathematics of fractals and in particular sets and measures arising from iterated function systems, especially self-similar and self-affine sets. Closely related is his research on Hausdorff and other fractal dimensions. He formulated '' Falconer's conjecture'' on the dimension of distance sets and conceived the notion of a digital sundial. In combinatorial geometry he established a lower bound of 5 for the chromatic number of the plane in the Lebesgue measurable case. Falconer was born at Bearsted Memorial Maternity Hospital outside Hampton Court Palace. He was educated at Kingston Grammar School, Kingston upon Thames and Corpus Christi College, Cambridge. He graduated in 1974 and c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Scientific American
''Scientific American'', informally abbreviated ''SciAm'' or sometimes ''SA'', is an American popular science magazine. Many famous scientists, including Albert Einstein and Nikola Tesla, have contributed articles to it. In print since 1845, it is the oldest continuously published magazine in the United States. ''Scientific American'' is owned by Springer Nature, which in turn is a subsidiary of Holtzbrinck Publishing Group. History ''Scientific American'' was founded by inventor and publisher Rufus Porter (painter), Rufus Porter in 1845 as a four-page weekly newspaper. The first issue of the large format newspaper was released August 28, 1845. Throughout its early years, much emphasis was placed on reports of what was going on at the United States Patent and Trademark Office, U.S. Patent Office. It also reported on a broad range of inventions including perpetual motion machines, an 1860 device for buoying vessels by Abraham Lincoln, and the universal joint which now can be found ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ian Stewart (mathematician)
Ian Nicholas Stewart (born 24 September 1945) is a British mathematician and a popular-science and science-fiction writer. He is Emeritus Professor of Mathematics at the University of Warwick, England. Education and early life Stewart was born in 1945 in Folkestone, England. While in the sixth form at Harvey Grammar School in Folkestone he came to the attention of the mathematics teacher. The teacher had Stewart sit mock A-level examinations without any preparation along with the upper-sixth students; Stewart was placed first in the examination. He was awarded a scholarship to study at the University of Cambridge as an undergraduate student of Churchill College, Cambridge, where he studied the Mathematical Tripos and obtained a first-class Bachelor of Arts degree in mathematics in 1966. Stewart then went to the University of Warwick where his PhD on Lie algebras was supervised by Brian Hartley and completed in 1969. Career and research After his PhD, Stewart was offered an a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |