|
Coherent Turbulent Structures
Turbulent flows are complex multi-scale and chaotic motions that need to be classified into more elementary components, referred to coherent turbulent structures. Such a structure must have temporal coherence, i.e. it must persist in its form for long enough periods that the methods of time-averaged statistics can be applied. Coherent structures are typically studied on very large scales, but can be broken down into more elementary structures with coherent properties of their own, such examples include hairpin vortices. Hairpins and coherent structures have been studied and noticed in data since the 1930s, and have been since cited in thousands of scientific papers and reviews.#Green, Sheldon I., “Fluid Vortices: Fluid mechanics and its applications” Dordrecht: Kluwer Academic Publishers, 1995. Print. https://books.google.com/books?id=j6qE7YAwwCoC&pg=PA254&lpg=PA254&dq=theodorsen+1952+hairpin&source=bl&ots=S9f7BlMhkg&sig=0qx5dJdvceQf22gm0li0Rt7UtL4&hl=en&sa=X&ei=1gNcU8DyOJWuyASBz ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Turbulent Flow
In fluid dynamics, turbulence or turbulent flow is fluid motion characterized by chaotic changes in pressure and flow velocity. It is in contrast to a laminar flow, which occurs when a fluid flows in parallel layers, with no disruption between those layers. Turbulence is commonly observed in everyday phenomena such as surf, fast flowing rivers, billowing storm clouds, or smoke from a chimney, and most fluid flows occurring in nature or created in engineering applications are turbulent. Turbulence is caused by excessive kinetic energy in parts of a fluid flow, which overcomes the damping effect of the fluid's viscosity. For this reason turbulence is commonly realized in low viscosity fluids. In general terms, in turbulent flow, unsteady vortices appear of many sizes which interact with each other, consequently drag due to friction effects increases. This increases the energy needed to pump fluid through a pipe. The onset of turbulence can be predicted by the dimensionless Reyn ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ellen Longmire
Ellen Kathryn Longmire is an American applied physicist and mechanical engineer known for her research in experimental fluid dynamics and turbulence. She is a professor of aerospace engineering and mechanics at the University of Minnesota, where she is also Associate Dean for Academic Affairs in the College of Science & Engineering,, the former chair of the American Physical Society Division of Fluid Dynamics, and one of three editors-in-chief of the journal ''Experiments in Fluids''. Education and career Longmire majored in physics at Princeton University, graduating in 1982. After a year at the Technical University of Braunschweig The Technische Universität Braunschweig (unofficially University of Braunschweig – Institute of Technology), commonly referred to as TU Braunschweig, is the oldest ' (comparable to an institute of technology in the American system) in Germany. ..., and work in industry at Honeywell, Hauni-Werke Koerber, and Science Applications International, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
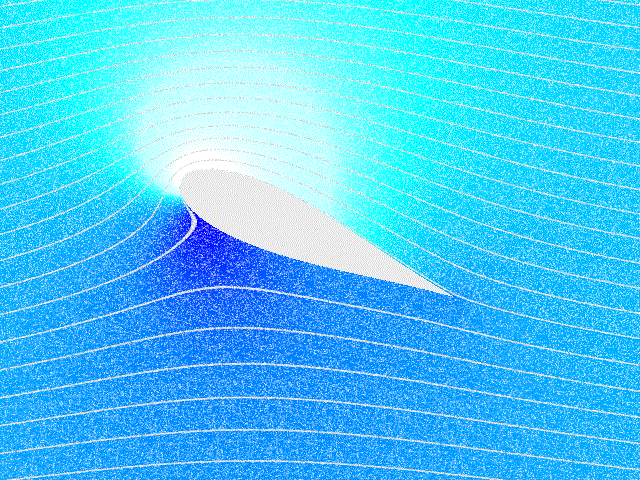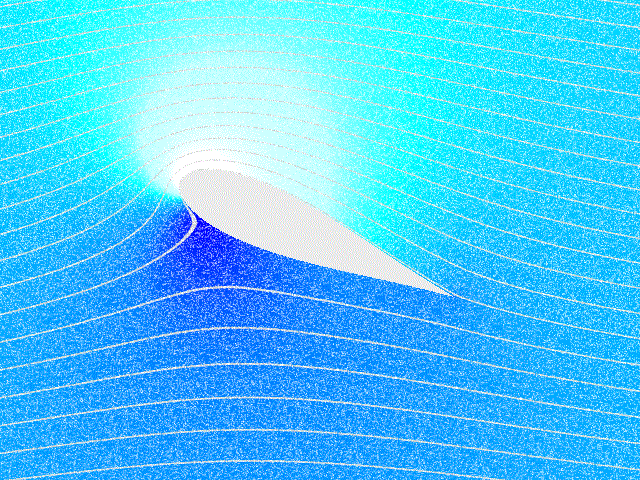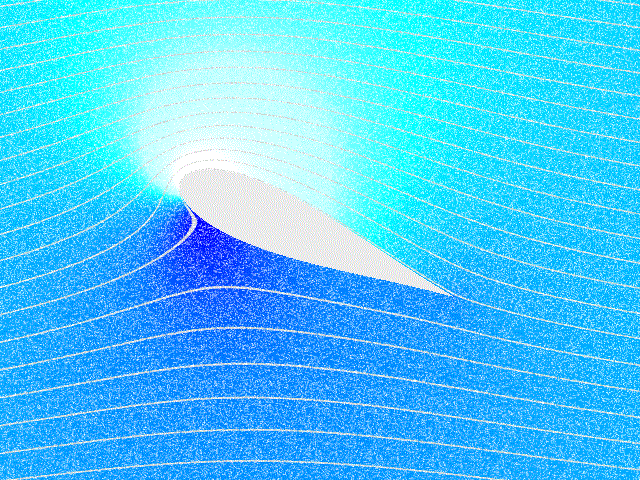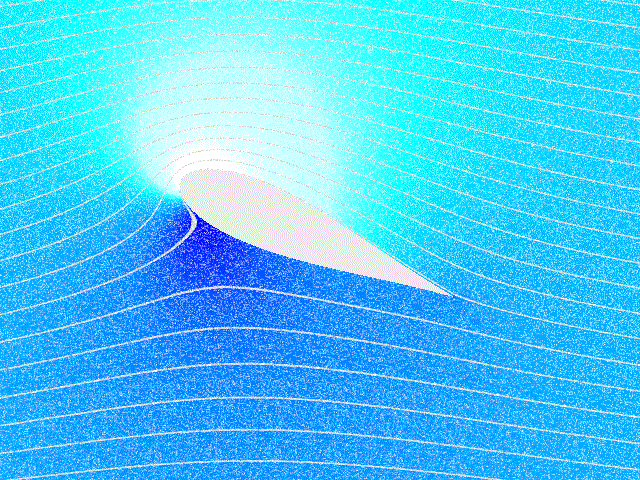
Aerodynamics
Aerodynamics, from grc, ἀήρ ''aero'' (air) + grc, δυναμική (dynamics), is the study of the motion of air, particularly when affected by a solid object, such as an airplane wing. It involves topics covered in the field of fluid dynamics and its subfield of gas dynamics. The term ''aerodynamics'' is often used synonymously with gas dynamics, the difference being that "gas dynamics" applies to the study of the motion of all gases, and is not limited to air. The formal study of aerodynamics began in the modern sense in the eighteenth century, although observations of fundamental concepts such as aerodynamic drag were recorded much earlier. Most of the early efforts in aerodynamics were directed toward achieving Aircraft#Heavier than air – aerodynes, heavier-than-air flight, which was first demonstrated by Otto Lilienthal in 1891. Since then, the use of aerodynamics through mathematical analysis, empirical approximations, wind tunnel experimentation, and computer simu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Direct Numerical Simulation
A direct numerical simulation (DNS)Here the origin of the term ''direct numerical simulation'' (see e.g. p. 385 in ) owes to the fact that, at that time, there were considered to be just two principal ways of getting ''theoretical'' results regarding turbulence, namely via turbulence theories (like the direct interaction approximation) and ''directly'' from solution of the Navier–Stokes equations. is a simulation in computational fluid dynamics (CFD) in which the Navier–Stokes equations are numerically solved without any turbulence model. This means that the whole range of spatial and temporal scales of the turbulence must be resolved. All the spatial scales of the turbulence must be resolved in the computational mesh, from the smallest dissipative scales (Kolmogorov microscales), up to the integral scale L, associated with the motions containing most of the kinetic energy. The Kolmogorov scale, \eta, is given by :\eta=(\nu^/\varepsilon)^ where \nu is the kinematic visco ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
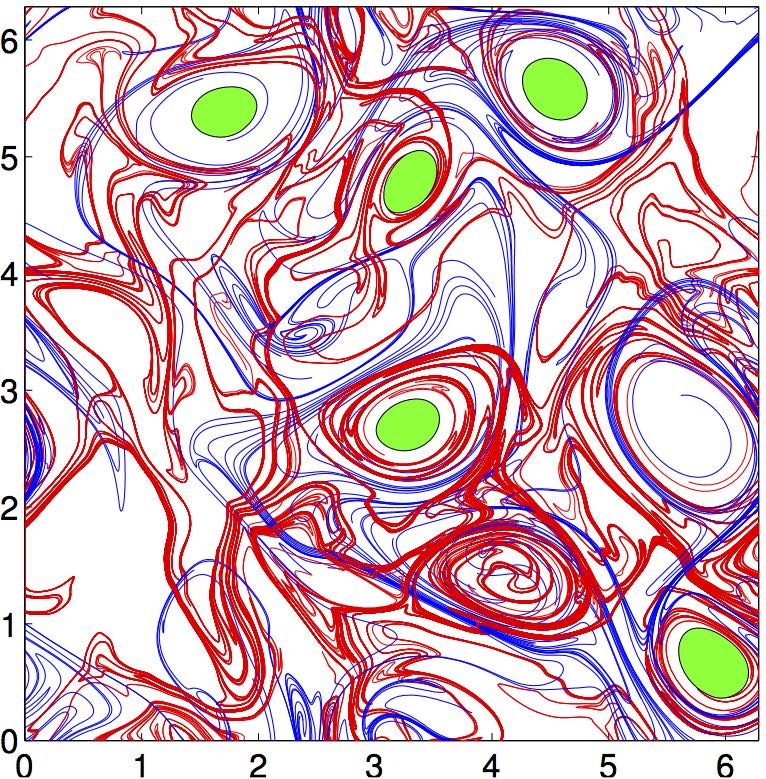
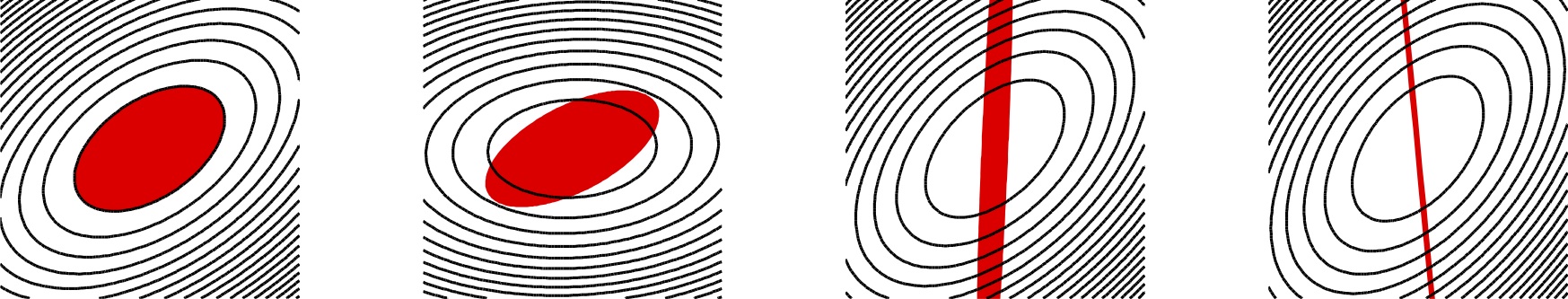
Lagrangian Coherent Structure
Lagrangian coherent structures (LCSs) are distinguished surfaces of trajectories in a dynamical system that exert a major influence on nearby trajectories over a time interval of interest. The type of this influence may vary, but it invariably creates a coherent trajectory pattern for which the underlying LCS serves as a theoretical centerpiece. In observations of tracer patterns in nature, one readily identifies coherent features, but it is often the underlying structure creating these features that is of interest. As illustrated on the right, individual tracer trajectories forming coherent patterns are generally sensitive with respect to changes in their initial conditions and the system parameters. In contrast, the LCSs creating these trajectory patterns turn out to be robust and provide a simplified skeleton of the overall dynamics of the system. The robustness of this skeleton makes LCSs ideal tools for model validation, model comparison and benchmarking. LCSs can also be used ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euler Equations (fluid Dynamics)
In fluid dynamics, the Euler equations are a set of partial differential equations governing adiabatic and inviscid flow. They are named after Leonhard Euler. In particular, they correspond to the Navier–Stokes equations with zero viscosity and zero thermal conductivity. The Euler equations can be applied to incompressible and compressible flows. The incompressible Euler equations consist of Cauchy equations for conservation of mass and balance of momentum, together with the incompressibility condition that the flow velocity is a solenoidal field. The compressible Euler equations consist of equations for conservation of mass, balance of momentum, and balance of energy, together with a suitable constitutive equation for the specific energy density of the fluid. Historically, only the equations of conservation of mass and balance of momentum were derived by Euler. However, fluid dynamics literature often refers to the full set of the compressible Euler equations – including ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dynamical Systems
In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, the random motion of particles in the air, and the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a set, without the need of a smooth space-time structure defined on it. At any given time, a dynamical system has a state representing a point in an appropriate state space. This state is often given by a tuple of real numbers or by a vector in a geometrical manif ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lagrangian Coherent Structures
Lagrangian coherent structures (LCSs) are distinguished surfaces of trajectories in a dynamical system that exert a major influence on nearby trajectories over a time interval of interest. The type of this influence may vary, but it invariably creates a coherent trajectory pattern for which the underlying LCS serves as a theoretical centerpiece. In observations of tracer patterns in nature, one readily identifies coherent features, but it is often the underlying structure creating these features that is of interest. As illustrated on the right, individual tracer trajectories forming coherent patterns are generally sensitive with respect to changes in their initial conditions and the system parameters. In contrast, the LCSs creating these trajectory patterns turn out to be robust and provide a simplified skeleton of the overall dynamics of the system. The robustness of this skeleton makes LCSs ideal tools for model validation, model comparison and benchmarking. LCSs can also be used ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Skeleton Turbulence
A skeleton is the structural frame that supports the body of an animal. There are several types of skeletons, including the exoskeleton, which is the stable outer shell of an organism, the endoskeleton, which forms the support structure inside the body, and the hydroskeleton, a flexible internal skeleton supported by fluid pressure. Vertebrates are animals with a vertebral column, and their skeletons are typically composed of bone and cartilage. Invertebrates are animals that lack a vertebral column. The skeletons of invertebrates vary, including hard exoskeleton shells, plated endoskeletons, or Sponge spicule, spicules. Cartilage is a rigid connective tissue that is found in the skeletal systems of vertebrates and invertebrates. Etymology The term ''skeleton'' comes . ''Sceleton'' is an archaic form of the word. Classification Skeletons can be defined by several attributes. Solid skeletons consist of hard substances, such as bone, cartilage, or cuticle. These can be further ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Laminar Flow
Laminar flow () is the property of fluid particles in fluid dynamics to follow smooth paths in layers, with each layer moving smoothly past the adjacent layers with little or no mixing. At low velocities, the fluid tends to flow without lateral mixing, and adjacent layers slide past one another smoothly. There are no cross-currents perpendicular to the direction of flow, nor eddies or swirls of fluids. In laminar flow, the motion of the particles of the fluid is very orderly with particles close to a solid surface moving in straight lines parallel to that surface. Laminar flow is a flow regime characterized by high momentum diffusion and low momentum convection. When a fluid is flowing through a closed channel such as a pipe or between two flat plates, either of two types of flow may occur depending on the velocity and viscosity of the fluid: laminar flow or turbulent flow. Laminar flow occurs at lower velocities, below a threshold at which the flow becomes turbulent. The thresho ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kelvin–Helmholtz Instability
The Kelvin–Helmholtz instability (after Lord Kelvin and Hermann von Helmholtz) is a fluid instability that occurs when there is velocity shear in a single continuous fluid or a velocity difference across the interface between two fluids. Kelvin-Helmholtz instabilities are visible in the atmospheres of planets and moons, such as in cloud formations on Earth or the Red Spot on Jupiter, and the atmospheres of the Sun and other stars. Theory overview and mathematical concepts Fluid dynamics predicts the onset of instability and transition to turbulent flow within fluids of different densities moving at different speeds. If surface tension is ignored, two fluids in parallel motion with different velocities and densities yield an interface that is unstable to short-wavelength perturbations for all speeds. However, surface tension is able to stabilize the short wavelength instability up to a threshold velocity. If the density and velocity vary continuously in space (wi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |