|
Birational Map
In mathematics, birational geometry is a field of algebraic geometry in which the goal is to determine when two algebraic varieties are isomorphic outside lower-dimensional subsets. This amounts to studying mappings that are given by rational functions rather than polynomials; the map may fail to be defined where the rational functions have poles. Birational maps Rational maps A rational map from one variety (understood to be irreducible) X to another variety Y, written as a dashed arrow , is defined as a morphism from a nonempty open subset U \subset X to Y. By definition of the Zariski topology used in algebraic geometry, a nonempty open subset U is always dense in X, in fact the complement of a lower-dimensional subset. Concretely, a rational map can be written in coordinates using rational functions. Birational maps A birational map from ''X'' to ''Y'' is a rational map such that there is a rational map inverse to ''f''. A birational map induces an isomorphism from a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
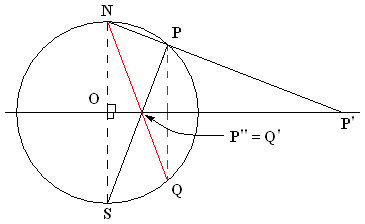
Stereographic Projection
In mathematics, a stereographic projection is a perspective projection of the sphere, through a specific point on the sphere (the ''pole'' or ''center of projection''), onto a plane (geometry), plane (the ''projection plane'') perpendicular to the diameter through the point. It is a smooth function, smooth, bijection, bijective function (mathematics), function from the entire sphere except the center of projection to the entire plane. It maps circle of a sphere, circles on the sphere to generalised circle, circles or lines on the plane, and is conformal map, conformal, meaning that it preserves angles at which curves meet and thus Local property, locally approximately preserves similarity (geometry), shapes. It is neither isometry, isometric (distance preserving) nor Equiareal map, equiareal (area preserving). The stereographic projection gives a way to representation (mathematics), represent a sphere by a plane. The metric tensor, metric induced metric, induced by the inverse s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Italian School Of Algebraic Geometry
In relation to the history of mathematics, the Italian school of algebraic geometry refers to mathematicians and their work in birational geometry, particularly on algebraic surfaces, centered around Rome roughly from 1885 to 1935. There were 30 to 40 leading mathematicians who made major contributions, about half of those being Italian. The leadership fell to the group in Rome of Guido Castelnuovo, Federigo Enriques and Francesco Severi, who were involved in some of the deepest discoveries, as well as setting the style. Algebraic surfaces The emphasis on algebraic surfaces—algebraic varieties of dimension two—followed on from an essentially complete geometric theory of algebraic curves (dimension 1). The position in around 1870 was that the curve theory had incorporated with Brill–Noether theory the Riemann–Roch theorem in all its refinements (via the detailed geometry of the theta-divisor). The classification of algebraic surfaces was a bold and successful att ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nef Line Bundle
In algebraic geometry, a line bundle on a projective variety is nef if it has nonnegative degree on every curve in the variety. The classes of nef line bundles are described by a convex cone, and the possible contractions of the variety correspond to certain faces of the nef cone. In view of the correspondence between line bundles and divisors (built from codimension-1 subvarieties), there is an equivalent notion of a nef divisor. Definition More generally, a line bundle ''L'' on a proper scheme ''X'' over a field ''k'' is said to be nef if it has nonnegative degree on every (closed irreducible) curve in ''X''. (The degree of a line bundle ''L'' on a proper curve ''C'' over ''k'' is the degree of the divisor (''s'') of any nonzero rational section ''s'' of ''L''.) A line bundle may also be called an invertible sheaf. The term "nef" was introduced by Miles Reid as a replacement for the older terms "arithmetically effective" and "numerically effective", as well as for the phrase "num ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Canonical Bundle
In mathematics, the canonical bundle of a non-singular algebraic variety V of dimension n over a field is the line bundle \,\!\Omega^n = \omega, which is the ''n''th exterior power of the cotangent bundle Ω on ''V''. Over the complex numbers, it is the determinant bundle of holomorphic ''n''-forms on ''V''. This is the dualising object for Serre duality on ''V''. It may equally well be considered as an invertible sheaf. The canonical class is the divisor class of a Cartier divisor ''K'' on ''V'' giving rise to the canonical bundle — it is an equivalence class for linear equivalence on ''V'', and any divisor in it may be called a canonical divisor. An anticanonical divisor is any divisor −''K'' with ''K'' canonical. The anticanonical bundle is the corresponding inverse bundle ω−1. When the anticanonical bundle of V is ample, V is called a Fano variety. The adjunction formula Suppose that ''X'' is a smooth variety and that ''D'' is a smooth divisor on ''X'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Minimal Model Program
In algebraic geometry, the minimal model program is part of the birational classification of algebraic varieties. Its goal is to construct a birational model of any complex projective variety which is as simple as possible. The subject has its origins in the classical birational geometry of surfaces studied by the Italian school, and is currently an active research area within algebraic geometry. Outline The basic idea of the theory is to simplify the birational classification of varieties by finding, in each birational equivalence class, a variety which is "as simple as possible". The precise meaning of this phrase has evolved with the development of the subject; originally for surfaces, it meant finding a smooth variety X for which any birational morphism f\colon X \to X' with a smooth surface X' is an isomorphism. In the modern formulation, the goal of the theory is as follows. Suppose we are given a projective variety X, which for simplicity is assumed non-singular. There are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Betti Number
In algebraic topology, the Betti numbers are used to distinguish topological spaces based on the connectivity of ''n''-dimensional simplicial complexes. For the most reasonable finite-dimensional spaces (such as compact manifolds, finite simplicial complexes or CW complexes), the sequence of Betti numbers is 0 from some point onward (Betti numbers vanish above the dimension of a space), and they are all finite. The ''n''th Betti number represents the rank of the ''n''th homology group, denoted ''H''''n'', which tells us the maximum number of cuts that can be made before separating a surface into two pieces or 0-cycles, 1-cycles, etc. For example, if H_n(X) \cong 0 then b_n(X) = 0, if H_n(X) \cong \mathbb then b_n(X) = 1, if H_n(X) \cong \mathbb \oplus \mathbb then b_n(X) = 2, if H_n(X) \cong \mathbb \oplus \mathbb\oplus \mathbb then b_n(X) = 3, etc. Note that only the ranks of infinite groups are considered, so for example if H_n(X) \cong \mathbb^k \oplus \mathbb/(2) , where \mat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Blowing Up
In mathematics, blowing up or blowup is a type of geometric transformation which replaces a subspace of a given space with all the directions pointing out of that subspace. For example, the blowup of a point in a plane replaces the point with the projectivized tangent space at that point. The metaphor is that of zooming in on a photograph to enlarge part of the picture, rather than referring to an explosion. Blowups are the most fundamental transformation in birational geometry, because every birational morphism between projective varieties is a blowup. The weak factorization theorem says that every birational map can be factored as a composition of particularly simple blowups. The Cremona group, the group of birational automorphisms of the plane, is generated by blowups. Besides their importance in describing birational transformations, blowups are also an important way of constructing new spaces. For instance, most procedures for resolution of singularities proceed by bl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Singular Point Of An Algebraic Variety
In the mathematical field of algebraic geometry, a singular point of an algebraic variety is a point that is 'special' (so, singular), in the geometric sense that at this point the tangent space at the variety may not be regularly defined. In case of varieties defined over the reals, this notion generalizes the notion of local non-flatness. A point of an algebraic variety which is not singular is said to be regular. An algebraic variety which has no singular point is said to be non-singular or smooth. Definition A plane curve defined by an implicit equation :F(x,y)=0, where is a smooth function is said to be ''singular'' at a point if the Taylor series of has order at least at this point. The reason for this is that, in differential calculus, the tangent at the point of such a curve is defined by the equation :(x-x_0)F'_x(x_0,y_0) + (y-y_0)F'_y(x_0,y_0)=0, whose left-hand side is the term of degree one of the Taylor expansion. Thus, if this term is zero, the tangent may ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Resolution Of Singularities
In algebraic geometry, the problem of resolution of singularities asks whether every algebraic variety ''V'' has a resolution, a non-singular variety ''W'' with a proper birational map ''W''→''V''. For varieties over fields of characteristic 0 this was proved in Hironaka (1964), while for varieties over fields of characteristic ''p'' it is an open problem in dimensions at least 4. Definitions Originally the problem of resolution of singularities was to find a nonsingular model for the function field of a variety ''X'', in other words a complete non-singular variety ''X′'' with the same function field. In practice it is more convenient to ask for a different condition as follows: a variety ''X'' has a resolution of singularities if we can find a non-singular variety ''X′'' and a proper birational map from ''X′'' to ''X''. The condition that the map is proper is needed to exclude trivial solutions, such as taking ''X′'' to be the subvariety of non- ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heisuke Hironaka
is a Japanese mathematician who was awarded the Fields Medal in 1970 for his contributions to algebraic geometry. Career Hironaka entered Kyoto University in 1949. After completing his undergraduate studies at Kyoto University, he received his Ph.D. in 1960 from Harvard University while under the direction of Oscar Zariski. Hironaka held teaching positions at Brandeis University from 1960-1963, Columbia University in 1964, and Kyoto University from 1975 to 1988. He was a professor of mathematics at Harvard University from 1968 until becoming ''emeritus'' in 1992 and was a president of Yamaguchi University from 1996 to 2002. Research In 1964, Hironaka proved that singularities of algebraic varieties admit resolutions in characteristic zero. This means that any algebraic variety can be replaced by (more precisely is birationally equivalent to) a similar variety which has no singularities. He also introduced Hironaka's example showing that a deformation of Kähler manifolds need ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chow's Lemma
Chow's lemma, named after Wei-Liang Chow, is one of the foundational results in algebraic geometry. It roughly says that a proper morphism is fairly close to being a projective morphism. More precisely, a version of it states the following: :If X is a scheme that is proper over a noetherian base S, then there exists a projective S-scheme X' and a surjective S-morphism f: X' \to X that induces an isomorphism f^(U) \simeq U for some dense open U\subseteq X. Proof The proof here is a standard one. Reduction to the case of X irreducible We can first reduce to the case where X is irreducible. To start, X is noetherian since it is of finite type over a noetherian base. Therefore it has finitely many irreducible components X_i, and we claim that for each X_i there is an irreducible proper S-scheme Y_i so that Y_i\to X has set-theoretic image X_i and is an isomorphism on the open dense subset X_i\setminus \cup_ X_j of X_i. To see this, define Y_i to be the scheme-theoretic image of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
