|
Asymptotes
In analytic geometry, an asymptote () of a curve is a line such that the distance between the curve and the line approaches zero as one or both of the ''x'' or ''y'' coordinates tends to infinity. In projective geometry and related contexts, an asymptote of a curve is a line which is tangent to the curve at a point at infinity. The word asymptote is derived from the Greek ἀσύμπτωτος (''asumptōtos'') which means "not falling together", from ἀ priv. + σύν "together" + πτωτ-ός "fallen". The term was introduced by Apollonius of Perga in his work on conic sections, but in contrast to its modern meaning, he used it to mean any line that does not intersect the given curve. There are three kinds of asymptotes: ''horizontal'', ''vertical'' and ''oblique''. For curves given by the graph of a function , horizontal asymptotes are horizontal lines that the graph of the function approaches as ''x'' tends to Vertical asymptotes are vertical lines near which the fun ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Asymptotic Curve Hvo1
In analytic geometry, an asymptote () of a curve is a line such that the distance between the curve and the line approaches zero as one or both of the ''x'' or ''y'' coordinates Limit of a function#Limits at infinity, tends to infinity. In projective geometry and related contexts, an asymptote of a curve is a line which is tangent to the curve at a point at infinity. The word asymptote is derived from the Greek language, Greek ἀσύμπτωτος (''asumptōtos'') which means "not falling together", from ἀ Privative alpha, priv. + σύν "together" + πτωτ-ός "fallen". The term was introduced by Apollonius of Perga in his work on conic sections, but in contrast to its modern meaning, he used it to mean any line that does not intersect the given curve. There are three kinds of asymptotes: ''horizontal'', ''vertical'' and ''oblique''. For curves given by the graph of a function, graph of a function (mathematics), function , horizontal asymptotes are horizontal lines tha ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Asymptote02 Vectorial
In analytic geometry, an asymptote () of a curve is a line such that the distance between the curve and the line approaches zero as one or both of the ''x'' or ''y'' coordinates tends to infinity. In projective geometry and related contexts, an asymptote of a curve is a line which is tangent to the curve at a point at infinity. The word asymptote is derived from the Greek ἀσύμπτωτος (''asumptōtos'') which means "not falling together", from ἀ priv. + σύν "together" + πτωτ-ός "fallen". The term was introduced by Apollonius of Perga in his work on conic sections, but in contrast to its modern meaning, he used it to mean any line that does not intersect the given curve. There are three kinds of asymptotes: ''horizontal'', ''vertical'' and ''oblique''. For curves given by the graph of a function , horizontal asymptotes are horizontal lines that the graph of the function approaches as ''x'' tends to Vertical asymptotes are vertical lines near which the fun ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Asymptote03
In analytic geometry, an asymptote () of a curve is a line such that the distance between the curve and the line approaches zero as one or both of the ''x'' or ''y'' coordinates tends to infinity. In projective geometry and related contexts, an asymptote of a curve is a line which is tangent to the curve at a point at infinity. The word asymptote is derived from the Greek ἀσύμπτωτος (''asumptōtos'') which means "not falling together", from ἀ priv. + σύν "together" + πτωτ-ός "fallen". The term was introduced by Apollonius of Perga in his work on conic sections, but in contrast to its modern meaning, he used it to mean any line that does not intersect the given curve. There are three kinds of asymptotes: ''horizontal'', ''vertical'' and ''oblique''. For curves given by the graph of a function , horizontal asymptotes are horizontal lines that the graph of the function approaches as ''x'' tends to Vertical asymptotes are vertical lines near which the fu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
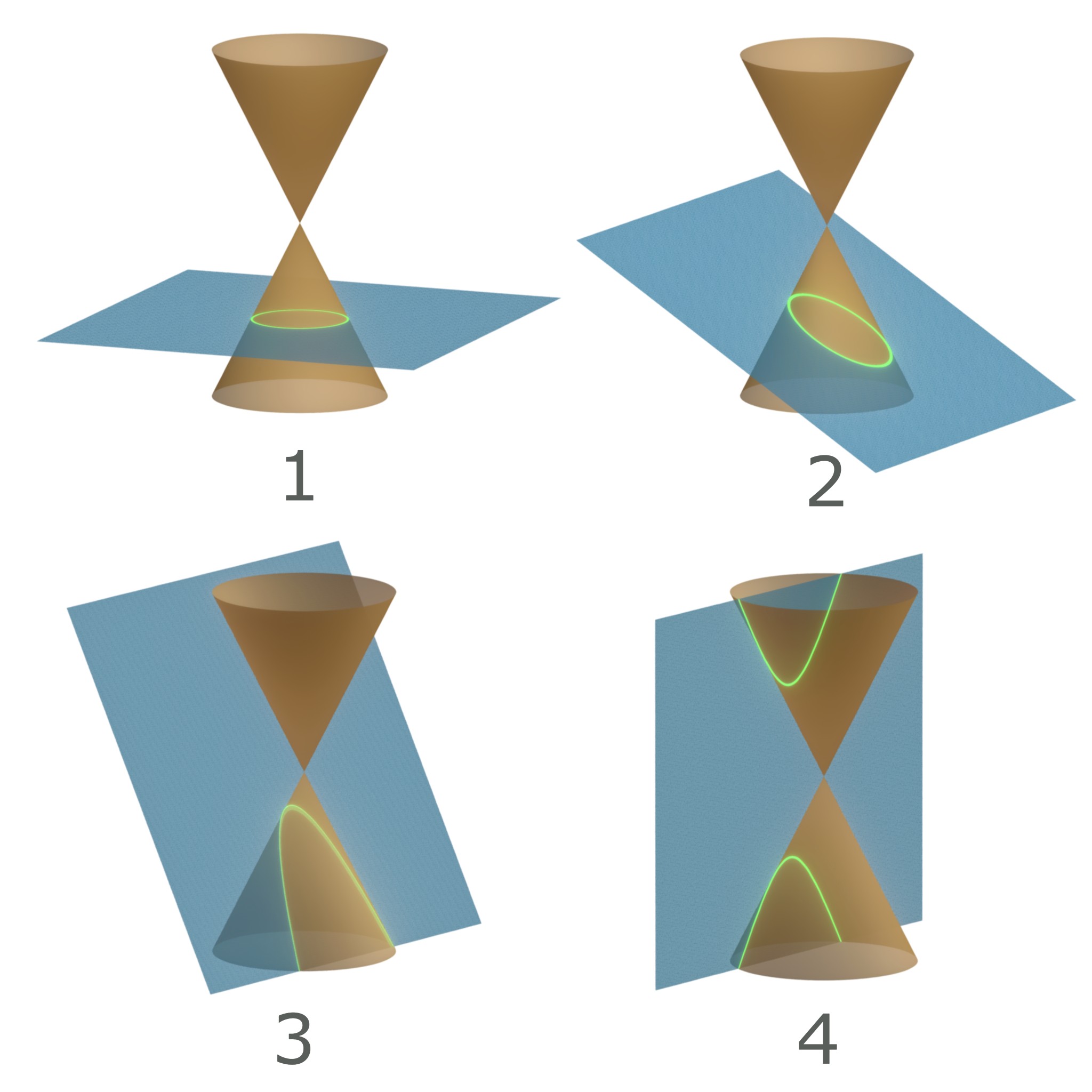
Hyperbola
In mathematics, a hyperbola (; pl. hyperbolas or hyperbolae ; adj. hyperbolic ) is a type of smooth curve lying in a plane, defined by its geometric properties or by equations for which it is the solution set. A hyperbola has two pieces, called connected components or branches, that are mirror images of each other and resemble two infinite bows. The hyperbola is one of the three kinds of conic section, formed by the intersection of a plane and a double cone. (The other conic sections are the parabola and the ellipse. A circle is a special case of an ellipse.) If the plane intersects both halves of the double cone but does not pass through the apex of the cones, then the conic is a hyperbola. Hyperbolas arise in many ways: * as the curve representing the reciprocal function y(x) = 1/x in the Cartesian plane, * as the path followed by the shadow of the tip of a sundial, * as the shape of an open orbit (as distinct from a closed elliptical orbit), such as the orbit of a s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hyperbola One Over X
In mathematics, a hyperbola (; pl. hyperbolas or hyperbolae ; adj. hyperbolic ) is a type of smooth function, smooth plane curve, curve lying in a plane, defined by its geometric properties or by equations for which it is the solution set. A hyperbola has two pieces, called Component (graph theory), connected components or branches, that are mirror images of each other and resemble two infinite bow (weapon), bows. The hyperbola is one of the three kinds of conic section, formed by the intersection of a plane (mathematics), plane and a double cone (geometry), cone. (The other conic sections are the parabola and the ellipse. A circle is a special case of an ellipse.) If the plane intersects both halves of the double cone but does not pass through the apex of the cones, then the conic is a hyperbola. Hyperbolas arise in many ways: * as the curve representing the multiplicative inverse, reciprocal function y(x) = 1/x in the Cartesian coordinate system, Cartesian plane, * as the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conic Sections
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of deg ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Limit Of A Function
Although the function (sin ''x'')/''x'' is not defined at zero, as ''x'' becomes closer and closer to zero, (sin ''x'')/''x'' becomes arbitrarily close to 1. In other words, the limit of (sin ''x'')/''x'', as ''x'' approaches zero, equals 1. In mathematics, the limit of a function is a fundamental concept in calculus and analysis concerning the behavior of that function near a particular input. Formal definitions, first devised in the early 19th century, are given below. Informally, a function ''f'' assigns an output ''f''(''x'') to every input ''x''. We say that the function has a limit ''L'' at an input ''p,'' if ''f''(''x'') gets closer and closer to ''L'' as ''x'' moves closer and closer to ''p''. More specifically, when ''f'' is applied to any input ''sufficiently'' close to ''p'', the output value is forced ''arbitrarily'' close to ''L''. On the other hand, if some inputs very close to ''p'' are taken to outputs that stay a fixed distance apart, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Apollonius Of Perga
Apollonius of Perga ( grc-gre, Ἀπολλώνιος ὁ Περγαῖος, Apollṓnios ho Pergaîos; la, Apollonius Pergaeus; ) was an Ancient Greek geometer and astronomer known for his work on conic sections. Beginning from the contributions of Euclid and Archimedes on the topic, he brought them to the state prior to the invention of analytic geometry. His definitions of the terms ellipse, parabola, and hyperbola are the ones in use today. Gottfried Wilhelm Leibniz stated “He who understands Archimedes and Apollonius will admire less the achievements of the foremost men of later times.” Apollonius worked on numerous other topics, including astronomy. Most of this work has not survived, where exceptions are typically fragments referenced by other authors like Pappus of Alexandria. His hypothesis of eccentric orbits to explain the apparently aberrant motion of the planets, commonly believed until the Middle Ages, was superseded during the Renaissance. The Apollonius crat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Asymptotic Analysis
In mathematical analysis, asymptotic analysis, also known as asymptotics, is a method of describing limiting behavior. As an illustration, suppose that we are interested in the properties of a function as becomes very large. If , then as becomes very large, the term becomes insignificant compared to . The function is said to be "''asymptotically equivalent'' to , as ". This is often written symbolically as , which is read as " is asymptotic to ". An example of an important asymptotic result is the prime number theorem. Let denote the prime-counting function (which is not directly related to the constant pi), i.e. is the number of prime numbers that are less than or equal to . Then the theorem states that \pi(x)\sim\frac. Asymptotic analysis is commonly used in computer science as part of the analysis of algorithms and is often expressed there in terms of big O notation. Definition Formally, given functions and , we define a binary relation f(x) \sim g(x) \qu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projective Geometry
In mathematics, projective geometry is the study of geometric properties that are invariant with respect to projective transformations. This means that, compared to elementary Euclidean geometry, projective geometry has a different setting, projective space, and a selective set of basic geometric concepts. The basic intuitions are that projective space has more points than Euclidean space, for a given dimension, and that geometric transformations are permitted that transform the extra points (called "points at infinity") to Euclidean points, and vice-versa. Properties meaningful for projective geometry are respected by this new idea of transformation, which is more radical in its effects than can be expressed by a transformation matrix and translations (the affine transformations). The first issue for geometers is what kind of geometry is adequate for a novel situation. It is not possible to refer to angles in projective geometry as it is in Euclidean geometry, because angle is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vertical Line Test
In mathematics, the vertical line test is a visual way to determine if a curve is a graph of a function In mathematics, the graph of a function f is the set of ordered pairs (x, y), where f(x) = y. In the common case where x and f(x) are real numbers, these pairs are Cartesian coordinates of points in two-dimensional space and thus form a subset ... or not. A function can only have one output, ''y'', for each unique input, ''x''. If a vertical line intersects a curve on an ''xy''-plane more than once then for one value of ''x'' the curve has more than one value of ''y'', and so, the curve does not represent a function. If all vertical lines intersect a curve at most once then the curve represents a function. See also * Horizontal line test Notes {{Reflist Functions and mappings ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |