|
Area-preserving Flow
In differential geometry, an equiareal map, sometimes called an authalic map, is a smooth map from one surface (mathematics), surface to another that preserves the areas of figures. Properties If ''M'' and ''N'' are two Riemannian manifold, Riemannian (or Pseudo-Riemannian manifold, pseudo-Riemannian) surfaces, then an equiareal map ''f'' from ''M'' to ''N'' can be characterized by any of the following equivalent conditions: * The surface area of ''f''(''U'') is equal to the area of ''U'' for every open set ''U'' on ''M''. * The pullback (differential geometry), pullback of the volume element, area element ''μ''''N'' on ''N'' is equal to ''μ''''M'', the area element on ''M''. * At each point ''p'' of ''M'', and tangent vectors ''v'' and ''w'' to ''M'' at ''p'',\bigl, df_p(v)\wedge df_p(w)\bigr, = , v\wedge w, \,where \wedge denotes the Euclidean Exterior algebra, wedge product of vectors and ''df'' denotes the pushforward (differential), pushforward along ''f''. Example An exam ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Differential Geometry
Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces, otherwise known as smooth manifolds. It uses the techniques of differential calculus, integral calculus, linear algebra and multilinear algebra. The field has its origins in the study of spherical geometry as far back as antiquity. It also relates to astronomy, the geodesy of the Earth, and later the study of hyperbolic geometry by Lobachevsky. The simplest examples of smooth spaces are the plane and space curves and surfaces in the three-dimensional Euclidean space, and the study of these shapes formed the basis for development of modern differential geometry during the 18th and 19th centuries. Since the late 19th century, differential geometry has grown into a field concerned more generally with geometric structures on differentiable manifolds. A geometric structure is one which defines some notion of size, distance, shape, volume, or other rigidifying structu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euclidean Plane
In mathematics, the Euclidean plane is a Euclidean space of dimension two. That is, a geometric setting in which two real quantities are required to determine the position of each point ( element of the plane), which includes affine notions of parallel lines, and also metrical notions of distance, circles, and angle measurement. The set \mathbb^2 of pairs of real numbers (the real coordinate plane) augmented by appropriate structure often serves as the canonical example. History Books I through IV and VI of Euclid's Elements dealt with two-dimensional geometry, developing such notions as similarity of shapes, the Pythagorean theorem (Proposition 47), equality of angles and areas, parallelism, the sum of the angles in a triangle, and the three cases in which triangles are "equal" (have the same area), among many other topics. Later, the plane was described in a so-called '' Cartesian coordinate system'', a coordinate system that specifies each point uniquely in a plane by a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jacobian Matrix And Determinant
In vector calculus, the Jacobian matrix (, ) of a vector-valued function of several variables is the matrix of all its first-order partial derivatives. When this matrix is square, that is, when the function takes the same number of variables as input as the number of vector components of its output, its determinant is referred to as the Jacobian determinant. Both the matrix and (if applicable) the determinant are often referred to simply as the Jacobian in literature. Suppose is a function such that each of its first-order partial derivatives exist on . This function takes a point as input and produces the vector as output. Then the Jacobian matrix of is defined to be an matrix, denoted by , whose th entry is \mathbf J_ = \frac, or explicitly :\mathbf J = \begin \dfrac & \cdots & \dfrac \end = \begin \nabla^ f_1 \\ \vdots \\ \nabla^ f_m \end = \begin \dfrac & \cdots & \dfrac\\ \vdots & \ddots & \vdots\\ \dfrac & \cdots ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
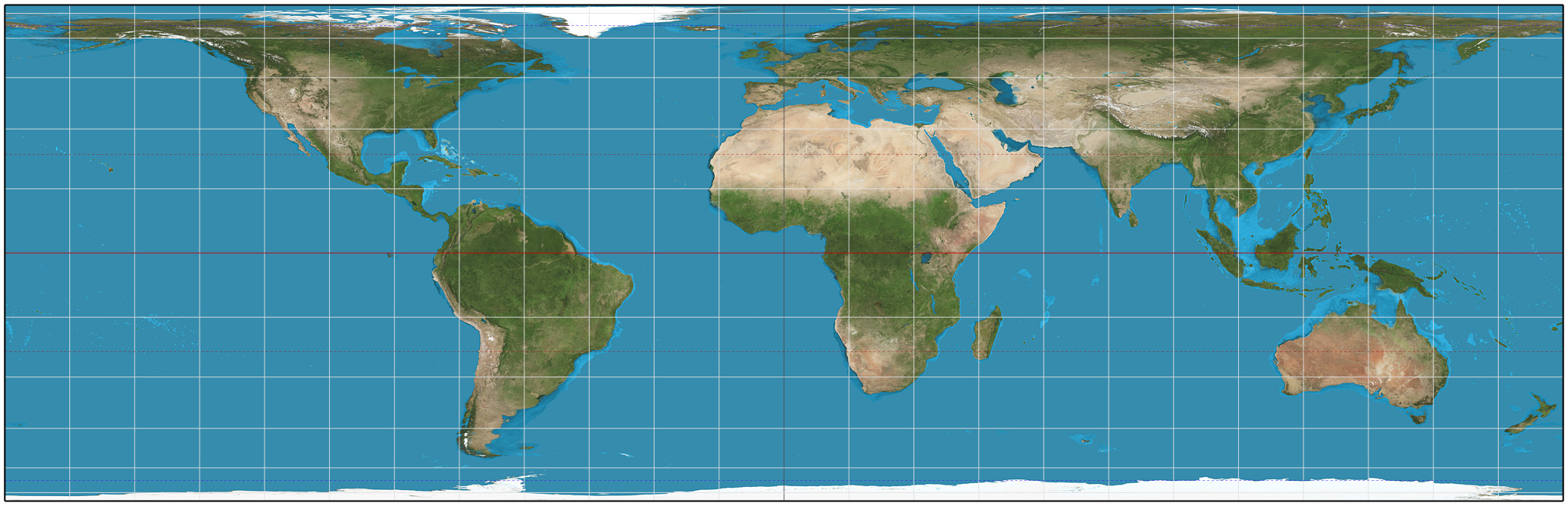
Equal-area Map Projection
In cartography, an equal-area projection is a map projection that preserves area measure, generally distorting shapes in order to do that. Equal-area maps are also called equivalent or authalic. An equal-area map projection cannot be conformal, nor can a conformal map projection be equal-area. Several equivalent projections were developed in an attempt to minimize the distortion of countries and continents of planet Earth, keeping the area constant. Equivalent projections are widely used for thematic maps showing scenario distribution such as population, farmland distribution, forested areas, etc. Description Equal area representation implies that a region of interest in a particular portion of the map will share the same proportion of area as in any other part of the map. Statistical grid The term "statistical grid" refers to a discrete grid (global or local) of an equal-area surface representation, used for data visualization, geocode and statistical spatial analysis. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
