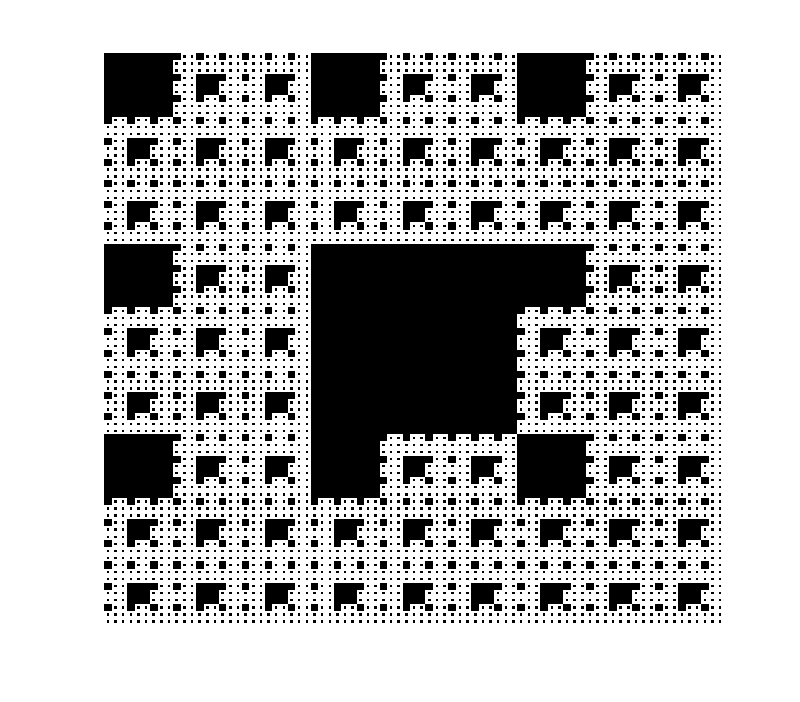
Vicsek Fractal
In mathematics the Vicsek fractal, also known as Vicsek snowflake or box fractal, is a fractal arising from a construction similar to that of the Sierpiński carpet, proposed by Tamás Vicsek. It has applications including as compact fractal antenna, antennas, particularly in cellular phones. ''Box fractal'' also refers to various iterated fractals created by a square tiling, square or rectangular grid with various boxes removed or absent and, at each iteration, those present and/or those absent have the previous image scaled down and drawn within them. The Sierpinski triangle may be approximated by a box fractal with one corner removed. The Sierpinski carpet is a box fractal with the middle square removed. Construction The basic square is decomposed into nine smaller squares in the 3-by-3 grid. The four squares at the corners and the middle square are left, the other squares being removed. The process is repeated recursively for each of the five remaining subsquares. The Vic ...
[...More Info...]       [...Related Items...]     OR:     [Wikipedia]   [Google]   [Baidu]