|
Van Lamoen Circle
In Euclidean plane geometry, the van Lamoen circle is a special circle associated with any given triangle T. It contains the circumcenters of the six triangles that are defined inside T by its three medians. Specifically, let A, B, C be the vertices of T, and let G be its centroid (the intersection of its three medians). Let M_a, M_b, and M_c be the midpoints of the sidelines BC, CA, and AB, respectively. It turns out that the circumcenters of the six triangles AGM_c, BGM_c, BGM_a, CGM_a, CGM_b, and AGM_b lie on a common circle, which is the van Lamoen circle of T. History The van Lamoen circle is named after the mathematician Floor van Lamoen https://nl.wikipedia.org/wiki/Floor_van_Lamoen who posed it as a problem in 2000. A proof was provided by Kin Y. Li in 2001, and the editors of the Amer. Math. Monthly in 2002. Properties The center of the van Lamoen circle is point X(1153) in Clark Kimberling's comprehensive list of triangle centers. In 2003, Alexey Myakis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Van Lamoen Circle
In Euclidean plane geometry, the van Lamoen circle is a special circle associated with any given triangle T. It contains the circumcenters of the six triangles that are defined inside T by its three medians. Specifically, let A, B, C be the vertices of T, and let G be its centroid (the intersection of its three medians). Let M_a, M_b, and M_c be the midpoints of the sidelines BC, CA, and AB, respectively. It turns out that the circumcenters of the six triangles AGM_c, BGM_c, BGM_a, CGM_a, CGM_b, and AGM_b lie on a common circle, which is the van Lamoen circle of T. History The van Lamoen circle is named after the mathematician Floor van Lamoen https://nl.wikipedia.org/wiki/Floor_van_Lamoen who posed it as a problem in 2000. A proof was provided by Kin Y. Li in 2001, and the editors of the Amer. Math. Monthly in 2002. Properties The center of the van Lamoen circle is point X(1153) in Clark Kimberling's comprehensive list of triangle centers. In 2003, Alexey Myakis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triangle Center
In geometry, a triangle center (or triangle centre) is a point in the plane that is in some sense a center of a triangle akin to the centers of squares and circles, that is, a point that is in the middle of the figure by some measure. For example, the centroid, circumcenter, incenter and orthocenter were familiar to the ancient Greeks, and can be obtained by simple constructions. Each of these classical centers has the property that it is invariant (more precisely equivariant) under similarity transformations. In other words, for any triangle and any similarity transformation (such as a rotation, reflection, dilation, or translation), the center of the transformed triangle is the same point as the transformed center of the original triangle. This invariance is the defining property of a triangle center. It rules out other well-known points such as the Brocard points which are not invariant under reflection and so fail to qualify as triangle centers. For an equilateral ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lester Circle
In Euclidean plane geometry, Lester's theorem states that in any scalene triangle, the two Fermat points, the nine-point center, and the circumcenter lie on the same circle. The result is named after June Lester, who published it in 1997, and the circle through these points was called the Lester circle by Clark Kimberling. Lester proved the result by using the properties of complex number In mathematics, a complex number is an element of a number system that extends the real numbers with a specific element denoted , called the imaginary unit and satisfying the equation i^= -1; every complex number can be expressed in the for ...s; subsequent authors have given elementary proofs, proofs using vector arithmetic, and computerized proofs. See also * Parry circle * * van Lamoen circle References External links *{{mathworld, id=LesterCircle, title=Lester Circle Theorems about triangles and circles ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Parry Circle
In geometry, the Parry point is a special point associated with a plane triangle. It is the triangle center designated X(111) in Clark Kimberling's Encyclopedia of Triangle Centers. The Parry point and Parry circle are named in honor of the English geometer Cyril Parry, who studied them in the early 1990s. Parry circle Let ''ABC'' be a plane triangle. The circle through the centroid and the two isodynamic points of triangle ''ABC'' is called the Parry circle of triangle ''ABC''. The equation of the Parry circle in barycentric coordinates is : \begin & 3(b^2-c^2)(c^2-a^2)(a^2-b^2)(a^2yz+b^2zx+c^2xy) \\ pt& + (x+y+z)\left( \sum_\text b^2c^2(b^2-c^2)(b^2+c^2-2a^2)x\right) =0 \end The center of the Parry circle is also a triangle center. It is the center designated as X(351) in Encyclopedia of Triangle Centers. The trilinear coordinates of the center of the Parry circle are : f(a,b,c) : f(b,c,a) : f(c,a,b) where f(a,b,c) = a(b^2-c^2)(b^2+c^2-2a^2) Parry point The Parry circle ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
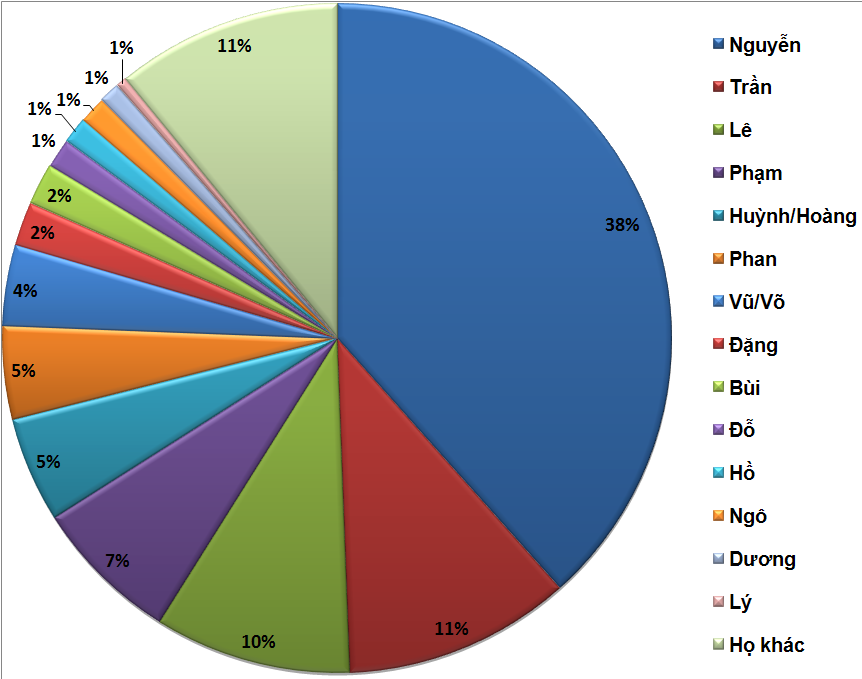
Nguyen Minh Ha
Nguyễn () is the most common Vietnamese surname. Outside of Vietnam, the surname is commonly rendered without diacritics as Nguyen. Nguyên (元）is a different word and surname. By some estimates 39 percent of Vietnamese people bear this surname.Lê Trung Hoa, ''Họ và tên người Việt Nam'', NXB Khoa học - Xã hội, 2005 Origin and usage "Nguyễn" is the spelling of the Sino-Vietnamese pronunciation of the Han character 阮 (, ). The same Han character is often romanized as ''Ruǎn'' in Mandarin, ''Yuen'' in Cantonese, ''Gnieuh'' or ''Nyoe¹'' in Wu Chinese, or ''Nguang'' in Hokchew. . Hanja reading (Korean) is 완 (''Wan'') or 원 (''Won'') and in Hiragana, it is げん (''Gen''), old reading as け゚ん (Ngen). The first recorded mention of a person surnamed Nguyen is a 317 CE description of a journey to Giao Châu undertaken by Eastern Jin dynasty (, ) officer and his family. Many events in Vietnamese history have contributed to the name's prominence. In ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Altitude (triangle)
In geometry, an altitude of a triangle is a line segment through a vertex and perpendicular to (i.e., forming a right angle with) a line containing the base (the side opposite the vertex). This line containing the opposite side is called the ''extended base'' of the altitude. The intersection of the extended base and the altitude is called the ''foot'' of the altitude. The length of the altitude, often simply called "the altitude", is the distance between the extended base and the vertex. The process of drawing the altitude from the vertex to the foot is known as ''dropping the altitude'' at that vertex. It is a special case of orthogonal projection. Altitudes can be used in the computation of the area of a triangle: one half of the product of an altitude's length and its base's length equals the triangle's area. Thus, the longest altitude is perpendicular to the shortest side of the triangle. The altitudes are also related to the sides of the triangle through the trigonome ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orthocenter
In geometry, an altitude of a triangle is a line segment through a vertex and perpendicular to (i.e., forming a right angle with) a line containing the base (the side opposite the vertex). This line containing the opposite side is called the ''extended base'' of the altitude. The intersection of the extended base and the altitude is called the ''foot'' of the altitude. The length of the altitude, often simply called "the altitude", is the distance between the extended base and the vertex. The process of drawing the altitude from the vertex to the foot is known as ''dropping the altitude'' at that vertex. It is a special case of orthogonal projection. Altitudes can be used in the computation of the area of a triangle: one half of the product of an altitude's length and its base's length equals the triangle's area. Thus, the longest altitude is perpendicular to the shortest side of the triangle. The altitudes are also related to the sides of the triangle through the trigonometri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Line Segment
In geometry, a line segment is a part of a straight line that is bounded by two distinct end points, and contains every point on the line that is between its endpoints. The length of a line segment is given by the Euclidean distance between its endpoints. A closed line segment includes both endpoints, while an open line segment excludes both endpoints; a half-open line segment includes exactly one of the endpoints. In geometry, a line segment is often denoted using a line above the symbols for the two endpoints (such as \overline). Examples of line segments include the sides of a triangle or square. More generally, when both of the segment's end points are vertices of a polygon or polyhedron, the line segment is either an edge (of that polygon or polyhedron) if they are adjacent vertices, or a diagonal. When the end points both lie on a curve (such as a circle), a line segment is called a chord (of that curve). In real or complex vector spaces If ''V'' is a vector spac ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cevian
In geometry, a cevian is a line that intersects both a triangle's vertex, and also the side that is opposite to that vertex. Medians and angle bisectors are special cases of cevians. The name "cevian" comes from the Italian mathematician Giovanni Ceva, who proved a well-known theorem about cevians which also bears his name. Length Stewart's theorem The length of a cevian can be determined by Stewart's theorem: in the diagram, the cevian length is given by the formula :\,b^2m + c^2n = a(d^2 + mn). Less commonly, this is also represented (with some rearrangement) by the following mnemonic: :\underset = \!\!\!\!\!\! \underset Median If the cevian happens to be a median (thus bisecting a side), its length can be determined from the formula :\,m(b^2 + c^2) = a(d^2 + m^2) or :\,2(b^2 + c^2) = 4d^2 + a^2 since :\,a = 2m. Hence in this case :d= \frac\sqrt2 . Angle bisector If the cevian happens to be an angle bisector, its length obeys the formulas :\,(b + c)^2 = a^ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Peter Y
Peter may refer to: People * List of people named Peter, a list of people and fictional characters with the given name * Peter (given name) ** Saint Peter (died 60s), apostle of Jesus, leader of the early Christian Church * Peter (surname), a surname (including a list of people with the name) Culture * Peter (actor) (born 1952), stage name Shinnosuke Ikehata, Japanese dancer and actor * ''Peter'' (album), a 1993 EP by Canadian band Eric's Trip * ''Peter'' (1934 film), a 1934 film directed by Henry Koster * ''Peter'' (2021 film), Marathi language film * "Peter" (''Fringe'' episode), an episode of the television series ''Fringe'' * ''Peter'' (novel), a 1908 book by Francis Hopkinson Smith * "Peter" (short story), an 1892 short story by Willa Cather Animals * Peter, the Lord's cat, cat at Lord's Cricket Ground in London * Peter (chief mouser), Chief Mouser between 1929 and 1946 * Peter II (cat), Chief Mouser between 1946 and 1947 * Peter III (cat), Chief Mouser between 194 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alexey Myakishev
Alexey, Alexei, Alexie, Aleksei, or Aleksey (russian: Алексе́й ; bg, Алексей ) is a Russian and Bulgarian male first name deriving from the Greek ''Aléxios'' (), meaning "Defender", and thus of the same origin as the Latin Alexius. Alexey may also be romanized as ''Aleksei'', ''Aleksey'', ''Alexej'', ''Aleksej'', etc. It has been commonly westernized as Alexis. Similar Ukrainian and Belarusian names are romanized as Oleksii (Олексій) and Aliaksiej (Аляксей), respectively. The Russian Orthodox Church uses the Old Church Slavonic version, Alexiy (Алексiй, or Алексий in modern spelling), for its Saints and hierarchs (most notably, this is the form used for Patriarchs Alexius I and Alexius II). The common hypocoristic is Alyosha () or simply Lyosha (). These may be further transformed into Alyoshka, Alyoshenka, Lyoshka, Lyoha, Lyoshenka (, respectively), sometimes rendered as Alesha/Aleshenka in English. The form Alyosha may b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kimberling Center
The Encyclopedia of Triangle Centers (ETC) is an online list of thousands of points or " centers" associated with the geometry of a triangle. It is maintained by Clark Kimberling, Professor of Mathematics at the University of Evansville. , the list identifies 52,440 triangle centers. Each point in the list is identified by an index number of the form ''X''(''n'')—for example, ''X''(1) is the incenter. The information recorded about each point includes its trilinear and barycentric coordinates and its relation to lines joining other identified points. Links to The Geometer's Sketchpad diagrams are provided for key points. The Encyclopedia also includes a glossary of terms and definitions. Each point in the list is assigned a unique name. In cases where no particular name arises from geometrical or historical considerations, the name of a star is used instead. For example, the 770th point in the list is named ''point Acamar''. The first 10 points listed in the Encyclopedi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
