|
Transformational Theory
Transformational theory is a branch of music theory developed by David Lewin in the 1980s, and formally introduced in his 1987 work, ''Generalized Musical Intervals and Transformations''. The theory—which models musical transformations as elements of a mathematical group—can be used to analyze both tonal and atonal music. The goal of transformational theory is to change the focus from musical objects—such as the "C major chord" or "G major chord"—to relations between musical objects (related by transformation). Thus, instead of saying that a C major chord is followed by G major, a transformational theorist might say that the first chord has been "transformed" into the second by the " Dominant operation." (Symbolically, one might write "Dominant(C major) = G major.") While traditional musical set theory focuses on the makeup of musical objects, transformational theory focuses on the intervals or types of musical motion that can occur. According to Lewin's descriptio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euclidean Vector
In mathematics, physics, and engineering, a Euclidean vector or simply a vector (sometimes called a geometric vector or spatial vector) is a geometric object that has magnitude (or length) and direction. Vectors can be added to other vectors according to vector algebra. A Euclidean vector is frequently represented by a ''directed line segment'', or graphically as an arrow connecting an ''initial point'' ''A'' with a ''terminal point'' ''B'', and denoted by \overrightarrow . A vector is what is needed to "carry" the point ''A'' to the point ''B''; the Latin word ''vector'' means "carrier". It was first used by 18th century astronomers investigating planetary revolution around the Sun. The magnitude of the vector is the distance between the two points, and the direction refers to the direction of displacement from ''A'' to ''B''. Many algebraic operations on real numbers such as addition, subtraction, multiplication, and negation have close analogues for vectors, operation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Musical Systems , the ability to perceive music or to create music
*
{{Music disambiguation ...
Musical is the adjective of music. Musical may also refer to: * Musical theatre, a performance art that combines songs, spoken dialogue, acting and dance * Musical film and television, a genre of film and television that incorporates into the narrative songs sung by the characters * MusicAL, an Albanian television channel * Musical isomorphism, the canonical isomorphism between the tangent and cotangent bundles See also * Lists of musicals * Music (other) * Musica (other) * Musicality Musicality (''music-al -ity'') is "sensitivity to, knowledge of, or talent for music" or "the quality or state of being musical", and is used to refer to specific if vaguely defined qualities in pieces and/or genres of music, such as melodiousness ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
University Of California, Riverside
The University of California, Riverside (UCR or UC Riverside) is a public university, public Land-grant university, land-grant research university in Riverside, California. It is one of the ten campuses of the University of California system. The main campus sits on in a suburban district of Riverside with a branch campus of in Palm Desert, California, Palm Desert. In 1907, the predecessor to UCR was founded as the UC Citrus Experiment Station, Riverside which pioneered research in biological pest control and the use of plant hormone, growth regulators responsible for extending the citrus growing season in California from four to nine months. Some of the world's most important research collections on University of California, Riverside Citrus Variety Collection, citrus diversity and Entomology Research Museum, entomology, as well as Eaton collection, science fiction and UCR/California Museum of Photography, photography, are located at Riverside. UCR's undergraduate UCR College ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alexander Rehding
Alexander Rehding is Fanny Peabody Professor of Music at Harvard University. Rehding is a music theorist and musicologist with a focus on intellectual history and media theory, known for innovative interdisciplinary work. His publications explore music in a wide range of contexts from Ancient Greek music to the Eurovision Song Contest—and even in outer space. His research has contributed to Riemannian theory, the history of music theory, sound studies, and media archaeology, reaching into the digital humanities and ecomusicology. Biography A native of Hamburg, Germany, Rehding was educated at Queens' College, Cambridge University. He held research fellowships at Emmanuel College, Cambridge, the Penn Humanities Forum (now Wolf Humanities Center at the University of Pennsylvania) and the Society of Fellows in the Liberal Arts at Princeton University before joining the Music Department at Harvard University in 2003, initially as Assistant Professor. He was promoted to a full profe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perspectives Of New Music
''Perspectives of New Music'' (PNM) is a peer-reviewed academic journal specializing in music theory and analysis. It was established in 1962 by Arthur Berger and Benjamin Boretz (who were its initial editors-in-chief). ''Perspectives'' was first published by the Princeton University Press, initially supported by the Fromm Music Foundation.David Carson Berry, "''Journal of Music Theory'' under Allen Forte's Editorship," ''Journal of Music Theory'' 50/1 (2006), 21, n49. The first issue was favorably reviewed in the ''Journal of Music Theory'', which observed that Berger and Boretz had produced "a first issue which sustains such a high quality of interest and cogency among its articles that one suspects the long delay preceding the yet-unborn Spring 1963 issue may reflect a scarcity of material up to their standard". However, as the journal's editorial "perspective" coalesced, Fromm became—in the words of David Gable—disenchanted with the "exclusive viewpoint hatcame to domina ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
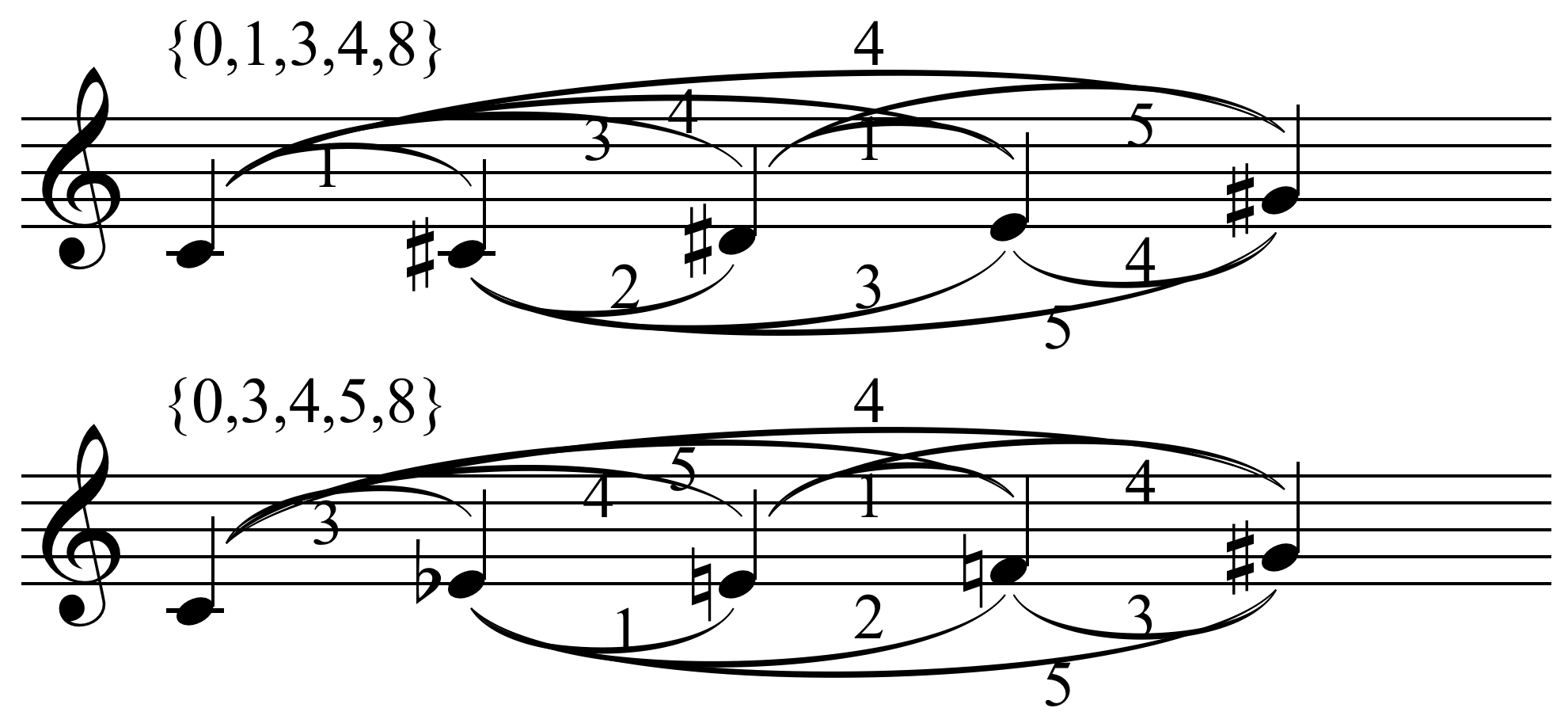
Interval Vector
In musical set theory, an interval vector is an array of natural numbers which summarize the intervals present in a set of pitch classes. (That is, a set of pitches where octaves are disregarded.) Other names include: ic vector (or interval-class vector), PIC vector (or pitch-class interval vector) and APIC vector (or absolute pitch-class interval vector, which Michiel Schuijer states is more proper.) While primarily an analytic tool, interval vectors can also be useful for composers, as they quickly show the sound qualities that are created by different collections of pitch class. That is, sets with high concentrations of conventionally dissonant intervals (i.e., seconds and sevenths) sound more dissonant, while sets with higher numbers of conventionally consonant intervals (i.e., thirds and sixths) sound more consonant. While the actual perception of consonance and dissonance involves many contextual factors, such as register, an interval vector can nevertheless be a h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pitch Space
In music theory, pitch spaces model relationships between pitches. These models typically use distance to model the degree of relatedness, with closely related pitches placed near one another, and less closely related pitches placed farther apart. Depending on the complexity of the relationships under consideration, the models may be multidimensional. Models of pitch space are often graphs, groups, lattices, or geometrical figures such as helixes. Pitch spaces distinguish octave-related pitches. When octave-related pitches are not distinguished, we have instead pitch class spaces, which represent relationships between pitch classes. (Some of these models are discussed in the entry on modulatory space, though readers should be advised that the term "modulatory space" is not a standard music-theoretical term.) Chordal spaces model relationships between chords. Linear and helical pitch space The simplest pitch space model is the real line. A fundamental frequency ''f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fred Lerdahl
Alfred Whitford (Fred) Lerdahl (born March 10, 1943, in Madison, Wisconsin) is the Fritz Reiner Professor Emeritus of Musical Composition at Columbia University, and a composer and music theorist best known for his work on musical grammar and cognition, rhythmic theory, pitch space, and cognitive constraints on compositional systems. He has written many orchestral and chamber works, three of which were finalists for the Pulitzer Prize for Music: ''Time after Time'' in 2001, String Quartet No. 3 in 2010, and '' Arches'' in 2011. Life Lerdahl studied with James Ming at Lawrence University, where he earned his BMus in 1965, and with Milton Babbitt, Edward T. Cone, and Earl Kim at Princeton University, where he earned his MFA in 1967. At Tanglewood he studied with Arthur Berger in 1964 and Roger Sessions in 1966. He then studied with Wolfgang Fortner at the Hochschule für Musik in Freiburg/Breisgau in 1968–69, on a Fulbright Scholarship. From 1991 to 2018 Lerdahl was F ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Richard Cohn
Richard Cohn (born 1955) is a music theorist and Battell Professor of Music Theory at Yale. He was previously chair of the department of music at the University of Chicago. Early in his career, he specialized in the music of Béla Bartók, but more recently has written about Neo-Riemannian theory, metric dissonance, equal divisions of the octave, and chromatic harmony. In 1994, he won the Society for Music Theory The Society for Music Theory (SMT) is an American organization devoted to the promotion of music theory as a scholarly and pedagogical discipline. It currently has a membership of over 1200, primarily in the United States. In the 1970s, few school ...'s Outstanding Publication Award for his article, "Transpositional Combination of Beat-Class Sets in Steve Reich’s Phase-Shifting Music," and he won it again in 1997 for "Maximally Smooth Cycles, Hexatonic Systems, and the Analysis of Late-Romantic Triadic Progressions." Cohn was the founding editor (2004–14) of ''Ox ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Neo-Riemannian Theory
Neo-Riemannian theory is a loose collection of ideas present in the writings of music theorists such as David Lewin, Brian Hyer, Richard Cohn, and Henry Klumpenhouwer. What binds these ideas is a central commitment to relating harmonies directly to each other, without necessary reference to a tonic. Initially, those harmonies were major and minor triads; subsequently, neo-Riemannian theory was extended to standard dissonant sonorities as well. Harmonic proximity is characteristically gauged by efficiency of voice leading. Thus, C major and E minor triads are close by virtue of requiring only a single semitonal shift to move from one to the other. Motion between proximate harmonies is described by simple transformations. For example, motion between a C major and E minor triad, in either direction, is executed by an "L" transformation. Extended progressions of harmonies are characteristically displayed on a geometric plane, or map, which portrays the entire system of harmonic r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Leittonwechsel
Parallel and counter parallel chords are terms derived from the German (''Parallelklang'', ''Gegenparallelklang'') to denote what is more often called in English the "relative", and possibly the "counter relative" chords. In Hugo Riemann's theory, and in German theory more generally, these chords share the function of the chord to which they link: subdominant parallel, dominant parallel, and tonic parallel.Haunschild, Frank (2000). ''The New Harmony Book'', p.47. . Riemann defines the relation in terms of the movement of one single note: For example, the major and and minor and . :The tonic, subdominant, and dominant chords, in root position, each followed by its parallel. The parallel is formed by raising the fifth a whole tone. :The minor tonic, subdominant, dominant, and their parallels, created by lowering the fifth (German)/root (US) a whole tone. The parallel chord (but ''not'' the counter parallel chord) of a major chord will always be the minor ch ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |