|
Stanisław Świerczkowski
Stanisław (Stash) Świerczkowski (16 July 1932 – 30 September 2015) was a Polish mathematician famous for his solutions to two iconic problems posed by Hugo Steinhaus: the three-gap theorem and the Non-Tetratorus Theorem. Early life and education Stanisław (Stash) Świerczkowski was born in Toruń, Poland. His parents were divorced during his infancy. When war broke out his father was captured in Soviet-controlled Poland and murdered in the 1940 Katyń Massacre. He belonged to the Polish nobility; Świerczkowski's mother belonged to the upper middle class and would have probably suffered deportation and murder by the Nazis. However she had German connections and was able to gain relatively privileged class 2 Volksliste citizenship. At the end of the war Świerczkowski's mother was forced into hiding near Toruń until she was confident that she could win exoneration from the Soviet-controlled government for her Volksliste status and be rehabilitated as a Polish citizen. Meanw ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Toruń
)'' , image_skyline = , image_caption = , image_flag = POL Toruń flag.svg , image_shield = POL Toruń COA.svg , nickname = City of Angels, Gingerbread city, Copernicus Town , pushpin_map = Kuyavian-Pomeranian Voivodeship#Poland#Europe , pushpin_relief=1 , pushpin_label_position = top , subdivision_type = Country , subdivision_name = , subdivision_type1 = Voivodeship , subdivision_name1 = , leader_title = City mayor , leader_name = Michał Zaleski , established_title = Established , established_date = 8th century , established_title3 = City rights , established_date3 = 1233 , area_total_km2 = 115.75 , population_as_of = 31 December 2021 , population_total = 196,935 ( 16th) Data for territorial unit 0463000. , population_density_km2 = 1716 , population_metro = 297646 , timezone = CET , utc_offset = +1 , timezone_DST = CEST , utc_offset_DST = +2 , coordinates = , elevatio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Three-gap Theorem
In mathematics, the three-gap theorem, three-distance theorem, or Steinhaus conjecture states that if one places points on a circle, at angles of , , , ... from the starting point, then there will be at most three distinct distances between pairs of points in adjacent positions around the circle. When there are three distances, the largest of the three always equals the sum of the other two. Unless is a rational multiple of , there will also be at least two distinct distances. This result was conjectured by Hugo Steinhaus, and proved in the 1950s by Vera T. Sós, , and Stanisław Świerczkowski; more proofs were added by others later. Applications of the three-gap theorem include the study of plant growth and musical tuning systems, and the theory of light reflection within a mirrored square. Statement The three-gap theorem can be stated geometrically in terms of points on a circle. In this form, it states that if one places n points on a circle, at angles of \theta, 2\theta, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Real Number
In mathematics, a real number is a number that can be used to measurement, measure a ''continuous'' one-dimensional quantity such as a distance, time, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every real number can be almost uniquely represented by an infinite decimal expansion. The real numbers are fundamental in calculus (and more generally in all mathematics), in particular by their role in the classical definitions of limit (mathematics), limits, continuous function, continuity and derivatives. The set of real numbers is mathematical notation, denoted or \mathbb and is sometimes called "the reals". The adjective ''real'' in this context was introduced in the 17th century by René Descartes to distinguish real numbers, associated with physical reality, from imaginary numbers (such as the square roots of ), which seemed like a theoretical contrivance unrelated to physical reality. The real numbers subset, include t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Axiom Of Determinacy
In mathematics, the axiom of determinacy (abbreviated as AD) is a possible axiom for set theory introduced by Jan Mycielski and Hugo Steinhaus in 1962. It refers to certain two-person topological games of length ω. AD states that every game of a certain type is determined; that is, one of the two players has a winning strategy. Steinhaus and Mycielski's motivation for AD was its interesting consequences, and suggested that AD could be true in the smallest natural model L(R) of a set theory, which accepts only a weak form of the axiom of choice (AC) but contains all real and all ordinal numbers. Some consequences of AD followed from theorems proved earlier by Stefan Banach and Stanisław Mazur, and Morton Davis. Mycielski and Stanisław Świerczkowski contributed another one: AD implies that all sets of real numbers are Lebesgue measurable. Later Donald A. Martin and others proved more important consequences, especially in descriptive set theory. In 1988, John R. St ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stan Wagon
Stanley Wagon is a Canadian- American mathematician, a professor of mathematics at Macalester College in Minnesota. He is the author of multiple books on number theory, geometry, and computational mathematics, and is also known for his snow sculpture. Biography Wagon was born in Montreal, to Sam and Diana (Idlovitch) Wagon. His sister Lila (Wagon) Hope-Simpson died in 2021. Wagon did his undergraduate studies at McGill University in Montreal, graduating in 1971. He earned his Ph.D. in 1975 from Dartmouth College, under the supervision of James Earl Baumgartner. He married mathematician Joan Hutchinson, and the two of them shared a single faculty position at Smith College and again at Macalester, where they moved in 1990. Books *''The Banach–Tarski Paradox'' (Cambridge University Press, 1985) *''Old and New Unsolved Problems in Plane Geometry and Number Theory'' (with Victor Klee, Mathematical Association of America, 1991)''Mathematica® in Action: Problem Solving Through Visu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Platonic Solids
In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent (identical in shape and size) regular polygons (all angles congruent and all edges congruent), and the same number of faces meet at each vertex. There are only five such polyhedra: Geometers have studied the Platonic solids for thousands of years. They are named for the ancient Greek philosopher Plato who hypothesized in one of his dialogues, the ''Timaeus'', that the classical elements were made of these regular solids. History The Platonic solids have been known since antiquity. It has been suggested that certain carved stone balls created by the late Neolithic people of Scotland represent these shapes; however, these balls have rounded knobs rather than being polyhedral, the numbers of knobs frequently differed from the numbers of vertices of the Platonic solids, there is no ball whose knobs match the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tetrahedra
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the ordinary convex polyhedra and the only one that has fewer than 5 faces. The tetrahedron is the three-dimensional case of the more general concept of a Euclidean simplex, and may thus also be called a 3-simplex. The tetrahedron is one kind of pyramid, which is a polyhedron with a flat polygon base and triangular faces connecting the base to a common point. In the case of a tetrahedron the base is a triangle (any of the four faces can be considered the base), so a tetrahedron is also known as a "triangular pyramid". Like all convex polyhedra, a tetrahedron can be folded from a single sheet of paper. It has two such nets. For any tetrahedron there exists a sphere (called the circumsphere) on which all four vertices lie, and another ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Valérie Berthé
Valérie Berthé (born 16 December 1968) is a French mathematician who works as a director of research for the Centre national de la recherche scientifique (CNRS) at the Institut de Recherche en Informatique Fondamentale (IRIF), a joint project between CNRS and Paris Diderot University. Her research involves symbolic dynamics, combinatorics on words, discrete geometry, numeral systems, tessellations, and fractals. Education Berthé completed her baccalauréat at age 16, and studied at the École Normale Supérieure from 1988 to 1993. She earned a licentiate and master's degree in pure mathematics from Pierre and Marie Curie University in 1989, a Diplôme d'études approfondies from University of Paris-Sud in 1991, completed her agrégation in 1992, and was recruited by CNRS in 1993. Continuing her graduate studies, she defended a doctoral thesis in 1994 at the University of Bordeaux 1. Her dissertation, ''Fonctions de Carlitz et automates: Entropies conditionnelles'' was supervis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Diophantine Approximation
In number theory, the study of Diophantine approximation deals with the approximation of real numbers by rational numbers. It is named after Diophantus of Alexandria. The first problem was to know how well a real number can be approximated by rational numbers. For this problem, a rational number ''a''/''b'' is a "good" approximation of a real number ''α'' if the absolute value of the difference between ''a''/''b'' and ''α'' may not decrease if ''a''/''b'' is replaced by another rational number with a smaller denominator. This problem was solved during the 18th century by means of continued fractions. Knowing the "best" approximations of a given number, the main problem of the field is to find sharp upper and lower bounds of the above difference, expressed as a function of the denominator. It appears that these bounds depend on the nature of the real numbers to be approximated: the lower bound for the approximation of a rational number by another rational number is larger than ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jan Mikusiński
Jan Mikusiński (April 3, 1913 Stanisławów – July 27, 1987 Katowice) was a Polish mathematician based at the University of Wrocław known for his pioneering work in mathematical analysis. Mikusiński developed an operational calculus – known as the ''Calculus of Mikusiński'' ( MSC 44A40), which is relevant for solving differential equations. His operational calculus is based upon an algebra of the convolution of functions with respect to the Fourier transform. From the convolution product he goes on to define what in other contexts is called the field of fractions or a quotient field. These ordered pairs of functions Mikusiński calls "operators", the "Mikusiński operators". He is also well known for Mikusinski's cube, the Antosik–Mikusinski theorem, and Mikusinski convolution algebra. A street in Katowice is named after Mikusiński. Selected publications * ''An Introduction to Analysis - From Number to Integral.'' Wiley 1993 * ''The Operational Calculus.'' Pergamon ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jan Mycielski
Jan Mycielski (born February 7, 1932 in Wiśniowa, Podkarpackie Voivodeship, Poland)Curriculum vitae from Mycielski's web site, retrieved 2010-03-10. is a Polish-American , a professor emeritus of mathematics at the . Academic career Mycielski received his Ph.D. in mathematics from the University of Wrocła ...[...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
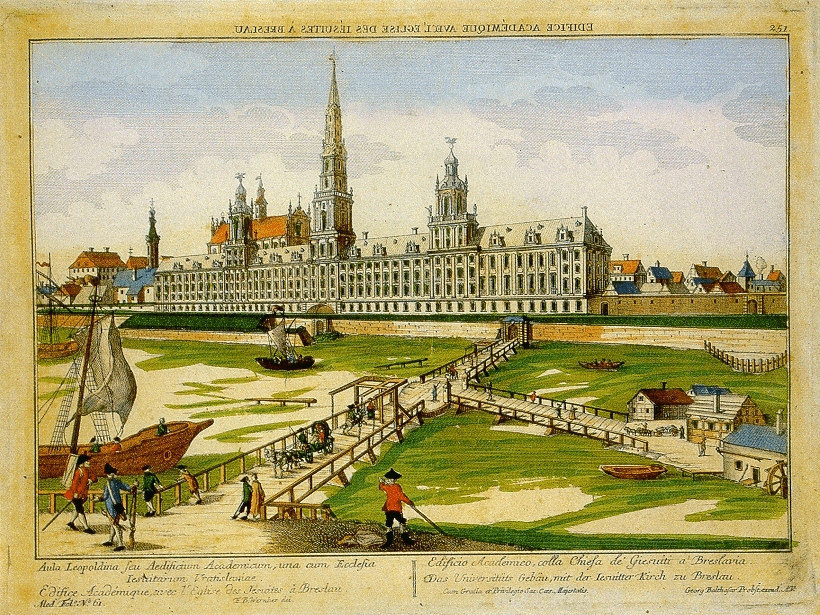
University Of Wrocław
, ''Schlesische Friedrich-Wilhelms-Universität zu Breslau'' (before 1945) , free_label = Specialty programs , free = , colors = Blue , website uni.wroc.pl The University of Wrocław ( pl, Uniwersytet Wrocławski, UWr; la, Universitas Wratislaviensis) is a public research university in Wrocław, Poland. It is the largest institution of higher learning in Lower Silesian Voivodeship, with over 100,000 graduates since 1945, including some 1,900 researchers, among whom many have received the highest awards for their contributions to the development of scientific scholarship. Renowned for its high quality of teaching, it was placed 44th by ''QS World University Rankings'': EECA 2016, and is situated on the same campus as the former University of Breslau, which produced 9 Nobel Prize winners. The university was founded in 1945, replacing the previous German University of Breslau. Following the territorial changes of Poland's borders, academics primarily ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |



.jpg)