|
Short-time Fourier Transform
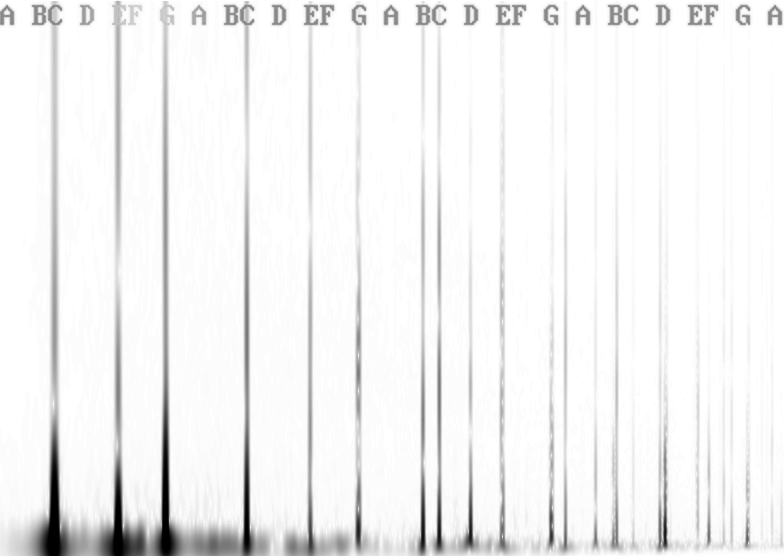
The short-time Fourier transform (STFT), is a Fourier-related transform used to determine the sinusoidal frequency and phase content of local sections of a signal as it changes over time. In practice, the procedure for computing STFTs is to divide a longer time signal into shorter segments of equal length and then compute the Fourier transform separately on each shorter segment. This reveals the Fourier spectrum on each shorter segment. One then usually plots the changing spectra as a function of time, known as a spectrogram or waterfall plot, such as commonly used in software defined radio (SDR) based spectrum displays. Full bandwidth displays covering the whole range of an SDR commonly use fast Fourier transforms (FFTs) with 2^24 points on desktop computers. Forward STFT Continuous-time STFT Simply, in the continuous-time case, the function to be transformed is multiplied by a window function which is nonzero for only a short period of time. The Fourier transform (a o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Fourier-related Transforms
This is a list of linear transformations of function (mathematics), functions related to Fourier analysis. Such transformations Map (mathematics), map a function to a set of coefficients of basis functions, where the basis functions are trigonometric function, sinusoidal and are therefore strongly localized in the frequency spectrum. (These transforms are generally designed to be invertible.) In the case of the Fourier transform, each basis function corresponds to a single frequency component. Continuous transforms Applied to functions of continuous arguments, Fourier-related transforms include: * Two-sided Laplace transform * Mellin transform, another closely related integral transform * Laplace transform * Fourier transform, with special cases: ** Fourier series *** When the input function/waveform is periodic, the Fourier transform output is a Dirac comb function, modulated by a discrete sequence of finite-valued coefficients that are complex-valued in general. These are cal ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
STFT - Windows
The short-time Fourier transform (STFT), is a Fourier-related transform used to determine the sinusoidal frequency and phase content of local sections of a signal as it changes over time. In practice, the procedure for computing STFTs is to divide a longer time signal into shorter segments of equal length and then compute the Fourier transform separately on each shorter segment. This reveals the Fourier spectrum on each shorter segment. One then usually plots the changing spectra as a function of time, known as a spectrogram or waterfall plot, such as commonly used in software defined radio (SDR) based spectrum displays. Full bandwidth displays covering the whole range of an SDR commonly use fast Fourier transforms (FFTs) with 2^24 points on desktop computers. Forward STFT Continuous-time STFT Simply, in the continuous-time case, the function to be transformed is multiplied by a window function which is nonzero for only a short period of time. The Fourier transform (a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
STFT Colored Spectrogram 375ms
The short-time Fourier transform (STFT), is a Fourier-related transform used to determine the sinusoidal frequency and phase content of local sections of a signal as it changes over time. In practice, the procedure for computing STFTs is to divide a longer time signal into shorter segments of equal length and then compute the Fourier transform separately on each shorter segment. This reveals the Fourier spectrum on each shorter segment. One then usually plots the changing spectra as a function of time, known as a spectrogram or waterfall plot, such as commonly used in software defined radio (SDR) based spectrum displays. Full bandwidth displays covering the whole range of an SDR commonly use fast Fourier transforms (FFTs) with 2^24 points on desktop computers. Forward STFT Continuous-time STFT Simply, in the continuous-time case, the function to be transformed is multiplied by a window function which is nonzero for only a short period of time. The Fourier transform (a o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
STFT Colored Spectrogram 125ms
The short-time Fourier transform (STFT), is a Fourier-related transform used to determine the sinusoidal frequency and phase content of local sections of a signal as it changes over time. In practice, the procedure for computing STFTs is to divide a longer time signal into shorter segments of equal length and then compute the Fourier transform separately on each shorter segment. This reveals the Fourier spectrum on each shorter segment. One then usually plots the changing spectra as a function of time, known as a spectrogram or waterfall plot, such as commonly used in software defined radio (SDR) based spectrum displays. Full bandwidth displays covering the whole range of an SDR commonly use fast Fourier transforms (FFTs) with 2^24 points on desktop computers. Forward STFT Continuous-time STFT Simply, in the continuous-time case, the function to be transformed is multiplied by a window function which is nonzero for only a short period of time. The Fourier transform (a o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
STFT Colored Spectrogram 25ms
The short-time Fourier transform (STFT), is a Fourier-related transform used to determine the sinusoidal frequency and phase content of local sections of a signal as it changes over time. In practice, the procedure for computing STFTs is to divide a longer time signal into shorter segments of equal length and then compute the Fourier transform separately on each shorter segment. This reveals the Fourier spectrum on each shorter segment. One then usually plots the changing spectra as a function of time, known as a spectrogram or waterfall plot, such as commonly used in software defined radio (SDR) based spectrum displays. Full bandwidth displays covering the whole range of an SDR commonly use fast Fourier transforms (FFTs) with 2^24 points on desktop computers. Forward STFT Continuous-time STFT Simply, in the continuous-time case, the function to be transformed is multiplied by a window function which is nonzero for only a short period of time. The Fourier transform (a o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hertz
The hertz (symbol: Hz) is the unit of frequency in the International System of Units (SI), equivalent to one event (or cycle) per second. The hertz is an SI derived unit whose expression in terms of SI base units is s−1, meaning that one hertz is the reciprocal of one second. It is named after Heinrich Rudolf Hertz (1857–1894), the first person to provide conclusive proof of the existence of electromagnetic waves. Hertz are commonly expressed in multiples: kilohertz (kHz), megahertz (MHz), gigahertz (GHz), terahertz (THz). Some of the unit's most common uses are in the description of periodic waveforms and musical tones, particularly those used in radio- and audio-related applications. It is also used to describe the clock speeds at which computers and other electronics are driven. The units are sometimes also used as a representation of the energy of a photon, via the Planck relation ''E'' = ''hν'', where ''E'' is the photon's energy, ''ν'' is its freq ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Window B
A window is an opening in a wall, door, roof, or vehicle that allows the exchange of light and may also allow the passage of sound and sometimes air. Modern windows are usually glazed or covered in some other transparent or translucent material, a sash set in a frame in the opening; the sash and frame are also referred to as a window. Many glazed windows may be opened, to allow ventilation, or closed, to exclude inclement weather. Windows may have a latch or similar mechanism to lock the window shut or to hold it open by various amounts. In addition to this, many modern day windows may have a window screen or mesh, often made of aluminum or fibreglass, to keep bugs out when the window is opened. Types include the eyebrow window, fixed windows, hexagonal windows, single-hung, and double-hung sash windows, horizontal sliding sash windows, casement windows, awning windows, hopper windows, tilt, and slide windows (often door-sized), tilt and turn windows, transom windows, sidel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Morlet Wavelet
In mathematics, the Morlet wavelet (or Gabor wavelet)0). The parameter \sigma in the Morlet wavelet allows trade between time and frequency resolutions. Conventionally, the restriction \sigma>5 is used to avoid problems with the Morlet wavelet at low \sigma (high temporal resolution). For signals containing only slowly varying frequency and amplitude modulations (audio, for example) it is not necessary to use small values of \sigma. In this case, \kappa_ becomes very small (e.g. \sigma>5 \quad \Rightarrow \quad \kappa_5, the frequency of the Morlet wavelet is conventionally taken to be \omega_\simeq\sigma. The wavelet exists as a complex version or a purely real-valued version. Some distinguish between the "real Morlet" vs the "complex Morlet". Others consider the complex version to be the "Gabor wavelet", while the real-valued version is the "Morlet wavelet". Uses Use in medicine In magnetic resonance spectroscopy imaging, the Morlet wavelet transform method offers an in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
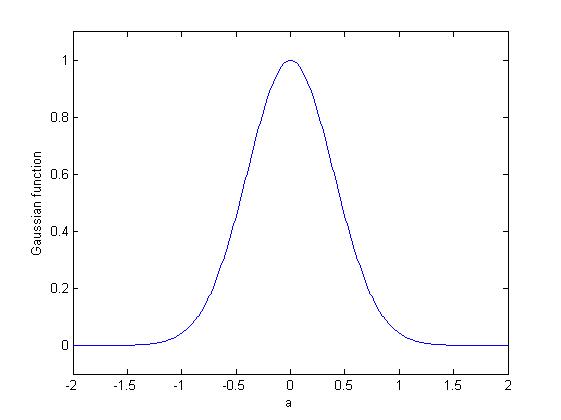
Gabor Transform
The Gabor transform, named after Dennis Gabor, is a special case of the short-time Fourier transform. It is used to determine the sinusoidal frequency and phase content of local sections of a signal as it changes over time. The function to be transformed is first multiplied by a Gaussian function, which can be regarded as a window function, and the resulting function is then transformed with a Fourier transform to derive the time-frequency analysis.E. Sejdić, I. Djurović, J. Jiang, “Time-frequency feature representation using energy concentration: An overview of recent advances,” ''Digital Signal Processing'', vol. 19, no. 1, pp. 153-183, January 2009. The window function means that the signal near the time being analyzed will have higher weight. The Gabor transform of a signal ''x''(''t'') is defined by this formula: : G_x(\tau,\omega) = \int_^\infty x(t)e^e^\,dt The Gaussian function has infinite range and it is impractical for implementation. However, a level of signific ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fourier Uncertainty Principle
A Fourier transform (FT) is a mathematics, mathematical Integral transform, transform that decomposes function (mathematics), functions into frequency components, which are represented by the output of the transform as a function of frequency. Most commonly functions of time or space are transformed, which will output a function depending on Frequency, temporal frequency or spatial frequency respectively. That process is also called ''analysis''. An example application would be decomposing the waveform of a musical Chord (music), chord into terms of the Sound intensity, intensity of its constituent Pitch (music), pitches. The term ''Fourier transform'' refers to both the frequency domain representation and the Operation (mathematics), mathematical operation that associates the frequency domain representation to a function of space or time. The Fourier transform of a function is a complex-valued function representing the Euler's formula, complex sinusoids that comprise the original ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gabor Limit
In quantum mechanics, the uncertainty principle (also known as Heisenberg's uncertainty principle) is any of a variety of mathematical inequalities asserting a fundamental limit to the accuracy with which the values for certain pairs of physical quantities of a particle, such as position, ''x'', and momentum, ''p'', can be predicted from initial conditions. Such variable pairs are known as complementary variables or canonically conjugate variables; and, depending on interpretation, the uncertainty principle limits to what extent such conjugate properties maintain their approximate meaning, as the mathematical framework of quantum physics does not support the notion of simultaneously well-defined conjugate properties expressed by a single value. The uncertainty principle implies that it is in general not possible to predict the value of a quantity with arbitrary certainty, even if all initial conditions are specified. Introduced first in 1927 by the German physicist Werner Hei ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uncertainty Principle
In quantum mechanics, the uncertainty principle (also known as Heisenberg's uncertainty principle) is any of a variety of mathematical inequalities asserting a fundamental limit to the accuracy with which the values for certain pairs of physical quantities of a particle, such as position, ''x'', and momentum, ''p'', can be predicted from initial conditions. Such variable pairs are known as complementary variables or canonically conjugate variables; and, depending on interpretation, the uncertainty principle limits to what extent such conjugate properties maintain their approximate meaning, as the mathematical framework of quantum physics does not support the notion of simultaneously well-defined conjugate properties expressed by a single value. The uncertainty principle implies that it is in general not possible to predict the value of a quantity with arbitrary certainty, even if all initial conditions are specified. Introduced first in 1927 by the German physicist Werner ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
