|
Robert Woodhouse
Robert Woodhouse (28 April 177323 December 1827) was a British mathematician and astronomer. Biography Early life and education Robert Woodhouse was born on 28 April 1773 in Norwich, Norfolk, the son of Robert Woodhouse, linen draper, and Judith Alderson, the daughter of a Unitarian minister from Lowestoft. Robert junior was baptised at St George's Church, Colegate, Norwich, on 19 May, 1773. A younger son, John Thomas Woodhouse, was born in 1780. The brothers were educated at the Paston School in North Walsham, north of Norwich. In May 1790 Woodhouse was admitted to Gonville and Caius College, Cambridge, the college where Paston pupils were traditionally sent. In 1795 he graduated as the Senior Wrangler (ranked first among the mathematics undergraduates at the university), and took the First Smith's Prize. He obtained his Master's degree at Cambridge in 1798. Marriage and career at Cambridge Woodhouse was a fellow of the college from 1798 to 1823, after which he resign ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Norwich
Norwich () is a cathedral city and district of Norfolk, England, of which it is the county town. Norwich is by the River Wensum, about north-east of London, north of Ipswich and east of Peterborough. As the seat of the See of Norwich, with one of the country's largest medieval cathedrals, it is the largest settlement and has the largest urban area in East Anglia. The population of the Norwich City Council local authority area was estimated to be 144,000 in 2021, which was an increase from 143,135 in 2019. The wider built-up area had a population of 213,166 in 2019. Heritage and status Norwich claims to be the most complete medieval city in the United Kingdom. It includes cobbled streets such as Elm Hill, Timber Hill and Tombland; ancient buildings such as St Andrew's Hall; half-timbered houses such as Dragon Hall, The Guildhall and Strangers' Hall; the Art Nouveau of the 1899 Royal Arcade; many medieval lanes; and the winding River Wensum that flows through the city ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fellow
A fellow is a concept whose exact meaning depends on context. In learned or professional societies, it refers to a privileged member who is specially elected in recognition of their work and achievements. Within the context of higher educational institutions, a fellow can be a member of a highly ranked group of teachers at a particular college or university or a member of the governing body in some universities (such as the Fellows of Harvard College); it can also be a specially selected postgraduate student who has been appointed to a post (called a fellowship) granting a stipend, research facilities and other privileges for a fixed period (usually one year or more) in order to undertake some advanced study or research, often in return for teaching services. In the context of research and development-intensive large companies or corporations, the title "fellow" is sometimes given to a small number of senior scientists and engineers. In the context of medical education in No ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Plumian Professor Of Astronomy And Experimental Philosophy
The Plumian chair of Astronomy and Experimental Philosophy is one of the major professorships in Astronomy at Cambridge University, alongside the Lowndean Professorship (which is now mainly held by mathematicians). The chair is currently held at the Institute of Astronomy in the University. The Plumian chair was founded in 1704 by Thomas Plume, a member of Christ's and Archdeacon of Rochester, to "erect an Observatory and to maintain a studious and learned Professor of Astronomy and Experimental Philosophy, and to buy him and his successors utensils and instruments quadrants telescopes etc." Trustees were appointed, and statutes drawn up by Isaac Newton, John Flamsteed and John Ellys. The first Professorship was awarded in 1706 to Roger Cotes, a former student of Newton, and the stipend was increased in 1768 by Dr Robert Smith, the second Plumian Professor. Plumian Professors # Roger Cotes (1706–1716) # Robert Smith (1716–1760) # Anthony Shepherd (1760–1796) # Samuel Vin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lucasian Professor Of Mathematics
The Lucasian Chair of Mathematics () is a mathematics professorship in the University of Cambridge, England; its holder is known as the Lucasian Professor. The post was founded in 1663 by Henry Lucas, who was Cambridge University's Member of Parliament in 1639–1640, and it was officially established by King Charles II on 18 January 1664. It was described by ''The Daily Telegraph'' as one of the most prestigious academic posts in the world. Since its establishment, the professorship has been held by, among others, Isaac Newton, Charles Babbage, George Stokes, Joseph Larmor, Paul Dirac, and Stephen Hawking. History Henry Lucas, in his will, bequeathed his library of 4,000 volumes to the university and left instructions for the purchase of land whose yielding should provide £100 a year for the founding of a professorship. Babbage applied for the vacancy in 1826, after Turton, but Airy was appointed. William Whewell (who considered applying, but preferred both Hersc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pierre-Simon Laplace
Pierre-Simon, marquis de Laplace (; ; 23 March 1749 – 5 March 1827) was a French scholar and polymath whose work was important to the development of engineering, mathematics, statistics, physics, astronomy, and philosophy. He summarized and extended the work of his predecessors in his five-volume ''Mécanique céleste'' (''Celestial Mechanics'') (1799–1825). This work translated the geometric study of classical mechanics to one based on calculus, opening up a broader range of problems. In statistics, the Bayesian interpretation of probability was developed mainly by Laplace. Laplace formulated Laplace's equation, and pioneered the Laplace transform which appears in many branches of mathematical physics, a field that he took a leading role in forming. The Laplacian differential operator, widely used in mathematics, is also named after him. He restated and developed the nebular hypothesis of the origin of the Solar System and was one of the first scientists to sugges ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Astronomy
Astronomy () is a natural science that studies astronomical object, celestial objects and phenomena. It uses mathematics, physics, and chemistry in order to explain their origin and chronology of the Universe, evolution. Objects of interest include planets, natural satellite, moons, stars, nebulae, galaxy, galaxies, and comets. Relevant phenomena include supernova explosions, gamma ray bursts, quasars, blazars, pulsars, and cosmic microwave background radiation. More generally, astronomy studies everything that originates beyond atmosphere of Earth, Earth's atmosphere. Cosmology is a branch of astronomy that studies the universe as a whole. Astronomy is one of the oldest natural sciences. The early civilizations in recorded history made methodical observations of the night sky. These include the Babylonian astronomy, Babylonians, Greek astronomy, Greeks, Indian astronomy, Indians, Egyptian astronomy, Egyptians, Chinese astronomy, Chinese, Maya civilization, Maya, and many anc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isoperimetry
In mathematics, the isoperimetric inequality is a geometric inequality involving the perimeter of a set and its volume. In n-dimensional space \R^n the inequality lower bounds the surface area or perimeter \operatorname(S) of a set S\subset\R^n by its volume \operatorname(S), :\operatorname(S)\geq n \operatorname(S)^ \, \operatorname(B_1)^, where B_1\subset\R^n is a unit sphere. The equality holds only when S is a sphere in \R^n. On a plane, i.e. when n=2, the isoperimetric inequality relates the square of the circumference of a closed curve and the area of a plane region it encloses. ''Isoperimetric'' literally means "having the same perimeter". Specifically in \R ^2, the isoperimetric inequality states, for the length ''L'' of a closed curve and the area ''A'' of the planar region that it encloses, that : L^2 \ge 4\pi A, and that equality holds if and only if the curve is a circle. The isoperimetric problem is to determine a plane figure of the largest possible area whose bo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Calculus Of Variations
The calculus of variations (or Variational Calculus) is a field of mathematical analysis that uses variations, which are small changes in functions and functionals, to find maxima and minima of functionals: mappings from a set of functions to the real numbers. Functionals are often expressed as definite integrals involving functions and their derivatives. Functions that maximize or minimize functionals may be found using the Euler–Lagrange equation of the calculus of variations. A simple example of such a problem is to find the curve of shortest length connecting two points. If there are no constraints, the solution is a straight line between the points. However, if the curve is constrained to lie on a surface in space, then the solution is less obvious, and possibly many solutions may exist. Such solutions are known as ''geodesics''. A related problem is posed by Fermat's principle: light follows the path of shortest optical length connecting two points, which depends up ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Treatise
A treatise is a formal and systematic written discourse on some subject, generally longer and treating it in greater depth than an essay, and more concerned with investigating or exposing the principles of the subject and its conclusions."Treatise." Merriam-Webster Online Dictionary. Accessed September 12, 2020. A monograph is a treatise on a specialized topic. Etymology The word 'treatise' first appeared in the fourteenth century as the Medieval English word ''tretis'', which evolved from the Medieval Latin ''tractatus'' and the Latin ''tractare'', meaning to treat or to handle. Historically significant treatises Table The works presented here have been identified as influential by scholars on the development of human civilization. Discussion of select examples Euclid's ''Elements'' Euclid's ''Elements'' has appeared in more editions than any other books except the ''Bible'' and is one of the most important mathematical treatises ever. It has been translated to numer ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
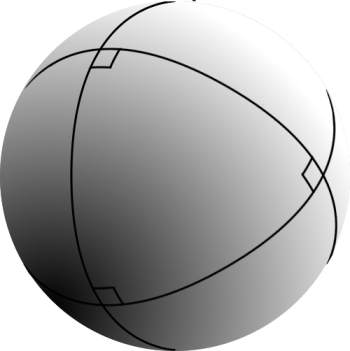
Spherical Trigonometry
Spherical trigonometry is the branch of spherical geometry that deals with the metrical relationships between the sides and angles of spherical triangles, traditionally expressed using trigonometric functions. On the sphere, geodesics are great circles. Spherical trigonometry is of great importance for calculations in astronomy, geodesy, and navigation. The origins of spherical trigonometry in Greek mathematics and the major developments in Islamic mathematics are discussed fully in History of trigonometry and Mathematics in medieval Islam. The subject came to fruition in Early Modern times with important developments by John Napier, Delambre and others, and attained an essentially complete form by the end of the nineteenth century with the publication of Todhunter's textbook ''Spherical trigonometry for the use of colleges and Schools''. Since then, significant developments have been the application of vector methods, quaternion methods, and the use of numerical methods. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trigonometry
Trigonometry () is a branch of mathematics that studies relationships between side lengths and angles of triangles. The field emerged in the Hellenistic world during the 3rd century BC from applications of geometry to astronomical studies. The Greeks focused on the calculation of chords, while mathematicians in India created the earliest-known tables of values for trigonometric ratios (also called trigonometric functions) such as sine. Throughout history, trigonometry has been applied in areas such as geodesy, surveying, celestial mechanics, and navigation. Trigonometry is known for its many identities. These trigonometric identities are commonly used for rewriting trigonometrical expressions with the aim to simplify an expression, to find a more useful form of an expression, or to solve an equation. History Sumerian astronomers studied angle measure, using a division of circles into 360 degrees. They, and later the Babylonians, studied the ratios of the sides of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Differential Notation
In differential calculus, there is no single uniform notation for differentiation. Instead, various notations for the derivative of a function or variable have been proposed by various mathematicians. The usefulness of each notation varies with the context, and it is sometimes advantageous to use more than one notation in a given context. The most common notations for differentiation (and its opposite operation, the antidifferentiation or indefinite integration) are listed below. Leibniz's notation The original notation employed by Gottfried Leibniz is used throughout mathematics. It is particularly common when the equation is regarded as a functional relationship between dependent and independent variables and . Leibniz's notation makes this relationship explicit by writing the derivative as :\frac. Furthermore, the derivative of at is therefore written :\frac(x)\text\frac\text\frac f(x). Higher derivatives are written as :\frac, \frac, \frac, \ldots, \frac. Thi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |



.jpg)