|
Roothaan Equations
The Roothaan equations are a representation of the Hartree–Fock equation in a non orthonormal basis set which can be of Gaussian-type or Slater-type. It applies to closed-shell molecules or atoms where all molecular orbitals or atomic orbitals, respectively, are doubly occupied. This is generally called Restricted Hartree–Fock theory. The method was developed independently by Clemens C. J. Roothaan and George G. Hall in 1951, and is thus sometimes called the ''Roothaan-Hall equations''. The Roothaan equations can be written in a form resembling generalized eigenvalue problem, although they are not a standard eigenvalue problem because they are nonlinear: :\mathbf \mathbf = \mathbf \mathbf \mathbf where F is the Fock matrix (which depends on the coefficients C due to electron-electron interactions), C is a matrix of coefficients, S is the overlap matrix of the basis functions, and \epsilon is the (diagonal, by convention) matrix of orbital energies. In the case of an o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Basis Set (chemistry)
In theoretical and computational chemistry, a basis set is a set of functions (called basis functions) that is used to represent the electronic wave function in the Hartree–Fock method or density-functional theory in order to turn the partial differential equations of the model into algebraic equations suitable for efficient implementation on a computer. The use of basis sets is equivalent to the use of an approximate resolution of the identity: the orbitals , \psi_i\rangle are expanded within the basis set as a linear combination of the basis functions , \psi_i\rangle \approx \sum_\mu c_ , \mu\rangle, where the expansion coefficients c_ are given by c_ = \sum_\nu \langle \mu, \nu \rangle^ \langle \nu , \psi_i \rangle. The basis set can either be composed of atomic orbitals (yielding the linear combination of atomic orbitals approach), which is the usual choice within the quantum chemistry community; plane waves which are typically used within the solid state community, or ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gaussian Orbital
In computational chemistry and molecular physics, Gaussian orbitals (also known as Gaussian type orbitals, GTOs or Gaussians) are functions used as atomic orbitals in the LCAO method for the representation of electron orbitals in molecules and numerous properties that depend on these. Rationale The use of Gaussian orbitals in electronic structure theory (instead of the more physical Slater-type orbitals) was first proposed by Boys in 1950. The principal reason for the use of Gaussian basis functions in molecular quantum chemical calculations is the 'Gaussian Product Theorem', which guarantees that the product of two GTOs centered on two different atoms is a finite sum of Gaussians centered on a point along the axis connecting them. In this manner, four-center integrals can be reduced to finite sums of two-center integrals, and in a next step to finite sums of one-center integrals. The speedup by 4-5 orders of magnitude compared to Slater orbitals outweighs the extra cost ent ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Slater-type Orbital
Slater-type orbitals (STOs) are functions used as atomic orbitals in the linear combination of atomic orbitals molecular orbital method. They are named after the physicist John C. Slater, who introduced them in 1930. They possess exponential decay at long range and Kato's cusp condition at short range (when combined as hydrogen-like atom functions, i.e. the analytical solutions of the stationary Schrödinger equation for one electron atoms). Unlike the hydrogen-like ("hydrogenic") Schrödinger orbitals, STOs have no radial nodes (neither do Gaussian-type orbitals). Definition STOs have the following radial part: : R(r) = N r^ e^\, where * is a natural number that plays the role of principal quantum number, = 1,2,..., * is a normalizing constant, * is the distance of the electron from the atomic nucleus, and * \zeta is a constant related to the effective charge of the nucleus, the nuclear charge being partly shielded by electrons. Historically, the effective nuclear charge w ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
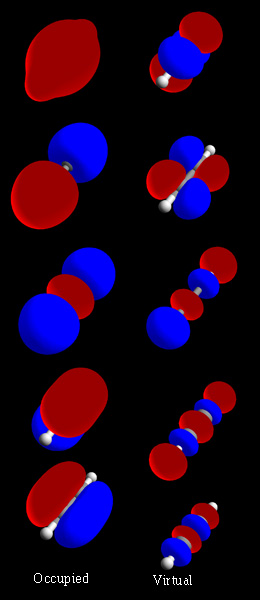
Molecular Orbital
In chemistry, a molecular orbital is a mathematical function describing the location and wave-like behavior of an electron in a molecule. This function can be used to calculate chemical and physical properties such as the probability of finding an electron in any specific region. The terms ''atomic orbital'' and ''molecular orbital'' were introduced by Robert S. Mulliken in 1932 to mean ''one-electron orbital wave functions''. At an elementary level, they are used to describe the ''region'' of space in which a function has a significant amplitude. In an isolated atom, the orbital electrons' location is determined by functions called atomic orbitals. When multiple atoms combine chemically into a molecule, the electrons' locations are determined by the molecule as a whole, so the atomic orbitals combine to form molecular orbitals. The electrons from the constituent atoms occupy the molecular orbitals. Mathematically, molecular orbitals are an approximate solution to the Schrö ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Atomic Orbital
In atomic theory and quantum mechanics, an atomic orbital is a function describing the location and wave-like behavior of an electron in an atom. This function can be used to calculate the probability of finding any electron of an atom in any specific region around the atom's nucleus. The term ''atomic orbital'' may also refer to the physical region or space where the electron can be calculated to be present, as predicted by the particular mathematical form of the orbital. Each orbital in an atom is characterized by a set of values of the three quantum numbers , , and , which respectively correspond to the electron's energy, angular momentum, and an angular momentum vector component ( magnetic quantum number). Alternative to the magnetic quantum number, the orbitals are often labeled by the associated harmonic polynomials (e.g., ''xy'', ). Each such orbital can be occupied by a maximum of two electrons, each with its own projection of spin m_s. The simple names s orbital ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Clemens C
Clemens is both a Late Latin masculine given name and a surname meaning "merciful". Notable people with the name include: Surname * Adelaide Clemens (born 1989), Australian actress. * Andrew Clemens (b. 1852 or 1857–1894), American folk artist * Aurelius Prudentius Clemens, 4th century Roman poet * Barry Clemens (born 1943), American basketball player * Bert A. Clemens (1874–1935), American politician * Brian Clemens (born 1931), British screenwriter and television producer * Clayton Clemens, American Professor of Government * Dan Clemens (1945–2019), American politician * Gabriel Clemens (born 1983), German darts player * George T. Clemens (1902–1992), American cinematographer * Harold W. Clemens (1918–1998), American politician * C. Herbert Clemens (born 1939), American mathematician * Isaac Clemens (1815–1880), Canadian farmer and politician * Jacob Clemens non Papa (c. 1510 to 1515–1555 or 1556), Franco-Flemish composer of the Renaissance * James Clem ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
George G
George may refer to: People * George (given name) * George (surname) * George (singer), American-Canadian singer George Nozuka, known by the mononym George * George Washington, First President of the United States * George W. Bush, 43rd President of the United States * George H. W. Bush, 41st President of the United States * George V, King of Great Britain, Ireland, the British Dominions and Emperor of India from 1910-1936 * George VI, King of Great Britain, Ireland, the British Dominions and Emperor of India from 1936-1952 * Prince George of Wales * George Papagheorghe also known as Jorge / GEØRGE * George, stage name of Giorgio Moroder * George Harrison, an English musician and singer-songwriter Places South Africa * George, Western Cape ** George Airport United States * George, Iowa * George, Missouri * George, Washington * George County, Mississippi * George Air Force Base, a former U.S. Air Force base located in California Characters * George (Peppa Pig), ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Proceedings Of The Royal Society A
''Proceedings of the Royal Society'' is the main research journal of the Royal Society. The journal began in 1831 and was split into two series in 1905: * Series A: for papers in physical sciences and mathematics. * Series B: for papers in life sciences. Many landmark scientific discoveries are published in the Proceedings, making it one of the most historically significant science journals. The journal contains several articles written by the most celebrated names in science, such as Paul Dirac, Werner Heisenberg, Ernest Rutherford, Erwin Schrödinger, William Lawrence Bragg, Lord Kelvin, J.J. Thomson, James Clerk Maxwell, Dorothy Hodgkin and Stephen Hawking. In 2004, the Royal Society began ''The Journal of the Royal Society Interface'' for papers at the interface of physical sciences and life sciences. History The journal began in 1831 as a compilation of abstracts of papers in the ''Philosophical Transactions of the Royal Society'', the older Royal Society publicati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Generalized Eigenvalue Problem
In linear algebra, eigendecomposition is the factorization of a matrix into a canonical form, whereby the matrix is represented in terms of its eigenvalues and eigenvectors. Only diagonalizable matrices can be factorized in this way. When the matrix being factorized is a normal or real symmetric matrix, the decomposition is called "spectral decomposition", derived from the spectral theorem. Fundamental theory of matrix eigenvectors and eigenvalues A (nonzero) vector of dimension is an eigenvector of a square matrix if it satisfies a linear equation of the form :\mathbf \mathbf = \lambda \mathbf for some scalar . Then is called the eigenvalue corresponding to . Geometrically speaking, the eigenvectors of are the vectors that merely elongates or shrinks, and the amount that they elongate/shrink by is the eigenvalue. The above equation is called the eigenvalue equation or the eigenvalue problem. This yields an equation for the eigenvalues : p\left(\lambda\right) = \det\l ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fock Matrix
In the Hartree–Fock method of quantum mechanics, the Fock matrix is a matrix approximating the single-electron energy operator of a given quantum system in a given set of basis vectors. It is most often formed in computational chemistry when attempting to solve the Roothaan equations for an atomic or molecular system. The Fock matrix is actually an approximation to the true Hamiltonian operator of the quantum system. It includes the effects of electron-electron repulsion only in an average way. Because the Fock operator is a one-electron operator, it does not include the electron correlation energy. The Fock matrix is defined by the Fock operator. For the restricted case which assumes closed-shell orbitals and single- determinantal wavefunctions, the Fock operator for the ''i''-th electron is given by:Levine, I.N. (1991) ''Quantum Chemistry'' (4th ed., Prentice-Hall), p.403 :\hat F(i) = \hat h(i)+\sum_^ \hat J_j(i)-\hat K_j(i)/math> where: :\hat F(i) is the Fock opera ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Overlap Matrix
In chemical bonds, an orbital overlap is the concentration of orbitals on adjacent atoms in the same regions of space. Orbital overlap can lead to bond formation. Linus Pauling explained the importance of orbital overlap in the molecular bond angles observed through experimentation; it is the basis for orbital hybridization. As ''s'' orbitals are spherical (and have no directionality) and ''p'' orbitals are oriented 90° to each other, a theory was needed to explain why molecules such as methane (CH4) had observed bond angles of 109.5°. Pauling proposed that s and p orbitals on the carbon atom can combine to form hybrids (sp3 in the case of methane) which are directed toward the hydrogen atoms. The carbon hybrid orbitals have greater overlap with the hydrogen orbitals, and can therefore form stronger C–H bonds.Pauling, Linus. (1960). ''The Nature Of The Chemical Bond''. Cornell University Press. A quantitative measure of the overlap of two atomic orbitals ΨA and ΨB o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |