|
Quantal Response Equilibrium
Quantal response equilibrium (QRE) is a solution concept in game theory. First introduced by Richard McKelvey and Thomas Palfrey, it provides an equilibrium notion with bounded rationality. QRE is not an equilibrium refinement, and it can give significantly different results from Nash equilibrium. QRE is only defined for games with discrete strategies, although there are continuous-strategy analogues. In a quantal response equilibrium, players are assumed to make errors in choosing which pure strategy to play. The probability of any particular strategy being chosen is positively related to the payoff from that strategy. In other words, very costly errors are unlikely. The equilibrium arises from the realization of beliefs. A player's payoffs are computed based on beliefs about other players' probability distribution over strategies. In equilibrium, a player's beliefs are correct. Application to data When analyzing data from the play of actual games, particularly from laborato ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nash Equilibrium
In game theory, the Nash equilibrium, named after the mathematician John Nash, is the most common way to define the solution of a non-cooperative game involving two or more players. In a Nash equilibrium, each player is assumed to know the equilibrium strategies of the other players, and no one has anything to gain by changing only one's own strategy. The principle of Nash equilibrium dates back to the time of Cournot, who in 1838 applied it to competing firms choosing outputs. If each player has chosen a strategy an action plan based on what has happened so far in the game and no one can increase one's own expected payoff by changing one's strategy while the other players keep their's unchanged, then the current set of strategy choices constitutes a Nash equilibrium. If two players Alice and Bob choose strategies A and B, (A, B) is a Nash equilibrium if Alice has no other strategy available that does better than A at maximizing her payoff in response to Bob choosing B, and Bob ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Matching Pennies
Matching pennies is the name for a simple game used in game theory. It is played between two players, Even and Odd. Each player has a penny and must secretly turn the penny to heads or tails. The players then reveal their choices simultaneously. If the pennies match (both heads or both tails), then Even keeps both pennies, so wins one from Odd (+1 for Even, −1 for Odd). If the pennies do not match (one heads and one tails) Odd keeps both pennies, so receives one from Even (−1 for Even, +1 for Odd). Theory Matching Pennies is a zero-sum game because each participant's gain or loss of utility is exactly balanced by the losses or gains of the utility of the other participants. If the participants' total gains are added up and their total losses subtracted, the sum will be zero. The game can be written in a payoff matrix (pictured right - from Even's point of view). Each cell of the matrix shows the two players' payoffs, with Even's payoffs listed first. Matching pennies ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bounded Rationality
Bounded rationality is the idea that rationality is limited when individuals make decisions, and under these limitations, rational individuals will select a decision that is satisfactory rather than optimal. Limitations include the difficulty of the problem requiring a decision, the cognitive capability of the mind, and the time available to make the decision. Decision-makers, in this view, act as satisficers, seeking a satisfactory solution, with everything that they have at the moment rather than an optimal solution. Therefore, humans do not undertake a full cost-benefit analysis to determine the optimal decision, but rather, choose an option that fulfils their adequacy criteria. An example of this being within organisations when they must adhere to the operating conditions of their company, this has the opportunity to result in bounded rationality as the organisation is not able to choose the optimal option. Some models of human behavior in the social sciences assume that hu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perfect Information
In economics, perfect information (sometimes referred to as "no hidden information") is a feature of perfect competition. With perfect information in a market, all consumers and producers have complete and instantaneous knowledge of all market prices, their own utility, and own cost functions. In game theory, a sequential game has perfect information if each player, when making any decision, is perfectly informed of all the events that have previously occurred, including the "initialization event" of the game (e.g. the starting hands of each player in a card game).Archived aGhostarchiveand thWayback Machine Perfect information defined at 0:25, with academic sources and . Perfect information is importantly different from complete information, which implies common knowledge of each player's utility functions, payoffs, strategies and "types". A game with perfect information may or may not have complete information. Games where some aspect of play is ''hidden'' from opponents - su ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
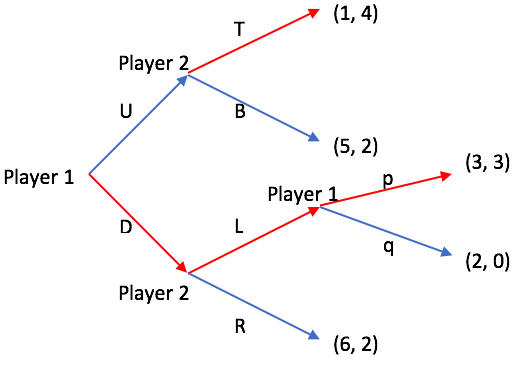
Sequential Game
In game theory, a sequential game is a game where one player chooses their action before the others choose theirs. The other players must have information on the first player's choice so that the difference in time has no strategic effect. Sequential games are governed by the time axis and represented in the form of decision trees. Sequential games with perfect information can be analysed mathematically using combinatorial game theory. Decision trees are the extensive form of dynamic games that provide information on the possible ways that a given game can be played. They show the sequence in which players act and the number of times that they can each make a decision. Decision trees also provide information on what each player knows or does not know at the point in time they decide on an action to take. Payoffs for each player are given at the decision nodes of the tree. Extensive form representations were introduced by Neumann and further developed by Kuhn in the earliest yea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
The Price Is Right (American Game Show)
''The Price Is Right'' is an American television game show created by Bob Stewart, Mark Goodson and Bill Todman where contestants compete by guessing the prices of merchandise to win cash and prizes. Contestants are selected from the studio audience as the announcer calls their names and invokes the show's famous catchphrase, "Come on down!" The program premiered on September 4, 1972, on CBS. Bob Barker was the series' longest-running host from its 1972 debut until his retirement in June 2007, when Drew Carey took over. Barker was accompanied by a series of announcers, beginning with Johnny Olson, followed by Rod Roddy and Rich Fields. In April 2011, George Gray became the announcer. The show has used several models, most notably Anitra Ford, Janice Pennington, Dian Parkinson, Holly Hallstrom, and Kathleen Bradley. While retaining some elements of the original 1956 version of the show, the 1972 version has added many new distinctive gameplay elements. ''The Price Is Righ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Subgame Perfection
In game theory, a subgame perfect equilibrium (or subgame perfect Nash equilibrium) is a refinement of a Nash equilibrium used in dynamic games. A strategy profile is a subgame perfect equilibrium if it represents a Nash equilibrium of every subgame of the original game. Informally, this means that at any point in the game, the players' behavior from that point onward should represent a Nash equilibrium of the continuation game (i.e. of the subgame), no matter what happened before. Every finite extensive game with perfect recall has a subgame perfect equilibrium. Perfect recall is a term introduced by Harold W. Kuhn in 1953 and ''"equivalent to the assertion that each player is allowed by the rules of the game to remember everything he knew at previous moves and all of his choices at those moves"''. A common method for determining subgame perfect equilibria in the case of a finite game is backward induction. Here one first considers the last actions of the game and determi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Extensive Form Game
An extensive-form game is a specification of a game in game theory, allowing (as the name suggests) for the explicit representation of a number of key aspects, like the sequencing of players' possible moves, their choices at every decision point, the (possibly imperfect) information each player has about the other player's moves when they make a decision, and their payoffs for all possible game outcomes. Extensive-form games also allow for the representation of incomplete information in the form of chance events modeled as " moves by nature". Finite extensive-form games Some authors, particularly in introductory textbooks, initially define the extensive-form game as being just a game tree with payoffs (no imperfect or incomplete information), and add the other elements in subsequent chapters as refinements. Whereas the rest of this article follows this gentle approach with motivating examples, we present upfront the finite extensive-form games as (ultimately) constructed here. This ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mean Field Theory
In physics and probability theory, Mean-field theory (MFT) or Self-consistent field theory studies the behavior of high-dimensional random (stochastic) models by studying a simpler model that approximates the original by averaging over degrees of freedom (the number of values in the final calculation of a statistic that are free to vary). Such models consider many individual components that interact with each other. The main idea of MFT is to replace all interactions to any one body with an average or effective interaction, sometimes called a ''molecular field''. This reduces any many-body problem into an effective one-body problem. The ease of solving MFT problems means that some insight into the behavior of the system can be obtained at a lower computational cost. MFT has since been applied to a wide range of fields outside of physics, including statistical inference, graphical models, neuroscience, artificial intelligence, epidemic models, queueing theory, computer-network p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Experimental Economics
Experimental economics is the application of experimental methods to study economic questions. Data collected in experiments are used to estimate effect size, test the validity of economic theories, and illuminate market mechanisms. Economic experiments usually use cash to motivate subjects, in order to mimic real-world incentives. Experiments are used to help understand how and why markets and other exchange systems function as they do. Experimental economics have also expanded to understand institutions and the law (experimental law and economics). A fundamental aspect of the subject is design of experiments. Experiments may be conducted in the field or in laboratory settings, whether of individual or group behavior. Variants of the subject outside such formal confines include natural and quasi-natural experiments. Experimental topics One can loosely classify economic experiments using the following topics: * Markets * Games * Evolutionary game theory * Decision making * Barg ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Logit Equilibrium
Quantal response equilibrium (QRE) is a solution concept in game theory. First introduced by Richard McKelvey and Thomas Palfrey, it provides an equilibrium notion with bounded rationality. QRE is not an equilibrium refinement, and it can give significantly different results from Nash equilibrium. QRE is only defined for games with discrete strategies, although there are continuous-strategy analogues. In a quantal response equilibrium, players are assumed to make errors in choosing which pure strategy to play. The probability of any particular strategy being chosen is positively related to the payoff from that strategy. In other words, very costly errors are unlikely. The equilibrium arises from the realization of beliefs. A player's payoffs are computed based on beliefs about other players' probability distribution over strategies. In equilibrium, a player's beliefs are correct. Application to data When analyzing data from the play of actual games, particularly from laborato ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bounded Rationality
Bounded rationality is the idea that rationality is limited when individuals make decisions, and under these limitations, rational individuals will select a decision that is satisfactory rather than optimal. Limitations include the difficulty of the problem requiring a decision, the cognitive capability of the mind, and the time available to make the decision. Decision-makers, in this view, act as satisficers, seeking a satisfactory solution, with everything that they have at the moment rather than an optimal solution. Therefore, humans do not undertake a full cost-benefit analysis to determine the optimal decision, but rather, choose an option that fulfils their adequacy criteria. An example of this being within organisations when they must adhere to the operating conditions of their company, this has the opportunity to result in bounded rationality as the organisation is not able to choose the optimal option. Some models of human behavior in the social sciences assume that hu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |