|
Polar Sun Synchronous Orbit
A Sun-synchronous orbit (SSO), also called a heliosynchronous orbit, is a nearly polar orbit around a planet, in which the satellite passes over any given point of the planet's surface at the same local mean solar time. More technically, it is an orbit arranged so that it precesses through one complete revolution each year, so it always maintains the same relationship with the Sun. Applications A Sun-synchronous orbit is useful for imaging, reconnaissance, and weather satellites, because every time that the satellite is overhead, the surface illumination angle on the planet underneath it is nearly the same. This consistent lighting is a useful characteristic for satellites that image the Earth's surface in visible or infrared wavelengths, such as weather and spy satellites, and for other remote-sensing satellites, such as those carrying ocean and atmospheric remote-sensing instruments that require sunlight. For example, a satellite in Sun-synchronous orbit might ascend acros ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heliosynchronous Orbit
A Sun-synchronous orbit (SSO), also called a heliosynchronous orbit, is a nearly polar orbit around a planet, in which the satellite passes over any given point of the planet's surface at the same local mean solar time. More technically, it is an orbit arranged so that it precesses through one complete revolution each year, so it always maintains the same relationship with the Sun. Applications A Sun-synchronous orbit is useful for imaging, reconnaissance, and weather satellites, because every time that the satellite is overhead, the surface illumination angle on the planet underneath it is nearly the same. This consistent lighting is a useful characteristic for satellites that image the Earth's surface in visible or infrared wavelengths, such as weather and spy satellites, and for other remote-sensing satellites, such as those carrying ocean and atmospheric remote-sensing instruments that require sunlight. For example, a satellite in Sun-synchronous orbit might ascend acros ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nodal Precession
Nodal precession is the precession of the orbital plane of a satellite around the rotational axis of an astronomical body such as Earth. This precession is due to the non-spherical nature of a rotating body, which creates a non-uniform gravitational field. The following discussion relates to low Earth orbit of artificial satellites, which have no measurable effect on the motion of Earth. The nodal precession of more massive, natural satellites like the Moon is more complex. Around a spherical body, an orbital plane would remain fixed in space around the gravitational primary body. However, most bodies rotate, which causes an equatorial bulge. This bulge creates a gravitational effect that causes orbits to precess around the rotational axis of the primary body. The direction of precession is opposite the direction of revolution. For a typical prograde orbit around Earth (that is, in the direction of primary body's rotation), the longitude of the ascending node decreases, that is the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Radian
The radian, denoted by the symbol rad, is the unit of angle in the International System of Units (SI) and is the standard unit of angular measure used in many areas of mathematics. The unit was formerly an SI supplementary unit (before that category was abolished in 1995). The radian is defined in the SI as being a dimensionless unit, with 1 rad = 1. Its symbol is accordingly often omitted, especially in mathematical writing. Definition One radian is defined as the angle subtended from the center of a circle which intercepts an arc equal in length to the radius of the circle. More generally, the magnitude in radians of a subtended angle is equal to the ratio of the arc length to the radius of the circle; that is, \theta = \frac, where is the subtended angle in radians, is arc length, and is radius. A right angle is exactly \frac radians. The rotation angle (360°) corresponding to one complete revolution is the length of the circumference divided by the radius, which i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sidereal Year
A sidereal year (, ; ), also called a sidereal orbital period, is the time that Earth or another planetary body takes to orbit the Sun once with respect to the fixed stars. Hence, for Earth, it is also the time taken for the Sun to return to the same position relative to Earth with respect to the fixed stars after apparently travelling once around the ecliptic. It equals for the J2000.0 epoch. The sidereal year differs from the solar year, "the period of time required for the ecliptic longitude of the Sun to increase 360 degrees", due to the precession of the equinoxes. The sidereal year is 20 min 24.5 s longer than the mean tropical year at J2000.0 . At present, the rate of axial precession corresponds to a period of 25,772 years, so sidereal year is longer than tropical year by 1,224.5 seconds (20 min 24.5 s, ~365.24219*86400/25772). Before the discovery of the precession of the equinoxes by Hipparchus in the Hellenistic period, the difference between sidereal and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
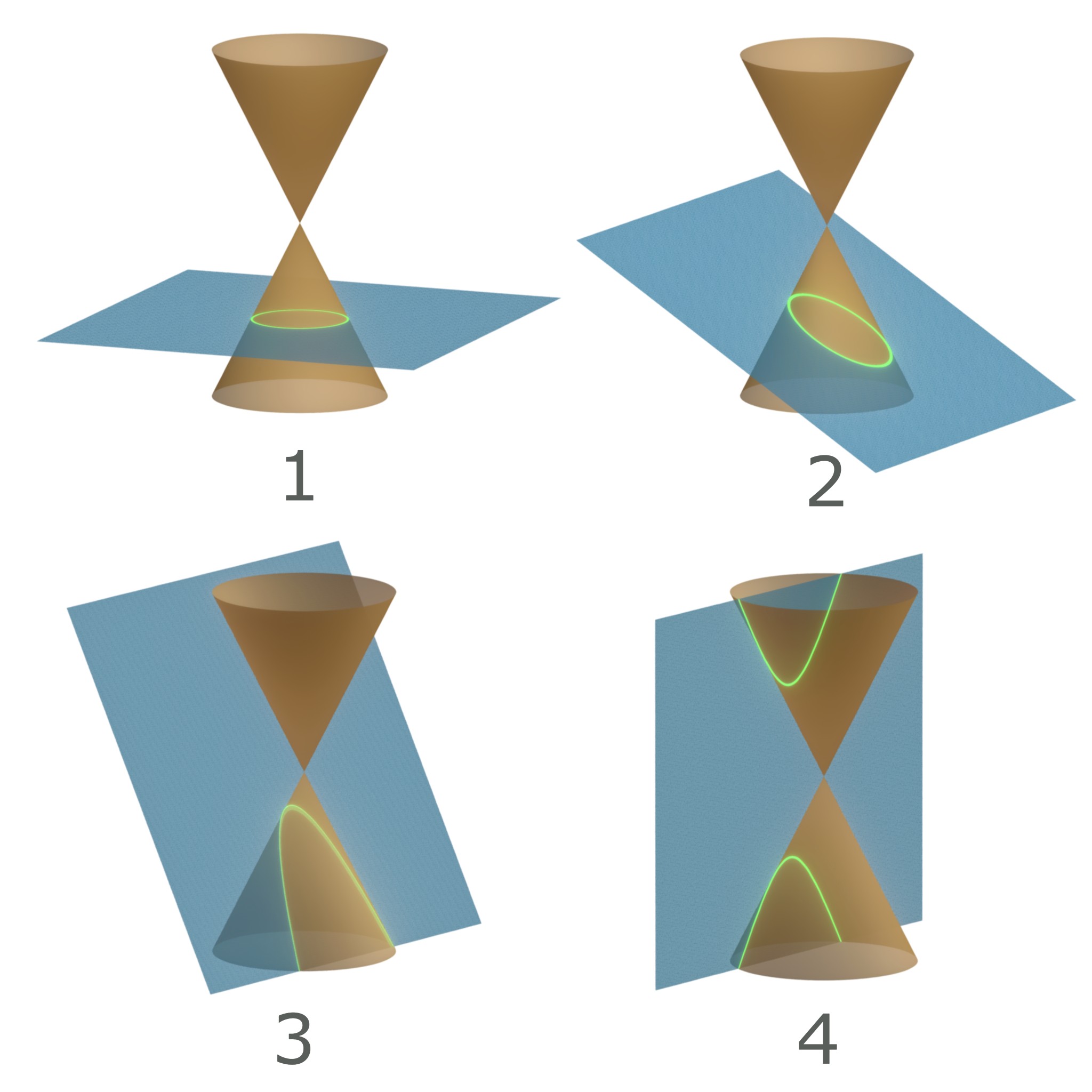
Conic Section
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Oblateness
Flattening is a measure of the compression of a circle or sphere along a diameter to form an ellipse or an ellipsoid of revolution (spheroid) respectively. Other terms used are ellipticity, or oblateness. The usual notation for flattening is and its definition in terms of the semi-axes of the resulting ellipse or ellipsoid is :: \mathrm = f =\frac . The ''compression factor'' is \frac\,\! in each case; for the ellipse, this is also its aspect ratio. Definitions There are three variants of flattening; when it is necessary to avoid confusion, the main flattening is called the first flattening.Torge, W. (2001). ''Geodesy'' (3rd edition). de Gruyter. and online web textsOsborne, P. (2008). The Mercator Projections'' Chapter 5.Rapp, Richard H. (1991). ''Geometric Geodesy, Part I''. Dept. of Geodetic Science and Surveying, Ohio State Univ., Columbus, Ohio/ref> In the following, is the larger dimension (e.g. semimajor axis), whereas is the smaller (semiminor axis). All flatt ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geopotential Model
In geophysics and physical geodesy, a geopotential model is the theoretical analysis of measuring and calculating the effects of Earth's gravitational field (the geopotential). Newton's law Newton's law of universal gravitation states that the gravitational force ''F'' acting between two point masses ''m''1 and ''m''2 with centre of mass separation ''r'' is given by :\mathbf = - G \frac\mathbf where ''G'' is the gravitational constant and r̂ is the radial unit vector. For a non-pointlike object of continuous mass distribution, each mass element ''dm'' can be treated as mass distributed over a small volume, so the volume integral over the extent of object 2 gives: with corresponding gravitational potential where ρ = ρ(''x'', ''y'', ''z'') is the mass density at the volume element and of the direction from the volume element to point mass 1. u is the gravitational potential energy per unit mass. The case of a homogeneous sphere In the special case of a sphere with a s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Venus
Venus is the second planet from the Sun. It is sometimes called Earth's "sister" or "twin" planet as it is almost as large and has a similar composition. As an interior planet to Earth, Venus (like Mercury) appears in Earth's sky never far from the Sun, either as morning star or evening star. Aside from the Sun and Moon, Venus is the brightest natural object in Earth's sky, capable of casting visible shadows on Earth at dark conditions and being visible to the naked eye in broad daylight. Venus is the second largest terrestrial object of the Solar System. It has a surface gravity slightly lower than on Earth and has a very weak induced magnetosphere. The atmosphere of Venus, mainly consists of carbon dioxide, and is the densest and hottest of the four terrestrial planets at the surface. With an atmospheric pressure at the planet's surface of about 92 times the sea level pressure of Earth and a mean temperature of , the carbon dioxide gas at Venus's surface is in the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mars
Mars is the fourth planet from the Sun and the second-smallest planet in the Solar System, only being larger than Mercury (planet), Mercury. In the English language, Mars is named for the Mars (mythology), Roman god of war. Mars is a terrestrial planet with a thin atmosphere (less than 1% that of Earth's), and has a crust primarily composed of elements similar to Earth's crust, as well as a core made of iron and nickel. Mars has surface features such as impact craters, valleys, dunes and polar ice caps. It has two small and irregularly shaped moons, Phobos (moon), Phobos and Deimos (moon), Deimos. Some of the most notable surface features on Mars include Olympus Mons, the largest volcano and List of tallest mountains in the Solar System, highest known mountain in the Solar System and Valles Marineris, one of the largest canyons in the Solar System. The North Polar Basin (Mars), Borealis basin in the Northern Hemisphere covers approximately 40% of the planet and may be a la ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Oblate Spheroid
A spheroid, also known as an ellipsoid of revolution or rotational ellipsoid, is a quadric surface obtained by rotating an ellipse about one of its principal axes; in other words, an ellipsoid with two equal semi-diameters. A spheroid has circular symmetry. If the ellipse is rotated about its major axis, the result is a ''prolate spheroid'', elongated like a rugby ball. The American football is similar but has a pointier end than a spheroid could. If the ellipse is rotated about its minor axis, the result is an ''oblate spheroid'', flattened like a lentil or a plain M&M. If the generating ellipse is a circle, the result is a sphere. Due to the combined effects of gravity and rotation, the figure of the Earth (and of all planets) is not quite a sphere, but instead is slightly flattened in the direction of its axis of rotation. For that reason, in cartography and geodesy the Earth is often approximated by an oblate spheroid, known as the reference ellipsoid, instead of a spher ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Retrograde Motion
Retrograde motion in astronomy is, in general, orbital or rotational motion of an object in the direction opposite the rotation of its primary, that is, the central object (right figure). It may also describe other motions such as precession or nutation of an object's rotational axis. Prograde or direct motion is more normal motion in the same direction as the primary rotates. However, "retrograde" and "prograde" can also refer to an object other than the primary if so described. The direction of rotation is determined by an inertial frame of reference, such as distant fixed stars. In the Solar System, the orbits around the Sun of all planets and most other objects, except many comets, are prograde. They orbit around the Sun in the same direction as the sun rotates about its axis, which is counterclockwise when observed from above the Sun's north pole. Except for Venus and Uranus, planetary rotations around their axes are also prograde. Most natural satellites have prograde or ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Minute
The minute is a unit of time usually equal to (the first sexagesimal fraction) of an hour, or 60 seconds. In the UTC time standard, a minute on rare occasions has 61 seconds, a consequence of leap seconds (there is a provision to insert a negative leap second, which would result in a 59-second minute, but this has never happened in more than 40 years under this system). Although not an SI unit, the minute is accepted for use with SI units. The SI symbol for ''minute'' or ''minutes'' is min (without a dot). The prime symbol is also sometimes used informally to denote minutes of time. History Al-Biruni first subdivided the hour sexagesimally into minutes, seconds, thirds and fourths in 1000 CE while discussing Jewish months. Historically, the word "minute" comes from the Latin ''pars minuta prima'', meaning "first small part". This division of the hour can be further refined with a "second small part" (Latin: ''pars minuta secunda''), and this is where the word "second" comes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |


.jpg)