|
Pi-system
In mathematics, a -system (or pi-system) on a set \Omega is a collection P of certain subsets of \Omega, such that * P is non-empty. * If A, B \in P then A \cap B \in P. That is, P is a non-empty family of subsets of \Omega that is closed under non-empty finite intersections.The nullary (0-ary) intersection of subsets of \Omega is by convention equal to \Omega, which is not required to be an element of a -system. The importance of -systems arises from the fact that if two probability measures agree on a -system, then they agree on the -algebra generated by that -system. Moreover, if other properties, such as equality of integrals, hold for the -system, then they hold for the generated -algebra as well. This is the case whenever the collection of subsets for which the property holds is a -system. -systems are also useful for checking independence of random variables. This is desirable because in practice, -systems are often simpler to work with than -algebras. For example, i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Finite Intersection Property
In general topology, a branch of mathematics, a non-empty family A of subsets of a set X is said to have the finite intersection property (FIP) if the intersection over any finite subcollection of A is non-empty. It has the strong finite intersection property (SFIP) if the intersection over any finite subcollection of A is infinite. Sets with the finite intersection property are also called centered systems and filter subbases. The finite intersection property can be used to reformulate topological compactness in terms of closed sets; this is its most prominent application. Other applications include proving that certain perfect sets are uncountable, and the construction of ultrafilters. Definition Let X be a set and \mathcal a nonempty family of subsets of that is, \mathcal is a nonempty subset of the power set of Then \mathcal is said to have the finite intersection property if every nonempty finite subfamily has nonempty intersection; it is said to have the strong ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Filter (set Theory)
In mathematics, a filter on a set X is a family \mathcal of subsets such that: # X \in \mathcal and \emptyset \notin \mathcal # if A\in \mathcal and B \in \mathcal, then A\cap B\in \mathcal # If A\subset B\subset X and A\in \mathcal, then B\in \mathcal A filter on a set may be thought of as representing a "collection of large subsets", one intuitive example being the neighborhood filter. Filters appear in order theory, model theory, and set theory, but can also be found in topology, from which they originate. The dual notion of a filter is an ideal. Filters were introduced by Henri Cartan in 1937 and as described in the article dedicated to filters in topology, they were subsequently used by Nicolas Bourbaki in their book '' Topologie Générale'' as an alternative to the related notion of a net developed in 1922 by E. H. Moore and Herman L. Smith. Order filters are generalizations of filters from sets to arbitrary partially ordered sets. Specifically, a filter on a s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Dynkin System
A Dynkin system, named after Eugene Dynkin, is a collection of subsets of another universal set \Omega satisfying a set of axioms weaker than those of -algebra. Dynkin systems are sometimes referred to as -systems (Dynkin himself used this term) or d-system. These set families have applications in measure theory and probability. A major application of -systems is the - theorem, see below. Definition Let \Omega be a nonempty set, and let D be a collection of subsets of \Omega (that is, D is a subset of the power set of \Omega). Then D is a Dynkin system if # \Omega \in D; # D is closed under complements of subsets in supersets: if A, B \in D and A \subseteq B, then B \setminus A \in D; # D is closed under countable increasing unions: if A_1 \subseteq A_2 \subseteq A_3 \subseteq \cdots is an increasing sequenceA sequence of sets A_1, A_2, A_3, \ldots is called if A_n \subseteq A_ for all n \geq 1. of sets in D then \bigcup_^\infty A_n \in D. It is easy to check that any Dyn ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Monotone Class Theorem
In measure theory and probability, the monotone class theorem connects monotone classes and -algebras. The theorem says that the smallest monotone class containing an algebra of sets G is precisely the smallest -algebra containing G. It is used as a type of transfinite induction to prove many other theorems, such as Fubini's theorem. Definition of a monotone class A is a family (i.e. class) M of sets that is closed under countable monotone unions and also under countable monotone intersections. Explicitly, this means M has the following properties: # if A_1, A_2, \ldots \in M and A_1 \subseteq A_2 \subseteq \cdots then A_i \in M, and # if B_1, B_2, \ldots \in M and B_1 \supseteq B_2 \supseteq \cdots then B_i \in M. Monotone class theorem for sets Monotone class theorem for functions Proof The following argument originates in Rick Durrett's Probability: Theory and Examples. Results and applications As a corollary, if G is a ring of sets (The) Ring(s) may re ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Family Of Sets
In set theory and related branches of mathematics, a family (or collection) can mean, depending upon the context, any of the following: set, indexed set, multiset, or class. A collection F of subsets of a given set S is called a family of subsets of S, or a family of sets over S. More generally, a collection of any sets whatsoever is called a family of sets, set family, or a set system. Additionally, a family of sets may be defined as a function from a set I, known as the index set, to F, in which case the sets of the family are indexed by members of I. In some contexts, a family of sets may be allowed to contain repeated copies of any given member, and in other contexts it may form a proper class. A finite family of subsets of a finite set S is also called a '' hypergraph''. The subject of extremal set theory concerns the largest and smallest examples of families of sets satisfying certain restrictions. Examples The set of all subsets of a given set S is called the pow ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Random Variable
A random variable (also called random quantity, aleatory variable, or stochastic variable) is a Mathematics, mathematical formalization of a quantity or object which depends on randomness, random events. The term 'random variable' in its mathematical definition refers to neither randomness nor variability but instead is a mathematical function (mathematics), function in which * the Domain of a function, domain is the set of possible Outcome (probability), outcomes in a sample space (e.g. the set \ which are the possible upper sides of a flipped coin heads H or tails T as the result from tossing a coin); and * the Range of a function, range is a measurable space (e.g. corresponding to the domain above, the range might be the set \ if say heads H mapped to -1 and T mapped to 1). Typically, the range of a random variable is a subset of the Real number, real numbers. Informally, randomness typically represents some fundamental element of chance, such as in the roll of a dice, d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Probability Distribution
In probability theory and statistics, a probability distribution is a Function (mathematics), function that gives the probabilities of occurrence of possible events for an Experiment (probability theory), experiment. It is a mathematical description of a Randomness, random phenomenon in terms of its sample space and the Probability, probabilities of Event (probability theory), events (subsets of the sample space). For instance, if is used to denote the outcome of a coin toss ("the experiment"), then the probability distribution of would take the value 0.5 (1 in 2 or 1/2) for , and 0.5 for (assuming that fair coin, the coin is fair). More commonly, probability distributions are used to compare the relative occurrence of many different random values. Probability distributions can be defined in different ways and for discrete or for continuous variables. Distributions with special properties or for especially important applications are given specific names. Introduction A prob ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Eugene Dynkin
Eugene Borisovich Dynkin (; 11 May 1924 – 14 November 2014) was a Soviet and American mathematician. He made contributions to the fields of probability and algebra, especially semisimple Lie groups, Lie algebras, and Markov processes. The Dynkin diagram, the Dynkin system, and Dynkin's lemma are named after him. Biography Dynkin was born into a Jewish family, living in Leningrad until 1935, when his family was exiled to Kazakhstan. Two years later, when Dynkin was 13, his father disappeared in the Gulag. Moscow University At the age of 16, in 1940, Dynkin was admitted to Moscow University. He avoided military service in World War II because of his poor eyesight, and received his MS in 1945 and his PhD in 1948. He became an assistant professor at Moscow, but was not awarded a "chair" until 1954 because of his political undesirability. His academic progress was made difficult due to his father's fate, as well as Dynkin's Jewish origin; the special efforts of Andrey Kolmogoro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Independence (probability Theory)
Independence is a fundamental notion in probability theory, as in statistics and the theory of stochastic processes. Two event (probability theory), events are independent, statistically independent, or stochastically independent if, informally speaking, the occurrence of one does not affect the probability of occurrence of the other or, equivalently, does not affect the odds. Similarly, two random variables are independent if the realization of one does not affect the probability distribution of the other. When dealing with collections of more than two events, two notions of independence need to be distinguished. The events are called Pairwise independence, pairwise independent if any two events in the collection are independent of each other, while mutual independence (or collective independence) of events means, informally speaking, that each event is independent of any combination of other events in the collection. A similar notion exists for collections of random variables. M ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
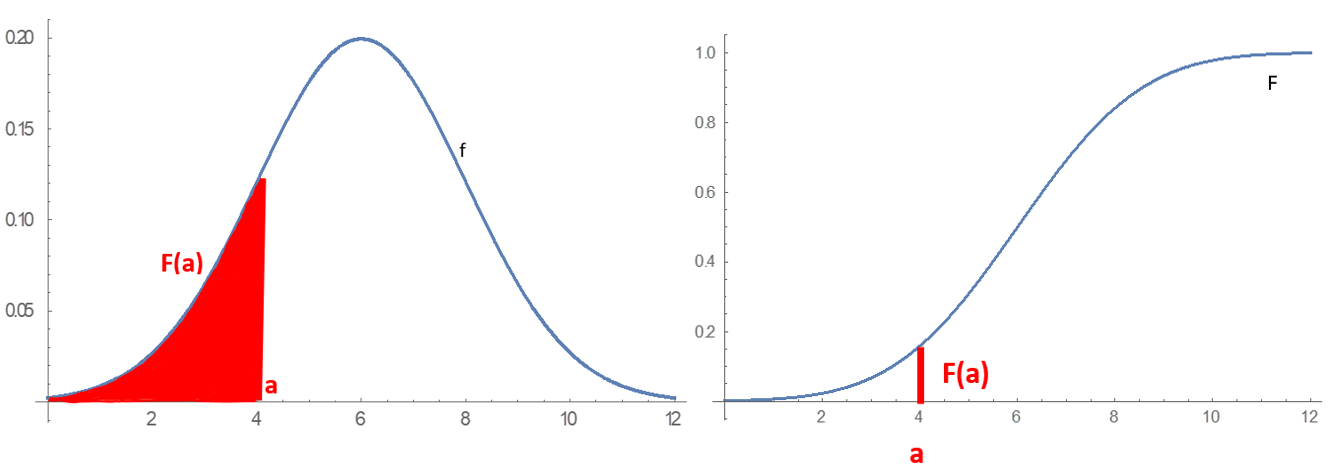
Cumulative Distribution Function
In probability theory and statistics, the cumulative distribution function (CDF) of a real-valued random variable X, or just distribution function of X, evaluated at x, is the probability that X will take a value less than or equal to x. Every probability distribution Support (measure theory), supported on the real numbers, discrete or "mixed" as well as Continuous variable, continuous, is uniquely identified by a right-continuous Monotonic function, monotone increasing function (a càdlàg function) F \colon \mathbb R \rightarrow [0,1] satisfying \lim_F(x)=0 and \lim_F(x)=1. In the case of a scalar continuous distribution, it gives the area under the probability density function from negative infinity to x. Cumulative distribution functions are also used to specify the distribution of multivariate random variables. Definition The cumulative distribution function of a real-valued random variable X is the function given by where the right-hand side represents the probability ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |